Coburn J.W., Herdlick J.D. College Algebra: Graphs and Models

Подождите немного. Документ загружается.

840 CHAPTER 9 Additional Topics in Algebra 9–80

College Algebra G&M—

30. 31. 32.

33. Sumpter reservoir contains 121,500 ft

of water and is being drained in the following way. Each day one-third of

the water is drained (and not replaced). Use a sequence/series to compute how much water remains in the pond

after 7 days.

34. Credit-hours taught at Cody Community College have been increasing at 7% per year since it opened in 2001 and

taught 1225 credit-hours. For the new faculty, the college needs to predict the number of credit-hours that will be

taught in 2015. Use a sequence/series to compute the credit-hours for 2015 and to ﬁnd the total number of credit

hours taught through the 2015 school year.

SECTION 9.4 Mathematical Induction

KEY CONCEPTS

•

Functions written in subscript notation can be evaluated, graphed, and composed with other functions.

•

A sum formula involving only natural numbers n as inputs can be proven valid using a proof by induction. Given

that S

represents a sum formula involving natural numbers, if (1) S

is true and (2) then S

must be true for all natural numbers.

•

Proof by induction can also be used to validate other relationships, using a more general statement of the

principle. Let P

be a statement involving the natural numbers n. If (1) P

is true (P

for and (2) the truth

of P

implies that is also true, then P

must be true for all natural numbers n.

EXERCISES

Use the principle of mathematical induction to prove the indicated sum formula is true for all natural numbers n.

35. 36.

and and

Use the principle of mathematical induction to prove that each statement is true for all natural numbers n.

37. 38. 39. is divisible by 2

SECTION 9.5 Counting Techniques

KEY CONCEPTS

•

An experiment is any task that can be repeated and has a well-deﬁned set of possible outcomes.

•

Each repetition of an experiment is called a trial.

•

Any potential outcome of an experiment is called a sample outcome.

•

The set of all sample outcomes is called the sample space.

•

An experiment with N(equally likely) sample outcomes that is repeated t times, has a sample space with N

elements.

•

If a sample outcome can be used more than once, the counting is said to be with repetition. If a sample outcome

can be used only once, the counting is said to be without repetition.

•

The fundamental principle of counting states: If there are p possibilities for a ﬁrst task, q possibilities for the

second, and r possibilities for the third, the total number of ways the experiment can be completed is pqr. This

fundamental principle can be extended to include any number of tasks.

•

If the elements of a sample space have precedence or priority (order or rank is important), the number of elements is

counted using a permutation, denoted

and read, “the distinguishable permutations of n objects taken r at a time.”

•

To expand

, we can write out the ﬁrst r factors of n! or use the formula

⫽

1n ⫺ r2!

⫺ 16

n⫺1

ⱕ 7

⫺ 14

ⱖ 3n ⫹ 1

⫽

n1n ⫹ 1212n ⫹ 12

⫽ n

⫽

n1n ⫹ 12

⫽ n

1 ⫹ 4 ⫹ 9 ⫹ 16 ⫹ 25 ⫹ 36 ⫹

⫹ n

;1 ⫹ 2 ⫹ 3 ⫹ 4 ⫹ 5 ⫹

⫹ n;

k⫹1

n ⫽ 12

⫹ a

k⫹1

⫽ S

k⫹1

兺

k⫽1

兺

k⫽1

12a

兺

k⫽1

5 a

cob19545_ch09_838-848.qxd 11/10/10 7:34 AM Page 840

9–81 Summary and Concept Review 841

College Algebra G&M—

•

If any of the sample outcomes are identical, certain permutations will be nondistinguishable. In a set containing n

elements where one element is repeated p times, another is repeated q times, and another r times

the number of distinguishable permutations is given by

•

If the elements of a set have no rank, order, or precedence (as in a committee of colleagues) permutations with the

same elements are considered identical. The result is the number of combinations,

EXERCISES

40. Three slips of paper with the letters A, B, and C are placed in a box and randomly drawn one at a time. Show all

possible ways they can be drawn using a tree diagram.

41. The combination for a certain bicycle lock consists of three digits. How many combinations are possible if

(a) repetition of digits is not allowed and (b) repetition of digits is allowed.

42. Jethro has three work shirts, four pairs of work pants, and two pairs of work shoes. How many different ways can

he dress himself (shirt, pants, shoes) for a day’s work?

43. From a ﬁeld of 12 contestants in a pet show, three cats are chosen at random to be photographed for a publicity

poster. In how many different ways can the cats be chosen?

44. Compute the following values by hand, showing all work:

a. 7! b.

45. Six horses are competing in a race at the McClintock Ranch. Assuming there are no ties, (a) how many different ways

can the horses ﬁnish the race? (b) How many different ways can the horses ﬁnish ﬁrst, second, and third place?

ﬁnish ﬁrst?

46. How many distinguishable permutations can be formed from the letters in the word “tomorrow”?

47. Quality Construction Company has 12 equally talented employees. (a) How many ways can a three-member crew

be formed to complete a small job? (b) If the company is in need of a Foreman, Assistant Foreman, and Crew

Chief, in how many ways can the positions be ﬁlled?

SECTION 9.6 Introduction to Probability

KEY CONCEPTS

•

An event E is any designated set of sample outcomes.

•

Given S is a sample space of equally likely sample outcomes and E is an event relative to S, the probability of E,

written P(E), is computed as where n(E) represents the number of elements in E, and n(S)

represents the number of elements in S.

•

The complement of an event E is the set of sample outcomes in S, but not in E and is denoted

•

Given sample space S and any event E deﬁned relative to S:

and

•

Two events that have no outcomes in common are said to be mutually exclusive.

•

If two events are not mutually exclusive,

•

If two events are mutually exclusive, .

EXERCISES

48. One card is drawn from a standard deck. What is the probability the card is a ten or a face card?

49. One card is drawn from a standard deck. What is the probability the card is a Queen or a face card?

50. One die is rolled. What is the probability the result is not a three?

51. Given and compute P1E

2.P1E

2⫽

,P1E

2⫽

, P1E

2⫽

P1E

or E

2S P1E

´ E

2⫽ P1E

2⫹ P1E

P1E

or E

2S P1E

´ E

2⫽ P1E

2⫹ P1E

2⫺ P1E

¨ E

152 P1E2⫹ P1⬃E2⫽ 1.

112 P1⬃S2⫽ 0,

122 0 ⱕ P1E2ⱕ 1,

132 P1S2⫽ 1,

142 P1E2⫽ 1 ⫺ P1⬃E2,

⬃E.

P1E2⫽

n1E2

n1S2

⫽

r!1n ⫺ r2!

p!q!r!

⫽

p!q!r!

1p ⫹ q ⫹ r ⫽ n2,

cob19545_ch09_838-848.qxd 11/10/10 7:34 AM Page 841

842 CHAPTER 9 Additional Topics in Algebra 9–82

College Algebra G&M—

52. Find P(E) given that and

53. To determine if more physicians should be hired, a medical clinic tracks the number

of days between a patient’s request for an appointment and the actual appointment

date. The table given shows the probability that a patient must wait d days. Based

on the table, what is the probability a patient must wait

a. at least 20 days b. less than 20 days

c. 40 days or less d. over 40 days

e. less than 40 and more than 10 days f. 30 or more days

SECTION 9.7 The Binomial Theorem

KEY CONCEPTS

•

To expand for n of “moderate size,” we can use Pascal’s triangle and observed patterns.

•

For any natural numbers n and r, where the expression (read “n choose r”) is called the binomial

coefﬁcient and evaluated as

•

If n is large, it is more efﬁcient to expand using the binomial coefﬁcients and binomial theorem.

•

The following binomial coefﬁcients are useful/common and should be committed to memory:

•

We deﬁne for example

•

The binomial theorem:

•

The kth term of can be found using the formula where

EXERCISES

54. Evaluate each of the following: 55. Use Pascal’s triangle to expand the expressions:

a. b. a. b.

Use the binomial theorem to:

56. Write the ﬁrst four terms of 57. Find the indicated term of each expansion.

a. b. a. fourth b. ; 10th

58. Mark Leland is a professional bowler who is able to roll a strike (knocking down all 10 pins on the ﬁrst ball) 91% of

the time. (a) What is the probability he rolls at least four strikes in the ﬁrst ﬁve frames? (b) What is the probability he

rolls ﬁve strikes (and scares the competition)?

12a ⫺ b2

1x ⫹ 2y2

;15a ⫹ 2b2

1a ⫹ 132

11 ⫹ 2i2

1x ⫺ y2

r ⫽ k ⫺ 1.a

n⫺r

,1a ⫹ b2

1a ⫹ b2

⫽ a

b a

⫹ a

b a

n⫺1

⫹ a

b a

n⫺2

⫹

⫹ a

n ⫺ 1

b a

n⫺1

⫹ a

b a

b⫽

n!1n ⫺ n2!

⫽

⫽ 1.0! ⫽ 1;

b⫽ 1

b⫽ n

n ⫺ 1

b⫽ n

b⫽ 1

b⫽

r!1n ⫺ r2!

bn ⱖ r,

1a ⫹ b2

n1S2⫽

.n1E2⫽

Wait (days d) Probability

0 0.002

0.07

0.32

0.43

0.17830 ⱕ d 6 40

20 ⱕ d 6 30

10 ⱕ d 6 20

0 6 d 6 10

1. The general term of a sequence is given. Find the

ﬁrst four terms and the 8th term.

a. b.

c. a

⫽ e

⫽ 3

n⫹1

⫽ 21a

⫺ 1

⫽

1n ⫹ 22!

⫽

n ⫹ 3

2. Expand each series and evaluate.

a. b.

c. d.

兺

k⫽1

兺

j⫽1

1⫺22a

兺

j⫽2

1⫺12

j ⫹ 1

兺

k⫽2

12k

⫺ 32

PRACTICE TEST

cob19545_ch09_838-848.qxd 12/17/10 1:07 AM Page 842

9–83 Practice Test 843

College Algebra G&M—

3. Identify the ﬁrst term and the common difference or

common ratio. Then ﬁnd the general term a

a. 7, 4, 1, b.

c. 4, 16, d. 10, 4,

4. Find the indicated value for each sequence.

a. ﬁnd a

b. ﬁnd n

c. ﬁnd a

d. ﬁnd n

5. Find the sum of each series.

c. For ﬁnd S

6. Each swing of a pendulum (in one direction) is 95%

of the previous one. If the ﬁrst swing is 12 ft, (a) ﬁnd

the length of the seventh swing and (b) determine

the distance traveled by the pendulum for the ﬁrst

seven swings.

7. A rare coin that cost $3000 appreciates in value 7%

per year. Find the value after 12 yr.

8. A car that costs $50,000 decreases in value by 15% per

year. Find the value of the car after 5 yr.

9. Use mathematical induction to verify that for

the sum formula is true

for all natural numbers n.

10. Use the principle of mathematical induction to

verify that is true for all

natural numbers n.

11. Three colored balls (aqua, brown, and creme) are to

be drawn without replacement from a bag. List all

possible ways they can be drawn using (a) a tree

diagram and (b) an organized list.

12. Suppose that license plates for motorcycles must

consist of three numbers followed by two letters. How

many license plates are possible if zero and “Z”

cannot be used and no repetition is allowed?

13. If one icon is randomly chosen from the

following set, ﬁnd the probability a mailbox is not

chosen: {,,,,,}.

14. Compute the following values by hand, showing all

work: (a) 6! (b)

(c)

15. An English major has built a collection of rare books

that includes two identical copies of The Canterbury

Tales (Chaucer), three identical copies of Romeo and

: 2

n⫺1

ⱕ 3

⫺ 1

⫽

⫺ n

⫽ 5n ⫺ 3,

6 ⫹ 3 ⫹

⫹

4 ⫺ 12 ⫹ 36 ⫺ 108 ⫹

兺

k⫽1

13k ⫹ 22

7 ⫹ 10 ⫹ 13 ⫹

⫹ 100

⫽⫺2, a

⫽ 486, r ⫽⫺3;

⫽ 24, r ⫽

;

⫽ 2, a

⫽⫺22, d ⫽⫺3;

⫽ 4, d ⫽ 5;

,p⫺32,⫺8,

p⫺8, ⫺6, ⫺4, ⫺2,p⫺2,

Juliet (Shakespeare), four identical copies of Faustus

(Marlowe), and four identical copies of The Faerie

Queen (Spenser). If these books are to be arranged

on a shelf, how many distinguishable permutations

are possible?

16. A company specializes in marketing various

cornucopia (traditionally a curved horn overﬂowing

with fruit, vegetables, gourds, and ears of grain) for

Thanksgiving table settings. The company has seven

fruit, six vegetable, ﬁve gourd, and four grain

varieties available. If two from each group (without

repetition) are used to ﬁll the horn, how many

different cornucopia are possible?

17. Use Pascal’s triangle to expand/simplify:

a. b.

18. Use the binomial theorem to write the ﬁrst three

terms of (a) and (b) .

19. Michael and Mitchell are attempting to make a

nonstop, 100-mi trip on a tandem bicycle. The

probability that Michael cannot continue pedaling

for the entire trip is 0.02. The probability that

Mitchell cannot continue pedaling for the entire trip

is 0.018. The probability that neither one can pedal

the entire trip is 0.011. What is the probability that

they complete the trip?

20. The spinner shown is spun once.

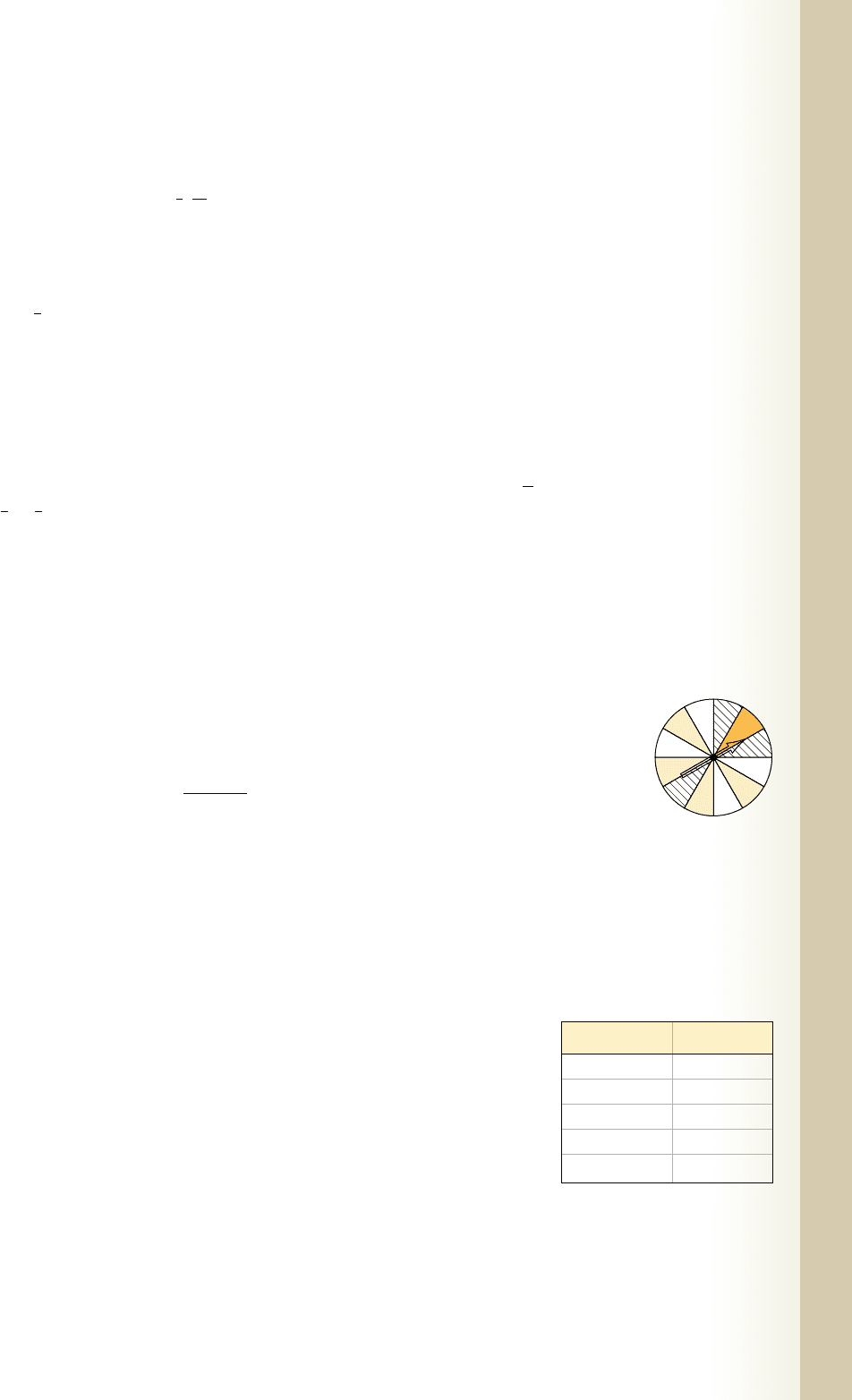
What is the probability of spinning

a. a striped wedge

b. a shaded wedge

c. a clear wedge

d. an even number

e. a two or an odd number

f. a number greater than nine

g. a shaded wedge or a number greater than 12

h. a shaded wedge and a number greater than 12

21. To improve customer service, a cable company

tracks the number of days a customer must wait until

their cable service is

installed. The table

shows the probability

that a customer must

wait d days. Based on

the table, what is the

probability a customer

waits

a. at least 2 days b. less than 2 days

c. 4 days or less d. over 4 days

e. less than 2 or at least 3 days

f. three or more days

1a ⫺ 2b

1x ⫹ 122

11 ⫹ i2

1x ⫺ 2y2

Wait (days d) Probability

0 0.02

0.30

0.60

0.05

0.033 ⱕ d 6 4

2 ⱕ d 6 3

1 ⱕ d 6 2

0 6 d 6 1

cob19545_ch09_838-848.qxd 12/17/10 1:07 AM Page 843

844 CHAPTER 9 Additional Topics in Algebra 9–84

College Algebra G&M—

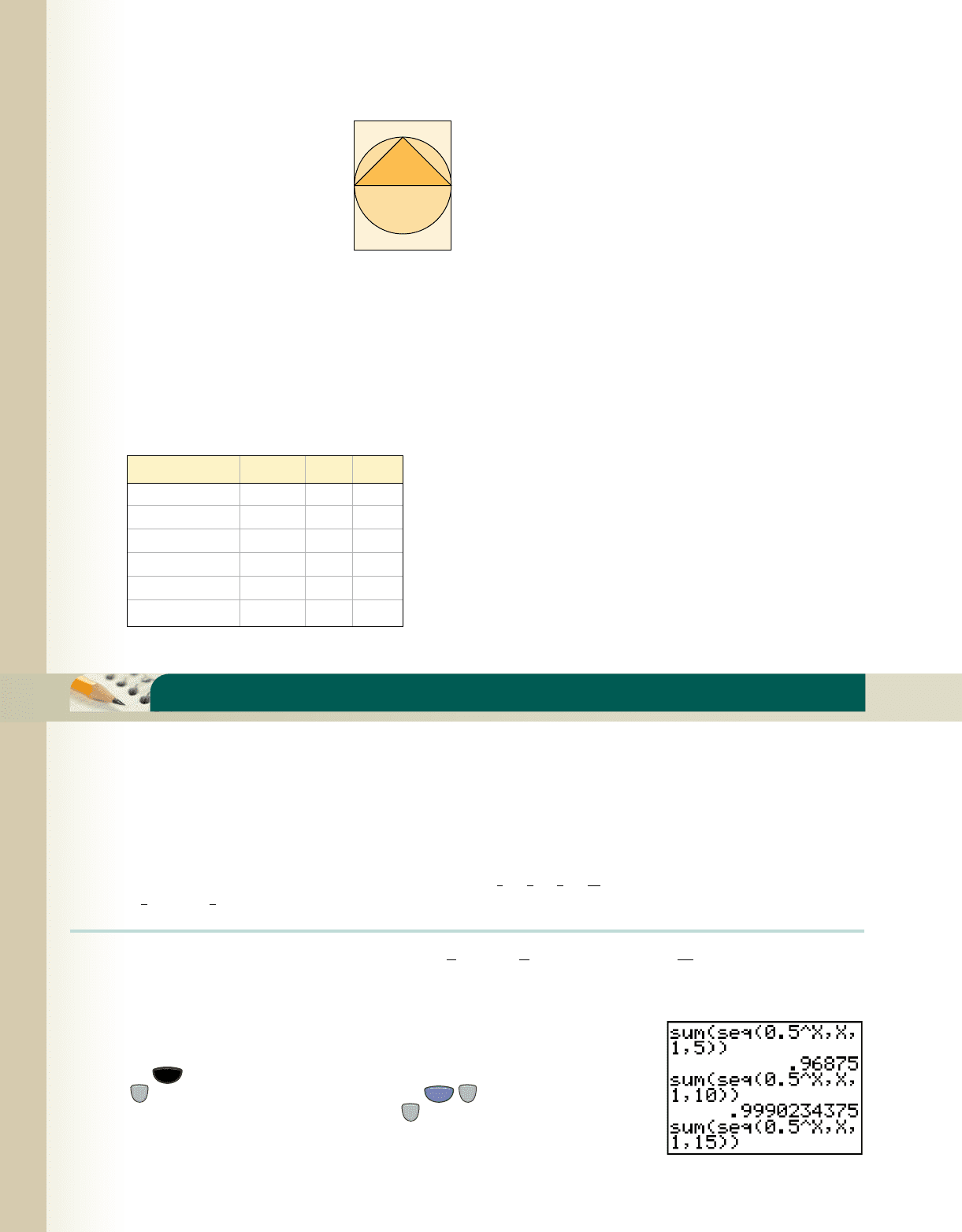
22. An experienced archer can hit

the rectangular target shown

100% of the time at a range

of 75 m. Assuming the

probability the target is hit is

related to its area, what is the

probability the archer hits

within the

a. triangle b. circle

c. circle but outside the triangle

d. lower half-circle

e. rectangle but outside the circle

f. lower half-rectangle, outside the circle

23. A survey of 100 union workers was taken to register

concerns to be raised at the next bargaining session.

A breakdown of those surveyed is shown in the table

in the right column. One out of the hundred will be

selected at random for a personal interview. What is

the probability the person chosen is a

a. woman or a craftsman

b. man or a contractor

c. man and a technician

d. journeyman or an apprentice

24. Cheddar is a 12-year-old male box turtle. Provolone

is an 8-year-old female box turtle. The probability

that Cheddar will live another 8 yr is 0.85. The

probability that Provolone will live another 8 yr is

0.95. Find the probability that

a. both turtles live for another 8 yr

b. neither turtle lives for another 8 yr

c. at least one of them will live another 8 yr

25. The quality control department at a lightbulb factory

has determined that the company is losing money

because their manufacturing process produces a

defective bulb 12% of the time. If a random sample

of 10 bulbs is tested, (a) what is the probability that

none are defective? (b) What is the probability that

no more than 3 bulbs are defective?

48 cm

64 cm

Expertise Level Women Men Total

Apprentice 16 18 34

Technician 15 13 28

Craftsman 9 9 18

Journeyman 7 6 13

Contractor 3 4 7

Totals 50 50 100

Inﬁnite Series, Finite Results

Although there were many earlier ﬂirtations with inﬁnite processes, it may have been the paradoxes of Zeno of Elea

(

.) that crystallized certain questions that simultaneously frustrated and fascinated early mathematicians. The

ﬁrst paradox, called the dichotomy paradox, can be summarized by the following question: How can one ever ﬁnish a

race, seeing that one-half the distance must ﬁrst be traversed, then one-half the remaining distance, then one-half the

distance that then remains, and so on an inﬁnite number of times? Although we easily accept that races can be ﬁnished,

the subtleties involved in this question stymied mathematicians for centuries and were not satisfactorily resolved until the

eighteenth century. In modern notation, Zeno’s ﬁrst paradox says This is a geometric series

with and

Illustration 1

䊳

For the geometric sequence with and the nth term is Use the “sum(” and

“seq(” features of your calculator to compute S

, S

, and S

(see Section 9.1). Does

the sum appear to be approaching some “limiting value?” If so, what is this value? Now

compute S

, S

, and S

. Does there still appear to be a limit to the sum? What

happens when you have the calculator compute S

Solution

䊳

the calculator and enter sum(seq (0.5^X, X, 1, 5)) on the home screen.

Pressing gives (Figure 9.69). Press to recall the expression

and overwrite the 5, changing it to a 10. Pressing shows

Repeating these steps gives and it seems that “1” may be a

limiting value. Our conjecture receives further support as S

, S

, and S

are closer

and closer to 1, but do not exceed it.

⫽ 0.9999694824,

⫽ 0.9990234375.

ENTER

2nd

⫽ 0.96875

ENTER

CLEAR

⫽

.r ⫽

⫽

r ⫽

⫽

⫹

6 1.

⬃450

CALCULATOR EXPLORATION AND DISCOVERY

Figure 9.69

cob19545_ch09_838-848.qxd 12/17/10 1:07 AM Page 844

Note that the sum of additional terms will create a longer string of 9’s. That the sum of an inﬁnite number of these

terms is 1 can be understood by converting the repeating decimal to its fractional form (as shown). For

and it follows that

For a geometric sequence, the result of an inﬁnite sum can be veriﬁed using . However, there are

many nongeometric, inﬁnite series that also have a limiting value. In some cases these require many, many more terms

before the limiting value can be observed.

Use a calculator to write the ﬁrst ﬁve terms and to ﬁnd S

, S

, and S

. Decide if the sum appears to be approaching

some limiting value, then compute S

and S

. Do these sums support your conjecture?

Exercise 1: and Exercise 2: and Exercise 3:

Additional Insight: Zeno’s ﬁrst paradox can also be “resolved” by observing that the “half-steps” needed to complete

the race require increasingly shorter (inﬁnitesimally short) amounts of time. Eventually the race is complete.

⫽

1n ⫺ 12!

r ⫽ 0.2a

⫽ 0.2r ⫽

⫽

1 ⫺ r

x ⫽

9 x ⫽

⫺x ⫽⫺0.9

10x ⫽ 9.9

x ⫽ 0.9,0.9

9–85 Strengthening Core Skills 845

College Algebra G&M—

STRENGTHENING CORE SKILLS

Probability, Quick-Counting, and Card Games

The card game known as Five Card Stud is often played for fun and relaxation, using toothpicks, beans, or pocket

change as players attempt to develop a winning “hand” from the ﬁve cards dealt. The various “hands” are given here

with the higher value hands listed ﬁrst (e.g., a full house is a better/higher hand than a ﬂush).

Five Card Hand Description Probability of Being Dealt

royal ﬂush ﬁve cards of the same suit in 0.000 001 540

sequence from 10 to Ace

straight ﬂush any ﬁve cards of the same suit in 0.000 013 900

sequence (exclude royal)

four of a kind four cards of the same rank, any

ﬁfth card

full house three cards of the same rank, with

one pair

ﬂush ﬁve cards of the same suit, no 0.001 970

sequence required

straight ﬁve cards in sequence, regardless

of suit

three of a kind three cards of the same rank, any

two other cards

two pairs two cards of the one rank, two of 0.047 500

another rank, one other card

one pair two cards of the same rank, any 0.422 600

three others

For this study, we will consider the hands that are based on suit (the ﬂushes) and the sample space to be ﬁve cards

dealt from a deck of 52, or

cob19545_ch09_838-848.qxd 11/10/10 7:57 PM Page 845

846 CHAPTER 9 Additional Topics in Algebra 9–86

College Algebra G&M—

A ﬂush consists of ﬁve cards in the same suit, a straight consists of ﬁve cards in sequence. Let’s consider that an

Ace can be used as either a high card (as in 10, J, Q, K, A) or a low card (as in A, 2, 3, 4, 5). Since the dominant

characteristic of a ﬂush is its suit, we ﬁrst consider choosing one suit of the four, then the number of ways that the

straight can be formed (if needed).

Illustration 1

䊳

What is the probability of being dealt a royal ﬂush?

Solution

䊳

For a royal ﬂush, all cards must be of one suit. Since there are four suits, it can be chosen in

ways. A

royal ﬂush must have the cards A, K, Q, J, and 10 and once the suit has been decided, it can happen in only this (one)

way or

. This means

Illustration 2

䊳

What is the probability of being dealt a straight ﬂush?

Solution

䊳

Once again all cards must be of one suit, which can be chosen in

ways. A straight ﬂush is any ﬁve

cards in sequence and once the suit has been decided, this can happen in 10 ways (Ace on down, King on down,

Queen on down, and so on). By the FCP, there are ways this can happen, but four of these will be

royal ﬂushes that are of a higher value and must be subtracted from this total. So in the intended context we have

Using these examples, determine the probability of being dealt

Exercise 1: a simple ﬂush (no royal or straight ﬂushes)

Exercise 2: three cards of the same suit and any two other (nonsuit) cards

Exercise 3: four cards of the same suit and any one other (nonsuit) card

Exercise 4: a ﬂush having no face cards

P 1straight flush2⫽

⫺ 4

⬇ 0.000 013 900

⫽ 40

P 1royal flush2⫽

⬇ 0.000 001 540.

1. Robot Moe is assembling memory cards for

computers. At 9:00

., 52 cards had been

assembled. At 11:00

., a total of 98 had been

made. Assuming the production rate is linear

a. Find the slope of this line and explain what it

means in this context.

b. Find a linear equation model for this data.

c. Determine how many cards Moe can assemble in

an eight-hour day.

d. Determine the approximate time that Moe began

work this morning.

2. Verify by direct substitution that is a

solution to

3. Solve using the quadratic formula:

State your answer in exact and

approximate form.

4. Sketch the graph of using

transformations of a tool box function. Plot

the x- and y-intercepts.

5. Write a variation equation and ﬁnd the value of k: Y

varies inversely with X and jointly with V and W. Y is

equal to 10 when and W ⫽ 12.X ⫽ 9, V ⫽ 5,

y ⫽ 1x ⫹ 4

⫺ 3

⫹ 5x ⫺ 7 ⫽ 0.

⫺ 4x ⫹ 5 ⫽ 0.

x ⫽ 2 ⫹ i

6. Graph the piecewise-deﬁned function and state the

domain and range.

7. Verify that and are

inverse functions. How are the graphs of f and g

related?

8. For the graph of g(x) shown, state where

a. b.

c. d.

e. f. local max

g. local min h.

i. g(4) j.

k. as

l. as

m. the domain of g(x)

9. Compute the difference quotient for each function

given.

a. b. h1x2⫽

x ⫺ 2

f 1x2⫽ 2x

⫺ 3x

x Sq, g1x2S

____

x S ⫺1

⫹

, g1x2S

____

g1⫺12

g1x2⫽ 2

g1x2T

g1x2cg1x27 0

g1x26 0g1x2⫽ 0

g1x2⫽ 1

x ⫹ 5f 1x2⫽ x

⫺ 5

y ⫽ •

⫺2 ⫺3 ⱕ x ⱕ⫺1

x ⫺1 6 x 6 2

2 ⱕ x ⱕ 3

CUMULATIVE REVIEW CHAPTERS R–9

5⫺5

⫺5

g(x)

cob19545_ch09_838-848.qxd 11/10/10 7:34 AM Page 846

10. Graph the polynomial function given. Clearly

indicate all intercepts.

11. Graph the rational function . Clearly

indicate all asymptotes and intercepts.

12. Write each expression in logarithmic form:

a. b.

13. Write each expression in exponential form:

a. b.

14. What interest rate is required to ensure that $2000

will double in 10 yr if interest is compounded

continuously?

15. Solve for x.

a. b.

16. Solve using matrices and row reduction:

17. Solve using a calculator and inverse matrices.

18. Find the equation of the hyperbola with foci at

and (6, 0) and vertices at and (4, 0).

19. Identify the center, vertices, and foci of the conic

section deﬁned by .

20. Use properties of sequences to determine a

and S

a. 262,144, 65,536, 16,384, 4096,

b. ,

21. Empty 55-gal drums are stacked at a storage facility

in the form of a pyramid with 52 barrels in the ﬁrst

row, 51 barrels in the second row, and so on, until

there are 10 barrels in the top row. Use properties of

sequences to determine how many barrels are in this

stack.

22. Three $20 bills, six $10 bills, and four $5 bills are

placed in a box, then two bills are drawn out and

placed in a savings account. What is the probability

the bills drawn are

a. ﬁrst $20, second $10

b. ﬁrst $10, second $20

c. both $5

d. ﬁrst $5, second not $20

e. ﬁrst $5, second not $10

f. ﬁrst not $20, second $20

⫹ 4y

⫺ 24y ⫹ 6x ⫹ 29 ⫽ 0

1⫺4, 021⫺6, 02

•

0.7x ⫹ 1.2y ⫺ 3.2z ⫽⫺32.5

1.5x ⫺ 2.7y ⫹ 0.8z ⫽⫺7.5

2.8x ⫹ 1.9y ⫺ 2.1z ⫽ 1.5

•

2a ⫹ 3b ⫺ 6c ⫽ 15

4a ⫺ 6b ⫹ 5c ⫽ 35

3a ⫹ 2b ⫺ 5c ⫽ 24

log13x ⫺ 22⫹ 1 ⫽ 4e

2x⫺1

⫽ 217

ln12x ⫺ 12⫽ 53 ⫽ log

11252

⫽ 3

⫺4

x ⫽ 10

h1x2⫽

⫺ 8

⫺ 1

1x2⫽ x

⫹ x

⫺ 4x ⫺ 4

23. The manager of Tom’s Tool and Equipment Rentals

knows that 4% of all tools rented are returned late.

Of the 12 tools rented in the last hour, what is the

probability that

a. exactly ten will be returned on time

b. at least eleven will be returned on time

c. at least ten will be returned on time

d. none of them will be returned on time

24. Use a proof by induction to verify

for all natural numbers n.

25. A park ranger tracks the number of campers at a

popular park from March to September

and collects the following data (month,

number of campers): (3, 56), (5, 126), and (9, 98).

Assuming the data is quadratic, draw a scatterplot

and construct a system of equations and solve

to obtain a parabolic equation model. (a) What month

had the maximum number of campers? (b) What was

this maximum number? (c) How many campers might

be expected in April? (d) Based on your model, what

month(s) is the park apparently closed to campers

(number of campers is zero or negative)?

Exercises 26 through 30 require the use of a graphing

calculator.

26. Solve the system. For this system x, y, and z are the

variables, with and e the well-known constants.

Round your answer to two decimal places.

27. Find the solution region for the system of linear

inequalities. Your answer should include a screen

shot or facsimile, and the location of any points of

intersection, rounded to four decimal places.

28. A triangle has vertices at (112.3, 98.5), (67.7, ),

and ( , 21.5). Use the determinant formula to

determine its area, rounded to the nearest tenth.

29. A recursive sequence is deﬁned by and

. Find S

, rounded to four

decimal places.

30. Solve the equation. Round your answer to two

decimal places.

log x ⫺ 2 ln x ⫹ 3 log

x ⫽ 6.

k⫹1

⫽ a

⫺ 0.4a

⫽ 0.3

⫺27␲

⫺39

112x ⫹ 39y 7 438

57x ⫺ 64y 6 101

x ⱖ 0

y ⱖ 0

•

␲x ⫹ 12

y ⫹ ez ⫽ 5

12x ⫹ ey ⫹ ␲z ⫽ 5

ex ⫹ ␲y ⫹ 12z ⫽ 5

␲

3 ⫻ 3

1m ⫽ 92

1m ⫽ 32

3 ⫹ 7 ⫹ 11 ⫹ 15 ⫹

⫹ 14n ⫺ 12⫽ n12n ⫹ 12

9–87 Cumulative Review Chapters R–9 847

College Algebra G&M—

cob19545_ch09_838-848.qxd 11/10/10 7:34 AM Page 847

This page intentionally left blank

College Algebra G&M—

A-1

The most fundamental requirement for learning algebra is mastering the words, symbols,

and numbers used to express mathematical ideas. “Words are the symbols of knowledge,

the keys to accurate learning” (Norman Lewis in Word Power Made Easy, Penguin Books).

A. Sets of Numbers, Graphing Real Numbers, and Set Notation

To effectively use mathematics as a problem-solving tool, we must ﬁrst be familiar

with the sets of numbers used to quantify (give a numeric value to) the things we

investigate. Only then can we make comparisons and develop equations that lead to

informed decisions.

Natural Numbers

The most basic numbers are those used to count physical objects: 1, 2, 3, 4, and so

on. These are called natural numbers and are represented by the capital letter ,

often written in the special font shown. We use set notation to list or describe a set of

numbers. Braces { } are used to group members or elements of the set, commas

separate each member, and three dots (called an ellipsis) are used to indicate a pattern

that continues indeﬁnitely. The notation is read, “ is the set of

numbers 1, 2, 3, 4, 5, and so on.” To show membership in a set, the symbol is used.

It is read “is an element of” or “belongs to.” The statements (6 is an element of

) and (0 is not an element of ) are true statements. A set having no elements

is called the empty or null set, and is designated by empty braces { } or the symbol

EXAMPLE 1

䊳

Writing Sets of Numbers Using Set Notation

List the set of natural numbers that are

a. greater than 100 b. negative

c. greater than or equal to 5 and less than 12

Solution

䊳

b. { }; all natural numbers are positive.

c. {5, 6, 7, 8, 9, 10, 11}

Now try Exercises 7 and 8

䊳

Whole Numbers

Combining zero with the natural numbers produces a new set called the whole

numbers We say that the natural numbers are a proper subset

of the whole numbers, denoted , since every natural number is also a whole

number. The symbol means “is a proper subset of.”(

 ( 

  50, 1, 2, 3, 4, p6.

5101, 102, 103, 104, p6

.

0 僆 

6 僆 

僆

  51, 2, 3, 4, 5, p6

LEARNING OBJECTIVES

In Section A.I you will review:

A. Sets of numbers,

graphing real numbers,

and set notation

B. Inequality symbols and

order relations

C. The absolute value of a

real number

D. The order of operations

The Language, Notation, and Numbers

of Mathematics

Appendix I

cob19545_app_001-013.qxd 12/21/10 8:09 PM Page 1