CIMA - C3 Fundamentals Of Business Mathematics

Подождите немного. Документ загружается.

220 8: Compounding ⏐ Part D Financial mathematics

Payments

NOW 1

(END)

Year 0 Year 1 Year 2 Year 3 Year 4

$400

× (1.10)

$400

× (1.10)

$400

× (1.10)

$400

× (1.10)

(Year 0)

The first year's investment will grow to $400 (1.10)

585.64

(Year 1)

The second year's investment will grow to $400 (1.10)

532.40

(Year 2)

The third year's investment will grow to $400 (1.10)

484.00

(Year 3)

The fourth year's investment will grow to $400 (1.10)

440.00

2,042.04

4.3 Geometric progressions

The example above was straightforward to calculate but if the time period was much longer, for example the

endowment element of a mortgage, it is easier to use the geometric progression formula to find the sum of the

terms

A geometric progression is a sequence of numbers in which there is a common or constant ratio between adjacent

terms.

An algebraic representation of a geometric progression is as follows.

A, AR, AR

, AR

,..., AR

n–1

where S is the terminal value

A is the first term

R is the common ratio

n is the number of terms

4.3.1 Geometric progression examples

Examples of geometric progressions are as follows.

(a) 2, 4, 8, 16, 32, where there is a common ratio of 2.

(b) 121, 110, 100, 90.91, 82.64, where (allowing for rounding differences in the fourth and fifth terms)

there is a common ratio of 1/1.1 = 0.9091.

Key term

T F

RWAR

Part D Financial mathematics ⏐ 8: Compounding 221

The sum of a geometric progression, S =

A(R 1)

−

Where S is the terminal value

A is the first term

R is the common ratio

n is the number of terms

This is the same as:

S = A [(1 + r

– 1)]

Where r is the interest rate

4.4 Terminal value calculations

The final value (or terminal value), S, of an investment to which equal annual amounts will be added is found

using the following formula.

S =

)1R(

)1R(A

−

(the formula for a geometric progression).

The solution to the example above can be written as (400 × 1.1) + (400 × 1.1

) + (400 × 1.1

) with

the values placed in reverse order for convenience. This is a geometric progression with A (the first term) = (400 ×

1.1), R = 1.1 and n = 4.

Using the geometric progression formula:

S =

A(R 1)

−

(400 ×1.1)×(1.1 - 1)

1.1-1

440 × 0.4641

0.1

= $2,042.04

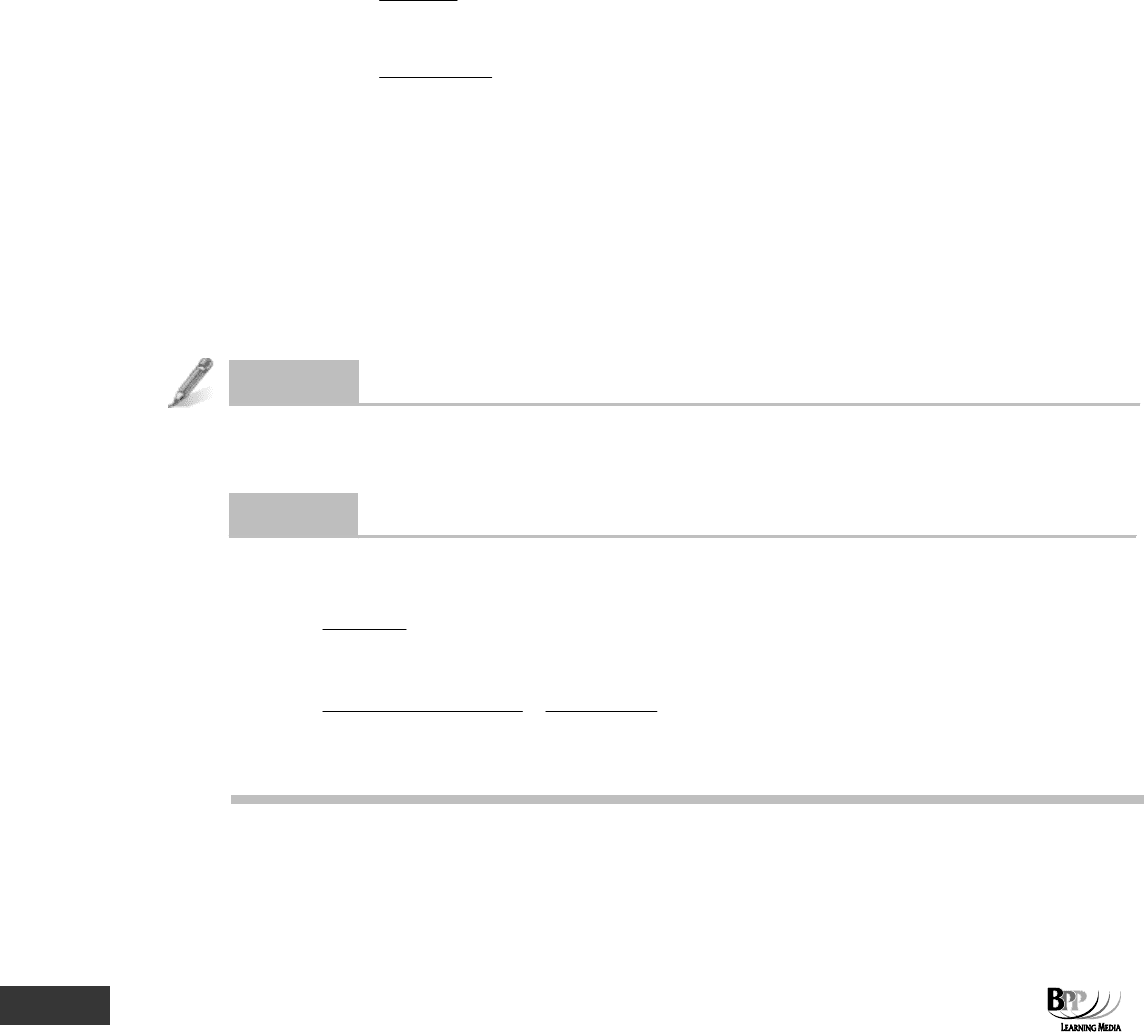
4.5 Example: Investments at the ends of years

(a) If, in the previous example, the investments had been made at the end of each of the first, second, third and

fourth years, so that the last $400 invested had no time to earn interest, we can show this situation on the

following time line.

Payments

NOW 1

(END)

Year 0 Year 1 Year 2 Year 3 Year 4

$400

× (1.10)

$400

× (1.10)

$400

× (1.10)

$400

(Year 0) No payment

Formula to

learn

T F

RWAR

222 8: Compounding ⏐ Part D Financial mathematics

(Year 1) The first year's investment will grow to $400 × (1.10)

(Year 2) The second year's investment will grow to $400

× (1.10)

(Year 3) The third year's investment will grow to $400

× (1.10)

(Year 4) The fourth year's investment remains at $400

The value of the fund at the end of the four years is as follows.

400 + (400

× 1.1) + (400 × 1.1

) + (400 × 1.1

)

This is a

geometric progression with

A = $400

R = 1.1

n = 4

If S =

1)A(R

−

S =

11.1

1)(1.1400

−

= $1,856.40

(b) If our investor made investments as in (a) above, but also put in a $2,500 lump sum one year from now, the

value of the fund after four years would be

$1,856.40 + $2,500 × 1.1

= $1,856.40 + $3,327.50 = $5,183.90

That is, we

can compound parts of investments separately, and add up the results.

Question

Geometric progression

A man invests $1,000 now, and a further $1,000 each year for five more years. How much would the total

investment be worth after six years, if interest is earned at the rate of 8% per annum?

Answe

This is a geometric progression with A (the first term) = $1,000 × 1.08, R = 1.08 and n = 6.

If S =

1)A(R

−

S =

108.1

)108.1()08.1000,1($

−

−××

1080 × 0.5869

0.08

= $7,923.15

Part D Financial mathematics ⏐ 8: Compounding 223

4.6 Sinking funds

A sinking fund is an investment into which equal annual instalments are paid in order to earn interest, so that by

the end of a given number of years, the investment is large enough to pay off a known commitment at that time.

Commitments include the replacement of an asset and the repayment of a mortgage.

With mortgages, the total of the constant annual payments (which are usually paid in equal monthly instalments)

plus the interest they earn over the term of the mortgage must be sufficient to pay off the initial loan plus accrued

interest. We shall be looking at mortgages later on in this chapter.

When replacing an asset at the end of its life, a company might decide to invest cash in a sinking fund during the

course of the life of the existing asset to ensure that the money is available to buy a replacement.

4.7 Example: Sinking funds

A company has just bought an asset with a life of four years. At the end of four years, a replacement asset will cost

$12,000, and the company has decided to provide for this future commitment by setting up a sinking fund into

which equal annual investments will be made, starting at year 1 (one year from now). The fund will earn interest at

12%.

Required

Calculate the annual investment.

Solution

Let us start by drawing a time line where $A = equal annual investments.

Payments

NOW 1

(END)

Year 0 Year 1 Year 2 Year 3 Year 4

× (1.12)

× 1.12

(Year 0) No payment

(Year 1) The first year's investment will grow to $A × (1.12)

(Year 2) The second year's investment will grow to $A × (1.12)

(Year 3) The third year's investment will grow to $A × (1.12)

(Year 4) The fourth year's investment will remain at $A.

The value of the fund at the end of four years is as follows.

A + A(1.12) + A(1.12

) + A(1.12

)

This is a geometric progression with

A = A

R = 1.12

n = 4

T F

RWAR

224 8: Compounding ⏐ Part D Financial mathematics

The value of the sinking fund at the end of year 4 is $12,000 (given in the question) therefore

$12,000 =

11.12

1)A(1.12

−

$12,000 = 4.779328A

∴ A =

4.779328

$12,000

= $2,510.81

Therefore, four investments, each of $2,510.81 should therefore be enough to allow the company to replace the

asset.

Question

Sinking funds

A farmer has just bought a combine harvester which has a life of ten years. At the end of ten years a replacement

combine harvester will cost $100,000 and the farmer would like to provide for this future commitment by setting

up a sinking fund into which equal annual investments will be made, starting

now. The fund will earn interest at

10% per annum.

Answe

The value of the fund at the end of ten years is a geometric progression with:

A = $A × 1.1

R = 1.1

n = 10

Therefore the value of the sinking fund at the end of ten years is $100,000.

∴ $100,000 =

11.1

1)(1.11.1A

−

−×

100,000 × 0.1 = A × 1.1 (1.1

–1)

A =

1)(1.11.1

0.1$100,000

−

61.75311670

$10,000

= $5,704.13

Part D Financial mathematics ⏐ 8: Compounding 225

5 Loans and mortgages

5.1 Loans

Most people will be familiar with the repayment of loans. The repayment of loans is best illustrated by means of an

example.

5.2 Example: Loans

Timothy Lakeside borrows $50,000 now at an interest rate of 8 percent per annum. The loan has to be repaid

through five equal instalments

after each of the next five years. What is the annual repayment?

Solution

Let us start by calculating the final value of the loan (at the end of year 5).

Using the formula S = X(1 + r)

Where X = $50,000

r = 8% = 0.08

n = 5

S = the sum invested after 5 years

∴ S = $50,000 (1 + 0.08)

= $73,466.40

The value of the initial loan after 5 years ($73,466.40) must equal the sum of the repayments.

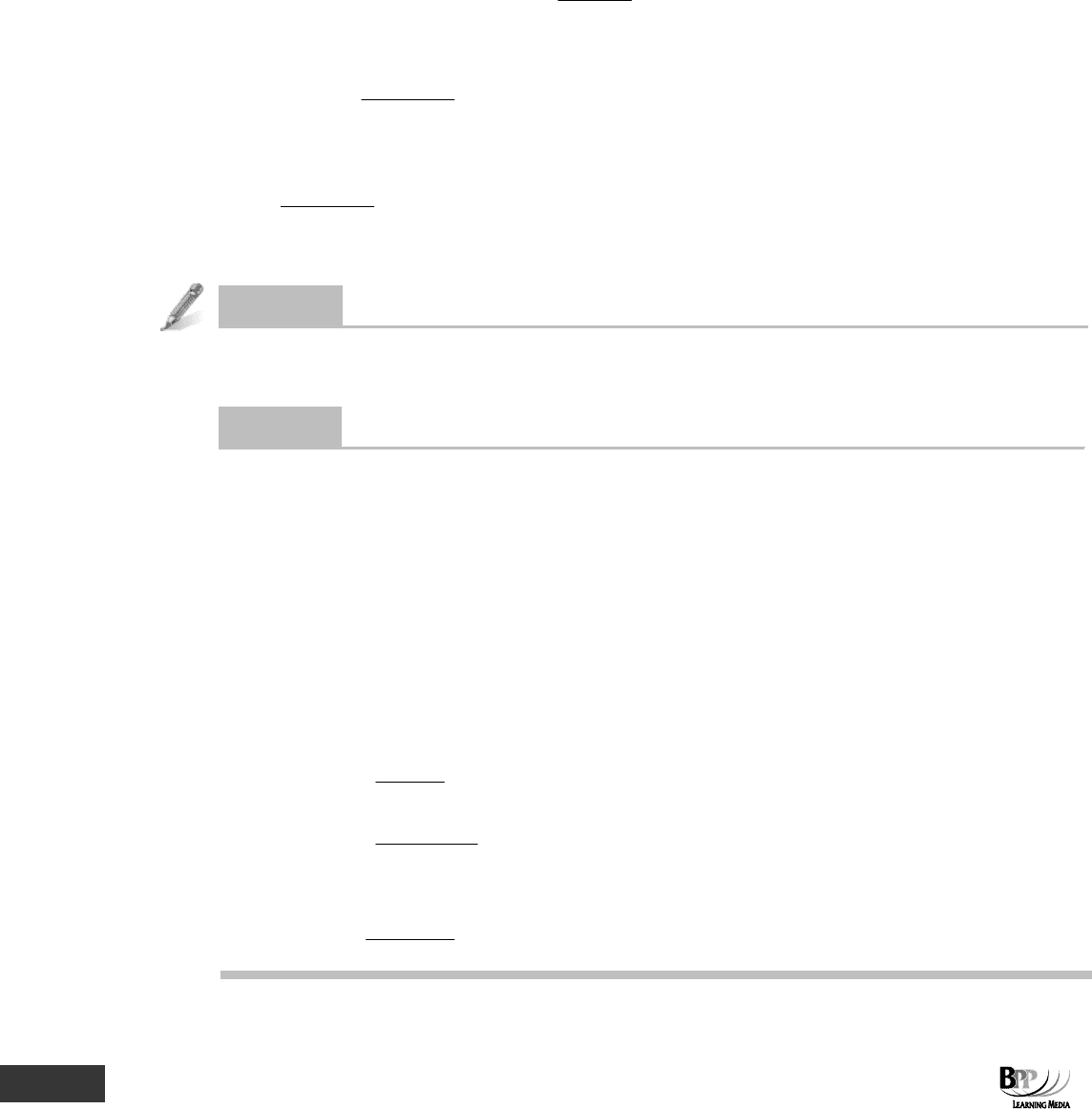
A time line will clarify when each of the repayments are made. Let $A = the annual repayments which start a year

from now, ie at year 1.

Repayments

NOW 1

(END)

Year 0 Year 1 Year 2 Year 3 Year 4 Year 5

× (1.08)

(Year 0) No payment

(Year 1) The first year's investment will grow to $A × (1.08)

(Year 2) The second year's investment will grow to $A × (1.08)

(Year 3) The third year's investment will grow to $A × (1.08)

(Year 4) The fourth year's investment will grow to $A × (1.08)

(Year 5) The fourth year's investment remains at $A.

226 8: Compounding ⏐ Part D Financial mathematics

The value of the repayments at the end of five years is as follows.

A + (A × 1.08) + (A × 1.08

) + (A × 1.08

)

This is a geometric progression with

A = A

R = 1.08

n = 5

The sum of this geometric progression, S =

)1A(R

−

= $73,466.40 since the sum of repayments must equal the

final value of the loan (ie $73,466.40).

S = $73,466.40 =

108.1

)108.1(A

−

$73,466.40 = A × 5.86660096

A =

86660096.5

40.466,73$

= $12,522.82

The annual repayments are therefore $12,522.82.

Question

Annual repayments

John Johnstone borrows $50,000 now at an interest rate of 7% per annum. The loan has to be repaid through ten

equal instalments after each of the next ten years. What is the annual repayment?

Answe

The final value of the loan (at the end of year 10) is

S = $50,000 (1+ 0.07)

= $98,357.57

The value of the initial loan after 10 years ($98,357.57) must equal the sum of the repayments.

The sum of the repayments is a geometric progression with

A = A

R = 1.07

n = 10

The sum of the repayments = $98,357.57

S = $98,357.57 =

(RA

1.07

A(1.07

= 13.816448A

∴ A =

13.816448

$98,357.57

= $7,118.88 per annum

Part D Financial mathematics ⏐ 8: Compounding 227

5.3 Sinking funds and loans compared

(a) Sinking funds. The sum of the regular savings, $A per period at r% over n periods must equal the

sinking fund required at the end of n periods.

(b)

Loan repayments. The sum of the regular repayments of $A per period at r% over n periods must

equal the final value of the loan at the end of n periods.

The final value of a loan can therefore be seen to be equivalent to a sinking fund.

5.4 Mortgages

As you are probably aware, when a mortgage is taken out on a property over a number of years, there are several

ways in which the loan can be repaid. One such way is the

repayment mortgage which has the following features.

• A certain amount, S, is borrowed to be paid back over n years

• Interest, at a rate r, is added to the loan retrospectively at the end of each year

• A constant amount A is paid back each year

Income tax relief affects repayments but, for simplicity, we will ignore it here.

5.5 Mortgage repayments

Consider the repayments on a mortgage as follows.

(a) At the end of one year A has been repaid.

(b) At the end of two years the initial repayment of A has earned interest and so has a value of A(1 + r)

and another A has been repaid. The value of the amount repaid is therefore A(1 + r) + A.

, the second repayment a

value of A (1 + r) and a third repayment of A will have been made. The value of the amount repaid is

therefore A(1 + r)

+ (1 + r) + A.

(d) At the end of n years the value of the repayments is therefore A (1 + r)

n–1

A(1 + r)

n–2

+ ... + A(1 + r)

+ A(1 + r) + A.

This is a

geometric progression with 'A' = A, 'R' = (1 + r) and 'n' = n and hence the sum of the repayments

]1r)1A[(

−+

)1R(A

−

5.6 Sum of repayments = final value of mortgage

During the time the repayments have been made, the initial loan has accrued interest.

The repayments must, at the end of n years, repay the initial loan plus the accrued interest.

Therefore the

sum of the repayments must equal the final value of the mortgage.

Sum of repayments = final value of mortgage

)1R(A

−

= SR

∴ A =

1)(R

1)(RSR

−

−×

228 8: Compounding ⏐ Part D Financial mathematics

5.7 Example: Mortgages

(a) Sam has taken out a $30,000 mortgage over 25 years. Interest is to be charged at 12%. Calculate the

monthly repayment.

(b) After nine years, the interest rate changes to 10%. What is the new monthly repayment?

Solution

(a) Final value of mortgage = $30,000 × (1.12)

= $510,002

Sum of repayments, S

Where A = annual repayment

R = 1.12

n = 25

∴ S =

11.12

1)A(1.12

−

= 133.334A

Sum of repayments = final value of mortgage

133.334A = $510,002

A =

334.133

002,510$

A = $3,825

If annual repayment = $3,825

Monthly repayment =

825,3$

= $318.75

(b) After 9 years, the

value of the loan = $30,000 × (1.12)

= $83,192

After 9 years, the

sum of the repayments =

)1R(A

−

Where A = $3,825

R = 1.12

n = 9

∴ Sum of repayments =

112.1

)112.1(825,3$

−

= $56,517

Value of loan at year 9

83,192

Sum of repayments at year 9

56,517

Loan outstanding at year 9

26,675

Part D Financial mathematics ⏐ 8: Compounding 229

A new interest rate of 10% is to be charged on the outstanding loan of $26,675 for 16 years

(25 – 9).

Final value of loan = $26,675 × (1.1)

= $122,571

Sum of repayments =

)1R(A

−

Where R = 1.1

n = 16

A = annual repayment

∴ Sum of repayments =

11.1

1)A(1.1

−

= 35.94973A

Final value of loan = sum of repayments

$122,571 = 35.94973A

∴ A =

94973.35

571,122$

A = $3,410

∴ monthly repayment =

410,3$

= $284.17

The final value of a loan/mortgage can be likened to a sinking fund also, since the final value must equate to the

sum of the periodic repayments (compare this with a sinking fund where the sum of the regular savings must

equal the fund required at some point in the future).

Question

Monthly repayment

Nicky Eastlacker has taken out a $200,000 mortgage over 25 years. Interest is to be charged at 9%. Calculate the

monthly repayment.

Answe

Final value of mortgage = $200,000 × (1.09)

= $1,724,616

Sum of repayments, S =

(RA

Where A = Annual repayment

R = 1.09

n = 25

Important!