Celletti A. Stability and Chaos in Celestial Mechanics

Подождите немного. Документ загружается.

4 1 Order and chaos

1.2 Linear stability

Let us consider the dynamical system described by the equation (1.1) with  degrees

of freedom and let J be the Jacobian matrix deﬁned as

J = J(x

) ≡ Df(x)=

⎛

⎜

⎝

∂f

∂x

...

∂f

∂x



∂f



∂x

...

∂f



∂x



⎞

⎟

⎠

In the proximity of an equilibrium position x

= x

we refer to

˙y

= J(x

)y ,y∈ R



, (1.6)

as the linearized system. Let 

be the number of eigenvalues of J(x

) with zero

real part, 

be the number of eigenvalues with positive real part and 

those with

negative real part.

Deﬁnition. The equilibrium position x

= x

is called hyperbolic if 

=0;itis

called an attractor if 

= ,arepeller if 

=  and a saddle if 

and 

are both

strictly positive.

Near a hyperbolic equilibrium the dynamics of (1.1) is equivalent to that of the

linearized system (1.6) as stated by the following result [84,93]:

Hartman–Grobman theorem. Consider the dynamical system described by

(1.1) and assume that it admits a hyperbolic equilibrium position x

. In a suitable

neighborhood of x

the system (1.1) is topologically equivalent

to the linearized

system (1.6).

Let us now discuss the stability of an equilibrium position; we ﬁrst introduce the

following deﬁnition of stability according to Lyapunov for a generic solution of the

system (1.1). To ﬁx the notations, let ·denote the distance function, e.g. the

Euclidean norm in R



Deﬁnition. The solution x

∗

= x

∗

(t) is said to be stable according to Lyapunov, if

for any ε>0 there exists δ = δ(ε) such that if x

) − x

∗

) <δat the initial

time t = t

,thenx(t) − x

∗

(t) <εfor any t ≥ t

The solution is said to be asymptotically stable if, for x

) −x

∗

) <δ, one has

lim

t→∞

x(t) − x

∗

(t) =0.

When the solution coincides with an equilibrium point, its linear stability can be

inferred from the analysis of the eigenvalues of the Jacobian matrix; we denote by

(λ

,...,λ



) the roots of the characteristic equation det(J − λI



)=0(I



is the

 ×  identity matrix) and we denote by v

∈ R



, j =1,...,, the eigenvector

associated to λ

. Then, the solution of the linearized system can be written in

the form y

(t)=





j=1

for some real or complex coeﬃcients α

which are

determined by the initial conditions.

Assuming for simplicity that  = 2, the equilibrium is characterized as follows,

according to the nature of the eigenvalues λ

, λ

i.e., there exists a homeomorphism which conjugates (1.1) and (1.6).

1.2 Linear stability 5

– if λ

, λ

are real negative numbers, then the equilibrium is a stable node;

– if λ

, λ

are real positive numbers, then the equilibrium is an unstable node;

– if λ

, λ

are real numbers with opposite signs, then the equilibrium is a saddle;

– if λ

, λ

are complex numbers with negative real part, then the equilibrium is

a stable focus;

– if λ

, λ

are complex numbers with positive real part, then the equilibrium is

an unstable focus;

– if λ

, λ

are purely imaginary and non–zero, then the equilibrium is a center.

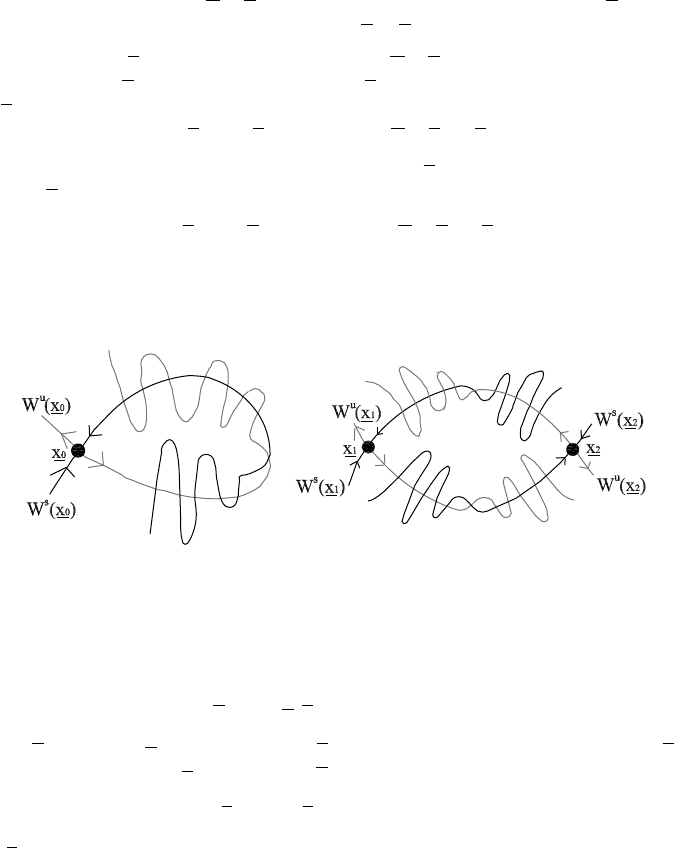
We next introduce the deﬁnitions of stable and unstable manifolds as well as those of

homoclinic and heteroclinic intersections (see, e.g., [80] for applications to Celestial

Mechanics). We denote by Φ

(t;˜x)theﬂow at time t with initial condition ˜x, namely

the solution at time t of (1.1) starting from x

=˜x.

Deﬁnition. Let x

be a saddle point and let Φ(t; x) be the ﬂow at time t with

initial condition x

.Thestable manifold W

) is the set of points which end–up

at x

, i.e.

) ≡{x ∈ R



: lim

t→∞

Φ(t; x)=x

} .

Analogously, we deﬁne the unstable manifold W

) as the set of points which

tend to x

for negative times, i.e.

) ≡{x ∈ R



: lim

t→−∞

Φ(t; x)=x

} .

The intersection between W

and W

is called a homoclinic point; if W

and W

belong to diﬀerent equilibrium positions, then the intersection is called a hetero-

clinic point (see Figure 1.2).

Fig. 1.2. Left: Stable and unstable manifolds of a homoclinic point. Right: An example

of heteroclinic intersections.

Let us brieﬂy transpose the main deﬁnitions and results to discrete systems.

Consider the –dimensional mapping

n+1

= f(x

) ,n∈ N , (1.7)

where x

∈ R



and f =(f

,...,f



); let x

be a ﬁxed point and denote by J ≡ J(x

)

the Jacobian matrix of f

computed at x

. The linearized system is written as

n+1

= Jy

,n∈ N ,

for y

∈ R



6 1 Order and chaos

Deﬁnition. Aﬁxedpointx

of (1.7) is called hyperbolic, if the eigenvalues of

J = J(x

) are real and one of them has magnitude greater than one. A ﬁxed point

is called elliptic, if the eigenvalues are complex with absolute value equal to one.

Aﬁxedpointx

is an attractor if the eigenvalues have magnitude less than unity

and it is a repellor if the magnitude is greater than one.

If f

in (1.7) is deﬁned on a space X with (X, d) being a metric space with

metric d : X ×X → R, then the deﬁnition of stability according to Lyapunov of a

ﬁxed point x

transposes as follows.

Deﬁnition. For any ε>0, there exists δ = δ(ε) such that for any x

∈ X with

d(x

,x) <δ,thend(f

),f

(x)) <εfor any n ∈ N. The ﬁxed point is asymp-

totically stable if lim

n→∞

d(f

),f

(x)) = 0 whenever d(x

,x) <δ.

Concerning the linear stability we proceed as follows. The general solution is a linear

combination of the form x





j=1

for suitable coeﬃcients α

, while v

denote the eigenvectors. If λ

, j =1,...,, are the eigenvalues associated to the

Jacobian J, then the ﬁxed point is linearly stable if |λ

|≤1 for all j =1,..., and

it is linearly unstable if for some j it is |λ

| > 1.

1.3 Conservative and dissipative systems

Let us consider a continuous system described by the equations (1.1); qualitatively

we can say that the system is conservative, if the volume of the phase space is

preserved. The system is said to be dissipative if the volume contracts or expands

along the ﬂow.

When the ﬂow associated to the evolution of the system is interpreted as a trans-

formation from x

to y representing the solutions at times t

and t

,say

≡ x(t

) → y ≡ x(t

) , (1.8)

then the volume of the phase space, denoted by V , changes according to the formula



dy =



|det(J)| dx , (1.9)

where J is the Jacobian of the transformation (1.8) and det(J) is its determinant.

Writing (1.8) as y

= h(x) for a suitable vector function h, one obtains that J coin-

cides with the Jacobian of h

,sayJ = Dh(x). The dynamical system is conservative

if |det(J)| = 1, while it is contractive if |det(J)| < 1 and expansive whenever

|det(J)| > 1.

With reference to equation (1.1), let F

(x) ≡ Φ(t; x) be the ﬂow at time t with

initial datum x

;since{F

(x)}

t∈R

is a solution of (1.1), by deﬁnition one has

(x)=f(F

(x)) ;

1.4 The attractors and basins of attraction 7

diﬀerentiating with respect to x one obtains the variational equations

(x)=Df(F

(x)) · DF

(x) . (1.10)

Let J

≡ DF

(x)andA(t) ≡ Df(F

(x)); then,

= A(t) J

. According to Liou-

ville’s formula [93], denoting by Tr(A) the trace of the matrix A, one has

(det(J

)) = Tr(A(t)) · det(J

) , where det J

=1. (1.11)

From (1.11) one ﬁnds det(J

)=e

Tr(A(s)) ds

and the system is said to be dissi-

pative and contractive if the phase space volume decreases with time, namely if

Tr(A(t)) < 0 for any t>0. If we set y

= F

(x) in (1.9), we obtain J

= J,sothat

det(J

)=det(J). In particular, the system is conservative if det(J

)=1.

An example of a dissipative system is provided by the damped pendulum described

by the equation

¨x + c ˙x +sinx = b cos t,

where c is a positive real constant and b is a real constant. Let us write such

equation as the following system of three ﬁrst–order diﬀerential equations:

˙x = y

˙y = −cy − sin x + b cos t

t =1.

One readily obtains that

A(t)=

⎛

⎝

010

−cos x(t) −c −b sin t

000

⎞

⎠

with Tr(A(t)) = −c<0; the contraction rate is therefore represented by the

quantity

det(J

)=e

Tr(A(s))ds

= e

−ct

1.4 The attractors and basins of attraction

In the framework of dynamical systems theory a relevant concept is provided by

that of the attractor; qualitatively it is deﬁned as the set toward which the dynam-

ical system evolves. A more precise deﬁnition is given as follows.

Deﬁnition. For a continuous ﬂow Φ

: R×R



→ R



associated to (1.1), the ω–limit

set corresponding to the initial condition x

is deﬁned as the set

ω(x

)={x ∈ R



: there exists a sequence {t

}→∞such that

; x

) → x as n →∞}.

8 1 Order and chaos

For a mapping of the form (1.4), let {x,f(x),f

(x),...} be the orbit associated

to the initial position x

according to the dynamics generated by f .Theω–limit

set corresponding to the initial condition x

is given by the accumulation points

)}, namely

ω(x

)={x ∈ R



: there exists a sequence {n

}∈Z

(strictly increasing) such that f

) → x as k →∞}.

An attractor is an ω–limit set which has the property to attract a set with non–zero

measure of initial values [146]. We can now give the following qualitative deﬁnition

of basin of attraction.

Deﬁnition. Consider a continuous or discrete system which admits an attractor;

the corresponding basin of attraction is composed by the set of initial conditions

which tend to the attractor as time goes on (for continuous systems) or as the

number of iterations increases (for discrete systems).

In other words, the basin of attraction is the closure of the set of initial points

whose trajectory approaches the attractor asymptotically. An attractor is said to

be chaotic, when it shows sensitivity to the choice of the initial conditions. More

precisely, let us suppose that we observe two trajectories, initially very close to

each other. Following their evolution with time, we speak of extreme sensitivity to

the initial conditions, if the distance between the two trajectories increases expo-

nentially with time. In such a case it is impossible to make a long–term prediction,

since small uncertainties in the initial conditions are greatly ampliﬁed in a relatively

short time.

There exist diﬀerent techniques for computing the basins of attraction. The most

intuitive is to select a sample of random initial conditions and to let them evolve

until they reach the attractor. It may happen that such a procedure requires a very

long computational time; henceforth, alternative techniques have been developed

in order to compute the basins of attraction using faster algorithms. To provide an

example, we mention a simple method, which is based on the following recipe [81].

Select a pair of points, say (P

), where P

is captured by the attractor, while

is observed to pass it. Having ﬁxed a precision δ,letP

and P

evolve, checking

that their distance remains smaller than δ. Denote by P

(j)

, P

(j)

the iterated points

at step j; if their distance becomes greater than δ, select a new position coinciding

with the point on the middle between P

(j)

and P

(j)

,andletitreplaceP

(j)

or P

(j)

according to whether it evolves, or not, to the attractor. This procedure leads to

the delimiting of the boundary of the basin of attraction within the precision δ.

We remark that basin boundaries can be subject to bifurcations, called meta-

morphoses, as a system parameter passes through a critical value; in some cases,

such a transition can also take place between a smooth curve and a fractal bound-

ary [146].

1.5 The logistic map 9

1.5 The logistic map

The logistic map is described by the non–linear, one–dimensional equation

j+1

= rx

(1 − x

)forx

∈ [0, 1] , (1.12)

where r is a real positive parameter. This equation is associated to the logistic

continuous equation originally introduced by P.–F. Verhulst [170] as a model of

population growth:

= rx(1 − x) .

In the mapping (1.12), if x

is the initial population, then x

represents the pop-

ulation at the year j; the parameter r denotes a combined rate for starvation and

reproduction. The logistic map was widely investigated in [133]; despite its relative

simplicity, it provides a huge variety of complex behaviors. In particular, two ef-

fects will be determined which correspond to starvation and reproduction, with a

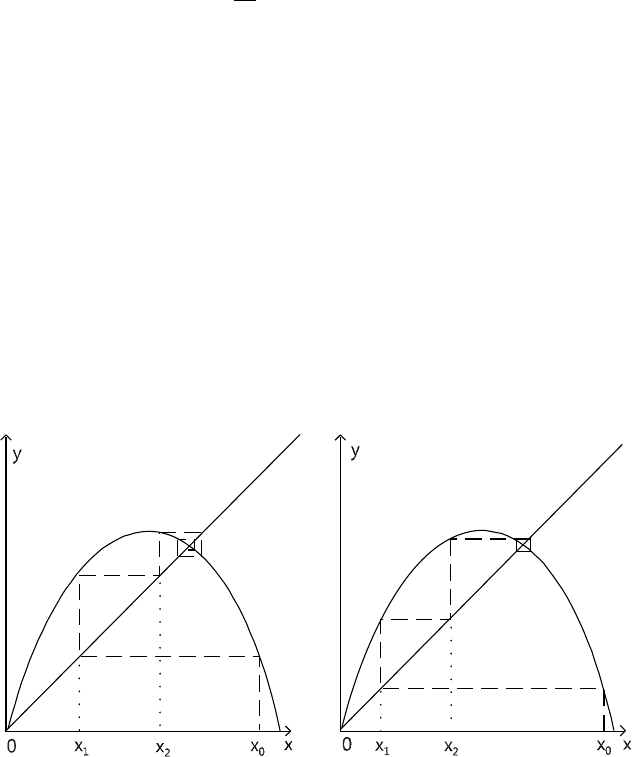
growth rate respectively decreasing and increasing. In order to provide a graphical

visualization of the iterations of the logistic map, one can proceed as described in

Figure 1.3: ﬁrst, one draws the parabolic curve y = rx(1 − x) on the diagram x

versus y as well as the diagonal line x = y. From a given abscissa x

one computes

the point on the parabola, proceed to meet horizontally the diagonal line, com-

pute the point with the same abscissa on the parabola and continue iterating this

procedure. The behavior of the population strongly depends on the value of the

parameter r and on the initial condition x

; for example, in Figure 1.3(a) the dy-

namics is attracted toward a ﬁxed point, while in Figure 1.3(b) the ﬁnal trajectory

is a periodic orbit of period 2.

(a) (b)

Fig. 1.3. Graphical iteration of the logistic map (1.12). (a)Astableﬁxedpoint;(b)a

periodic orbit of period 2.

10 1 Order and chaos

We start by analyzing the logistic map for the speciﬁc value r = 4, namely

j+1

=4x

(1 − x

)withx

∈ [0, 1] .

For x

∈ [0, 1] deﬁne ξ

∈ [0, 1] through the change of variables

=sin



πξ



;

one obtains the equality sin

(

πξ

n+1

)=sin

(πξ

) which implies that ξ

n+1

= ±2ξ

k for k ≥ 0 integer. Since ξ

∈ [0, 1], there is a univocal choice for k which leads to

the deﬁnition of the following map:

n+1

=2ξ

for 0 ≤ ξ

n+1

=2− 2ξ

for

≤ ξ

≤ 1 . (1.13)

The mapping (1.13) is known as the tent map which can be written also as

n+1

=1− 2|ξ

−

|. Direct iterations of the mapping show that any initial con-

dition is subject to a stretching and folding process, thus providing an exponential

divergence of nearby orbits with the folding keeping the trajectory bounded. Due

to the extreme sensitivity to the choice of the initial conditions, the tent mapping

is shown to be chaotic.

Let us proceed with the analysis of the logistic map within the interval

0 <r<4. The equilibrium points are the solutions of the equation rx

(1−x

)=x

which provides the two points x

(0)

= 0 and x

(1)

r−1

. Denoting by f(x) ≡

rx(1 −x), since f

(0) = r one obtains that the origin is stable for 0 <r<1andit

is unstable when 1 <r<4. The point x

(1)

does not exist for 0 <r<1 (recall that

x belongs to the interval [0, 1]); due to the fact that f

(1)

)=2−r one ﬁnds that

(1)

is stable for |2 − r| < 1, namely 1 <r<3, and it is unstable for 3 <r<4.

Within the interval 3 <r<4 the map shows a sequence of bifurcations with the

appearance of cycles of higher length. Two–cycles (namely oscillations between two

values) appear as far as r>3. In fact, the condition for two–cycles is

n+2

= rx

n+1

(1 − x

n+1

)=r[rx

(1 − x

)][1 − rx

(1 − x

)] = x

which is equivalent to

−r



− 2x

1+r

1 − r



=0.

Writing this equation as

−r



−

r − 1



−

r +1



=0,

we recognize that the quadratic equation admits real solutions provided r ≥ 3, from

which two–cycles appear as ﬁxed points of the square of the mapping. This behavior

1.5 The logistic map 11

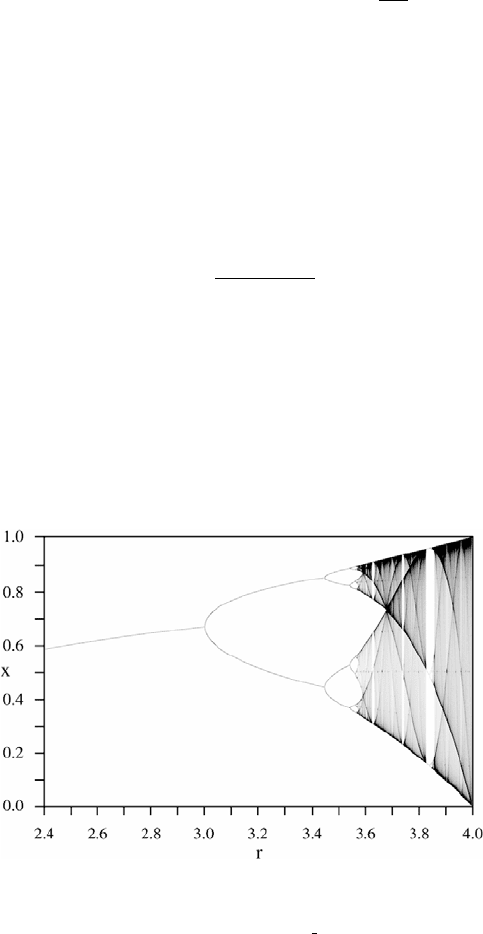
is described by the bifurcation diagram of Figure 1.4, which provides the change of

structure of the orbit, through a period–doubling bifurcation, as the parameter r

is varied.

The qualitative description of the results is the following (compare also with

Figure 1.4):

– if 0 <r<1 the origin is stable;

– if 1 <r<3 the dynamics admits the stable solution

r−1

;

– for 3 ≤ r<3.45 the dynamics may show an oscillation between 2 values;

– for 3.45 ≤ r<3.54 the dynamics may show an oscillation between 4 values;

– for 3.54 ≤ r<3.57 the dynamics may exhibit an oscillation between 8, 16,

32. . . values, showing a so–called period–doubling cascade;

– for 3.57 ≤ r<4 there is the onset of chaos with high sensitivity to changes in

the initial conditions; nevertheless for isolated values of the parameter, there

might be islands of stability.

If {r

} denotes the sequence of parameters at which successive period doublings

take place, then it can be shown that the sequence formed by the quantities

≡

j+1

− r

j+2

− r

j+1

admits the limit

lim

j→∞

=4.669201 ...

ThelastnumberiscalledtheFeigenbaum constant [65]; it provides the rate of

appearance of successive bifurcations as a universal behavior, since it is observed not

only for the logistic map, but also for a class of suitable dissipative one–dimensional

mappings undergoing a period–doubling cascade.

Fig. 1.4. Bifurcation diagram of the logistic map providing the period doubling of the

limit cycles as the parameter r is varied

(after http://en.wikipedia.org/wiki/File:LogisticMap

BifurcationDiagram.png).

12 1 Order and chaos

1.6 The standard map

One of the most popular mappings in the theory of Dynamical Systems is the so–

called standard map, which was introduced by B.V. Chirikov in [47]. It is deﬁned

by the equations



= y + εf(x)



= x + y



, (1.14)

where y ∈ R, x ∈ T ≡ R/(2πZ), ε is a positive real parameter, called the perturbing

parameter, and f = f(x) is an analytic, periodic function. Notice that the mapping

can also be written using the following notation:

j+1

= y

+ εf(x

)

j+1

= x

+ y

j+1

for j ≥ 0 . (1.15)

The classical standard map is obtained setting f(x)=sinx:



= y + ε sin x



= x + y



We list below some properties of the standard map, referring to its formulation

(1.15).

(i) The mapping (1.15) is conservative, since the determinant of the corresponding

Jacobian is equal to one; in fact, setting f

) ≡

∂f(x

)

∂x

, the determinant of

the Jacobian (1.15) is equal to

det



1 εf

)

11+εf

)



=1. (1.16)

(ii) For ε = 0 the mapping (1.15) reduces to

j+1

= y

j+1

= x

+ y

j+1

for j ≥ 0 . (1.17)

Therefore y

is constantly equal to the initial value y

, while we can write

= x

+ jy

for any j ≥ 0.

(iii) The ﬁxed points of the standard map are obtained by solving the equations

j+1

= y

j+1

= x

;

from the ﬁrst equation one has f(x

) = 0, while the second equation provides

j+1

=0=y

. For the classical standard map the ﬁxed points are given by

the pairs (y

)=(0, 0) and (y

)=(0,π). The linear stability of such

points is investigated by computing the ﬁrst variation, which can be written



δy

j+1

δx

j+1



= M



δy

δx



1.6 The standard map 13

where M denotes the matrix appearing in (1.16) computed at the ﬁxed point.

The corresponding eigenvalues are determined by solving the characteristic

equation

− (2 ± ε)λ +1=0,

where the positive sign holds for the ﬁxed point (0, 0), while the negative sign

must be taken for (0,π). Since one eigenvalue associated to (0, 0) is greater

than one, the ﬁxed point is unstable. For ε<4 the eigenvalues associated to

the point (0,π) are complex conjugate with real part less than one; therefore

the position (0,π)isstable.

We now describe the behavior of the mapping as ε varies. We have seen (item

(ii)above)thatforε = 0 the coordinate y

is held ﬁxed, while x

j+1

= x

+ y

.In

particular, if the initial value of the y

variable is equal to a rational multiple of

2π, then the trajectory {(x

)}, k ∈ Z

, is a periodic orbit. For example, let us

suppose that we start with the initial datum (y

)=(

π, x

); then, the successive

iterations of the x coordinate are given by the following sequence: x

π, x

π,

+2π.Sincex

varies on the torus, after 3 iterations one gets back to the initial

point; in this case one speaks of a periodic orbit of period 3. In general, if y

=2π

with p, q positive integers (q = 0), one obtains a periodic orbit of period q.Itis

readily seen that the quantity p measures how many times the interval [0, 2π)is

run before coming back to the starting position.

The situation drastically changes when an irrational initial condition y

is taken

in place of a rational initial point. For ε =0they–value remains constant, while

one can show that when the number of iterations of the mapping is increased the

x–variable densely ﬁlls the line y = y

(Figure 1.5(a)). Such straight lines are quasi–

periodic invariant curves, since on these curves a quasi–periodic motion takes place

such that the dynamics comes indeﬁnitely close to the initial conditions at regular

intervals of time, though never exactly retracing itself (as is the case for the periodic

orbits).

In order to distinguish between periodic orbits and quasi–periodic motions, one

can introduce the rotation number which is deﬁned as the quantity (independent

of the initial condition)

ω ≡ lim

j→∞

− x

In the unperturbed case it is ω = y

,sinceforε = 0 equations (1.15) reduce to

= y

= x

+ jy

According to the value of the rotation number we distinguish between periodic and

quasi–periodic motions. Indeed, if ω =2π

with p, q integers (q = 0), then y

= y

and x

= x

+2πp = x

(modulus 2π)andthemotionisperiodic.If

2π

is irrational,

the dynamics associated to (1.15) with ε = 0 is quasi–periodic.

In conclusion, for ε = 0 the system reduces to (1.17) and it is said to be inte-

grable, since the dynamics can be exactly solved: all motions are recognized as being

periodic or quasi–periodic. A non–integrable system occurs when it is not possible