Blanchet L., Spallicci A., Whiting B. (Eds.) Mass and Motion in General Relativity
Подождите немного. Документ загружается.


The State of Current Self-Force Research 401
where
G
˛
WD g
ı
h
2
.
˛/ı
˛;ı
i
(4)
F
˛ˇ
WD 2g
ı
.˛
ˇ/ı
: (5)
Here, D
2
is the scalar field wave operator, that is,
D
2
WD g
ı
@
@
ı
g
ı
ı
@
: (6)
The source is given by
T
˛ˇ
D
Z
1
1
1
p
g
ı
4
h
x
x
p
./
i
u
˛
u
ˇ
d; (7)
and includes only delta functions but not their derivatives. This approach is now
being undertaken [36].
3 Frequency-Domain Calculations of the Self-Force
The most popular approach for calculating the self-force in the FD is to use
mode-sum regularization or the Detweiler–Whiting decomposition. Specifically,
one calculates directly the colatitude ` modes of the divergent, bare self-force, and
then subtracts the divergent piece. If this is done carefully enough, one obtains the
unique finite, physical self-force.
The simplest case is that of scalar field self-force sourced by a Schwarzschild
circular orbit. Indeed, this case was the first to be studied [12, 23]. In principle,
one may find numerically the large ` behavior of the individual field modes, and
perform mode-sum regularization using the numerically obtained values. While this
approach is not impossible, one can obtain better and more accurate results using
the analytically obtained regularization parameters. The latter were calculated for
generic Kerr geodesics, so that they are readily available [8]. A similar approach
was successfully applied also for the gravitational self-force (radial Schwarzschild
trajectories) [7].
3.1 Mode-Sum Regularization
In its simplest form, the mode-sum approach requires finding the contributions
to the self-force mode by mode. The contribution to the physical self-force from
the tail part of the Green’s function can be decomposed into stationary Teukolsky
modes, and then summed over the frequencies ! and the azimuthal numbers m.

402 L.M. Burko
The self-force equals then the limit " ! 0
of the sum over all ` modes, of the
difference between the force sourced by the entire world line (the bare force
bare
f
`
)
and the force sourced by the half-infinite world line to the future of ",wherethe
particle has proper time D 0,and" is an event along the past (<0) world line.
Next, we seek a regularization function h
`
which is independent of ", such that the
series
X
`
bare
f
`
h
`
(8)
converges. Once such a regularization function is found, the regularized self-force
is then given by
reg
f
D
X
`
bare
f
`
h
`
D
; (9)
where D
is a finite valued function of the metric of the space-time of the back-
ground, and of the orbital parameters. It has been shown that the regularization
function h
`
has the general form
h
`
D
` C
1
2
A
C B
C
` C
1
2
1
C
: (10)
The functions A
;B
;C
,andD
are the regularization parameters, and they are
functions of the background metric and of the orbital parameters. For generic Kerr
geodesics, it was shown that C
D 0 (which guarantees that the self-force has
no logarithmic divergences), and that D
D 0. Note, that unless it is known that
D
D 0 (or any other function for that matter), one may not calculate the self-
force from just the numerically found asymptotic behavior of the ` modes of the
bare force. It is therefore fortuitous that D
D 0 for all Kerr geodesics, which may
enable practical numerical evaluation of the regularization parameters. However,
such calculations are slow, and require many ` modes, which is computationally
expensive.
A much better way to proceed is to evaluate the regularization parameters A
;B
analytically, which can be done because they depend only on the local behavior of
the Green’s function. One may even further speed up the convergence of the sum
over all ` modes by including the contributions of terms that are higher orders in
1=.` C 1=2/. The first use in this technique was made in [12], and it was further
developed in [23].
3.2 The Detweiler–Whiting Regular Part of the Self-Force
In [23], the approach to mode-sum regularization was different: The retarded scalar
field
ret
was divided to a singular part
S
and a regular part
R
, such that
ret
D
S
C
R
: (11)
The State of Current Self-Force Research 403
Since both the retarded and the singular fields are solutions to the inhomogeneous
wave equation that is sourced by the point particle, it immediately follows that
the regular field is a solution of the homogeneous wave equation. It was shown
by Detweiler and Whiting [24], that the correct physical self-force is found when
the regular piece
R
is used instead of the tail part as in the mode-sum approach
discussed above. The Detweiler–Whiting regular piece of the field, that satisfies
the homogeneous wave equation, provides us with an alternative, and for many
purposes clearer, conceptual viewpoint of the origin of the self-force. The latter
originates from the free waves that the particle emitted at retarded times. The parti-
cle moves on a geodesic orbit in a perturbed space-time that is regular everywhere in
a neighborhood of the world line. This viewpoint complements the older self-force
viewpoint, that maintains that the particle moves along an accelerated world line in
the background space-time. Either viewpoint has advantages, and we contend that
using them complementarily may add insight to practical applications.
The gravitational self-force for circular Schwarzschild orbits was calculated by
Detweiler in [22]. The calculation was done in the FD and in the Regge–Wheeler
gauge, and was done from the viewpoint of geodesic motion in a (smooth) perturbed
spacetime. That is, the particle is following geodesic motion not in Schwarzschild,
but rather in Schwarzschild endowed with a linear perturbation field that is sourced
by the particle itself at retarded times. Detweiler also considered observables, that
is, gauge independent quantities, that are the only meaningful quantities to seek in
the gravitational waveforms. Specifically, Detweiler found in [22] the effects on the
orbital frequency and on the rate of passage of proper time along the world line.
Detweiler was also able to show agreement of his results with the post-Newtonian
results in the weak-field limit. Very importantly, Detweiler’s results were also shown
to be in agreement, to within numerical accuracy, with the TD calculations done
from the complementary viewpoint, that is, that of accelerated motion on a fixed
background, that we discuss below in the following section [45].
4 Time-Domain Calculations of the Self-Force
TD calculations have a number of advantages over FD calculations. First, one is
freed from the dependence of the computation time on the orbital parameters. Sec-
ond, it is quite straightforward to specify any world line, and the computation would
continue much in the same way. Last, back reaction of the self-force on the world
line appears to be done in a more natural way in the TD.
4.1 1C1D Numerical Simulations
In the case of the Teukolsky equation progress on the TD front was lagging behind
the FD approach, as discussed above. For self-force calculations, however, the first
TD computation of the self-force appeared shortly after the first FD calculation,
404 L.M. Burko
albeit for the very simplified problem of scalar field self-force, and radial free fall
in Schwarzschild [4]. In [4], the summation over all ` modes of the self-force was
expedited by approximating the remainder of the partial sum over ` modes with the
use of the trigamma function.
The TD calculation of scalar field self-force in the case of radial fall in
Schwarzschild was then followed in [7] for its gravitational self-force counter-
part. The most significant added difficulty in the gravitational self-force case is
the gauge problem. Specifically, the mode-sum regularization approach is based
on the regularization parameters being calculated in the Lorenz gauge. However,
the full, or bare force is most easily calculated in a gauge that simplifies the field
equations, namely the Regge–Wheeler gauge for the Schwarzschild space-time.
To do the subtraction meaningfully, one needs to have both pieces of the self-force
be given in the same gauge. In [7] this problem was fortuitously avoided, because
of an accidental coincidence of the results for the regularization parameters in the
two gauges for the particular world line of interest. This fortunate coincidence
occurs because for radial Schwarzschild geodesics the transformation between the
Lorenz gauge and the Regge–Wheeler gauge is regular. For more general orbits this
transformation is singular. Other advantages to working in the Lorenz gauge are
that it makes hyperbolicity manifest, and that the local singularity of the particle’s
field is isotropic and isolated.
Circular Schwarzschild orbits were first considered for the gravitational self-
force in [10]. The entire calculation was done in [10] in the Lorenz gauge in the
TD. The calculation in [10] was done in 1C1D, which simplified the calculations
considerably, and facilitated the successful calculation of the self-force. However,
this approach is not generalizable to more complicated orbits, because of the direct
use made of the spherical symmetry of the background to reduce the number of
dimensions.
4.2 2C1D Numerical Simulations
One therefore needs 2C1D simulations. The problem with 2C1D calculations of the
self-force is twofold. First, obviously the computational problem is harder in 2C1D
than in 1C1D. The bigger problem, however, is that the mode-sum regularization
method was developed for the decomposition of the full (retarded) perturbation
field into multipole modes, specifically into ` modes, followed by the applica-
tion of a certain mode-by-mode regularization procedure that depends crucially on
the large ` behavior of the individual modes. Decomposition of the field is most
naturally done in 2C1D in azimuthal m modes, not multipoles `. One therefore
needs to first have a robust regularization scheme that is based on the field being
decomposed into azimuthal m modes. Such a regularization scheme was indeed
presented in [6].

The State of Current Self-Force Research 405
4.2.1 m-Mode Regularization
Letting the space-time of a rotating black hole be the background metric g
and the
(assumed small) perturbation due to the particle be the metric h
, one may write
the vacuum Einstein equations as G
˛ˇ
.g
Ch
/ D 8 T
˛ˇ
, which can be written
as a linearized perturbation over a fixed background in the Lorenz gauge (for the
trace-reversed metric perturbations) as follows (see [10] for details and definitions)
r
ˇ
r
ˇ
N
h
.x
˛
/ C2R
N
h
D16 T
; (12)
where the stress-energy tensor of a point particle of mass m moving along an arbi-
trary world-line x
˛
p
./ is given by Eq. 7.
As the Kerr space-time is axisymmetric, TD solutions are most convenient when
the computational domain is at least 2C1 dimensional (two space dimensions and
time). While 3 space dimensions are possible, the computational costs involved
would be high, in addition to the lack of a viable regularization method without
any mode decomposition. In 2C1 dimensions one solves for the azimuthal modes
of the full field. The linearized Einstein equations then take a form similar to Eq. 3.
In the context of the Schwarzschild space-time, the numerical solution was done in
double-null coordinates [5], which allows for an explicit expression of the solution
at a grid point in which it is unknown, when all expressions are evaluated at the
center of each computational cell. As the total field is decomposed into azimuthal
modes, the divergent behavior of each m mode can be found analytically. Indeed, in
the much simpler context of a Schwarzschild black hole background, this analytical
behavior was found explicitly in terms of a perturbation expansion [5]. Specifically,
the typical metric function h diverges approaching the particle as
h.x/
d
; (13)
where d is a measure of the distance from the evaluation point x to the point-like
particle (along a space-like geodesic). It can then be shown, that the m mode of the
field diverges logarithmically [5]. This situation is not as convenient as in the case
that the bare field is decomposed into spherical harmonic modes. In the latter case,
each `; m mode of the field is regular, even though the total field diverges. In our
case, although each m mode in itself is singular, the singular piece can be found an-
alytically. In the context of a Schwarzschild black hole, this logarithmic divergence
was handled by applying a so-called puncture scheme [5]. Specifically, outside a
world tube the numerical solution of the bare field is found. The world tube thick-
ness allows for a natural cut off to be introduced, so that no numerical divergences
occur. Inside the world tube, however, where the bare field grows unboundedly, one
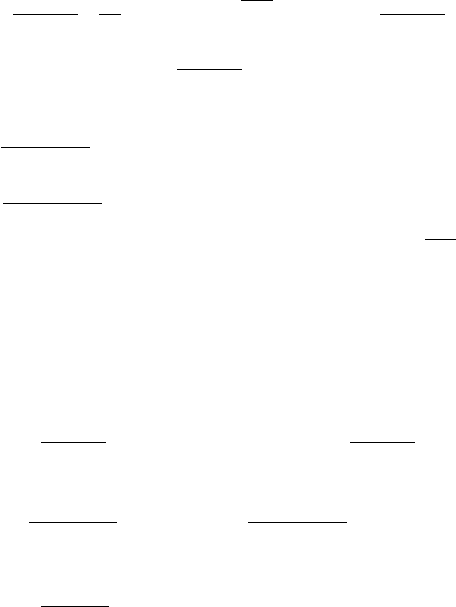
can introduce a “regular field mode” so that
h
m
R
WD h
m
h
m
p
; (14)
406 L.M. Burko
which can be solved numerically inside the world tube as it is regular. The key point
is that h
m
p
is known analytically (i.e., it has the same logarithmic divergence as the
full field). One can then use the known analytic relation of the bare and regular
fields, and match smoothly across the surface of the world tube.
4.2.2 The Square of the Geodesic Distance
The square of the geodesic distance is given by
S D S
0
C S
1
C S
2
C; (15)
where
S
0
D .g
˛ˇ
C u
˛
u
ˇ
/ıx
˛
ıx
ˇ
; (16)
S
1
D .g
˛ˇ
C u
˛
u
ˇ
/
ˇ
ıx
˛
ıx
ıx
: (17)
For a circular Schwarzschild orbit,
S
0
D
r 2M
r 3M
M
r
ıx
0
ıx
0
2
p
Mrıx
0
ıx
3
C
r
r 2M
ıx
1
ıx
1
Cr
2
ıx
2
ıx
2
C r
2
r 2M
r 3M
ıx
3
ıx
3
; (18)
S
1
D
M
.r 2M /
2
ıx
1
ıx
1
ıx
1
C
ıx
1
r
2
.r 3M /
h
M.r M/ıx
0
ıx
0
C r
3
.r 3M / ıx
2
ıx
2
Cr
3
.r M/ıx
3
ıx
3
2r.r M/
p
Mrıx
0
ıx
3
i
:
(19)
We would normally take a t D const hypersurface, and evaluate the square of the
geodesic distance on that hypersurface, that is, ıx
0
D 0. Our expressions then
simplify to
S
0
D
r
r 2M
ıx
1
ıx
1
C r
2
ıx
2
ıx
2
C r
2
r 2M
r 3M
ıx
3
ıx
3
; (20)
S
1
D
M
.r 2M /
2
ıx
1
ıx
1
ıx
1
C
ıx
1
r
2
.r 3M /
r
3
.r 3M / ıx
2
ıx
2
C r
3
.r M/ıx
3
ıx
3
: (21)
Finally "
P
D
p
S
0
C S
1
, neglecting terms of higher order.

The State of Current Self-Force Research 407
4.2.3 The Puncture Function
One introduces next the puncture function
P
˛ˇ
D
4
"
P
.x/
h
Nu
˛
Nu
ˇ
C
N
˛
Nu
ˇ
C
N
ˇ
Nu
˛
Nu
ıx
i
: (22)
Here, the barred quantities are defined as follows. First, the point Nz
˛
.t/ is a point
along the particle’s world line, at the time that the field point x
˛
is evaluated. That
is, it is the intersection of the spatial slice containing x
˛
with the particle’s world
line. Then, Nu
˛
and
N
˛ˇ
are evaluated on the particle’s world line at the same point.
Finally, here ıx
˛
WD x
˛
Nz
˛
.
The puncture function is applied for the trace-reverse metric perturbations, that
is,
P
˛ˇ
N
h
P
˛ˇ
.Wedefinetheresidual field
N
h
res
˛ˇ
WD
N
h
˛ˇ
N
h
P
˛ˇ
: (23)
Therefore,
r
r
N
h
˛ˇ
C 2R
˛ˇ
N
h
Dr
r
N
h
res
˛ˇ
C
N
h
P
˛ˇ
C 2R
˛ˇ
N
h
res
C
N
h
P
D
r
r
N
h
res
˛ˇ
C 2R
˛ˇ
N
h
res
C
r
r
N
h
P
˛ˇ
C 2R
˛ˇ
N
h
P
D16 T
˛ˇ
; (24)
so that the residual field satisfies the equation
r
r
N
h
res
˛ˇ
C 2R
˛ˇ
N
h
res
D16 T
˛ˇ
r
r
N
h
P
˛ˇ
C 2R
˛ˇ
N
h
P
WD Z
res
˛ˇ
:(25)
Notice, that the puncture field is known analytically. Numerically, Eq. 25 is the one
that needs to be evaluated.
Having found the regularized metric perturbations
N
h
res
˛ˇ
, one can now find the
self-force. Specifically,
f
˛
D
1
2
g
˛
C u
˛
u
h
res
I
C h
res
I
h
res
I
u
u
; (26)
or, alternatively,
f
˛
D k
˛ˇ ı
N
h
res
ˇIı
; (27)
where
k
˛ˇ ı
D
1
2
g
˛ı
u
ˇ
u
g
˛ˇ
u
u
ı
1
2
u
˛
u
ˇ
u
u
ı
C
1
4
g
ˇ
u
˛
u
ı
C
1
4
g
˛ı
g
ˇ
: (28)

408 L.M. Burko
Notice, that Eq. 26 is written for the residual piece of the metric perturbations, while
Eq. 27 is written for the trace-reversed perturbations.
This approach was applied successfully by Barack and Golbourn [5]forthe
scalar field self-force for a circular Schwarzschild orbit. Most importantly, even
though the Schwarzschild background is spherically symmetric, no use of this sym-
metry was done explicitly in [5]. Therefore, the generalization to the Kerr case, and
to more generic orbits, should be possible. The gravitational self-force counterpart
is now in progress [35].
5 Post-adiabatic Self-Force-Driven Orbital Evolution
5.1 The Importance of Second-Order Self-Forces
Adiabatic templates can describe EMRI waveforms to O.=M/
1
. To obtain the
self-force O.=M/
0
corrections, it is not sufficient to include the self-force to lead-
ing order in the small body’s mass : one needs also to include the self force to the
next order, that is, to O.
2
/. In support of this claim, we present here a simple ar-
gument, based on [14], for the simplest case of quasi-circular Schwarzschild orbits.
One should bear in mind, however, that the regularization problem of second-order
self forces is a rather difficult one, because it includes highly singular source terms
that make the standard retarded solutions diverge [44].
Let a nonspinning test body of mass be accelerated because of its self-force
f
SF
˛
in a quasi-circular orbit around a Schwarzschild black hole of mass M (sat-
isfying =M 1), such that its four-velocity is u
˛
and its proper time is .The
equations of motion (hereafter EOM) are given by
Du
˛
d
D f
SF
ˇ
g
˛ˇ
; (29)
which we solve perturbatively order by order in =M . The metric in the usual
Schwarzschild coordinates is given by
ds
2
DF.r/dt
2
C F
1
.r/ dr
2
C r
2
d˝
2
; (30)
where F.r/ D 1 2M=r. Here, d˝
2
D d#
2
C sin
2
#d
2
.
As the orbit is planar, the # component of the EOM is trivial. We use the nor-
malization condition for u
˛
, namely u
˛
u
˛
D1, to eliminate u
t
from the EOM
Du
i
=d D
1
f
SF
k
g
ik
, i; k D t;r;,whereD denotes covariant differentiation
compatible with the metric (30). We next use the t component of the EOM to elim-
inate Pu
t
. We can simplify the EOM to first-order (nonlinear) ordinary differential
equations by taking Pr D V.r/, Px D Vx
0
.r/,wherex denotes any quantity. We find
the EOM to be
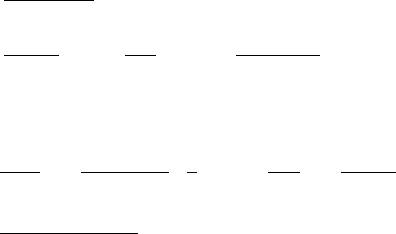
The State of Current Self-Force Research 409
VV
0
3MV
2
r.r 2M /
.r 2M /
1
.u
t
/
2
1
2M
r
f
SF
r
C
V
1 2M=r
f
SF
t
D 0; (31)
and
V
0
3MV
r
4
C 2
M=r
3
C
1 2M=r
2
r
V
1
3M
r
f
SF
t
.u
t
/
2
2.M=r
3
C /
1=2
.u
t
/
2
r
2
f
SF
D 0; (32)
where .u
t
/
2
D 1=Œ1 3M=r r
2
V
2
=.1 2M=r/. We denote by ˝ the orbital
frequency as measured at infinity, and by an overdot and a prime (partial) differen-
tiation with respect to coordinate time t and r, respectively. Here, measures the
deviation from Kepler’s law, that is,
˝
2
D M=r
3
C .r/: (33)
This last relation is gauge independent, although each of the terms on the right-
hand side (RHS) are separately gauge dependent. Specifically, we fix the gauge by
choosing the first term on the RHS to include the radial Schwarzschild coordinate
r: under an infinitesimal coordinate transformation x
˛
! x
0˛
Dx
˛
C
˛
where the
gauge vector
˛
DO./, the metric perturbations h
˛ˇ
! h
0
˛ˇ
Dh
˛ˇ
2r
.˛
ˇ/
.
In the last expression, the covariant derivative operator r
˛
is compatible with
the background metric (30). A gauge choice of the metric perturbationsh
˛ˇ
(e.g., the
Regge–Wheeler or the Lorenz gauges) is therefore equivalent to a condition on the
gauge vector
˛
. The latter may have a radial component, that changes the radial
coordinate description of the orbit. As noted by Detweiler [21], while the angular
velocity ˝ is gauge invariant, the radius of the orbit r is not. This means that un-
der a gauge transformation the different terms on the RHS of Eq. 33 change, but in
such a manner that their combination, or the left-hand side (LHS) of (33)isinvari-
ant. One may, therefore, fix the gauge (possibly up to a residual gauge freedom) by
determining the ratio of the terms on the RHS of (33), or, alternatively, by fixing the
geometrical meaning of the symbol r appearing on the RHS of (33). In particular,
the latter may be fixed to equal the Schwarzschild radial coordinate of the unper-
turbed Schwarzschild background (30). By clarifying the geometrical meaning of
r we therefore effectively fix the gauge. Notice that this fixing is not equivalent to
merely choosing which coordinates are used to describe the background geometry.
Our analysis below does not depend, however, on the choice of gauge. One may
indeed, for the purpose of the present argument, just argue that the LHS of (33)is
determined in some gauge.
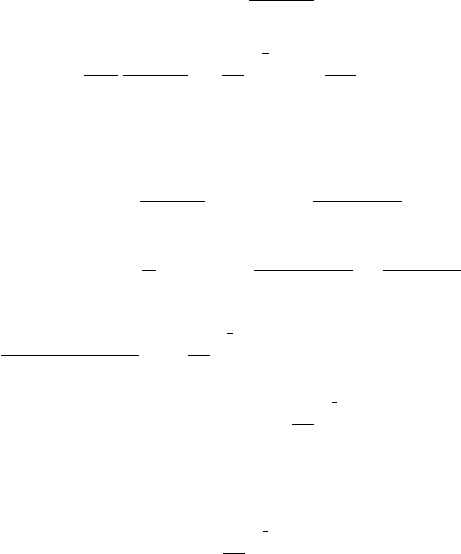
We next expand in powers of " WD =M (do not confuse with the symbol " used
above, denoting the orbital eccentricity) as .r/ D
.1/
C
.2/
, V D V
.1/
C V
.2/
,
410 L.M. Burko
and a
i
D a
.1/
i
C a
.2/
i
, x
.j /
denoting the term in x which is at O."
j
/,anda
i
being
the (self) acceleration. We then expand the self-force as f
SF
i
D f
.1/
i
C f
.2/
i
,where
f
.j /
i
D a
.j /
i
. Solving perturbatively, we find the first-order terms to be
.1/
D
r 3M
r
2
f
.1/
r
; (34)
V
.1/
D
2r
M
r 3M
r 6M
"
M
r
1
2
1
2M
r
f
.1/
C Mf
.1/
t
#
; (35)
and the second-order terms to be
.2/
D
r 3M
r
2
"
f
.2/
r
C r
2
V
.1/
f
.1/
t
.r 2M /
2
#
C
r
.1/
f
.1/
r
3M V
.1/
2
r.r 2M /
2
C
V
.1/
V
.1/
0
r 2M
; (36)
V
.2/
D
r.r 3M /
2
M
2
.r 6M /
2
"
2
M
r
1
2
f
.1/
f
.1/
0
r
r.r 2M /
2
.r 3M / C
M
r
1
2
f
.1/
f
.1/
r
.5r 6M /.r 2M /
.r 3M / C 2Mf
.1/
t
f
.1/
0
r
r
2
.r 2M /.r 3M /
C4Mf
.1/
t
f
.1/
r
r
2
.r 3M / C 2M
2
f
.2/
t
.r 6M /
C 2
M
r
3
2
f
.2/
.r 2M /.r 6M /
#
: (37)
The first-order corrections for the orbital parameters, given in Eqs. 34 and 35,
involve dissipative and conservative effects. Dissipation is included in Eq. 35,
whereas Eq. 34 involves conservative changes to the particle’s world line. Indeed,
the latter depends only on f
.1/
r
, that for circular Schwarzschild orbits is purely con-
servative.
The second-order corrections to the orbital parameters, given by Eqs. 36 and 37
mix conservative and dissipative effects, and includes terms that are quadratic in
f
.1/
i
, in addition to terms that are linear in the second-order self-force, namely f
.2/
i
.
The key element in our argument, is now to show that the O.=M/
0
cor-
rections to the gravitational waveforms depend on Eqs. 36 and 37, and therefore
depend on f
.2/
i
.
We can study the importance of the higher-order correction by considering
two dimensionless quantities, d N
cyc
=d.ln f/, the number of orbits spent in a
logarithmic interval of frequencies, and V=.r!/. Then, we compare these quantities
