Bird R.B., Stewart W.E., Lightfoot E.N. Transport Phenomena

Подождите немного. Документ загружается.

s24.6 Mass Transport in Porous Media

797

Table

24.6-1

Experimental Verification of Graham's Law

IT.

Graham,

Phil.

Mag., 2,175,269,351 (1833);

Mason

and

Kronstadt, IMP-ARO(D)-12, University of Maryland,

Institute for Molecular Physics, March 20,19671.

Gas Grahama Mason and ~ronstadtl'

\%)

calculations can be used for microporous membranes, but only if there are no significant

intermolecular forces between the solutes and the pore walls.

Modeling viscous flow in these systems has already been discussed in 56.4, and it is

common practice to describe such a flow, for the low Reynolds numbers of most interest

here, by the Blake-Kozeny expression (Eq. 6.4-9) [see however Rhodes5 (Chapter

5),

Sahimi2 (Chapter 6), Stanitk2 (Chapter

3)]:

Here

is the superficial mass-average velocity. Note from the discussion of 519.2 that

the velocity used here is the mass-average velocity of the fluid through the porous

material.

To obtain macroscopic descriptions we may use the generalized Maxwell-Stefan

equations (Eq. 24.5-4), and we shall restrict ourselves here to concentration- and

pressure-driven flow. Moreover, when the mobile species are small relative to pore di-

mensions, the boundary conditions simplify to continuity of species concentration and

pressure at the interface between the external and "intrapore" fluid.

Simplify the Maxwell-Stefan equations for the diffusion of a binary dilute solution,

a large

solute species

in a solvent

through a macroporous medium M, a matrix with pores large

Transport *om a

compared to the diameters of both mobile species, but small enough that lateral concentration

Binary External

gradients within each pore may be neglected.

Solution

SOLUTION

We begin by determining the pressure-flow relationship and note that we have two ways of

doing this: the Blake-Kozeny equation

(Eq.

24.6-14), and the diffusion-based result

(Eq.

24.5-

20) of the previous section.

For high velocities through the porous material and pores large relative to molecular di-

mensions, it is the mass-average velocity that must be proportional to the pressure gradient,

798

Chapter 24 Other Mechanisms for Mass Transport

and we may assume that the Blake-Kozeny equation governs the flow. We begin by rewriting

Eq. 24.6-14) as

and Eq. 24.5-20 as

Equating the coefficients of v, and

in these two equations then yields descriptions of

Di,

and

BLM,

respectively. If the pores are small relative to the molecular dimensions and the

mass-average velocity is not large relative to the diffusional velocities v,

one is in a still

poorly studied flow region, and one must resort either to experiment or to an appropriate

molecular model.17

To determine the rate of solute transport, we turn to

Eq.

24.5-25

noting that the diffusivities

that section already include the factor

E/T.

However, if the pore dimensions are very large with

respect to the effective diameters of the solute and solvent molecules, the ratio

BAB/BLM

will

be very small. We can thus obtain

in which

where the superscript ext refers to conditions in the external solution of the same composition

as the pore fluid. Equation 24.6-17 may in turn be rewritten as

which is Fick's first law modified for void fraction and tortuosity. It is widely used.

Exactly as in unconfined fluids, one cannot determine net flow, or pressure drop, from

diffusional considerations alone. One needs a

flux

ratio or equivalent.

specific example is

supplied by freeze-drying, where water vapor must diffuse through a porous region of dried

solid and where inert gases may be assumed stagnant. This region is also interesting in that

conditions can vary from simple continuum diffusion, as here, through the slip-flow region,

and on to the Knudsen region.4b

It must be remembered that Eq. 24.6-19 and the equations leading up to it represent only

the direct effect of molecular diffusion. The convective dispersion resulting from interparticle

mixing and local departures from rectilinear flow must be added when using the volume-

averaged convective equation (see 520.5, Butt12 g5.2.5, and ~evens~iel'~ 513.2).

QUESTIONS FOR DISCUSSION

How does equilibrium thermodynamics have to be supplemented in order to study non-

equilibrium systems, such as those that involve velocity, temperature, and concentration

gradients?

What new transport coefficients arise in multicomponent mixtures and what do they

describe?

To what extent does this chapter explain the origin of

Eq.

19.3-3? Is that equation completely

correct?

Is Eq. 24.1-6 really the starting point for the derivation of the complete expressions for the

fluxes? Discuss its origin.

Z.-Y.

Yan,

Weinbaum, and

Pfeffer,

Fluid

Mech.,

162,415438

(1986).

Problems

799

How are the thermal diffusion coefficient, the thermal diffusion ratio, and the Soret coeffi-

cient defined? Can the signs of these quantities be predicted a priori?

How can one start with

Eq.

24.2-8 and obtain Eq. 17.9-I? What restrictions have to be placed

on Eq. 17.9-I?

What is the proper driving force for diffusion: the gradient of the concentration, the gradient

of the activity, or some other quantity?

Discuss the Clusius-Dickel column for isotope separation.

To describe the steady-state operation of an ultracentrifuge it is not necessary to know any

transport properties. Does this seem odd?

10.

What various physical phenomena need to be understood in order to describe diffusion in

porous media?

PROBLEMS

24A.1.

Thermal diffusion.

(a) Estimate the steady-state separation of

and

occurring in the simple thermal diffu-

sion apparatus shown in Fig. 24.2-1 under the following conditions:

is 200K,

is 600K, the

mole fraction of deuterium is initially 0.10, and the effective average

is 0.0166.

(b) At what temperature should this average

have been evaluated?

Answers:

(a) The mole fraction of

is higher by 0.0183 in the hot bulb

(b) 330K

24A.2.

Ultracentrifugation of proteins. Estimate the steady-state concentration profile when a typi-

cal albumin solution is subjected to a centrifugal field 50,000 times the force of gravity under

the following conditions:

Cell length

1.0 cm

Molecular weight of albumin

45,000

Apparent density of albumin in solution

M~/V~

1.34 g/cm3

Mole fraction of albumin (at

O),

XAO

Apparent density of water in the solution

1.00 g/cm3

Temperature

75°F

Answer:

exp(-22.7z), with

in cm

24A.3.

Ionic diffusivities. The limiting (that is, at zero concentration) equivalent ionic conduc-

tances, in dimensions of cm2/ohm

g-equiv for the following ions at 25°C are:' Na', 50.10;

K',

73.5; C1-, 76.35. Calculate the corresponding ionic diffusivities from the definion

Note that F

96,500 coulombs/g-equiv, RT/F

25.692 mv at 25OC, and

coulomb

am-

pere. s.

24B.1.

The dimensions of the Lorentz force. Show how the Lorentz force on a charge moving

through a magnetic field corresponds to the first term added to the linear d, of Eq. 25.4-51

and gives a consistent set of units for this quantity.

Suggestion:

Note that cRTd, represents the

motive force for diffusive motion of species

per unit volume and that the usual dimensions

of the magnetic induction are 1 Weber

Newton-second/Coulomb-meter.

24B.2.

Junction potentials. Consider two well-mixed reservoirs of aqueous salt at 25OC, as in Fig.

24.4-2, separated by a stagnant region. Salt concentrations are 1.0 N on the left (1) and 0.1

on the right (2). Estimate junction potentials for NaCl and for KC1 using the ion diffusivities

Robinson and R.

Stokes,

Electrolyte Solutions,

revised edition, Butterworths, London

(1965),

Table

6.1.

800

Chapter 24 Other Mechanisms for Mass Transport

of Problem 24A.3. Assume constant ion activity coefficients. Which compartment will be the

more positive? Why?

Donnan exclusion. The sulfonic acid membrane used by scattergood' had the following

equilibrium internal composition when immersed in 0.1 N NaC1:

Organic sulfonic acid polymer

1.03 g-equiv/liter

Water

13.2 g-equiv/liter

Chloride ion

c,--

0.001 g-equiv/liter

Sodium ion

c,,+

1.031 g-equiv/liter

Calculate the distribution coefficient of sodium chloride

Note that the concentration of water in the external solution is about 55.5 g-mol/liter.

Answer:

0.064

Osmotic pressure. Typical sea water, containing 3.45% by weight of dissolved salts, has a

vapor pressure 1.84% below that of pure water. Estimate the minimum possible transmem-

brane pressure required to produce pure water, if the membrane is ideally selective.

Answer:

about 25 atm

Permeability of

perfectly selective filtration membrane. Develop an expression for the hy-

draulic permeability of the perfectly selective membrane described in Example 22.8-5 in terms

of the diffusional parameters introduced in 324.5.

Answer:

BLJRTS, where

is the membrane thickness

Model insensitivity. In modeling a porous medium as a parallel network of channels one

must allow both for the toruous nature ("tortuosity"

of real systems and also the restriction

of the transport to the fraction

of the cross section that is available for flow. Equation 24.6-3

then must be modified to

An alternate approach is to consider the transport process to be a diffusion of species

through an immobilized set of giant molecules3 (these particles comprising the porous

medium). This model yields the expression

Compare these two equations, noting that the value of is often about 0.4.

Expressions for the mass

flux.

(a) Show how to transform the left side of Eq. 24.2-8 into the left side of Eq. 24.2-9. First

rewrite the former as follows:

Rewrite the second term as a sum over

all

and then add a term to compensate for the modi-

fication of the sum. Note that this change has introduced into the sum a term containing Baa,

Scattergood and E.

Lightfoot,

Trans. Faraday

Soc.,

64,1135-1146 (1968).

Evans,

111,

Watson, and

Mason,

Chem. Phys.,

35,2076-2083

(1961).

Problems

801

which was not defined because it was not needed. Now, we are at liberty to define

B,,

in any

way we choose, and the choice we make is

--X-

Baa p=lDap

X-=o

P=1

Of,

all

This choice enables us to obtain the left side of Eq. 24.2-9, and also the auxiliary relation given

after Eq. 24.2-9 is, in fact, just Eq. 24C.1-3 above.

(b)

Next repeat the above derivation by replacing

[vP

(DE/pp)V

TI,

and verify that

both the diffusion terms and the thermal diffusion terms of Eq. 24.2-8 may be transformed

into the corresponding terms in Eq. 24.2-9.

24C.2.

Differential centrifugation. The lysing (bursting) of

coli cells has produced a dilute sus-

pension of inclusion bodies, hard insoluble aggregates of a desired protein, unlysed cells, and

unwanted dissolved proteins. For purposes of this problem all may be considered as spheres

with the properties indicated here.

Cells Inclusion bodies Proteins

Mass or equivalent 1.89

g 2.32

g 50 kilodaltons

Density (g/ml)

.07 1.3

1.3

Can these materials be effectively separated by centrifugation? Explain.

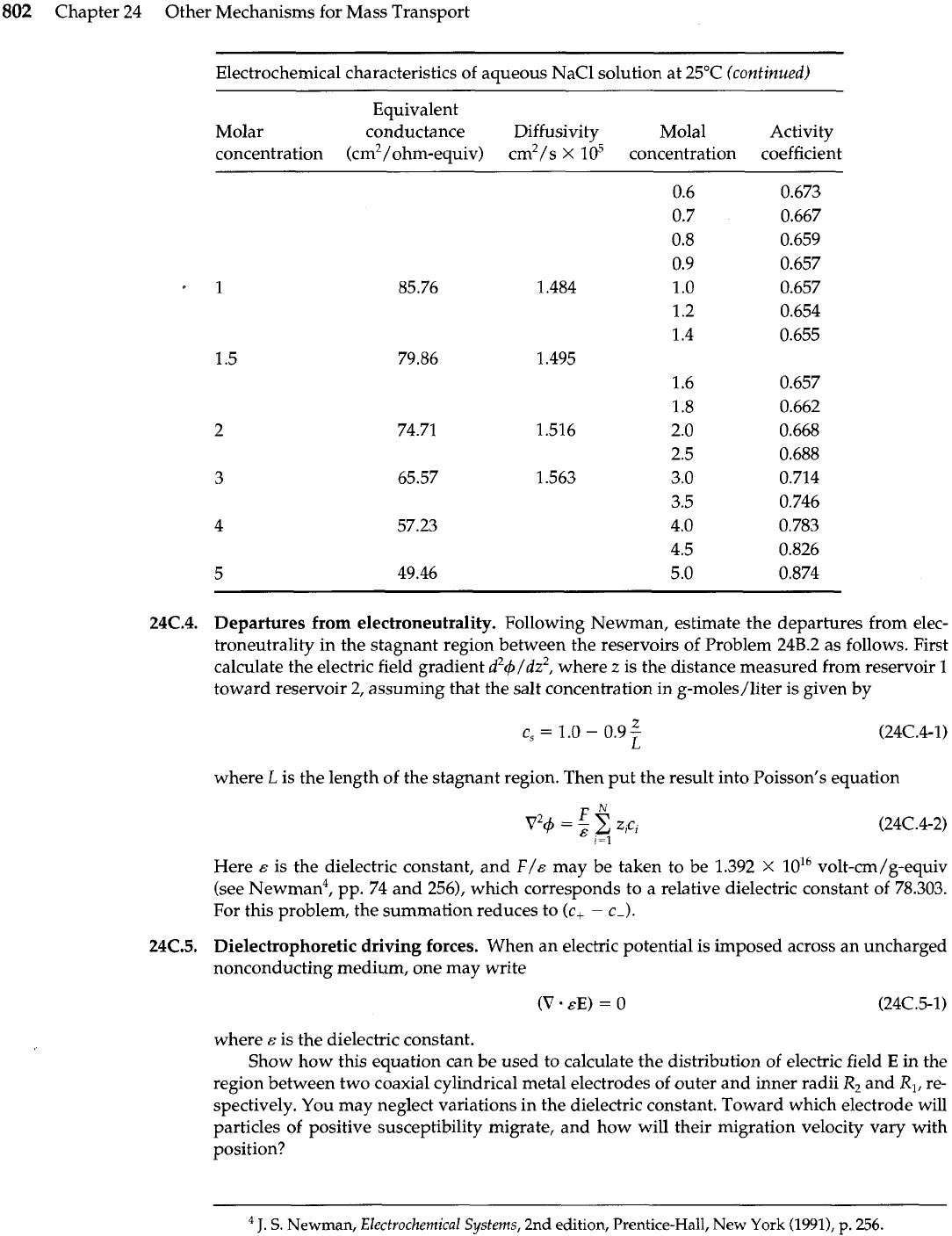
24C.3.

Transport characteristics of sodium chloride. In the accompanying table1 equivalent con-

ductance, diffusivity, and thermodynamic activity coefficients are given for sodium chloride

at 25°C. The first two are given as functions of the molarity (M), and the third for molality (m).

It may be assumed for the purposes of this problem that M/m

0.019m. Limiting ionic

equivalent conductances (that is, at infinite dilution) are 50.10 and 76.35, respectively. The salt

equivalent conductance in turn is defined as

where the specific conductance

KSp

L/AR,

where

is the resistance of a volume of solution

of length

and cross-sectional area

Use these data to discuss the sensitivity of the solution

behavior to the three diffusivities

BNa1,, B,,

and

BK,lcl

needed to describe this response

to solution concentration.

Electrochemical characteristics of aqueous NaCl solution at 25OC

Equivalent

Molar

conductance Diffusivity Molal

Activity

concentration (cm2/ohm-equiv) cm2/s

lo5

concentration coefficient

802

Chapter 24 Other Mechanisms for Mass Transport

Electrochemical characteristics of aqueous NaCl solution at 25OC

(continued)

Equivalent

Molar conductance

Diffusivity

Molal Activity

concentration (cm2/ohm-equiv) cm2 /s

concentration coefficient

24C.4.

Departures from electroneutrality. Following Newman, estimate the departures from elec-

troneutrality in the stagnant region between the reservoirs of Problem

24B.2

as follows. First

calculate the electric field gradient d24/dz2, where z is the distance measured from reservoir

toward reservoir

assuming that the salt concentration in g-moles/liter is given by

where

is the length of the stagnant region. Then put the result into Poisson's equation

Here

is the dielectric constant, and F/E may be taken to be 1.392

1016

volt-cm/g-equiv

(see Newman4, pp. 74 and 256), which corresponds to a relative dielectric constant of 78.303.

For this problem, the summation reduces to

(c,

c-).

24C.5.

Dielectrophoretic driving forces. When an electric potential is imposed across an uncharged

nonconducting medium, one may write

where

is the dielectric constant.

Show how this equation can be used to calculate the distribution of electric field

in the

region between two coaxial cylindrical metal electrodes of outer and inner radii R, and R,, re-

spectively. You may neglect variations in the dielectric constant. Toward which electrode will

particles of positive susceptibility migrate, and how will their migration velocity vary with

position?

Newman,

Electrochemical

Systems,

2nd

edition,

Prentice-Hall, New York (19911,

256.

Problems

803

Effects

small inclusions in a dielectric medium. The production of field nonlinearities by

embedded particles can be illustrated by considering the limiting case of a single particle of

radius

in an otherwise uniform field. The field distribution in both the external medium

and the particle are defined by Laplace's equation,

V2+

0, and by the boundary condition

on the sphere surface (here the indices s and

stand for sphere and continuum).

Develop expressions for

and

+,,

ArcosO for large r.

Frictionally induced selective filtration. Describe the glucose rejection behavior of a cello-

phane516 that shows no thermodynamic rejection. You may assume glucose mole fraction in

the feed to the membrane to be 0.01 and the following properties:

Here the subscripts

w, and

refer to glucose, water, and the membrane matrix, respectively.

Partial answer: The high-flow-limiting mole fraction of sugar in the filtrate is 0.00242.

Thermodynamically induced selective filtration. Describe the behavior of the hypothetical

membrane for which

1.0, solute activity coefficients are unity, and

B,',/B~,,

v,,/V,.

Partial answer: The high-flow limiting product solute concentration is 0.1 times that in the

feed.

Facilitated transport. Consider here the transport of a solute S across a homogeneous mem-

brane from one external solution to another as a complex CS with a carrier C unable to leave

the membrane phase. The solute

may be considered to be insoluble in the membrane and

convection to be negligible (see Fig. 24C.8). Assume further that:

Equilibrium exists at both membrane surfaces according to

ccs

K~c~cs

(24C.9-1)

where the concentration of S is that in the external solution, and those of C and CS are in

the membrane.7

Both C and CS follow the simple rate expression

D,,Aci.

Develop a general expression for the transport rate of

in terms of the total amount of carrier

plus carrier complex present in the membrane, the solution concentrations of S, the quantity

K,,

and the diffusivities. What is the maximum rate of transport of S (that is, as its concentra-

tion at the left of the diagram becomes very high and that at the right is zero)?

Membrane

Fig.

24C.8.

Elementary facilitated transport. Concentra-

tion profiles for the solute (S), the carrier (C), and the

Distance

complex (CS).

Ginzburg and

Katchalsky,

Gen. Physiol.,

47,403418 (1963).

Kaufmann and

Leonard,

AlChE Journal,

14,110-117 (1968).

See, however,

D. Goddard,

Schultz, and

Bassett,

Chem. Eng. Sci.,

25,665-683 (1970),

and

D. Stein,

The Movement

Molecules across Cell Membranes,

Academic Press, New York

(1984).

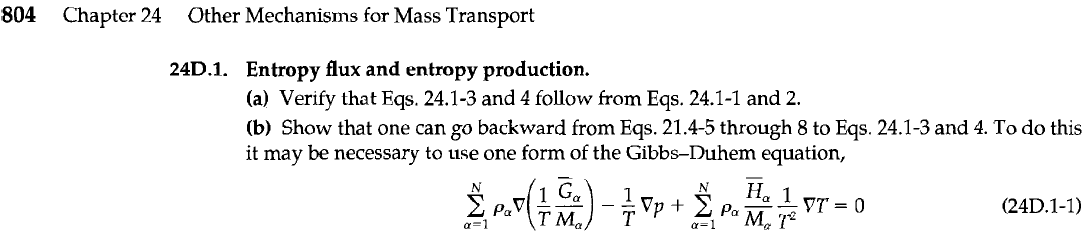
804

Chapter 24 Other Mechanisms for Mass Transport

24D.1.

Entropy

flux

and entropy production.

(a) Verify that Eqs. 24.1-3 and 4 follow from Eqs. 24.1-1 and

(b)

Show that one can go backward from Eqs. 21.4-5 through

to Eqs. 24.1-3 and 4. To do this

it may be necessary to use one form of the Gibbs-Duhem equation,

Postface

Of all the messages we have tried to convey in this long text, the most important is to

recognize the

key

role of the equations of change, developed in Chapters 3,11, and

19.

Writ-

ten at the microscopic continuum level, they are the key link between the very complex

motions of individual molecules and the observable behavior of most systems of engi-

neering interest. They can be used to determine velocity, pressure, temperature, and

concentration profile, as well as the fluxes of momentum, energy, and mass, even in

complicated time-dependent systems. They are applicable to turbulent systems, and

even when complete a priori solutions prove infeasible, simplify the efficient use of data

through dimensional analysis. Integrated forms of the equations of change provide the

macroscopic balances.

No introductory text can, however, meet the needs of every reader. We have at-

tempted, therefore, to provide a solid basis in the fundamentals needed to tackle presently

unforeseen applications of transport phenomena in an intelligent way. We have also

given extensive references to sources where additional information can be found. Some

of these references contain specialized data or introduce powerful problem-solving tech-

niques. Others show how transport analysis can be incorporated into equipment and

process design.

We have therefore concentrated on relatively simple examples that illustrate the

characteristics of the equations of change and the kinds of questions they are capable of

answering. This has required largely neglecting the very powerful numerical techniques

available for solving difficult problems. Fortunately, there are now many monographs

numerical techniques and packaged programs of greater or lesser generality. Graphics

programs are also available, which greatly simplify the presentation of data and simula-

tions.

It should also be recognized that great advances are being made in the molecular

theory of transport phenomena, ranging from improved techniques for predicting the

transport properties to the development of new materials. Molecular dynamics and Brown-

ian dynamics simulation techniques are proving to be very powerful for understanding

such varied systems as ultra-low density gases, thin films, small pores, interfaces, col-

loids, and polymeric liquids.

Simple models of turbulent transport have been included, but these are only a modest

introduction to a large and important field. Highly sophisticated techniques have been

developed for specialized areas, such as predicting the forces and torques on aircraft, the

combustion processes in automobiles, and the performance of fluid mixers. It is hoped

that the interested reader will not stop with our very limited introductory discussion.

Conversely, we have greatly expanded our coverage of boundary-layer phenomena,

because its importance and power are now being recognized in many applications. Once

primarily the province of aerodynamicists, boundary-layer techniques are now widely

used in many fields of heat and mass transfer, as well as in fluid mechanics. Applications

abound in such varied fields as catalysis, separation processes, and biology.

Of great and increasing importance is non-Newtonian behavior, encountered in the

preparation and use of films, lubricants, adhesives, suspensions, and emulsions. Biologi-

cal examples are exceedingly important, ranging from the operation of the joints to drag-

reducing slimes on marine animals, and down to the very basic problem of digesting

foodstuffs.

No music and no oral communication would be possible without compressible

pow,

area we have neglected because of space limitations. Compressible flow is also of critical

806

Postface

importance in the design of airplanes, re-entry vehicles in our space program, and in pre-

dicting meteorological phenomena. The awesome destructive power of tornadoes is a

challenging example of the latter.

Some problems involving transport phenomena in

chemically reacting systems

have

been presented. For simplicity, we have taken the chemical kinetics expressions to be of

rather idealized forms. For in combustion, flame propagation, and explosion

phenomena more realistic descriptions of the kinetics will be needed. The same is true in

biological systems, and the understanding of the functioning of the human body will re-

quire much more detailed descriptions of the interactions among chemical kinetics,

catalysis, diffusion, and turbulence.

In basic terms, each of us is internally powered by the close equivalent of fuel cells,

with current carried primarily by cations, in particular protons, rather than electrons.

There are also

complex electrical transport phenomena

taking place in the now ubiquitous

microelectronic devices such as computers and cellular phones. We have provided a

very modest introduction to electrotransport, but again the reader is urged to go on to

more specialized sources.

No engineering project can be conceived, let alone completed, purely through use of

the descriptive disciplines, such as transport phenomena and thermodynamics. Engi-

neering, in the last analysis, depends heavily on heuristics to supplement incomplete

knowledge. Transport phenomena can, however, prove immensely helpful by providing

useful approximations, starting with order-of-magnitude estimates, and going on to suc-

cessively more accurate approximations, such as those provided by boundary-layer the-

ory. It is therefore important, perhaps in a second reading of this text, to seek

shape- and

model-insensitive descriptions

by examining the numerical behavior of our model systems.