Benzoni-Gavage S., Serre D. Multi-dimensional Hyperbolic Partial Differential Equations: First-order Systems and Applications

Подождите немного. Документ загружается.

Well-posedness in Sobolev spaces 65

Existence in C ([0,T]; H

s−1

) We note that the adjoint operator

∗

= −∂

−



(x, t)

∂

−



∂

(x, t)

− B

(or its opposite, to ﬁt with the standard time evolution) is also symmetrizable.

As a matter of fact, Σ(t)

−1

is a functional symmetrizer for P (t)

∗

. In particular,

the estimate (2.1.9) in Remark 2.1 can be applied to L

∗

. Now we introduce the

space

E := {ϕ ∈ C

∞

([0,T]; H

+∞

)) ; ϕ(T )=0}.

Applying (2.1.9) to −s (where s is the regularity index of the source term f)and

∗

instead of L yields the estimate

ϕ(t)

−s

≤ C



L

∗

ϕ(τ)

−s

dτ (2.1.15)

for all ϕ ∈ E and t ∈ [0,T]. Hence the operator L

∗

restricted to E is one-to-one.

This enables us to deﬁne a unique linear form  on L

∗

E by

(L

∗

ϕ)=



f(t) ,ϕ(t) 

−s

dt + g, ϕ(0) 

−s

. (2.1.16)

By (2.1.15) and the Cauchy–Schwarz inequality, we see that

(L

∗

ϕ)

≤ 2 C ( T f

(0,T ;H

)

+ g

) L

∗

ϕ

(0,T ;H

−s

)

By the Hahn–Banach theorem,  thus extends to a continuous form

on L

(0,T; H

−s

). And by the Riesz theorem, we ﬁnd u ∈ L

(0,T; H

−s

)



such that

(L

∗

ϕ)=



u(t) ,L

∗

ϕ(t) 

−s

dt (2.1.17)

for all ϕ ∈ E . In particular, for ϕ ∈ D (R

× (0,T)) we get that



f(t) ,ϕ(t) 

−s

dt =



Lu(t) ,ϕ(t) 

−s

dt.

In other words, we have Lu = f in the sense of distributions. This implies that

∂

u = P (t)u + f belongs to L

(0,T; H

s−1

), hence u belongs to C ([0,T]; H

s−1

Finally, integrating by parts in (2.1.17), substituting Lu = f and using (2.1.16),

we obtain

g, ϕ(0) 

−s

= u(0) ,ϕ(0) 

s−1

−s+1

for all ϕ ∈ D (R

× [0,T)). A standard argument then shows that u(0) = g.

66 Linear Cauchy problem with variable coeﬃcients

Regularity If f belongs to C

∞

([0,T]; H

+∞

)) and g belongs to H

+∞

)

then u belongs to C ([0,T]; H

+∞

)) from the above construction applied to

arbitrary large s. The equation ∂

u = P (t)u + f then implies that u belongs

to C

([0,T]; H

+∞

)) for all k ∈ N.Iff and g are less regular, we can use

molliﬁers to construct sequences f

∈ D (R

× [0,T]) and g

∈ D (R

) such that

(0,T ;H

)

−−−−−−−→

k→∞

f, g

−−−−→

k→∞

For all k there is a solution u

∈ C

∞

([0,T]; H

+∞

)) corresponding to the

source term f

and the initial data g

. Applying the estimate in (2.1.8) to u

−

yields the inequality

u

(t) − u

(t)

≤ C



g

− g





f

− f

(τ)

dτ



for all k, m ∈ N.Thisimpliesthat(u

) is a Cauchy sequence in C ([0,T]; H

)

and thus converges, say towards u ∈ C ([0,T]; H

). In the limit we have Lu = f

and u(0) = g. By uniqueness (in C ([0,T]; H

s−1

)), we have u = u, the solution

constructed by a duality argument. Observe then that u = u satisﬁes the energy

estimate in (2.1.14) by passing to the limit in (2.1.8) applied to u

. This completes

the proof. 

Remark 2.8 The end of this proof is sometimes referred to as a weak=strong

argument. Indeed, if we say a strong solution is a solution that is the limit of

inﬁnitely smooth solutions of regularized problems, and a weak solution the one

obtained by duality, it shows that any weak solution is necessarily a strong one.

Remark 2.9 This theorem is also valid backward, that is, prescribing u(T )

instead of u(0). This fact will be used in the application of the Holmgren Principle

below.

Remark 2.10 In the proof above, we have crucially used the inﬁnite smooth-

ness of coeﬃcients. In fact, H

-well-posedness is also true for H

coeﬃcients

provided that s is large enough. We reproduce from [132] the corresponding

precise result below, which is the continuation of Theorem 2.4 and is useful in

non-linear analysis.

Theorem 2.7 Assume s>

+1 and take T>0. For a function v belonging

to L

∞

([0,T]; H

)) ∩ C ([0,T]; H

)) and such that

∂

v ∈ L

∞

([0,T]; H

s−1

)) ∩ C ([0,T]; H

s−1

)),

we consider the diﬀerential operator

= ∂



(v(x, t)) ∂

where the n × n matrices A

are C

∞

functions of their argument v ∈ R

Well-posedness in Sobolev spaces 67

If A(v, ξ)=



(v)ξ

admits a symbolic symmetrizer, then for all

f ∈ L

∞

([0,T]; H

)) ∩ C ([0,T]; H

)) and g ∈ H

the Cauchy problem

u = f, u(0) = g

has a unique solution u ∈ L

([0,T]; H

)). Furthermore, u belongs to

C ([0,T]; H

)) and enjoys an estimate

u(t)

≤ K e

γt

u(0)

+ C



γ(t−τ)

L

u(τ)

dτ,

where K>0 depends boundedly on v

∞

([0,T ];W

1,∞

))

and C, γ depend bound-

edly on

sup



v

∞

([0,T ];H

))

, ∂

v

∞

([0,T ];H

s−1

))



Sketch of proof As for Theorem 2.6, the existence part of the proof uses the

adjoint operator

∗

= −∂

−



∂

(v))

of L

and energy estimates in the space of negative index H

−s

. The existence of a

symmetrizer for L

∗

,namelyS

−1

, is of course crucial to derive those estimates. By

Theorem 2.4 it does imply an energy estimate, though a priori only in H

with

+ 1. However, as already observed in Remark 2.3, the only place where

the proof of Theorem 2.4 makes use of the restriction on m is in the comparison

between L

∗

and

∗

= −∂

−



∂

(v))

∗

If we invoke Proposition C.15, which shows that

(L

∗

− P

∗

) φ

−s

≤ C(v

) φ 

−s

for all φ ∈ C

∞

× [0,T]), and perform the estimate of φ

−s

in terms of

P

∗

φ

−s

as in the proof of Theorem 2.4, we eventually obtain

φ(t)

−s

≤ K e

γt

φ(0)

−s

+ C



γ(t−τ)

L

φ(τ)

−s

dτ

for all φ ∈ D (R

× [0,T]). Once we have this estimate, the same arguments

as in the proof of Theorem 2.6 show the existence of a solution u ∈

([0,T],H

). Using the equation L

u = f , we see that additionally u ∈

([0,T]; H

s−1

)) → C ([0,T]; H

s−1

)).

The rest of the proof consists in showing more regularity on u.Thiscan

be done thanks to the estimate in Theorem 2.4 for m = s and a weak-strong

68 Linear Cauchy problem with variable coeﬃcients

argument, the latter being also used to prove u satisﬁes the H

energy estimate

(hence its uniqueness). See [132] for more details. (Also see the proof of Theorem

2.8 hereafter for a similar method in L

.) 

Theorem 2.7 deals with smooth solutions. There is also a L

-well-posedness

result for Lipschitz coeﬃcients, relying on the energy estimate (2.1.12) in Theo-

rem 2.5, which can be stated as follows.

Theorem 2.8 Let v belong to W

1,∞

× (0,T)), and consider the operator

= ∂



(v(x, t)) ∂

where A

are C

∞

functions of their argument v ∈ R

and A(v, ξ)=



(v) admits a symbolic symmetrizer. Then for all f ∈ L

× (0,T))

and g ∈ L

), the Cauchy problem

u = f, u(0) = g

has a unique solution u ∈ C ([0,T]; L

)), which enjoys the estimate

u(t)

≤ K



γt

u(0)



γ(t−τ)

f(τ)

dτ



where K>0 and γ>0 depend boundedly on v

1,∞

)

Proof Using the energy estimate (2.1.12) in Theorem 2.5, we can ﬁnd a weak

solution u ∈ L

([0,T]; L

)) by the same duality argument as in the proof of

Theorem 2.7, with H

±s

replaced by L

. It remains to show u in fact belongs to

C ([0,T]; L

)), and does satisfy the L

energy estimate in (2.1.12).

We take a mollifying kernel ρ ∈ D

∞

; R

), deﬁne ρ

(x)=ε

−d

ρ(x/ε), and

consider the operator R

associated with the convolution by ρ

.Thenu

:= R

belongs to L

([0,T]; H

+∞

)) and goes to u in L

([0,T]; L

)), and similarly

for f

:= R

f, while g

:= R

g belongs to H

+∞

) and goes to g in L

)when

ε goes to zero. Furthermore, by Theorem C.14 we have

lim

ε→0

[L

]u(t)

)

=0.

Therefore,

∂

= f

+ L

− [L

belongs to L

([0,T]; L

)), and consequently u

belongs to H

× [0,T]).

Thanks to Remark 2.4 this additional regularity of u

allows us to apply the

energy estimate in (2.1.12) (Theorem 2.5) to u

, and of course also to u

− u



Well-posedness in Sobolev spaces 69

Hence

u

(t) − u



(t)

≤ K



γt

u

(0) − u



(0)



γ(t−τ)

L

(τ) − L



(τ)

dτ



for all ε, ε



∈ (0, 1). Since u

(0) = g

goes to u(0) = g in L

), and (using

the Lebesgue theorem in the time direction),

= f

+[L

goes to L

u = f in L

× (0,T)), the inequality here above implies that

) is a Cauchy sequence in C ([0,T]; L

)). By uniqueness of limits in

([0,T]; L

)) (and the Lebesgue theorem again), u is necessarily the limit

of (u

)inC ([0,T]; L

)). Finally, by passing to the limit in

u

(t)

≤ K



γt

u

(0)



γ(t−τ)

L

(τ)

dτ



we obtain

u(t)

≤ K



γt

u(0)



γ(t−τ)

L

u(τ)

dτ



Uniqueness readily follows from this estimate. 

Finally, for Friedrichs-symmetrizable systems with Lipschitz coeﬃcients, H

well-posedness also holds true, as stated in the following result, which will be

used in the Initial Boundary Value Problem theory.

Theorem 2.9 Assume v ∈ W

1,∞

× (0,T)) and the operator

∂



(w) ∂

is Friedrichs symmetrizable for w in a domain containing the range of v.(As

usual, A

aresupposedtobeC

∞

functions of w, and so the Friedrichs sym-

metrizer.) Then for all g ∈ H

), the Cauchy problem

u =0,u(0) = g

has a unique solution u ∈ C ([0,T]; H

)) ∩ C

([0,T]; L

)).

Proof Unsurprisingly, the proof relies on a regularization of the coeﬃcients

andontheH

estimate in (2.1.13). Consider a molliﬁer ρ

and v

= ρ

∗ v.

Then v

is bounded independently of ε in W

1,∞

× (0,T)) and goes to v in

C ([0,T]; L

∞

)). By Theorem 2.6, the Cauchy problem

=0,u

(0) = ρ

∗ g

70 Linear Cauchy problem with variable coeﬃcients

admits a unique solution u

∈ C

∞

([0,T]; H

∞

)). Applying (2.1.13) to u

see (u

) is uniformly bounded in C ([0,T]; H

)). Furthermore, (u

)isaCauchy

sequence in C ([0,T]; L

)).Indeed,bytheL

energy estimate (2.1.12), we have

u

(t) − u



(t)

))

≤ K



γt

u

(0) − u



(0)



γ(t−τ)

L

− u



)(τ)

dτ



and

L

− u



)(τ)

≤ ( u



C ([0,T ];H

))

+ u





C ([0,T ];H

))

)

× max

A

) − A



)

C ([0,T ];L

∞

))

Therefore, u

converges to some u ∈ C ([0,T]; L

)). Furthermore, for all t ∈

[0,T], u

(t) belongs to H

) by weak compactness of bounded balls in H

and by L

–H

interpolation, u

converges to u in C ([0,T]; H

)) for all s ∈

[0, 1). It is not obvious that u belongs to C ([0,T]; H

)) though. If we can

prove that u is continuous at t = 0 in the space H

), then for the same

reason, by translating time it will also be right continuous at any t

∈ [0,T], as

well as left continuous by reversing the time. Now by an ε/3 argument together

with the continuity of u

at t = 0, the convergence of ρ

∗ g to g in H

and the

convergence of u

to u in C ([0,T]; H

)), we already see that

lim

t0

u(t) − g

)

for all s ∈ [0, 1), and we want to prove that

lim

t0

u(t) − g

)

=0.

We can ﬁrst show that u(t) converges to g as t goes to 0+ in H

), the Sobolev

space H

) equipped with the weak topology. Indeed, for all φ in H

−1

and

ψ ∈ H

−s

(for s<1), we have

|φ, u(t) − g 

−1

|≤u(t) − g

φ − ψ

−1

+ ψ

−s

u(t) − g

in which φ − ψ

−1

can be made arbitrarily small, u(t) − g

is bounded

independently of t and u(t) − g

is already known to go to zero with t.Soby

a standard result on weak topology, the strong convergence of u(t)tog in H

will be proved if we can show that

lim sup

t0

u(t)

≤g

And this inequality is a (tricky) consequence of (2.1.13) applied to u

, or more

precisely of the reﬁned version

u

(t)

1,v

(t)

≤ e

γt

u

(0)

1,v

, (2.1.18)

Well-posedness in Sobolev spaces 71

where we have deﬁned a modiﬁed, equivalent norm on H

u

1,v

= 



(v) u







(v) ∂

u

with S

a Friedrichs symmetrizer of the operator. We postpone the proof of

(2.1.18) for a moment, and complete the proof of Theorem 2.9. Observe that

(t)) also converges to u(t) uniformly on [0,T]inH

. Indeed, for all φ in H

−1

and ψ ∈ H

−s

with s ∈ (0, 1), we have

sup

t∈[0,T ]

|φ, (u

− u)(t) 

−1

|≤u

− u

C ([0,T ];H

))

φ − ψ

−1

+ ψ

−s

u

− u

C ([0,T ];H

))

where the ﬁrst term in the right-hand side can be made arbitrarily small, and

the second term is already known to tend to 0. Therefore, using the convergence

of v

in C ([0,T]; L

∞

)), we get by passing to the limit in (2.1.18),

sup

τ∈[0,t]

u(t)

1,v(t)

≤ lim sup

ε0

sup

τ∈[0,t]

u

(t)

1,v

≤ e

γt

g

1,v(0)

hence

lim sup

t0

u(t)

1,v(t)

≤g

1,v(0)

This shows that u(t)doesgotog strongly in H

when t goes to zero.

In conclusion, up to manipulating the time as explained above, this implies

u belongs to C ([0,T]; H

)), and by passing to the limit in L

0 in the sense of distributions, L

u = 0, which implies u is also in

([0,T]; L

)). 

Proof of the inequality in (2.1.18) We omit the subscript ε. Revisiting the

proof of (2.1.13) (in the case L

u = 0) and diﬀerentiating the equation

∂

u +



(v) ∂

u =0

before multiplying by S

(v), we get

∂

u +



(v) ∂

∂

u =



[∂

(v)∂

] u,

where

[∂

(v)∂

] u(t)

)

≤A

(v(t))

1,∞

)

u(t)

)

72 Linear Cauchy problem with variable coeﬃcients

Now we compute

u(t)

1,v(t)

= R

u, u +



R

∂

u, ∂

u

+2Re



α,β

S

(v)∂

u, [∂

(v)∂

] u ,

where

:= ∂

(v)+



∂

(v) A

(v)),

and infer by the Cauchy–Schwarz inequality that

u(t)

1,v(t)

≤ γ u(t)

1,v(t)

with

γ :=

S

(v)

−1



∞

×[0,T ])

(R



∞

×[0,T ])



A

(v(t))

1,∞

)



2.2 Local uniqueness and ﬁnite-speed propagation

Most of the deﬁnitions introduced in Chapter 1 extend to the variable-coeﬃcient

systems by ‘freezing’ the coeﬃcients. We already used the notions of Friedrichs-

symmetrizable systems (Deﬁnition 2.1) and constantly hyperbolic systems (in

Theorem 2.3) as a generalization of Deﬁnition 1.2. In view of Deﬁnition 1.5, we

can also associate to system (2.0.1) a characteristic cone

char(x, t):={(ξ,λ) ∈ R

× R ;det(A(x, t, ξ)+λI

)=0}

at each point (x, t) ∈ R

× R

, and the corresponding forward cone Γ(x, t), which

is the connected component of (0, 1) in (R

× R) \ char(x, t). Additionally, for a

symmetrizable system with symbolic symmetrizer S(x, t, ξ), we can also deﬁne

Υ(x, t)={(ξ,τ) ∈ R

× R ; S(x, t, ξ)(τI

+ A(x, t, ξ)) > 0 }, (2.2.19)

where positivity is to be understood in the sense of Hermitian matrices. Observe

that the set Υ(x, t) is still an open cone due to the homogeneity degree 0 of S in

ξ. If the operator L is constantly hyperbolic, then Γ(x, t) is the set of (ξ,λ)such

that all the roots τ of the equation

det ( A(x, t, ξ)+(λ + τ)I

)=0

are strictly negative, that is,

Γ(x, t)={(ξ,λ) ; Sp( λI

+ A(x, t, ξ)) ⊂ (0, +∞) }.

Local uniqueness and ﬁnite-speed propagation 73

We thus have, for a system both Friedrichs symmetrizable and constantly hyper-

bolic,

Γ(x, t)={(ξ,λ); S(x, t, ξ)(λI

+ A(x, t, ξ)) > 0 } =Υ(x, t)

for any symmetrizer S(x, t, ξ).

Deﬁnition 2.5 Let H be a smooth hypersurface in R

× R

. Denoting by n the

normal vector to H, we say that H is

i) characteristic at point (x, t) if n(x, t) ∈ char(x, t),

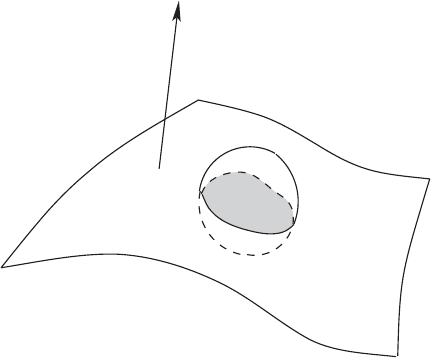
ii) space-like at point (x, t) if n(x, t) ∈−Γ(x, t) ∪ Γ(x, t).

If i), or respectively ii), holds for all (x, t), H is simply said to be characteristic,

or space-like, respectively.

By deﬁnition of Γ, a space-like surface is, of course, not characteristic. And

the most natural example of a space-like surface is {t =0}! The interest of

space-like surfaces is that they are associated with local uniqueness results.

Theorem 2.10

i) Assuming that the system (2.0.1) is constantly hyperbolic and f ≡ 0,letH

be a space-like hypersurface at (x

). Then there exists a neighbourhood

N of (x

) such that, if u is a C

solution of (2.0.1) in N and u|

H∩N

≡

0 then u|

≡ 0. (The reader may refer to Figure 2.1.)

ii) Assuming that the system (2.0.1) is Friedrichs symmetrizable and f ≡ 0,

let L be a lens made of two space-like surfaces, H and K , sharing the

)

Figure 2.1: Illustration of local uniqueness for constantly hyperbolic systems

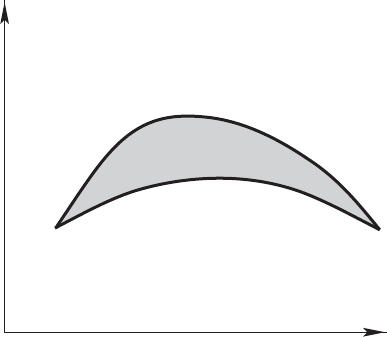
74 Linear Cauchy problem with variable coeﬃcients

Figure 2.2: Illustration of local uniqueness for Friedrichs-symmetrizable systems

same boundary. If u is a C

solution of (2.0.1) in L and if u ≡ 0 on H,

for instance, then u ≡ 0 also on K . (The reader may refer to Figure 2.2.)

Proof We begin with ii), the proof of which is more elementary. It is analogous

to the localized computations performed in Sections 1.3.1 and 1.3.3. We denote

by S

a Friedrichs symmetrizer of (2.0.1). Multiplying (2.0.1) by u

∗

we get



∂

u, u)+





∂

u, u)=



(Ru, u),

where R := ∂



∂

)+S

B + B

(like in Proposition 2.2). Inte-

grating the left-hand side yields the equality



u, u)+





u, u)



u, u)+





u, u)+



(Ru, u) ,

where n

denotes the t-component and n

the x

-component of n.Sincen

belongs to −Υ ∪ Υ, the matrix S

( n



) is deﬁnite, with the same

sign at all points (x, t)of∂L by continuity. Assume, for instance, that it is

positive. Then there exists γ>0 such that

( n



) ≥ γI