Bear H.S. Understanding Calculus

Подождите немного. Документ загружается.

Exact Differentials

SupposeF(x, y) is defined in someregionR, and

dF(x,y) =P(x,y) dx + Q(x,y) dy;

(43.1)

that is, assume

thatP(x,y) =Fx(x,y) and Q(x,y) =Fy(x,y). In this casewe saythatPdx +Qdy

is an exact differential in the regionR. Line integralsare particularlysimple if the differen-

tial is exact.

To evaluate

Pdx + Qdy where the differential is exact as in (43.1), we let C be a

curve inR from

(a, b) to (c, d), with Cparameterized by

x =x(t), Y =y(t), a

=::;

(43.2)

Thus (x(a), y(a» = (a, b) and

(x(f3),

y(f3»

= (c, d), and the integral is evaluated as

follows:

Pdx + Qdy=

JI3[F

(x(t),y(t»x'(t)

+ F (x(t),y(t»y'(t)] dt. (43.3)

C a x y

If we let G(t) =F(x(t), y(t», then the integrandon the right in (43.3)is just G'(t), so

1Pdx + Qdy = rG'(t) dt

C a

G(f3)

- G(a)

F(x(f3),

y(f3»

- F(x(a), y(a»

=F(c,

d)-F(a,

b). (43.4)

In otherwords,if Pdx + Qdy= dF, then for any curve C from (a, b) to (c, d),

Lp(X,

y) dx + Q(x,

dy =F(c, d) - F(a, b).

(43.5)

267

268

Understanding Calculus

Wecan also write (43.5)as

(C' d)

. dF =F(c, d) - F(a, b)

(a, b)

(43.6)

(43.7)

to emphasizethe similaritywith one-variable integration. For an exact differential, the line

integralis independent of path, and dependsonly on the end points of the curve.

EXAMPLE

43.1

Giventhat 2xy dx +(x

3y2)

dy is exact,find a functionF(x, y) such that dF(x,y) is the differential,

anduse

F(x,y) to calculate fc2xy dx + (x

3y2)

dy whereC is the arc of the circle(x - 2)2+ (y -

1)2

= 1

from(2, 2) to (3, 1).

Solution

SinceFx(x,y) = 2xy,F(x,y) is the x-antiderivative of2xy:

F(x,y)

= f

2xydx=x

2y+CP(Y).

Herey is consideredconstant, and the arbitraryfunction

CP(Y)

is a "constant of integration". Now use

Fy(x,

y) = Q(x,y):

Fix,y)

[xly +

CP(Y)]

= xl +

cp'(Y);

Q(x,y)

3y2.

Hence

cp'(y)

3y2,

CP(Y)

= y.

Finally,

F(x,y)

+ y.

(43.8)

(43.9)

Now to calculatethe line integral,we forgetabout the curve C, and just evaluate F(x, y) at the

endpoints:

l2xy

dx+(xl +

3y2)

dy = F(3, 1) - F(2, 2)

= 3

•

1 + 1- (2

•

2 + 2

)

10-16

=-6.

Nowthe questionarises:how do we knowwhethera differential Pdx + Qdy is exact or

not? There is an obviousnecessary condition. If P(x, y)

Fx(x,

y) and Q(x,y) =

Fy(x,

y), then

we must have

P=F

=Q.

y xy

If Pdx

+ Qdy is exact, then Q

= P

It turns out that the condition Q

= P

is also sufficient

for Pdx

+Qdy to be exact,at least with a little quibblingaboutthe geometryof the region.

If Q

= P

in the whole plane, then the integral of Pdx + Qdy will be independent of

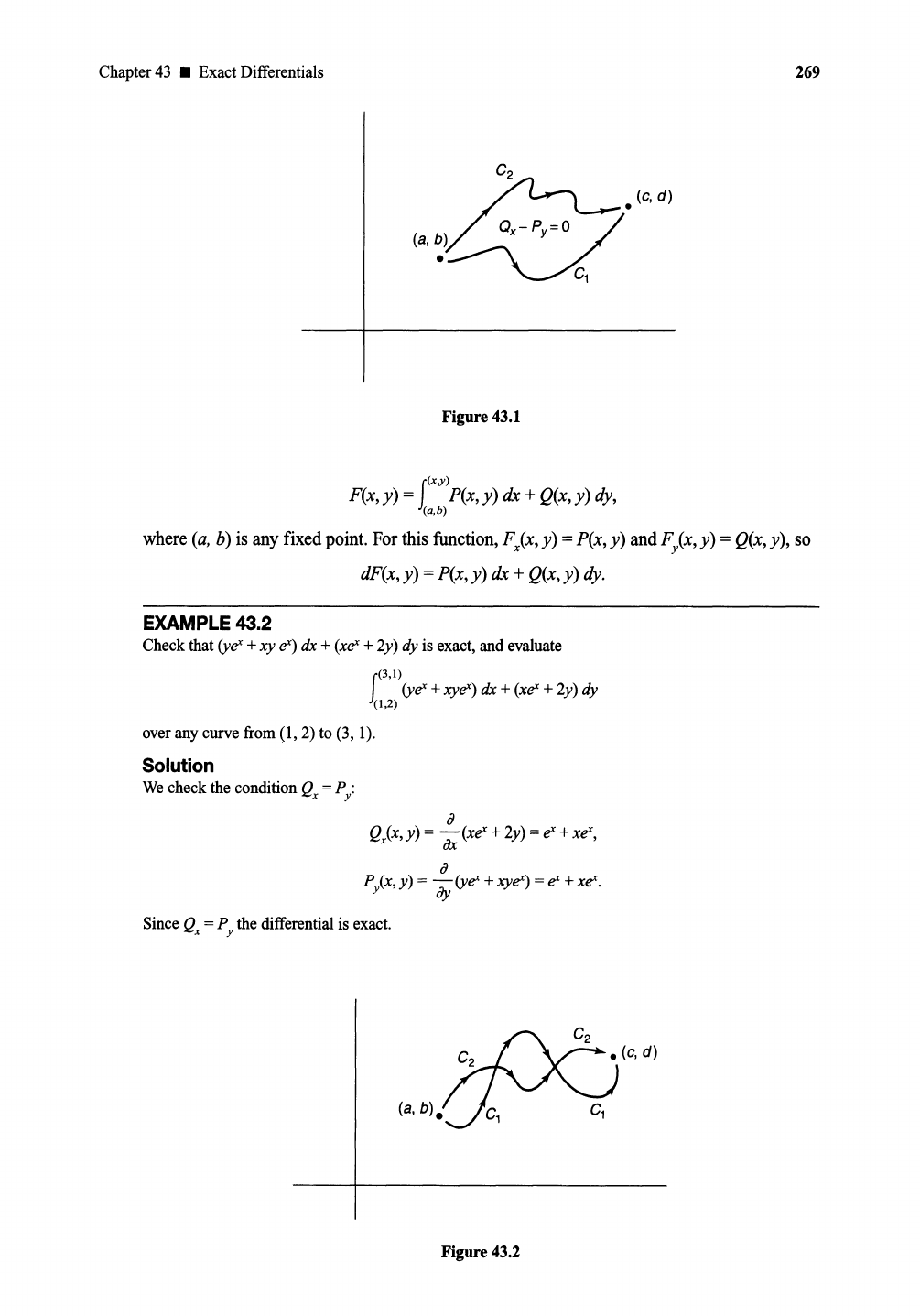
path. To see this, let C

and C

be two curves from (a, b) to (c, d), then C

is a closed

curve (Figure43.1), and since

0 inside C

the line integralaroundthe bound-

ary curve is zero by Green'sTheorem. That is,

I Pdx +Qdy=I Pdx + Qdy

for any two curves with the same end points. If C

and C

intersect each other on the way

from

(a, b) to (c, d), then we apply Green's Theorem to each loop (Figure43.2) and get the

sameresult.

If Q

so that the integralof Pdx + Qdy is independentof path, then we can define

a functionF(x,

y) by

Chapter43 • ExactDifferentials

Figure 43.1

• (c, d)

269

(x,y)

F(x, y) = P(x, y) dx +Q(x, y) dy,

(a,b)

where

(a, b) is anyfixedpoint.Forthis

function,

Fx(x,y) =P(x, y) andFy(x,y) =Q(x, y), so

dF(x,y) =

P(x,y)

dx+

Q(x,y)

dy.

EXAMPLE43.2

Checkthat

(ye

+xy

dx +

(xe

+2y) dy is exact,and evaluate

(3,1)

(ye

xye)

dx +

(xe

+2y) dy

(1,2)

overany curve from (1, 2) to (3, 1).

Solution

Wecheckthe conditionQ

= P

Qx(x,

y) =

(xe

+2y) =e +xe',

Py(x, y) =

(ye

xye)

= e +xe'.

SinceQ

= P

the differential is exact.

Figure 43.2

270

Understanding Calculus

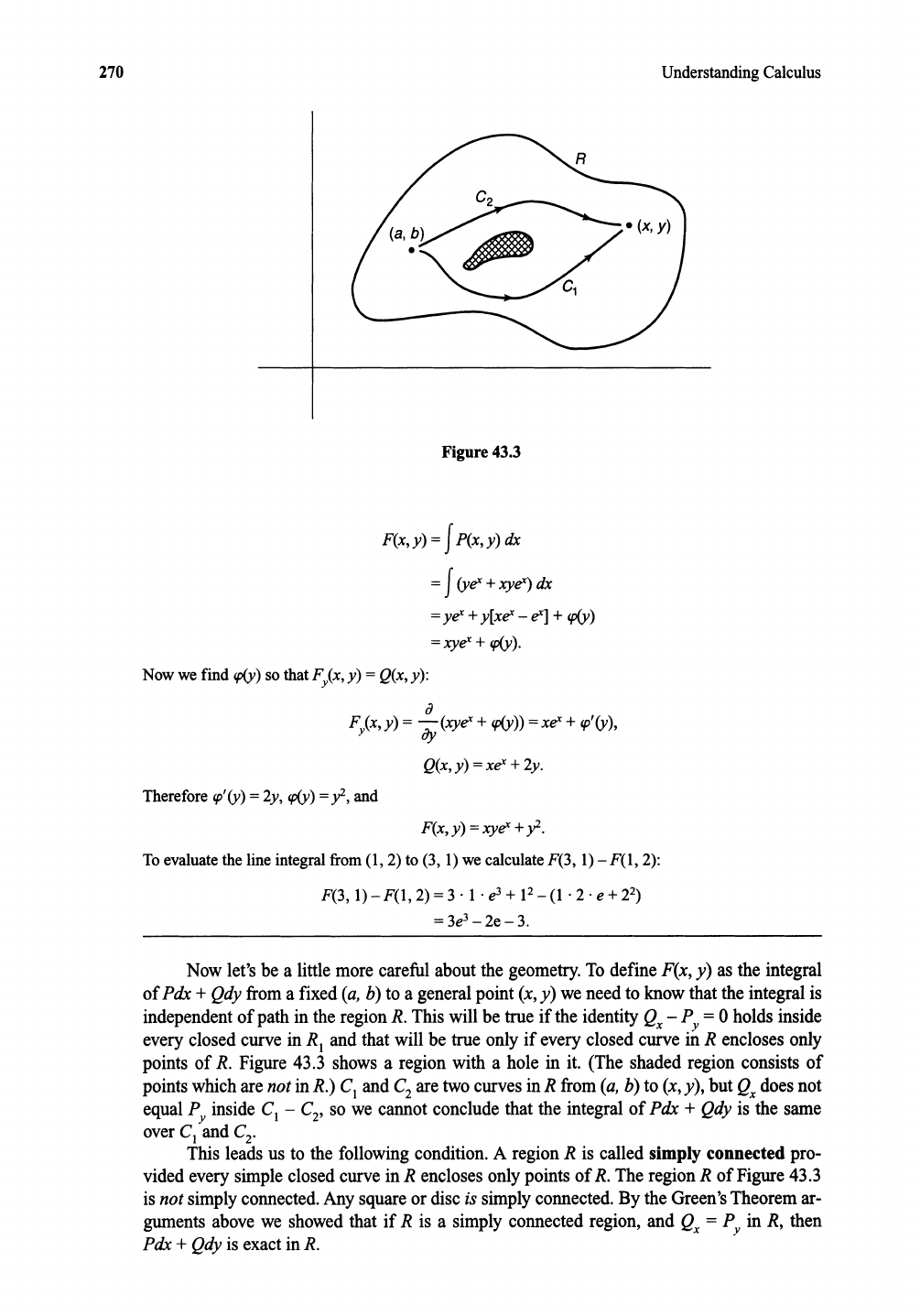
Figure

43.3

F(x,y) = JP(x,y) dx

= J

(yex

xyex)

=ye' +

y[xeX

eX]

CP(Y)

xyeX

CP(Y).

Now we find

CP(Y)

so that Fy(x,y) = Q(x,y):

F (x,y) =

-(xyeX

CP(Y))

<xe'

cp'(y),

Q(x,y) =

xe'

+ 2y.

Therefore

cp'

(y) = 2y,

CP(Y)

y2,

and

F(x,y) =xyeX+

y2.

To evaluate the line integral from (1, 2) to (3, 1) we calculate F(3, 1) - F(I, 2):

F(3,

-F(I,

2) = 3

·1

. e

+ 1

·2·

e + 2

)

=3e

3-2e-3.

Now let's be a little more careful about the geometry. To define F(x,y) as the integral

Pdx + Qdy from a fixed

(a,

b) to a generalpoint (x, y) we need to know that the integral is

independent of path in the region

R. This will be true if the identityQ

P = 0 holds inside

every closed curve in R, and that will be true only

every closed curve

J;.

R encloses only

points of

R. Figure 43.3 shows a region with a hole in it. (The shaded region consists of

pointswhichare

not in R.) C

and C

are two curves inR from

(a,

b) to (x,y), but Q

doesnot

equal

inside C

so we cannot conclude that the integral of Pdx + Qdy is the same

over

and C

•

This leads us to the following condition. A region R is called simply connected pro-

vided every simpleclosed curve in

R enclosesonly points of R. The region R of Figure43.3

not simplyconnected. Any square or disc is simplyconnected. By the Green'sTheoremar-

guments above we

showed

that if R is a simply connected region, and Q

= P

in R, then

Pdx + Qdy is exact in R.

Chapter43 • ExactDifferentials

271

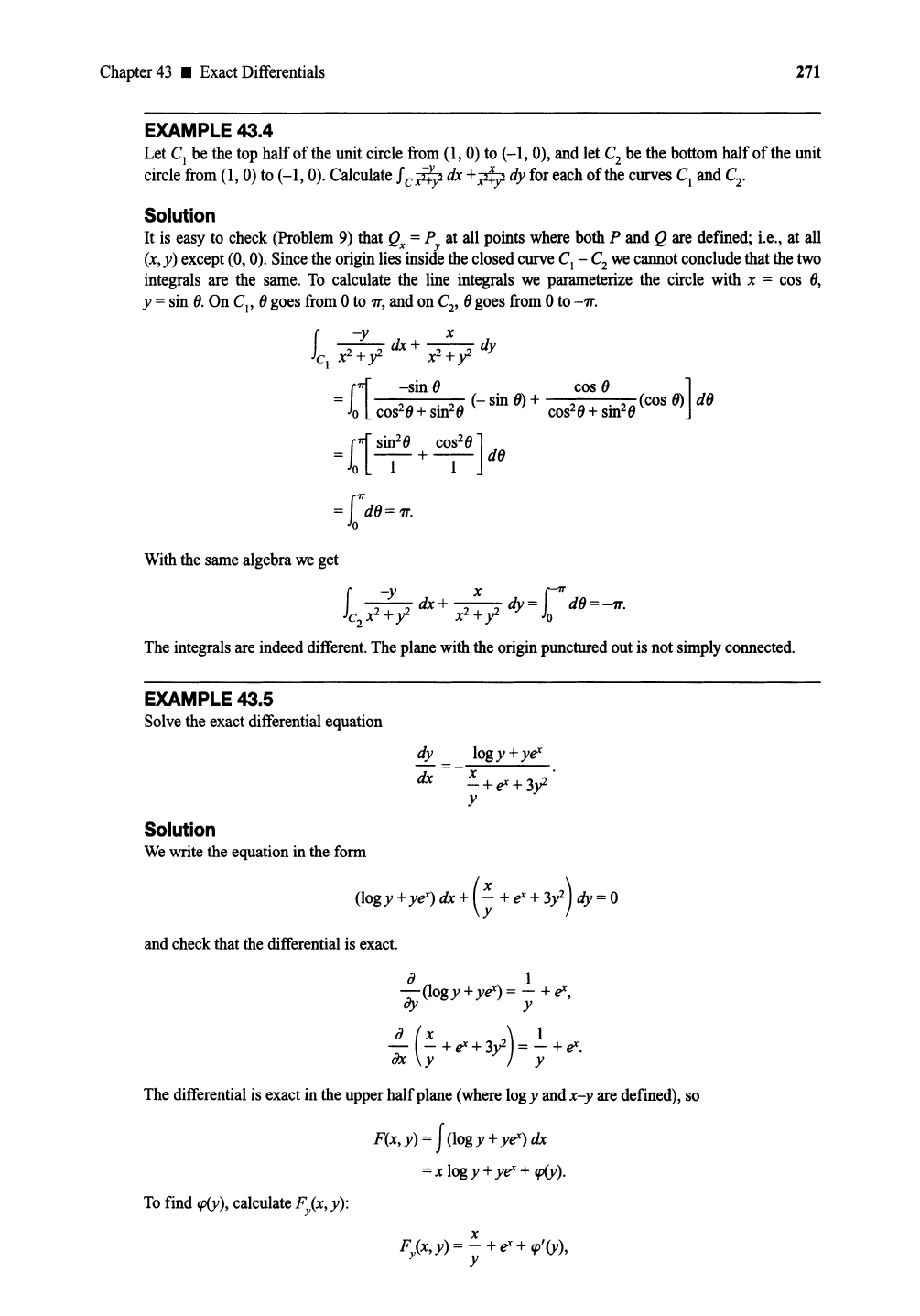
EXAMPLE

43.4

Let C

be the top half of the unit circle from (1, 0) to (-1, 0), and let C

be the bottomhalf of the unit

circlefrom(1,0) to (-1, 0). Calculate

feR

dx+~

dyforeach of the curves C

and C

•

Solution

It is easy to check (Problem 9) that Q

= P

at all points where both P and Q are defined; i.e., at all

(r, y) except(0, 0). Sincethe originlies insidethe closedcurveC

wecannotconcludethatthe two

integrals are the same. To calculate the line integrals we parameterize the circle with

x = cos 6,

y = sin 6.On C

0 goes from 0 to 11', and on C

, 0 goes from0 to

-11'.

-y

2"":2

dx +

2"":2

+Y x +r

-sin 6 cos 6 ]

20·

sin 6)+ 20 . 2 a (cos 6)

o cos + sm cos + sm u

sin

cos

]

--+--

o 1 1

Withthe same algebrawe get

-y

i-71'

2"":2

dx +

2"":2

dy =

-11'.

X +Y x +r 0

The integralsare indeeddifferent. Theplane withthe originpuncturedout is not simplyconnected.

EXAMPLE

43.5

Solvethe exactdifferential equation

Solution

Wewritethe equationin the form

dy =

logy +

yeX

x .

eX+

3y2

(log y +yex)dx+

+ ex+

3y2

) dy = 0

and checkthat the differential is exact.

a 1

(logy +

yeX)

= Y+ e',

) 1

- - +eX+3y2 = - +

eX.

ax y y

The differential is exactin the upperhalf plane (wherelogy and x-yare defined),so

F(x,y) = f(logy +yex)dx

= x logy +ye' +

CP(Y).

Tofind

CP(Y),

calculate

Fy(x,

y):

F (x,y) = - +

lp'(Y),

y y

272

Understanding Calculus

Q(x,y)

= -

+e+3y2.

Therefore

q/(y)

3y,

lp(y)

= y3, and

F(x,y)

logy +

+I.

The differential equationis dF(x, y) =0, so the solutionis F(x, y) =c; i.e.,

x logy + ye' + 1= c.

PROBLEMS

Checkthat the following differentials are exact in the givenregionR. Evaluatef

cPdx

+Qdy for

the given curve C. Then find

F so dF = Pdx + Qdy, and check your answer by evaluating

F(c, d) - F(a, b) where(a, b) and (c, d) are the end-points of C.

43.1

f c 2xy dx + (x

y) dy; C is the straightline from (0, 0) to (4, 3).R is the plane.

43.2

fc(x +y)dx + (x +e

)

dy; C is the verticalline from(2,0) to (2, 5). R is the plane.

43.3

XZ~?

dx +

dy; C is the arc of the circlex

= 1 from

("4,

(~,

~).

R is

the right half-plane

x >

Showthe differential equationsare exact,and solvethem.

43.4 (x

+cosy)

+2xy =

dy e

43.5

= SID y-xeY.

43.6 (3x

2xI)

dx + (2x

y - 3x

)dy =

43.7

sinydx+(xcosy+

l)dy=O

43.8

-x+

43.9 Showthat Q

= P

exceptat (0, 0) if P(x, y) = xi.Jy, Q(x,y) =

X2~YZ'

CHAPTER

1.1 (a, b) and (b, a) are symmetric about the line y = x.

1.2 m

=-~

1.3 m = 1

1.4 m = 2

1.5 m =

-1

1.6 m

=-1

1.7 m

=-~

1 3

. Y -

-2

+ 2

1.9 y =

-~x

1.10 y = 2x + 7

1.11 y =

-4x

- 1

1.12 y =

-3x

1.13 y =

-2x

1.14

y=7x-5

1.15 (iii) k =

1.16 x - 2y + 2 +

~(x

+ y - 4) = 0, or x - y = 0

1.17

y=2x-2

1.18

-3x

+ y + 8 = 0

1.19 8.1

1.20 y - 4 = .466(x - 3)

CHAPTER

2.1 Downward parabola with axis x = 0, vertex (0, 0)

2.2 Upward parabola with axis

x = 0, vertex (0, 0)

Answers

273

274 Answers

2.3 Upward parabola with axis x = 0, vertex (0, 0)

2.4 Upward parabola with axis

x =

-1,

vertex (

-1,

2.5 Upward parabola with axis

x = 0, vertex (0, 1)

2.6 Downward parabola with axis

x = 0, vertex (0, 1)

2.7 Upward parabola with axis

x = 1, vertex (1, 1)

2.8 Downward parabola with axis

x = 1, vertex (1, 1)

2.9 Circle with center (0, 0), radius 2

2.10 Circle with center

(1,0),

radius 1

2.11 Circle with center (0, 2), radius 3

2.12 Ellipse through

(±3,

0) and (0,

±2)

2.13 Ellipse through

(±1,

0) and (0,

±2)

2.14 Ellipse through

(±3,

0) and (0,

±2)

2.15 Hyperbola through

(±1,

0), asymptotes y = ±x

2.16 Hyperbola through (0,

±1),

asymptotes y = ±x

2.17 Hyperbola through

(J2,

J2)

and

(-J2,

-J2),

asymptotes x = 0 and y = 0

2.18 Hyperbola through (2, 1) and (0,

-1),

asymptotes y = 0 and x = 1

2.19 x

+ y2 - 2x + 4y = 0

2.20 y =

1(x

+ 1)2

CHAPTER 3

3.1 4

3.2 20

3.3 27

3.4 6

3.5 !

3.6 !

3.7

-b

3.8

-2

3.9 n

3.10

y-2=4(x-l)

3.11

y-l=-4(x-2)

3.12

y-4=~(x-4)

3.13 y - 1 =

-~(x

- 1)

3.14 s'(2) = 36 ft/sec; s' (0) = 100 ft/sec; max height = 156.25 ft

3.15

s(2)

= 104;

s'(2)

= 84 ftlsec

3.16

= 4Jrt

3.17 W'(100) =

-to

lb/min

CHAPTER 4

4.1

= 20x

+ 2x + 1

4.2

-6x

Answers

4.3

-12x-

+x-

+6x

4.4

-8x-

9x-

+ 5 + 3x

4.5

= 3x

3x-

4.6

= 10x

10x-

4.7

=6x

2+6x+2

4.8

= 4x

4.9

= lOx + 2 +

3x-

dy -

-4x

•

- (x

2_1)2

4 11

dy _

-3x

•

(2+x

3)2

4 12 dy _ 2x

3+3x

•

(x+1)2

4 13

- (2x

4+x

2+1)

- (2x

2+1)2

414

-4

(2x-1)2

415

- S 1

•

2,Ji

- XI

4 16

- -.L

· dx 2

'\I

,Ji

4.17

~dd

=-~

2x~

4.20

= (x

+ 2)(x - 2) + (x + 1)(2x)(x - 2) + (x + 1)(x

+ 2)

4.21

-3x-

4(x

+ 3)(x

- 4) +

3(x2

4) +

3(x

+ 3)(2x)

CHAPTER

5.1

= 12(1 + 3x)3

5.2

= 5(x + x

2)4(1

+ 2x)

5.3

-2(1

+ 2x)-2

5.4

-10(x

7)-11(3x

+7x

)

5.5

-3(2

+ 3x)-2

5.6

-2x(1

+ x

2)-2

5.7

= x(1 + x

)- 4

5 8 dy _

3(,Ji+

1)2

•

2,Ji

5 9

-6(2x+1)2(x

2+x-1)

. dx - (1

5 10

- 5x

2(l-x)

• dx -

2,Ji(x+

1)6

5.11

_~x2(x3

2)-~

5.12

-2(.JI+X

+xr

(2k + 1)

5.13

-6(2x

+ 1)-4

5.14

-5(x

+ 1)-6

1_1

•

-2'X

5 16

1_1

•

-2'X

5.17

-x(x

9)-~

275

276

dz -

(l+JX+ij

· dx -

JX+T

5.19

= 17 cm

2/sec

5.20

ft/min;

= 1 ft

2/min

5 21

dT 5 d /

4J3

egrees sec

5 22

5·

• dt = 18Jr In. sec

5 23

[ft. -

_3_

· dt - 2

.Jr2·5

- 10

Jr2

5.24

5 25 dy

-x

· dx = Ja

- x

5.26 t = 2

5.27 g'(x) = (2x +

1)/(x

+ x)

CHAPTER

6.3

-2

sin 2x

6.4

= 3 cos(3x + 1)

6.5

-2cosx

sinx

6.6

= cos

X - sin

6.7

= 16 sin 8x cos 8x

6.8

-15

cos

2(5x

+ 1) sin(5x + 1)

-sinx

. dx -

2Jl+cosx

6.10

2(sinx

cosx)(cosx

sinx)

6.11

= x sec x tan x + sec x

6.12

4sec

24x

6.13

= sec

+secxtan

6.14

= 2 sec

x tan x

6.15

= 2 tan x sec

6.16

= 3 sec' x tan x

617

(l+cosx)cosx+sin

· dx - (l+cosx)2 - l-t-cosr

6.18

2(tanx

+ 1) sec

6.19

-3(2x

+ 1) sin 3x + 4x cos 3x

dy (5x cos

5x-sin

5x)

· dx

= x

6.21

dy _ 2x

sinx-(x

2+l)

cosx

dx -

sin

6 22 dy cosx(x

cosx+sinx)+x

sin

• dx

= cos

x+sinx

cosx

= cos

25 tan(x + ) = sin(x+y) =

sinxcosy+c~sxs~ny

· Y cos(x+y)

cosxcosy-smxsmy

Divide top and bottom by cos x cos Y.

6.26 600 ft/min or 10ft/sec

Answers