ASM Metals HandBook Vol. 8 - Mechanical Testing and Evaluation

Подождите немного. Документ загружается.

All peel tests have the common characteristic that failure propagates from an initially debonded area. They also

generally involve large displacements/deformations. For these and other reasons, linear elastic stress analysis is

often not well suited to peel tests. The stresses and strains in the peel configuration are complex and seldom

well understood. Test results are generally not given in terms of stress but rather as force per unit length

required to peel the specimen. It is, therefore, generally difficult to compare the results from a peel test with

those from other testing methods.

Because of the large deformations involved in peel tests, the analysis of such geometries is very difficult except

under certain simplifying assumptions (Ref 3, 4, 6, 9, and 10). Some very interesting and informative

observations can be made on the basis of simplifying assumptions and approximations. Indeed, considerable

useful work has been completed using peel tests. The informative work of Gardon (Ref 10) and Kaelble (Ref

11) is noteworthy. The polymer research group at The University of Akron, under the direction of Professor A.

Gent, has been particularly adroit in applying peel techniques and the concepts of fracture mechanics (see the

section “Adhesive Fracture Mechanics Tests” in this article) to obtain critical information and insight into the

behavior of adhesive joints (Ref 12, 13). The peel specimen is, in principle, a very versatile geometry for

obtaining adhesive fracture energy because various combinations of mode I and mode II loadings can be

applied by varying the peel angle (Ref 3). The stress analyses of Adams and Crocombe (Ref 14) have provided

additional insight into the peeling mechanisms. They examined the stress distributions in peel specimens using

elastic large-displacement, finite-element analysis techniques.

References cited in this section

3. G.P. Anderson, S.J. Bennett, and K.L. DeVries, Analysis and Testing of Adhesive Bonds, Academic

Press, 1977

4. A.J. Kinlock, Adhesion and Adhesives, Chapman and Hall, 1987

6. K.L. Mittal, Adhesive Joints, Plenum Press, 1984

7. Adhesives, Annual Book of ASTM Standards, Vol 15.06, ASTM (updated annually)

9. G.P. Anderson and K.L. DeVries, Predicting Strength of Adhesive Joints from Test Results, Int. J.

Fract., Vol 39, 1989, p191–200

10. J.L. Gardon, Peel Adhesion, I. Some Phenomenological Aspects of the Test, J. Appl. Polym. Sci., Vol 7,

1963, p 654

11. D.H. Kaelble, Theory and Analysis of Peel Adhesion: Mechanisms and Mechanics, Trans. Soc. Rheol.,

Vol 3, 1959, p 161

12. A.N. Gent and G.R. Hamed, Peel Mechanics, J. Adhes., Vol 7, 1975, p 91

13. A.N. Gent and G.R. Hamed, J. Appl. Polym. Sci., Vol 21, 1977, p 2817

14. R.D. Adams and A. Crocombe, J. Adhes., Vol 12, 1981, p 127

Testing of Adhesive Joints

K.L. DeVries and Paul Borgmeier, University of Utah

Lap Shear Tests

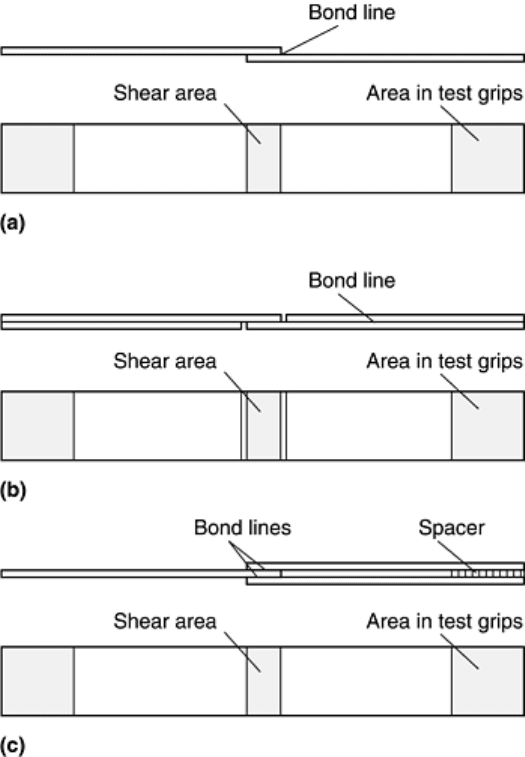
The most popular test geometry for testing adhesive joints is the lap shear specimen. Its appeal is probably

based on the fact that it closely duplicates the geometry used in many practical joints. These lap joints are

popular for several reasons:

• They facilitate use of larger contact areas than, for example, a butt joint.

• They are easier to make and align than butt joints.

• The adhesive is not exposed to “direct” tensile stresses. Direct tensile stresses are known to have

deleterious effects on adhesives.

Typical lap shear test specimens for which ASTM standards have been written are presented in Fig. 3 (Ref 7).

The specimens shown in this figure conform most closely to ASTM Standards D 1002, D 3163, D 3164, D

3165, and D 3528 for testing adhesives used to bond metals, plastics, and laminates. These represent only a

small sampling of the more than two dozen standards in the Annual Book of ASTM Standards, Volume 15.06,

that relate to shear testing. These other standards range from descriptions of block-type sample configurations

for testing lumber and wood bonding in shear by compression loading, through descriptions of devices to

simultaneously expose samples to lap shear stresses and extremes in temperature. Still others describe apparatus

for exposing lap joints to sustained loads (using springs) to measure long-term creep or time to failure.

Fig. 3 Typical lap shear geometries. (a) ASTM D 1002, D 3163, and D 3164. (b) ASTM D 3165. (c) ASTM

D 3528. Source: Ref 7

The results from lap shear tests are generally reported as the force at failure divided by the bonded area (overlap

area). Such values are listed in a number of reference books and manufacturers' literature for a wide variety of

adhesives. The reference book on types of adhesives (Ref 1) lists typical lap shear strength values for literally

thousands of commercial adhesives. Such tables of “shear strength” values are without doubt of considerable

utility for comparison and other purposes. However, their use also can lead to faulty expectations and

conceptions. Otherwise knowledgeable designers might logically assume from the tabulations that these

average stress values could, in a straightforward manner, be used to design an adhesive joint.

For example, the tabulated shear stress value for a given adhesive from an ASTM D 1002 test might be given as

3000 psi. It might be assumed that this adhesive is to be used to bond two 25 mm (1 in.) wide by 3 mm (0.12

in.) thick 7075-T6 aluminum pieces together to carry a tensile load of 3200 lb with a safety factor of two. First,

the designer must ascertain whether the aluminum pieces can carry such a load. Typically, 7075-T6 aluminum

has a yield strength slightly in excess of 65 ksi for an allowable stress of 32.5 ksi. The pieces in question would

have an allowable load of 4000 lb, which is more than the 3200 lb required in the design. The “straightforward”

method to design the joint would be to assume that the allowable shear strength for the adhesive used in the

joint would be 3000/2 = 1500 psi, suggesting that an overlap of 3200/1500 = 2.13 in. would be sufficient to

support the load. This is, in fact, the approach taught by a variety of otherwise very good texts on material

science and mechanical design. However, doubling the length of a lap joint almost never doubles its load-

carrying capacity, and the increased joint strength is usually much less than doubled. The length of overlap

recommended in ASTM D 1002 is 12.7 mm (0.50 in.). Typically, quadrupling the amount of overlap does not

increase the load at failure by anywhere near a factor of four. For reasons given in the next few paragraphs, it is

likely that it is not even the value of the maximum shear stress that determines the failure of the “lap shear

joint.” As this article reveals, joint failure is more likely determined by the value of secondary induced cleavage

stresses.

The stresses along the bond line of lap specimens are not constant. The bond stress distribution is highly

dependent on the thickness of the adherends and the adhesive as well as the length of overlap. As a

consequence, the load to initiate failure also varies markedly with both the adherend(s) and adhesive-bond

thicknesses. The failure load increases very nearly linearly with width of the overlap but increases in a very

nonlinear manner with length of the overlap. As the load is increased in a lap shear test, the debonding

generally initiates at or near one of the bond terminations. Elastic stress analysis generally indicates that the

stresses are singular at these termination points. Debond initiation in lap shear specimens can perhaps,

therefore, be best characterized in terms of fracture mechanics parameters, which are discussed in the section

“Adhesive Fracture Mechanics Tests” in this article. In addition, it has been demonstrated that for debonds after

initiation, crack propagation is dominated by crack- opening mode displacements (mode I). For this reason and

reasons given in the next couple of paragraphs, the word shear in the test titles and generally reported in test

results may, therefore, be a misnomer.

It has been known for many years that the shear stresses in the bond line of lap specimens are accompanied by

tensile stresses. Many analyses have been completed for lap shear geometries, almost all of which have clearly

demonstrated the presence of induced tensile stresses in so-called lap shear specimens under load. In 1938,

Volkersen (Ref 15) obtained expressions for the stresses in a lap shear joint by considering the differential

displacements of the adherends and neglecting bending. This study was followed in 1944 by the now classical

treatment of Goland and Reissner (Ref 16) who used standard beam theory and strength of materials concepts

to obtain expressions for the joint stresses. Plantema (Ref 17) combined the results of these two earlier

investigations to include shear effects in the system.

Because the stress state of the lap shear joint is so complex and does not lend itself to closed-form solutions, it

is only logical that as numerical methods became available, researchers would apply them to analyze adhesive

joints. Wooley and Carver (Ref 18), for example, used finite-element methods to calculate the joint stresses.

They compared their results with the results obtained by Goland and Reissner and reported very good

agreement. Adams and Peppiatt (Ref 19) used a two- dimensional finite-element code to analyze the stresses in

a standard lap shear joint and also reported good agreement with Goland and Reissner. These authors also

investigated the effect of a spew (triangular adhesive fillet) on the calculated stresses. A nonlinear finite-

element analysis of the single lap joint was completed by Cooper and Sawyer (Ref 20) in 1979.

Anderson and DeVries conducted a linear elastic stress analysis of a typical single lap joint (Ref 21) making use

of plane-strain finite- element computer programs using elements as small as 0.00025 cm (0.0001 in.). They

considered steel (modulus of elasticity, 207 GPa; Poisson's ratio, 0.30) adherends of various thicknesses bonded

with a 0.25 mm (0.01 in.) thick epoxy (modulus of elasticity, 2.76 GPa; Poisson's ratio, 0.34). The overlap

region was taken as 13 mm (0.5 in.) long. The results of these analyses are shown in Fig. 4. Note that both the

shear and tensile stresses are distributed very nonlinearly over the length of the bond region. Reference 21

reports stresses resulting from other adherend thicknesses. As the bond termini is approached, both shear and

normal stresses appear to become singular. Careful analysis in this region suggests that the local mode I stresses

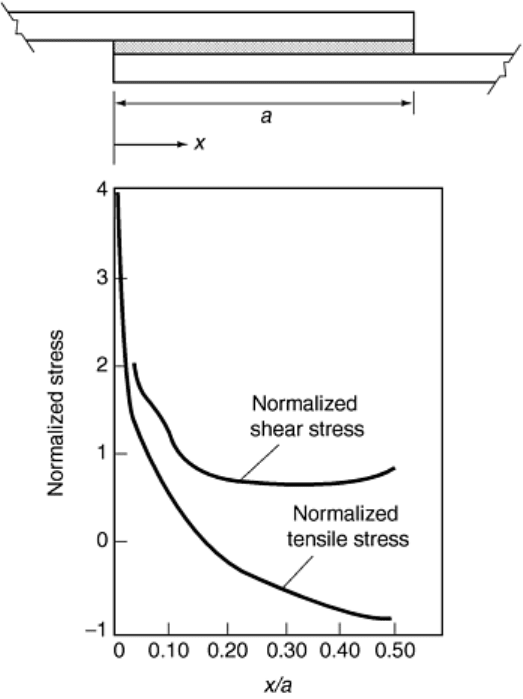
(tensile or crack opening) are significantly higher than mode II stresses (shear). Perhaps even more importantly,

the mode I energy release rate is greater than that for mode II. From these results, it might be concluded that lap

shear specimens fail by mode I crack growth. Therefore, the failure of lap shear specimens is usually governed

by tensile stress rather than shear stresses. This is true for double lap joints as well as single lap joints (Ref 22,

23).

Fig. 4 Bond line tensile and shear stresses in lap shear specimen (adherend thickness = 1.6 mm, or 0.06

in.)

As noted, the end(s) of the overlap on bond termini on lap shear specimens are points of stress concentration

and of large induced tensile stresses. While this closely simulates many practical situations, some have

suggested that for determination of intrinsic adhesive properties, it would be useful if these termini could be

eliminated. ASTM E 229 “Standard Test Method for Shear Strength and Shear Modulus of Structural

Adhesives” is a test designed specifically for this purpose. In this test, the adhesive is applied in the form of a

thin annulus ring bonded between two relatively rigid adherends in circular disc form. Torsion shear forces are

applied to the adhesive through this circular specimen, which produces a peripherally uniform stress

distribution. The maximum stress in the adhesive at failure is taken to represent the shear strength of the

adhesive. By measuring the angle of twist experienced by the adhesive and having knowledge of sample

geometry, it is possible to calculate the strain. A stress- strain curve can then be established from which the

adhesive's effective shear modulus can be determined.

References cited in this section

1. Adhesives, Edition 6, D.A.T.A. Digest International Plastics Selector, 1991

7. Adhesives, Annual Book of ASTM Standards, Vol 15.06, ASTM (updated annually)

15. O. Volkersen, Die Nietraftverteilung in Zugbeanspruchten Nietverblendugen mit Knastaten

Laschenquerschntlen, Luftfahrt forsch., Vol 15, 1938, p 41

16. M. Goland and E. Reissner, The Stresses in Cemented Joints, J. Appl. Mech., Vol 11, 1944, p 17

17. J.J. Plantema, “De Schuifspanning in eme Limjnaad,” Rep. M1181, Nat. Luchtvaart-laboratorium,

Amsterdam, 1949

18. G.R. Wooley and D.R. Carver, J. Aircr., Vol 8 (No. 19), 1971, p 817

19. R.D. Adams and N.A. Peppiatt, Stress Analysis of Adhesive-Bonded Lap Joints, J. Strain Anal., Vol 9

(No. 3), 1974, p 185

20. P.S. Cooper and J.W. Sawyer, “A Critical Examination of Stresses in an Elastic Single Lap Joint,”

NASA Tech. Rep. 1507, NASA Scientific and Technical Information Branch, 1979

21. G.P. Anderson and K.L. DeVries, Analysis of Standard Bond-Strength Tests, Treatise on Adhesion and

Adhesives, Vol 6, R.L. Patrick, K.L. DeVries, and G.P. Andersen, Ed., Marcel Dekker, 1989

22. J.K. Strozier, K.J. Ninow, K.L. DeVries, and G.P. Anderson, Adhes. Sci. Rev., Vol 1, 1987, p 121

23. G.P. Anderson, D.H. Brinton, K.J. Ninow, and K.L. DeVries, A Fracture Mechanics Approach to

Predicting Bond Strength, Advances in Adhesively Bonded Joints, Proceedings of a Conference at the

Winter Annual Meeting of ASME, 27 Nov-2 Dec 1988 (Chicago), S. Mall, K.M. Liechti, and J.K.

Vinson, Eds., ASME, 1988, p 98–101

Testing of Adhesive Joints

K.L. DeVries and Paul Borgmeier, University of Utah

Tensile Tests

Generally, the idea of mechanical failure produces a vision of an object being pulled apart by tensile force. As

noted previously, most practical adhesive joints are designed to avoid (or at least reduce) direct tensile forces

across the bond line. Examples of such joints are lap joints and scarf joints. It was also pointed out that for

many joints, where it appears that the primary loading is shear, failure might be initiated by the induced

secondary tensile stresses. There are, therefore, reasons why an adhesive's or adhesive joint's tensile strength

might be of interest. Accordingly, the third most common type of adhesive joint strength test is the tensile test.

ASTM has also formalized this type of test.

The geometries of several tensile tests for which there are specific ASTM test procedures are shown in Fig. 5

(Ref 7). Some of these test geometries seem relatively simple; however, it has been demonstrated that the

stresses along the bond line have a rather complex dependence on geometric factors and adhesive and adherent

properties (adhesive thickness and its variation across the bonded surface, modulus, Poisson's ratio, and so on)

(Ref 21).

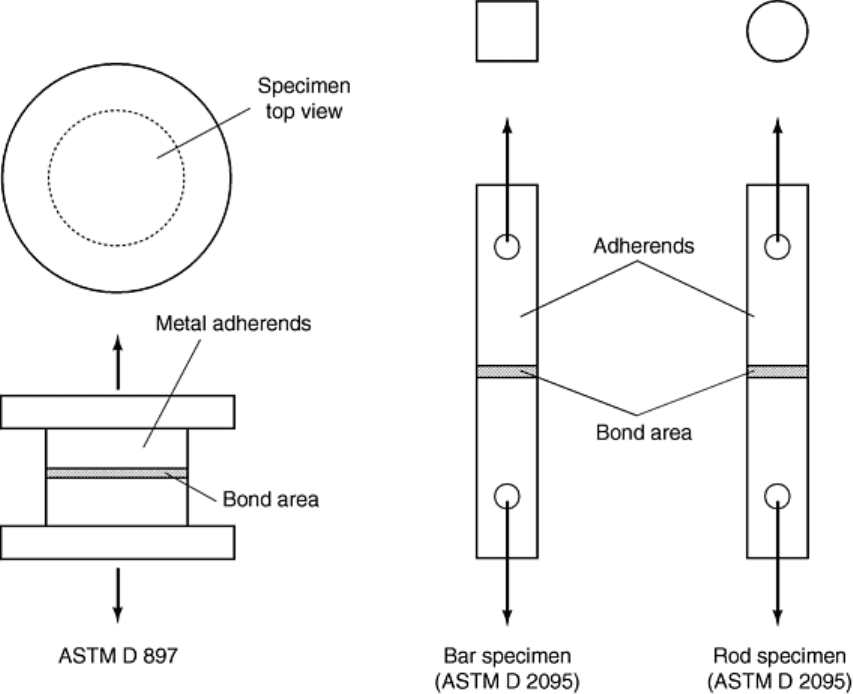
Fig. 5 Typical specimen geometries for testing the tensile strength of adhesive joints. Source: Ref 7

It is almost always difficult to load tensile adhesion specimens in an axisymmetric manner, even if the sample

itself is axisymmetric. Nonaxisymmetric loads have been shown to reduce the bond failure load capability and

to cause large scatter in the resulting failure data. Superficially, the geometry for standard tensile adhesion tests

is deceptively simple. The result of the tensile adhesion test, as normally reported by experimentalists, is simply

the failure load divided by the cross-sectional area of the adhesive (Ref 22). Such average stress at failure can

be very misleading. Because of the differences in mechanical properties of the adhesive and adherend, the

stresses may become singular at the bond edges when analyzed using linear elastic analysis (Ref 21, 23). Even

if the edge singularity is neglected, the stress field in the adhesive is very complex and nonuniform, with

maximum values differing markedly from the average value (Ref 21, 23).

Some sense of the complex nature of the stresses can be obtained by visualizing a butt joint of a low modulus

polymer (e.g., a rubber) between two steel cylinders. As these are pulled apart, the rubber elongates much more

readily than the steel. Poisson's effect will cause a tendency for the rubber to contract laterally. However, if it is

tightly bound to the metal, it is restrained from contracting, and shear stresses are induced at the bond line.

Reference 9 provides the results of a finite element analysis that demonstrates how these stresses vary across

the sample. As noted, for an elastic analysis, both the shear and tensile stresses are singular (tending to infinity)

at the outer periphery.

For the tensile specimen configurations considered to this point, the applied loading is intended to be

axisymmetric. There is another class of specimen in which the dominant stress is deliberately tensile but in

which the loading is obviously “off center.” At least four ASTM standards describe so-called cleavage

specimens and tests. These tests are a logical preface to the next section in this article, “Adhesive Fracture

Mechanics Tests”. The reader familiar with cohesive fracture mechanics will see a similarity between the test

specimen in ASTM D 1062 (Fig. 6) and the compact tensile specimen commonly used in fracture mechanics

testing. ASTM D 1062 specifies reporting the test results as force required, per unit width, to initiate failure in

the specimen, while in fracture mechanics, the results are given as G

with units of J/m

, which might be

interpreted as the energy required to create a unit surface. A knowledgeable and enterprising reader may want

to adapt the D 1062 specimen for obtaining fracture mechanics parameters. ASTM D 3807, “Standard Test

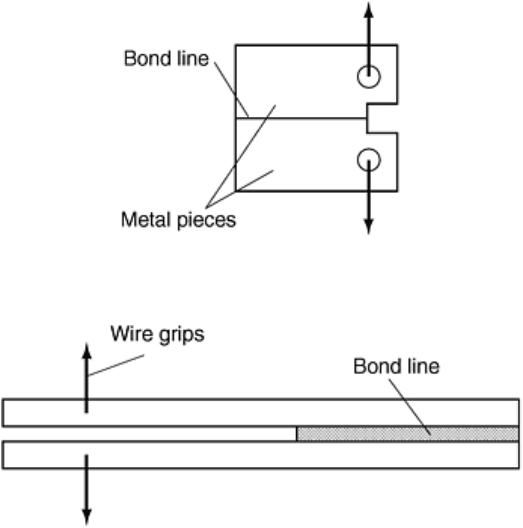
Method for Strength Properties of Adhesives in Cleavage Peel by Tension Loading,” uses a different geometry

to measure the cleavage strength. In this case, two 25.4 mm (1 in.) wide by 6.35 mm (0.25 in.) thick plastic

strips 177 mm (7 in.) long are bonded over a length of 76 mm (3 in.) on one end, leaving the other ends free and

separated by the thickness of the adhesive. Approximately 25 mm (1 in.) from the end of each of these free

segments, a “gripping wire” is attached as shown in Fig. 7. During testing, these wires are clamped in the jaws

of a universal testing machine and the sample pulled to failure. The results are reported as load per unit width

(kg/m or lb/in.). Again, it would be possible to analyze this sample in terms of fracture mechanics, but it is

unnecessary because, as the next section explains, this analysis is done in ASTM D 3433 for a very similar

beam geometry.

Fig. 6 Specimen for testing the cleavage strength of metal-to-metal adhesive bonds (ASTM D 1062)

Fig. 7 Specimen for testing cleavage peel (by tension loading) (ASTM D 3807)

ASTM D 5041 also makes use of a sample composed of two thin sheets bonded together over part of their

length. In this case, forcing a wedge (45° angle) between the unbonded portion of the sheets facilitates the

separation. The results are typically given as “failure initiation energy” or “failure propagation energy” (i.e.,

areas under the load deformation curve).

This latter test is similar to another test, formalized as ASTM D 3762, that has been found very useful for

studying time-environmental effects on adhesive bonds. This test is called by various names, but the authors

prefer the name “Boeing Wedge Test” (Ref 24, 25). The test has been used by personnel at this and other

aerospace companies to screen various adhesives, surface treatment, and so on for long-term loading at high

temperatures and humidities. For testing, two long, slender strips of candidate structural materials are first

treated with the prescribed surface treatment(s) and bonded over part of their length with a candidate adhesive

(Fig. 8). As in the test described in the previous paragraph, the free ends are forced apart by a wedge. The

amount of separation by the wedge (determined by wedge thickness and depth of insertion) determines the

value of the stresses in the adhesive. These stresses can, of course, be adjusted and the values calculated from

mechanics of material concepts. When the wedge is in place, the sample is placed in an environmental chamber.

At periodic time intervals, the length of the crack is measured, and a plot of crack length versus time is

constructed. The more satisfactory adhesives and/or surface treatments are those for which the crack is arrested

or grows very slowly. While the environmental chamber typically contains hot, humid air, there is no reason

why other environmental agents cannot be studied by the same method, including immersion in liquids.

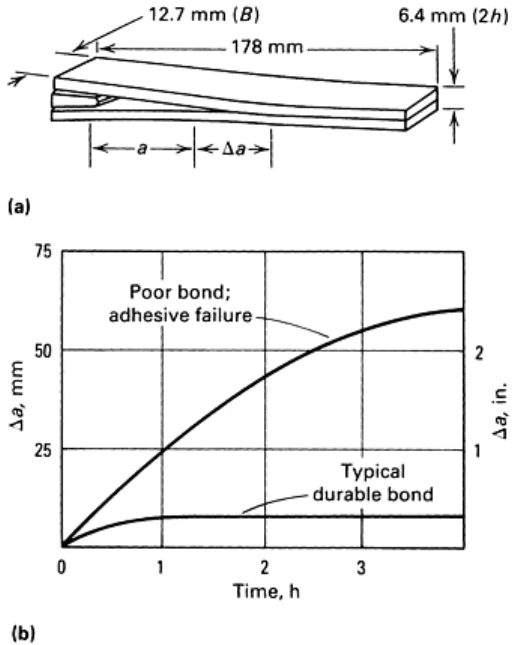
Fig. 8 Boeing wedge test (ASTM D 3762) (a) Test specimen. (b) Typical crack propagation behavior at 49

°C (120 °F) and 100% relative humidity. a, distance from load point to initial crack tip; Δa, growth

during exposure. Source: Ref 49

References cited in this section

7. Adhesives, Annual Book of ASTM Standards, Vol 15.06, ASTM (updated annually)

9. G.P. Anderson and K.L. DeVries, Predicting Strength of Adhesive Joints from Test Results, Int. J.

Fract., Vol 39, 1989, p191–200

21. G.P. Anderson and K.L. DeVries, Analysis of Standard Bond-Strength Tests, Treatise on Adhesion and

Adhesives, Vol 6, R.L. Patrick, K.L. DeVries, and G.P. Andersen, Ed., Marcel Dekker, 1989

22. J.K. Strozier, K.J. Ninow, K.L. DeVries, and G.P. Anderson, Adhes. Sci. Rev., Vol 1, 1987, p 121

23. G.P. Anderson, D.H. Brinton, K.J. Ninow, and K.L. DeVries, A Fracture Mechanics Approach to

Predicting Bond Strength, Advances in Adhesively Bonded Joints, Proceedings of a Conference at the

Winter Annual Meeting of ASME, 27 Nov-2 Dec 1988 (Chicago), S. Mall, K.M. Liechti, and J.K.

Vinson, Eds., ASME, 1988, p 98–101

24. V.L. Hein and F. Erodogan, Stress Singularities in a Two-Material Wedge, Int. J. Fract.,Vol 7, 1971, p

317

25. J.A. Marceau, Y. Moji, and J.C. McMillan, A Wedge Test for Evaluating Adhesive Bonded Surface

Durability, 21st SAMPE Symposium, Vol 21, 6–8 April 1976

49. J.C. McMillan, Developments in Adhesives in Engineering, 2nd ed., Applied Science, London, 1981, p

243

Testing of Adhesive Joints

K.L. DeVries and Paul Borgmeier, University of Utah

Adhesive Fracture Mechanics Tests

Fracture mechanics originated with the pioneering efforts of A.A. Griffith in the early 1920s. The field

remained relatively dormant until the late 1940s when it was developed into a very effective and valuable

design tool to describe and predict “cohesive” crack growth. Interested readers are referred to a number of

excellent texts on fracture mechanics (e.g., Ref 26 and Fatigue and Fracture, Volume 19 of the ASM

Handbook).

In the 1960s and 1970s, researchers began exploring the use of the concepts of fracture mechanics in adhesive

joint analysis as reviewed in Ref 3. These methods have the potential to use the results from a test joint to

predict the strength of other joints with different geometries.

In a common fracture mechanics approach (including Griffith's papers), the conditions for failure are calculated

by equating the energy lost from the strain field as a “crack” grows to the energy consumed in creating the new

crack surface. This energy per unit area, G

, determined from standard tests, is called by various names,

including the Griffith fracture energy, the specific fracture energy, the fracture toughness, or the energy release

rate.

In 1975, ASTM Committee D-14 adopted a test configuration and testing method with fracture mechanics

ramifications based on the pioneering efforts of Mostovy and Ripling (Ref 27, 28). The method is described in

ASTM D 3433 “Standard Test Method for Fracture Strength in Cleavage of Adhesives in Bonded Joints.”

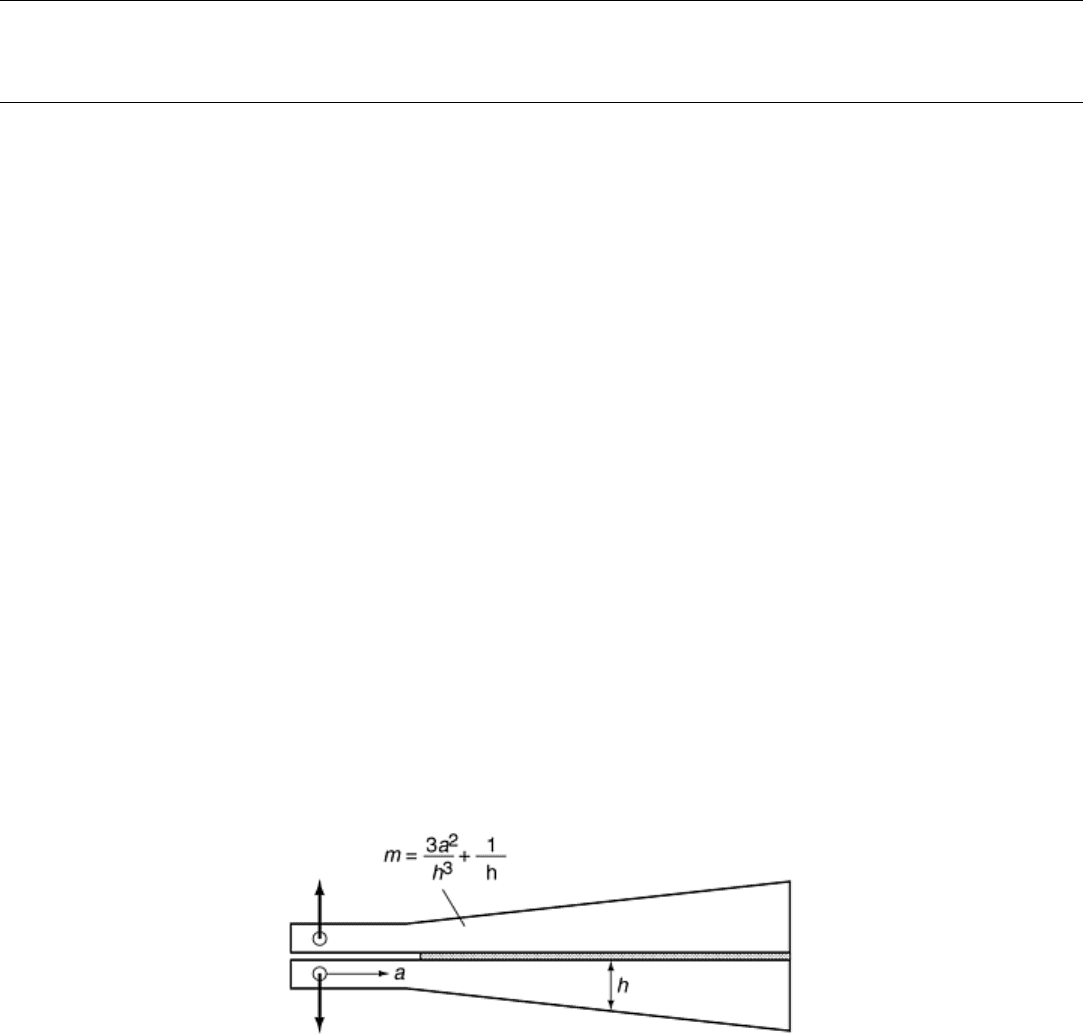
Figure 9 shows the shape and dimensions for one specimen type recommended for use in this standard. The

specimen is composed of two “beams” adhesively bonded over much of their length as shown. Testing is

accomplished by pulling the specimen apart by means of pins passing through the holes shown near the

sample's left end. This adhesive sample configuration and loading to failure gives rise to the sample's nickname,

“split-cantilever beam.” Another recommended geometry in ASTM D 3433 is similar except the adherends are

not tapered.

Fig. 9 Specimen for the contoured double-cantilever-beam test (ASTM D 3433)

It should now be clear that the stress distribution in adhesive joints is generally complex. Furthermore, the

details of this distribution are highly dependent on specific details of the joint system. The maximum stresses in

the bond almost always differ markedly from the average value, and elastic analyses often exhibit mathematical

singularities at geometric or material discontinuities. From these observations, it should be clear that the use of

the conventionally reported results from most tests (i.e., values of the average stress at failure) would be of little

use in designing joints that differ in any significant detail from the sample test configuration.

For the resolution of this problem, the concepts of fracture mechanics have much to offer. One of the more

popular and graphically appealing approaches to fracture mechanics views the joint as a system in which failure

(often considered as the growth of a crack) of a material (or joint) requires the stresses at the crack tip to be

sufficient to break bonds and an energy balance. It is hypothesized that even if the stresses are very large (often

theoretically infinite), a crack can grow only if sufficient energy is released from the stress field to account for

the energy required to create the new crack (or adhesive debond) surface as the fractured region enlarges. The

specific value of this energy (J/m

, or in. · lbf/in.

, of crack area) for the adhesive bonding problem uses the

same basic titles as given previously but prefaced with the term adhesive. Hence, adhesive fracture toughness

might be used to distinguish adhesive failure from tests of cohesive fracture. The word adhesion is dropped

from the comparable term when cohesive failure is being considered. The cohesive and adhesive embodiments

of fracture mechanics both involve a stress-strain analysis and an energy balance.

The analytical methods of fracture mechanics (both cohesive and adhesive) are described in Ref 3 and 25.

These are not repeated here other than a few comments on the concepts and a brief outline of a numerical

approach that can be applied where analytical solutions are tedious or impossible. Inherent in fracture

mechanics is the concept that natural cracks or other stress risers exist in materials and that final failure of an

object often initiates at such points. For a crack (or region of debond) situated in an adhesive layer, modern

computation techniques are available (most notably, finite element methods) that facilitate the computation of

stresses and strains throughout a body, even if analytical solutions may not be possible. The stresses and strains

are calculated throughout the entire adhesive system (adhesive and all adherends), including the effects of a

crack in the bond. These can then be used to calculate the strain energy, U

, stored in the body for the particular

crack size, A

. Next, the hypothetical crack is allowed to grow to a slightly larger area, A

, and the preceding

process is repeated to determine the strain energy, U

. This approach to fracture mechanics assumes that at

critical crack growth conditions, the energy loss from the stress-strain field goes into the formation of the new

fracture energy. The quantity ΔU/ΔA is called the energy release rate, where ΔU = U

- U

and ΔA = A

- A

The so-called critical energy release rate (ΔU/ΔA)

crit

is that value of the energy release rate that will cause the

crack to grow. Loads that result in energy release rates lower than this critical value will not cause failure to

proceed from the given crack, while loads that produce energy release rates greater than this value will cause it

to accelerate. This critical energy release rate value is equivalent to the adhesive fracture energy, or work of

adhesion, previously noted. While the model just described is conceptually useful, computer engineers have

devised other convenient ways of computing the energy required to “create” the new surface, such as the crack

closure method (Ref 29, 30).

It is hoped that this simple model of fracture mechanics will help the reader who is unfamiliar with fracture

mechanics to visualize the concepts of fracture mechanics. The molecular mechanisms responsible for the

fracture energy or fracture toughness are not completely understood. They generally involve more than simply

the energy required to rupture a plane of molecular bonds. In fact, for most practical adhesives, the energy to

rupture these bonds is a small but essential fraction of the total energy. The total energy includes energy that is

lost because of viscous, plastic, and other dissipation mechanisms at the tip of the crack. As a result, linear

elastic stress analyses are inexact.

While fracture mechanics has found extensive use in cohesive failure considerations, its use for analyzing

failure of adhesive systems is more recent. There has, however, been a significant amount of research and

development in the adhesive fracture mechanics area. To review it all, even superficially, would take more

space than is allocated for this article. A small sampling of publications in this extensive and rich area of

research is listed as Ref 12, 13, 26, 27, 28, and 31, 32, 33, 34, 35, 36, 37, 38, 39, 40, 41, 42, 43, 44, 45, 46, 47,

48, 49, 50, 51, 52, 53, 54, 55, 56, 57, 58. Not only is this listing incomplete, but also many of the researchers

listed have scores of other publications. It is hoped that the one or two listed for each investigator will provide

the reader with a starting point from which more details can be found from reference cross listings, searching of

citation indexes, abstracting services, and so on. These investigators have treated such subjects as theory; mode

dependence, effects of shape, thickness, and other geometric dependence; plasticity and other nonlinearities;

numerical methods; testing techniques; different adhesive types; rate and temperature effects; fatigue; and

failure of composites, as well as a wide variety of other factors and considerations in adhesion.

Modern finite element or other numerical methods have no difficulty in treating nonlinear behavior. Physical

understanding of material behavior at such levels is lacking, and effective use of the capabilities of such

computer codes depends, to a large extent, on the experimental determination of these properties. For many

problems, it has become conventional to lump all dissipative effects together into the fracture energy and not be

overly concerned with separating this quantity into its individual energy-absorbing components. Another

fracture mechanics approach, called the J-integral, has some advantages in treating nonlinear as well as elastic

behavior (Ref 51, 52, 59, and 60).

It was noted previously that most adhesive systems are not linearly elastic up to the failure point. Nevertheless,

researchers have shown that elastic analyses of many systems can be very informative and useful. Several

adhesive systems are sufficiently linear so that it is possible to lump the plastic deformation and other energy

dissipative mechanisms at the crack tip into the adhesive fracture energy (critical energy release rate) term.

There has recently been some significant success in explaining many aspects of adhesive performance and