Ames W.F., Harrel E.M., Herod J.V. (editors). Differential Equations with Applications to Mathematical Physics
Подождите немного. Документ загружается.


230
Elliott
€I.
Lieb
and
Jan
Philip
Solovej
As
we know from elementary thermodynamics, the energy per
unit volume
as
a
function of the particle density
(p(z)
in our case)
is the Legendre transform of the pressure
as
a
function
of
the chem-
ical potential
(Iv(z)I).
Thus, corresponding
to
-(2/15~~)1v(z)1~/~
in
(7),
there is the energy
(3/5)(3~~)~/~p(z)~/~,
which is the usual
kinetic energy expression in
TF
theory. Likewise, corresponding to
(6)
there is
a
kinetic energy which we call
w~(p(z)).
It is no longer
proportional to
p(~)~/~
but it is still
a
convex function of
p(z).
It is
proportional to
p(z)”lB2
for small
p,
while it is asymptotically equal
to
(3/5)(3~’)~/~p(z)~/~
as
p(s)
-+
00.
4
The
Many-Electron
Atom
The essential ingredient in the study of the many-electron Hamilto-
nian
HN
is to reduce it to
a
one-electron problem
HA
-t
l&(z)
with
an effective mean field potential
Ve~(z)
=
-Z/lzI+J
lz-yl-’p(y)d3y.
This reduction involves approximating the repulsive energy
in the ground
state
+
by
In standard
TF
theory the justification of this approximation is
done by using the correlation inequality of Lieb and Oxford (see
[6]
and
[7]).
This very same argument (and inequality) work in the pres-
ence of
a
magnetic field. If
B
is
not too large compared with
Z
it
continues to be effective. However, in the hyper-strong case
B
>>
Z3
the argument is no longer effective, the reason being that the corre-
lation estimate is three dimensional in nature, while the atom is now
effectively one-dimensional. The proof of
a
correlation estimate ap-
plicable in the hyper-strong case is difficult and will appear elsewhere
“91).
The
density
p
appearing in the mean field potential
Ve~
will not
be taken to be the exact (unknown) density of the ground state,

Atoms
in
the Magnetic Field
of
a Neutron Star
231
but rather an approximation to the exact density obtained from the
density functionals that we shall now define.
Armed with the foregoing, we introduce
a
(magnetic field depen-
dent)
TF
theory by means of the following functional of the unknown
electron density
p(z):
It differs from the usual
TF
functional only in the replacement of
(c~nst.)p(z)~/~ by
w~(p(z)).
We call this functional the
Magnetic
Thomas-Fermi Functional.
It
is
studied in detail in
[9].
The
paper
[13]
seems to be the earliest reference that uses
a
Thomas-
Fermi theory that takes
all
Landau levels into account. This theory
was
also studied in
[a]
and put on
a
rigorous basis in
[14]
for the
regime
B
N
Z4I3.
We now choose
our
density
p
to be the unique minimizer for
EMTF
constrained to the set
Jp
5
N.
We define the energy function that
appears in Theorem
1
to be the infimum
Theorems
4
and
5
play an essential role in the proof of Theorem
1.
What makes the proof work when
B
<<
Z3
is the fact that in the
analysis of the mean-field, one-particle Hamiltonian,
HA
+
V,s(z),
with
V,n(z)
=
-Z/lzI
+
JIz
-
~I-~p(y)d~y,
and with
p
being the
density that minimizes the
TF
energy, we are in the semiclassical
regime. The potential
'I/er(z)
has the following behavior in Z and
B
V,ff(z)
=
Z4/3v(2'/3z)
if
B
6
Z4i3
veff(z)
=
z
4/5B2/5v(Z-'/5B2/5z)
if
B
2
Z4I3,
(9)
where
v
is
a
function that does not depend significantly on
B
and
2.
Concentrating on the case
B
2
Z4I3
we see, by
a
simple rescal-
ing, that the Hamiltonisn
I€A
+
Vem(z)
is unitarily equivalent to the
operator
Z4/5B2/5[((hp
-
ba(z))
t
v(z)],
(
10)

232
Elliott
H.
Lieb
and
Jan
Philip
Solovej
where
In the opposite case, when
B
&
Z4f3,
we get
Z413
in place
of
Z4f5B2f5
in
(10)
and
h
=
(B/Z3)'/' and
b
=
(B2/Z)l15.
(11)
h
=
Z-'13 and
b
=
B/Z.
(12)
When
h
is small we can study
(10)
by semiclassical methods.
If
B
>>
Z413
we can replace
WB(~)
by its asymptotic form and
we define the
Strong Thomas-Fermi
functional
The analysis
Of
EMTF
and
ESTF,
which is
a
separate story in itself,
leads to the conclusions stated in
1,2
and
3
of Sect.
2.
Conclusions
1
and
2
were proved by Yngvason
[14];
3
is new. Since the
TF
energy
functional has
a
unique minimizing
p(z)
(because
&MTF
is strictly
convex in
p)
this
p
must be spherically symmetric. Thus we are led
to the following remarkable conclusion:
If
BIZ3
--+
0
as
Z
+
00,
the atom is always spherical (to leading
order) despite the fact
that
B
has
a
leading order eflect on the ground
state energy.
In region
2,
B
x
Z413,
we cannot say that all the electrons
are
in
the lowest Landau band, but
if
B
>>
Z413,
they are
-
as
the following
theorem states precisely.
THEOREM
6
If
IIf
is
the projection in the physical Hilbert space
onto the subspace where
all
electrons are in the lowest Landau band,
we can define the
confined
energy
Econf(N,
B,
2)
E
ground state energy of
II~HNII~.
(13)
Then,
if
N
<
XZ
for
some fixed
X
>
0,
we have that
E,-,,d(N,
B,
Z)/E(N,
B,
Z)
--+
1
if
B
--+
00
and
if
Z4f3/B
--+
0.
(14)

Atoms in the Magnetic Field
of
a Neutron Star
233
What happens
if
B
M
Z3?
Semiclassical analysis breaks down
(in the sense of being no longer asymptotically exact
as
2
+
00).
The atom is no longer spherical. However, the atom is
so
non-
semiclassical (one person called it post-modern) that another analysis
becomes possible. This analysis, which we discuss next, is reminis-
cent of Hartree theory for bosons
-
even though it is relevant for
fermionic electrons!
It
is
only the motion parallel to the magnetic field which can
no longer be described semiclassically. The motion perpendicular to
the field is still well approximated classically.
To
be more precise,
the atom consists of
a
bundle of one dimensional quantum systems
indexed by the position
21
=
(21,~)
perpendicular to the field
B.
The state of one of these one-dimensional systems is described by
a
finite family of orthogonal functions
e,,,
(j)
j
=
1,2..
.
in
L2(R)
which are not normalized but satisfy
lle?)ll
5
B/27r.
This condition
follows from the Pauli principle and the fact that the two-dimensional
density of states in the lowest Landau band is exactly
B/27r.
We can combine the functions
eg),
j
=
1,2,.
.
.
into
a
density
Then
7
satisfies
(a)
0
5
r,,
5
(B/27r)I
as an operator on
L2(R)
(b)
Jn2
Tr~zpq[~,,]d2x~
=
N
=
the total number of electrons
We can now approximate the energy by the functional
EDM(N,
B,
2)
=
inf{€(y)
:
7
satisfies (a) and (b) above}.
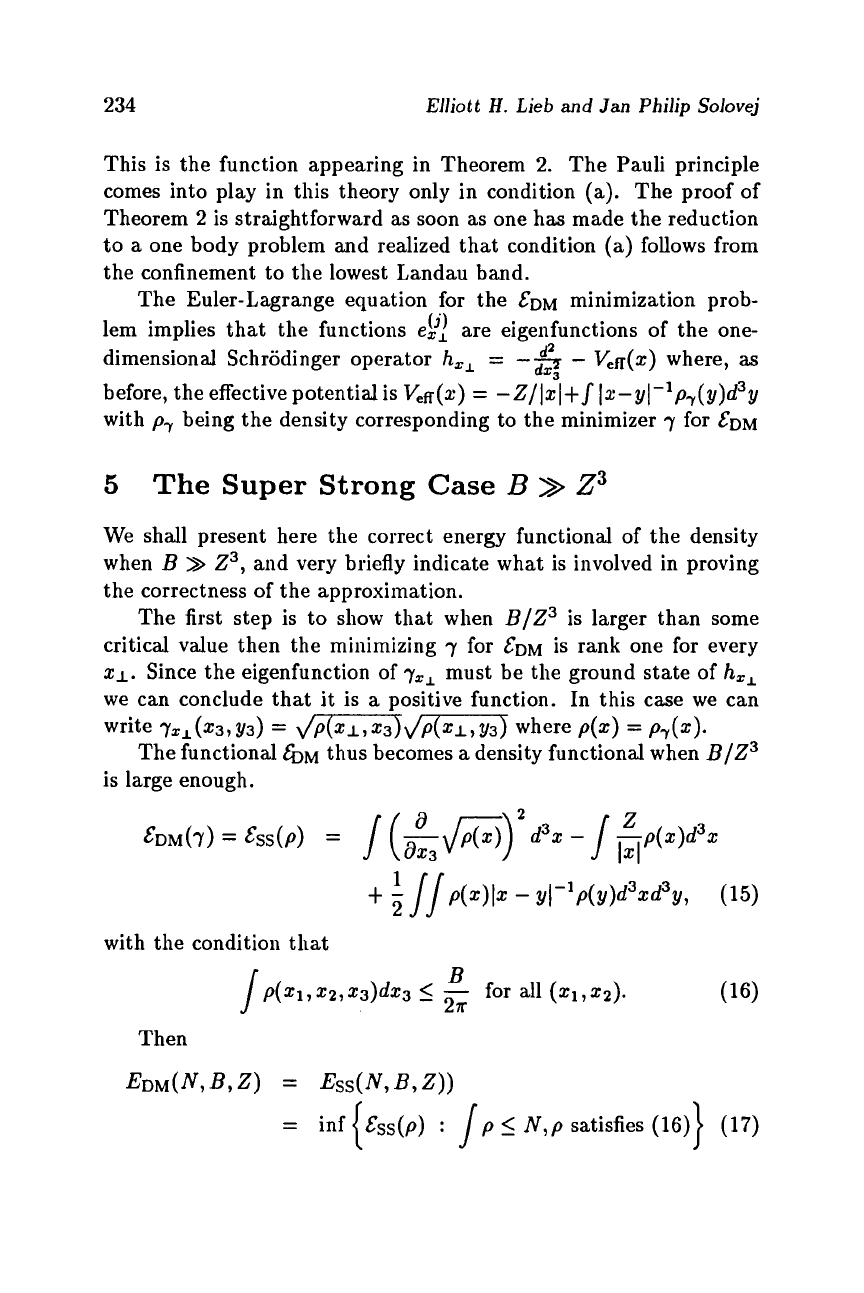
234
Elliott
H.
Lieb
and
Jan
Philip Solovej
This is the function appearing in Theorem
2.
The Pauli principle
comes into play in this theory only in condition (a). The proof of
Theorem
2
is straightforward as soon as one has made the reduction
to
a
one body problem and realized that condition
(a)
follows from
the confinement to the lowest Landau band.
The Euler-Lagrange equation for the
&DM
minimization prob-
lem implies that the functions
e$j
are eigenfunctions of the one-
before, the effective potential is
V&(z)
=
-Z/lzI+J
Iz-yl-’p.y(y)d3y
with
pr
being the density corresponding to the minimizer
7
for
&DM
dimensional Schrodinger operator
h,,
=
-q
d2
-
Ve~(z)
where,
as
5
The Super Strong
Case
B
>>
Z3
We shall present here the correct energy functional of the density
when
B
>>
Z3,
and very briefly indicate what is involved in proving
the correctness of the approximation.
The first step is to show that when
BIZ3
is larger than some
critical value then the minimizing
7
for
&DM
is rank one for every
21.
Since the eigenfunction
of
T,,
must be the ground state of
h,,
we can conclude that it is
a
positive function. In this case we can
The functional
&M
thus becomes
a
density functional when
BIZ3
write
7&3,
Y3)
=
Jpmdm
where
P(2)
=
P.y(.)’
is large enough.
with the condition that
Then
=
inf
{&ss(p)
:
/p
5
N,p
satisfies
(16) (17)
I

Atoms
in
the hfagnetic Field
of
a Neutron
Star
235
We can now ask
for
the limit of
&ss
if
BIZ3
-+
00,
Z
-,
00
and
N/Z
is fixed. With some effort one can prove that
&ss
then simplifies
to another functional, which we call the
hyper-strong functional
of
a
one-dimensional
density
pl(x), x
E
IR,.
That is, the atom is now
so
thin compared to its length that only the average density and its
variation along the direction parallel to
B
matter.
It is convenient, in defining this average density, to rescale the
variables. Thus, setting
77
=
B/(27rZ3),
and taking
(2
In
q)-'
as
the
unit of length, we define
which has the normalization
Jpl(x)dx
=
N/Z.
The hyper-strong
functional is
00
1
EHS(P1)
- -
Jm
(-&dz)2dx
-
PdO)
t
2
J
Pl(x)2dx.
(19)
--oo --oo
In
other words, apart from some scalings,
the
Coulonzb potential
is
replaced
by
a
Diruc
delta function!
Using
(19)
we define
a
rescaled
energy
We assert that under the conditions stated above,
Z3(h
T~)~EHs(N/Z)/E(N,
B,
2)
+
1
as
Z
+
00,
BIZ3
-
00
and
N/Z
is fixed.
A
remarkable fact is that the minimizing
p1
can be evaluated
exactly. The Euler-Lagrange equation is (with
$2
=
p1
and Lagrange
multiplier
p)
-
li;(x)
-
+(O)S(X)
t
$3(x)
=
-p$,(x).
(21)
With
X
=
N/Z,
there are solutions only for
X
5
2
(not
X
5
1
as in
TF
theory):
+(x)
=
+
for
x
<
2
$(x)
=
d(2+
for
X
=
2,
2
Slnh[~(2--X)~Z)+C]
(22)

236
Elliott
H.
Lieb
and
Jan
Philip Solovej
with tanhc
=
(2
-
X)/2.
The energy is
11 1
4 48
EHS(X)
=
=
--A
+
EX2
-
--A3.
Bibliography
[l]
I.
F’ushiki,
E.
H. Gudmundsson, and C.
J.
Pethick,
Surface struc-
ture
of
neutron stars with high magnetic fields,
Astrophys. Jour.
342,
(1989), 958-975.
[2]
I.
Fushiki,
E.
H. Gudmundsson,
C.
J.
Pethick, and
J.
Yngvason,
Matter in
a
magnetic field
in
the Thomas-Fermi and related
theories,
Ann.
of
Phys. (in press).
[3]
B.
Helffer and D. Robert,
Calcul fonctionel
par
la
transformet!
de Mellin et operuteurs admissible,
Jour. Func. Anal.
53,
(1983),
246-268.
[4]
V.
Ivrii, in preparation.
[5]
B.
B.
Kadomtsev,
Heavy Atoms in
an
Ultrastrong Magnetic
Field,
Soviet Phys.
JETP
31,
(1970), 945-947.
[6]
E.
H. Lieb,
Thomas-Fermi and related theories
of
atoms
and
molecules,
Rev. Mod. Phys.
53,
(1981) 603-641.
Errata,
54,
(1982) 311.
(71
E.
H.
Lieb and
S.
Oxford,
Improved lower bound
on
the indirect
Coulomb energy,
Int.
J.
Quant. Chem.
19,
(1981), 427-439.
[8]
E.
H. Lieb and
B.
Simon,
The Thomas-Ferrni theory
of
atoms,
molecules and solids,
Adv. in Math.
23,
(1977), 22-116.
[9]
E.H. Lieb,
J.
P.
Solovej and
J.
Yngvason, in preparation.
[lo]
E.
H.
Lieb and W.
E.
Thirring,
A
bound for the moments
of
the
eigenvalues
of
the Schrodinger Hamiltonian and their relation to
Sobolev inequalities,
in
Studies in kfathematical Physics: Essays
in
Honor
of
Valentine Baqrnann,
ed.
E.
H. Lieb,
B.
Simon and
A.
S.
Wightman, Princeton University Press
(1977).

Atoms in the Magnetic Field
of
a
Neutron Star
237
[ll]
R.
0.
Mueller,
A.
R.
P.
Rau and
L.
Spruch,
Statistical Model of
Atoms in Intense Magnetic Fields,
Phys. Rev. Lett.
26,
(1971)
1136.
[12]
M. Ruderman,
Mutter in superstrong magnetic fields: The
sur-
face ofa neutron star,
Phys.
Rev. Lett.
27,
(1971), 1306-1308.
[13]
Y. Tomishima and
K.
Yonei,
Thomas Fermi theory for atoms in
a strong magnetic field,
Progr.
Theor.
Phys.,
59,
(1978), 683-
696.
[14]
J.
Yngvason,
Tliomas-Fermi theory for matter in a magnetic
field as
a
limit
of
quantum mechanics,
Lett. Math.
Phys.
22,
(1991) 107-117.

This page intentionally left blank
Algebraic Riccati Equations
Arising
in
Game
Theory and
in
IP-Control Problems
for
a
Class
of
Abstract Systems
C.
McMillan and
R.
Triggiani
University
of
Virginia
Abstract
We consider the abstract framewoin of
[4,
class
,
)]
whic
I
models
a
variety of mixed partial diflerential equation problems in
a
smooth bounded
doma.in
R
c
R",
arbitrary
n,
with boundary
L2(0,
T;
aO)-control. These include second-order hyperbolic equa-
tions, first-order hyperbolic systems, Euler-Bernoulli, Kirchhoff,
Schroedinger equations, etc.
For these dynamics we set and
solve
a
min-max game theory problem
-
a
fortiori the Hoo-robust stabi-
lization problem
-
in terms of an algebraic Riccati equation to express
the optimal quantities in pointwise feedback form.
1
Introduction
1.1
Problem
Setting
Let
U
(control) and
Y
(state) be separable Hilbert spaces. We in-
trod u ce the following abstract state equation
G(t)
=
Ay(2)
t
Bu(t)
t
Gw(2)
in
[D(A*)]';
y(0)
=
yo
E
Y
(1)
Differential Equations with
Copyright
@
1993
by
Academic Press, hc.
Applications
to
Mathematical All rights
of
reproduction in any
form
reserved.
Physics
ISBN
0-12-056740-7
239
