Ames W.F., Harrel E.M., Herod J.V. (editors). Differential Equations with Applications to Mathematical Physics
Подождите немного. Документ загружается.


This page intentionally left blank

Atoms
in
the Magnetic Field
of
a
Neutron
Star
Elliott
H.
Lieb' and Jan Philip Solovej
Department
of
Mathematics
Princeton University
Princeton,
NJ
08544
Abstract
The ground state energy of an atom of nuclear charge
Ze
and
in
a
magnetic field
B
is evaluated exactly in the asymptotic regime
Z
+
00.
We
present the results of
a
rigorous analysis that reveals the
existence of
5
regions as
Z
+
00:
B
<<
Z4I3,
B
M
Z4I3,
Z4I3
<<
B
<<
Z3,
B
M
Z3,
B
>>
Z3.
Different regions have different physics and
different asymptotic theories. Regions
1,2,3,5
are described exactly
by
a
simple density functional theory, but only in regions
1,2,3
is it of
the semiclassical Thomas-Fermi form. Region
4
cannot be described
exactly by any simple density functional theory; surprisingly, it can
be described by
a
simple
density
matrix
functional theory.
1
Introduction
In these talks we shall discuss the effect on matter, specifically the
ground state of atoms, of
a
very strong magnetic field. Results ob-
tained in collaboration with
J.
Yngvason will be summarized and
details will appear elsewhere
[9].
The physical motivation for study-
ing extremely strong magnetic fields of the order of
10'2-1013
Gauss
'Work supported
by
U.S.
National Science Foundation grant no. PHY9O-
19433.
Differential Equations with Applications
to Mathematical
Physics
ISBN
0-12-056740-7
Copyright
@
1992
by the authont.
Reproduction
of
this
article in its entirety
by any means is permitted.
221

222
Elliott
H.
Lieb
and
Jan
Philip
Solovej
is that they are supposed to exist on the surface of neutron stars.
This study
was
essentially begun in the early
70’s
with the work of
Kadomtsev
[5],
Rudernian
[12]
and Mueller, Rau and Spruch
[ll];
see
[l]
and
[2]
for further references. The argument given to explain these
strong fields is that in the collapse, resulting in the neutron star, the
magnetic field lines are trapped and thus become very dense. The
structure of matter in strong magnetic fields is, therefore,
a
question
of considerable interest in astrophysics. Mathematically, the problem
turns out to involve an interesting exercise in semiclassical analysis.
We use units in which
e
=
h
=
2me
=
1.
The natural unit
of
length is h2/2mee2, i.e., half the Bohr radius. The natural unit
of
magnetic field strength that we shall use is
(2me)2e3~/h3
=
9.4
x
lo9
Gauss. This is the field for which the magnetic length
dm
equals
half the Bohr radius. Thus, in our units,
B
z
lo2
-
lo3
for some
neutron stars.
The atomic nucleus of principal interest on the surface of
a
neu-
tron star is presumably iron with
2
=
2G.
This number is large and
hence it is sensible to ask (rigorously) about the limit of the ground
state energy of an atom
as
2
+
00.
We shall calculate this limit
exactly; its application to
2
=
26
instead of
2
=
00
will entail some
errors
-
for which we can give bounds.
2
Main
Results
To give the quantum mechanical energy of
a
charged spin-f particle
in
a
magnetic field
B,
we have to make
a
choice of vector potential
A(z),
satisfying
B
=
V
x
A.
The energy is then given by the Pauli
Hamil tonian
Here
p
=
-iV
and
cr
=
(01,
u2,
us),
are the Pauli matrices. We can
also
write
HA
=
(p
-
A)2
-
B
-
cr.
We shall here concentrate on the
case where
B
is constant, say
B
=
(O,O,
B),
with
B
2
0. We choose
A
=
fB
x
X.
The Hamiltonian describing an atom with
N
electrons and nu-
clear charge
2
(with fixed nucleus) in
a
constant magnetic field
B

Atom
in the Magnetic Field
of
a
Neutron Star
223
N
The operator
HN
acts on the Hilbert space
'HN
=
A
L2(R3;
C2)
of
antisymmetric (i.e., fermionic) spinor-valued functions. We are in-
terested in
E(N,
B,
2)
=
inf specx,
HN,
the ground state energy
Of
HN.
We want to let
B
and
2
go
to infinity. It is surprising, but true,
that there are
five
different regimes in
B
and
2,
depending on the
relative magnitudes
of
B
and
2.
In the following
p(z)
is the electron
density in the ground state
+:
The five regions are the following.
1.
B
<<
Z413,
2
large:
The effect of the magnetic field is negligible.
Standard Thomas-Fermi
(TF)
theory is exact
as
2
-,
00,
and
therefore the electron density
is
spherical to leading order.
2.
B
N
Z4I3,
2
Zurge:
The magnetic field becomes important
but the density is still almost spherical and stable atoms are
almost neutral (see
[14]).
A
modified
TF
theory (depending on
the constant
B/Z413),
in which the energy,
as
in standard
TF
theory, is approximated by
a
functional of the density
p
alone,
is exact as
2
+
00.
We call this functional the Magnetic
Thomas-Fermi (MTF) functional (see Sect.
4
below).
3.
Z413
<<
B
<<
Z3,
2
large':
The magnetic field is increasingly
important.
To
leading order
all
electrons will be confined to
the lowest Landau band. The modified
TF
theory is still ex-
act
as
2
-,
00.
In fact, the modified
TF
theory simplifies
somewhat in this region compared to the MTF functional from
the previous region. We call the new functional the Strong
Thomas-Fermi
(STF)
functional. The only difference between
STF and standard
TF
theory is that the usual
p513
is replaced

224
Elliott
H.
Lieb
and
Jan
Philip
Solovej
by
p3/B2,
while in the MTF theory from the previous region
the function that replaces
p5/3
is more complicated (see
(8)
below). The density is almost spherical and stable atoms are
almost neutral. Furthermore, the atom is getting smaller. The
atomic radius behaves like
Z'I5
B-2/5
=
Z-'I3(
B/Z4/3)-2/5.
4.
B
N
Z3,
2
large:
The modified
TF
theories are no longer
applicable. Indeed, we shall in general not approximate the
energy by functionals of the density
p
alone. The energy is
approximated by
a
more complicated functional to be described
below in Sect.
4
depending on
a
one particle density matrix. We
call this functional the Density Matrix (DM) functional. When
BIZ3
is large enough this functional again reduces to
a
density
functional. For the first time the atom is no longer spherical to
leading order. The length scale of the atom behaves like
2-'
and the energy like
Z3.
5.
B
>
Z3,
2
large:
In this hyper-strong case the atom is
es-
sentially one-dimensional. We can find
a
new functional, the
Hyper-Strong
(HS)
functional depending only on the one-di-
mensional density
7 obtained from
p
by integrating
p
over the
directions perpendicular to the field
B,
i.e.,
The energy behaves like
29/5B2/5
=
Z7I3(B/Z
4/3
)
2/5
.
p(23)
=
//p(xl,X?,X3)d2ld+2
-
The energy behaves like 23[ln(B/23)]2 and the length scale
along the magnetic field is 2-'[1n(B/Z3)]-', while the radius
perpendicular to the field is
Z-'(B/Z3)-'/2.
The mathematically more precise statements of these results in-
volve two energy functions
EMTF(N,
B,
2)
and
EDM(N,
B,
2).
The
energy
EMTF
is obtained as the minimum of the magnetic Thomas-
Fermi functional mentioned under
2
above, and
EDM
is the minimum
of the density matrix functional mentioned under
4.
The exact defi-
nitions of these functionals are given in Sect.
4
below.
The energies
EMTF
and
EDM
correspond to unique minimizers
for the respective functionds. We denote the densities for these
minimizers by
PMTF
and
PDM
respectively.

Atoms
in
the Magnetic Field
of
a
Neutron
Star
225
In the case when
B
=
0
the energy
EMTF(N,O,Z)
is the energy
of standard
TF
theory. It is known
[8]
(see also
[S])
that
TF
theory
is asymptotically
exact
as
Z
+
00
with
N/Z
fixed, i.e.,
EMTF(N,O,Z)/E(N,O,Z)
-+
1
as
Z
-+
00.
Is
the same true when
B
#
O?
The answer, surprisingly, depends
on the relative magnitudes
of
B
and
2,
according to the
5
regions
outlined above.
THEOREM
1
Let
N/Z
be fixed
and
suppose
BIZ3
-+
0
as
Z
-+
00.
Then
EMTF(N,
B,
Z)/E(N,
B,
2)
-+
1
as
Z
-+
00.
This theorem covers the regions
1-3
above.
For
the regions
4
and
5
we have
THEOREM
2
Let
N/Z
be fixed and suppose
B/Z4I3
-+
00
as
Z
-+
00.
Then
Notice that there is an overlap
of
the regions of validity
of
the
The energy functions satisfy the scalings
two theorems. In fact, both theorems cover region
3
above.
EMTF(N,
B,
z)
=
z7/3~MTF(~/z,
B/z~/~,
1)
and
EDM(N,B,Z)
=
Z3EDM(N/Z,B/Z3,1)
In region 2 there is
a
non-trivial parameter
B/Z4I3.
Likewise in
region
4
there is
BIZ3.
In the other three regions these parameters
enter in
a
trivial way since they are tending either to
0
or
00.
Region
1
corresponds to
B/Z4I3
+
0
and
B/Z3
-+
0
in which
case
EMTF(N/Z,
1)
+
EMTF(N/Z,
0,1),

226
Elliott
H.
Lieb
and
Jan
Philip
Solovej
which is the energy of standard
TF
theory.
asymptotic expansion
EMTF(N/Z,B/Z~/~,
1)
X
(B/z4/3)2/5E~~~(N/Z)
as
+
00
,
where
E~TF
is an energy function obtained from the simplified
TF
theory described under
3
above.
The overlap of the regions of validity of Theorems
1
and
2
implies
that
Region
3
corresponds to
B/Z4i3
-,
00,
in which case we have the
EDM(N/Z,B/Z~,
1)
X
(B/z3)2/5Es~~(N/z)
as
BIZ3
-+
0
.
Finally, region
5
corresponds to
BIZ3
-+
00,
where the following
asymptotic formula holds
EDM(N/Z,B/Z~,
1)
X
[~I~(B/Z~)]~EHS(N/Z)
as
BIZ3
-+
00
,
where
EHS
is an energy function obtained from the one-dimensional
functional mentioned in
5
above.
The energies
EMTF,
EDM,
E~TF
and
EHS
correspond to unique
minimizers for the respective functionals.
We denote the densities
for these minimizers by
PMTF,
PDM,
ps~~
and
pHs
respectively. We
can prove that these densities approximate the quantum density
p.
However, to state these approximations we have to introduce different
scalings in the different regions. In fact, the above approximating
densities satisfy the following scaling relations
THEOREM
3
(Convergence
of
the density)
In the five difler-
ent regions the
following
relations hold
as
Z
-,
00.
These limits
are
all
in weak
L:,,=
:
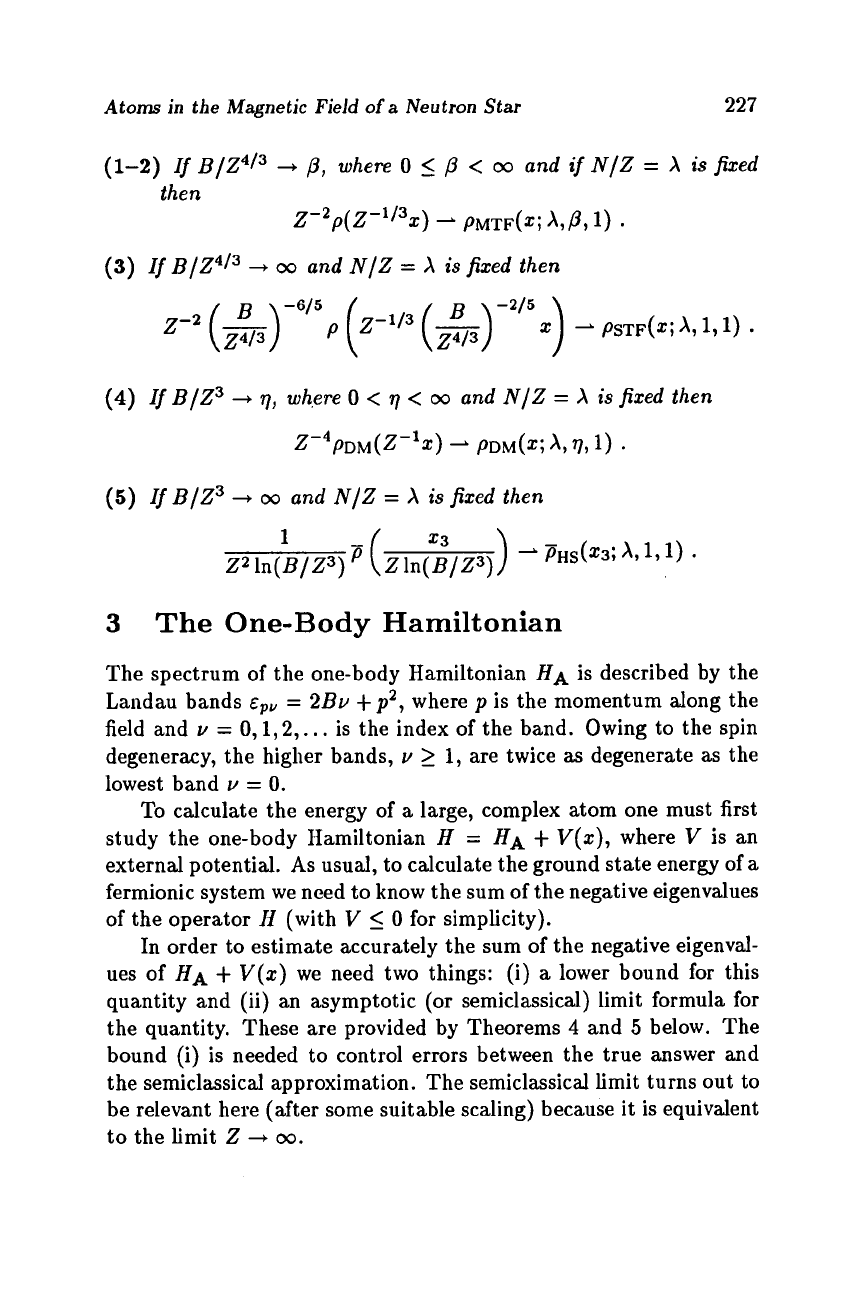
Atoms
in the Magnetic Field
of
a
Neutron
Star
227
(1-2)
If
B/Z4I3
-+
/3,
where
0
5
/3
<
00
and
if
NIZ
=
A
is
fixed
then
z-~~(z-'/~x)
~MTF(x;
X,P,
1)
.
(3)
If
B/Z4I3
+
00
and
NIZ
=
X
is
fixed then
(4)
If
BIZ3
+
q,
wh.ere
0
<
q
<
00
and
NIZ
=
X
is
fixed then
z-4PDM(z-'2) PDM(x;
A,
7~1)
-
(5)
If
BIZ3
+
00
and
NIZ
=
X
is
fixed then
1
Z21n(B/Z3)'
(
Zln(B/Z3)
3
The One-Body Hamiltonian
The spectrum of the one-body Hamiltonian
HA
is described by the
Landau bands
cpv
=
2Bu
+
p2, where p is the momentum along the
field and
u
=
0,1,2,.
. .
is the index of the band. Owing to the spin
degeneracy, the higher bands,
v
2
1,
are twice
as
degenerate as the
lowest band
u
=
0.
To calculate the energy of
a
large, complex atom one must first
study the one-body Hamiltonian
H
=
HA
+
V(x),
where
V
is an
external potential.
As
usual, to calculate the ground state energy of
a
fermionic system
we
need to know the sum
of
the negative eigenvalues
of the operator
H
(with
V
5
0
for simplicity).
In order to estimate accurately the sum of the negative eigenval-
ues
of
HA
-+
V(x)
we need two things: (i)
a
lower bound
for
this
quantity and (ii) an asymptotic
(or
semiclassical) limit formula for
the quantity. These are provided by Theorems
4
and
5
below. The
bound (i)
is
needed to control errors between the true answer and
the semiclassical approximation. The semiclassical limit turns out to
be relevant here (after some suitable scaling) because it is equivalent
to the limit
Z
-+
00.

228
Elliott
H.
Lieb
and
Jan
Philip
Solovej
There is an important difference between
HA
and the operator
(p
-
A)2
which has no spin dependence. While the spectrum of
(p
-
A)2
is
(B,
m)
the spectrum of
HA
is
(0,
m).
Indeed, one can
bound the sum of the negative eigenvalues of
(p
-
A)2
-
V(z)
by
-L
J
IV(Z)~~/~
dx,
(where
L
is some fixed constant) according to the
standard Lieb-Thirring inequality (even with
a
magnetic field the
proof of this inequality given in
[lo]
is
still correct if one appeals to the
diamagnetic inequality). However, in the case
Of
HA+V
the question
is somewhat more subtle. In fact,
if
JIV13/2
<
00,
the operator
(p
-
A)2
+
V
has
a
finite number of negative eigenvalues, while
the operator
HA
+
V
can have infinitely many negative eigenvalues
(compare
[4]).
We can, however, prove
[9]
the following bound which
is important in our proofs.
THEOREM
4
There exist universal constants
L1,
L2
>
0
such that
if we let
ej(B,
V),
j
=
1,2,.
. .
denote the negative eigenvalues
of
HA
+
V
with
V
5
0
then
We can choose
L1
as close
to
2/3n
as we please, compensating with
L2
large.
The first term on the right side
is
a
contribution from the lowest
We now ask the question of
a
semiclassical analog of
(4).
Thus,
band,
u
=
0.
For
large
B
this is the leading term.
consider the operator
WP
-
ba(4)
-
gl2
t
w
9
(5)
where
a($)
=
ti
x
x,
i
=
(O,O,
1)
and
v
5
0.
If one computes the leading term in
h-’
of the sum of the negative
eigenvalues of
(5)
for fixed
b
one finds as in
[3]
that there is no
b
dependence. In our case, however, we shall not assume
b
fixed,
or
more precisely not assume that
b
is small compared with
h-’.
The
reason for this is that
in
the application to neutron stars it is not
true,
as
we
shall discuss below, that
b
<<
h-l.

Atoms
in
the Magnetic Field
of
a
Neutron Star
229
The interesting fact is, however, that we can prove ([9])
a
semi-
classical formula for the sum of the negative eigenvalues of the oper-
ator
(5),
which holds uniformly in
6
(even for large
6).
THEOREM
5
Let ej(h,
6,
v),
j
=
1,.
. .
,
denote the negative eigen-
values
of
the operator
(5),
with
v
5
0. Then
uniformly in
6,
where
Escl
is the semiclassical approximation defined
6Y
00
Esd(h,
b,
4
=
-
3
h-26/(Iv(x)13/2
+
2
x[lv(x)l
-
2v6h]y2)
d3x
.
Here [t]+
=
t if t
>
0, zero otherwise.
u=l
(6)
The formula
(6)
was already implicitly noted in
[14].
The inte-
grand in
(6)
looks peculiar, but it has the following simple physical
interpretation. Take
a
cubic box of volume
L3
in
R3
and let the
number
p
>
0
be some fixed Fermi level
(or
chemical potential).
Then add together all the negative eigenvalues of
HA
-
p.
In the
thermodynamic limit (large
L)
we can do this addition simply by
us-
ing the known Landau levels, and the total energy per unit volume
is the integrand in
(G)
in which
Iv(x)I
is set equal to
p.
For
6h
<
1,
the right side
of
(6)
reduces to the standard semi-
classical formula from [3],
(Recall that we are counting the spin which accounts for the
2
in
front of the sum in
(G).)
For
bh
>>
1,
the sum in (6) is negligible,
and we are left with the first term.
Formula
(6) (with
h
replaced by
1)
can be compared with the
Lieb-Thirring inequality
(4),
which holds even outside the semiclas-
sical regime. The two terms in
(4)
correspond to respectively the
b
+
00
(first term) and
6
+
0
(last term) asymptotics of
(6)
.
