Ames W.F., Harrel E.M., Herod J.V. (editors). Differential Equations with Applications to Mathematical Physics
Подождите немного. Документ загружается.

lS0
Nail
H.
Ibragimov
which satisfies the condition
X1
V
X2
=
0
of
Theorem l(iv). The
op-
erators
(10)
are of the type
I1
from Table
2.
Therefore
a
linearization
is obtained
by
turning to the canonical variables
in which the operators
(10)
become
in accordance with Table
2.
Then, excluding the special solution
y
=
const., we have the transformed equation
(9):
-/I
y
+1=0.
Example
4.
We now take equations
and verify that the
Question:
When a nonlinear equation of the form
(11)
is lin-
earized?
has the
Answer
:
Never.
Indeed, our equation
(11)
is
a
particular case of
Eq.
(2)
with
coefficients
F1
=
F2
=
F3
=
0.
The system
(3)
is
2,
=
z2
+
FW
-
Fy,
zy
=
-zw,
W,
=
ZW,
wy
=
-w2,
and one of the integrability conditions, namely
yields
Fyy
=
0.
It follows that
Eq.
(ll),
where
F(x,y)
is nonlinear in
y,
is not lin-
earizable.
Linearization
of
Ordinary Differential Equations
181
Example
5.
Here we discuss in detail
a
construction of
a
lin-
earization. One can readily find that the equation
with an arbitrary function
f
admits- the 2-dimensional Lie algebra
spanned by
(13)
2a
a a
28
XI=
x
-+
xy-,
x2
=
xy-+ y
-.
dX
aY
ax
ay
This algebra belongs to the type
I1
of Table
2.
Therefore Eq.
(12)
can be linearized and
a
linearizing change of variables
Z
=
4(x, y),
g
=
$(x,
y)
is obtained from the conditions
We have from
(14)
the following four equations to determine
4,
$:
m=g(:),
$=--+h(x).
1
X
X
By these functions the first Eq.
(lG)
is satisfied identically while the
second one gives
4
=
y/x.
We choose
h
=
0
to obtain the following
change of variables:
After this transformation the equation
(12)
becomes
y”
+
f(T)
=
0.

182
Nail
H.
Ibragimov
2
First
Order
Equations
In the case of first-order equations Theorem
1
is replaced by the
following.
Theorem
2
Given a first-order onlinary differential equation
one can
by
means
of
an appropriate change
of
variables
transform
(17)
into any given equation
We consider here, instead of general changes
(18)
of both inde-
pendent and dependent variables, transformations of the dependent
variable only:
B
=
+(Y).
(20)
If
Eq.
(17)
is linear, then after transformation
(20)
we have, in
general,
a
nonlinear equation
(19).
This equation will be
a
particular
case of equations possessing
a
fundamental system of solutions,
or
a
nonlinear superposition principle
([5]-[9]).
Further, any first-order
ODE
possessing
a
nonlinear superposition can be written after
a
transformation
(20)
in
the form of
a
Riccati equation
yl
=
P(x)
+
Q(x)y
+
R(x)y2.
(21)
So,
the question is when is
Eq.
(21)
linearized by
a
transformation
of the form
(20)?
We formulate an answer
as
follows
([lo]):
Theorem
3
If
the Riccati equation
(21)
possesses one
of
the follow-
ing
four properties, then it should possess all
of
them:
(i) Eq.
(21)
is
linearized
by
a transformation
(20):
(ii)
Eq.
(21)
can be written
in
the
form
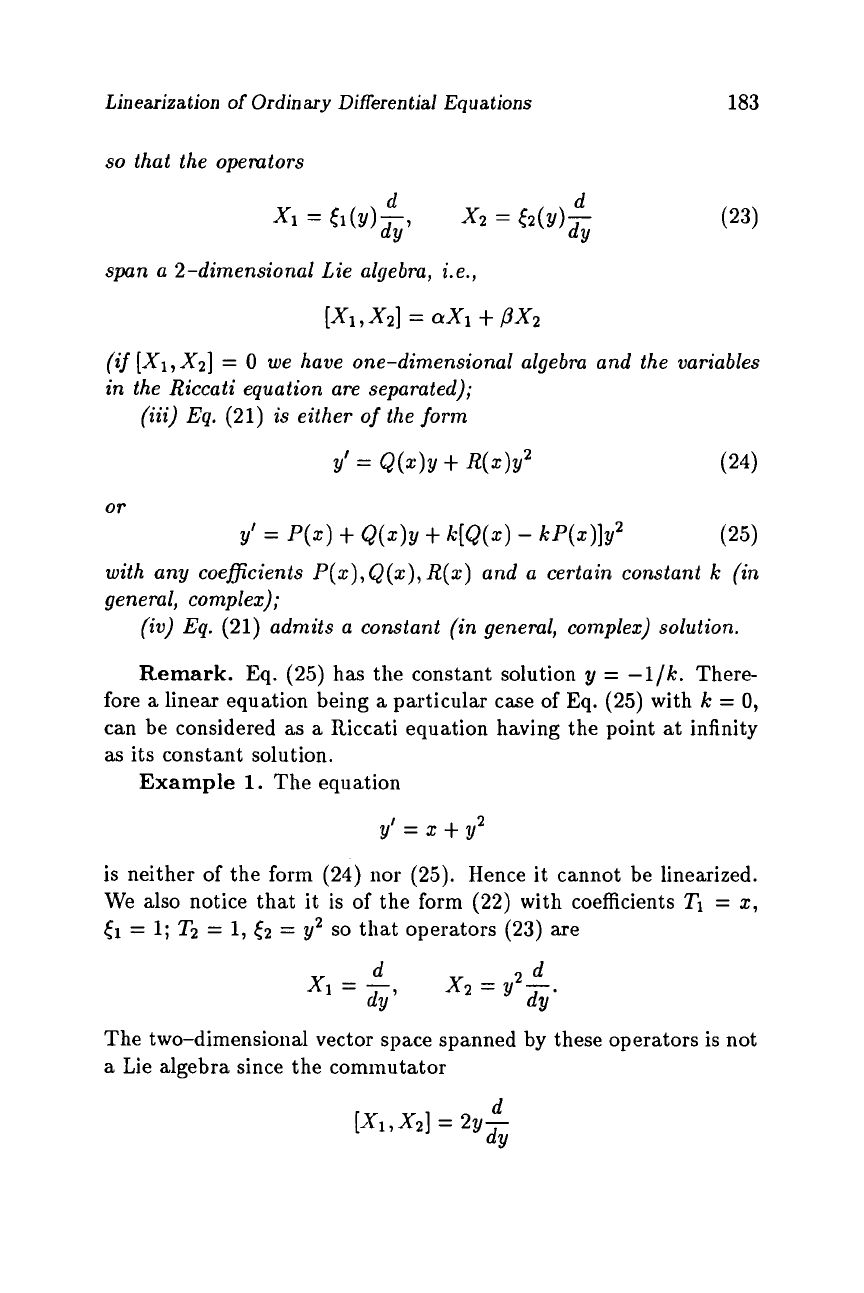
Linearization
of
Ordinary Differential Equations
183
so
that the operators
d
d
x1
=
&(Y)-,
x2
=
(2(Y)-
dY
dY
(23)
span a 2-dimensional Lie algebra, i.e.,
[Xl,
x21
=
ax1
+
PX2
(if
[Xl,X2]
=
0
we have one-dimensional algebra and the variables
in
the Riccati equation are separated);
(iii) Eq. (21)
is
either
of
the form
Yl=
QWY
+
R(X)Y2
(24)
Or
Yl
=
P(x>
+
Q(4Y
+
“(4
-
WXC>lY2
(25)
with any coefficients P(x), Q(x),
R(z)
and a certain constant
k
(in
general, complex);
(iv) Eq. (21) admits a constant (in general, complex) solution.
Remark.
Eq.
(25)
has the constant solution
y
=
-l/k.
There-
fore
a
linear equation being
a
particular case of Eq.
(25)
with
k
=
0,
can be considered as
a
Riccati equation having the point at infinity
as its constant solution.
Example
1.
The equation
y’=x+y
2
is neither of the form
(24)
nor
(25).
Hence it cannot be linearized.
We also notice that it is of the form
(22)
with coefficients
TI
=
2,
(1
=
1;
T2
=
1,
(2
=
y2
so
that operators
(23)
are
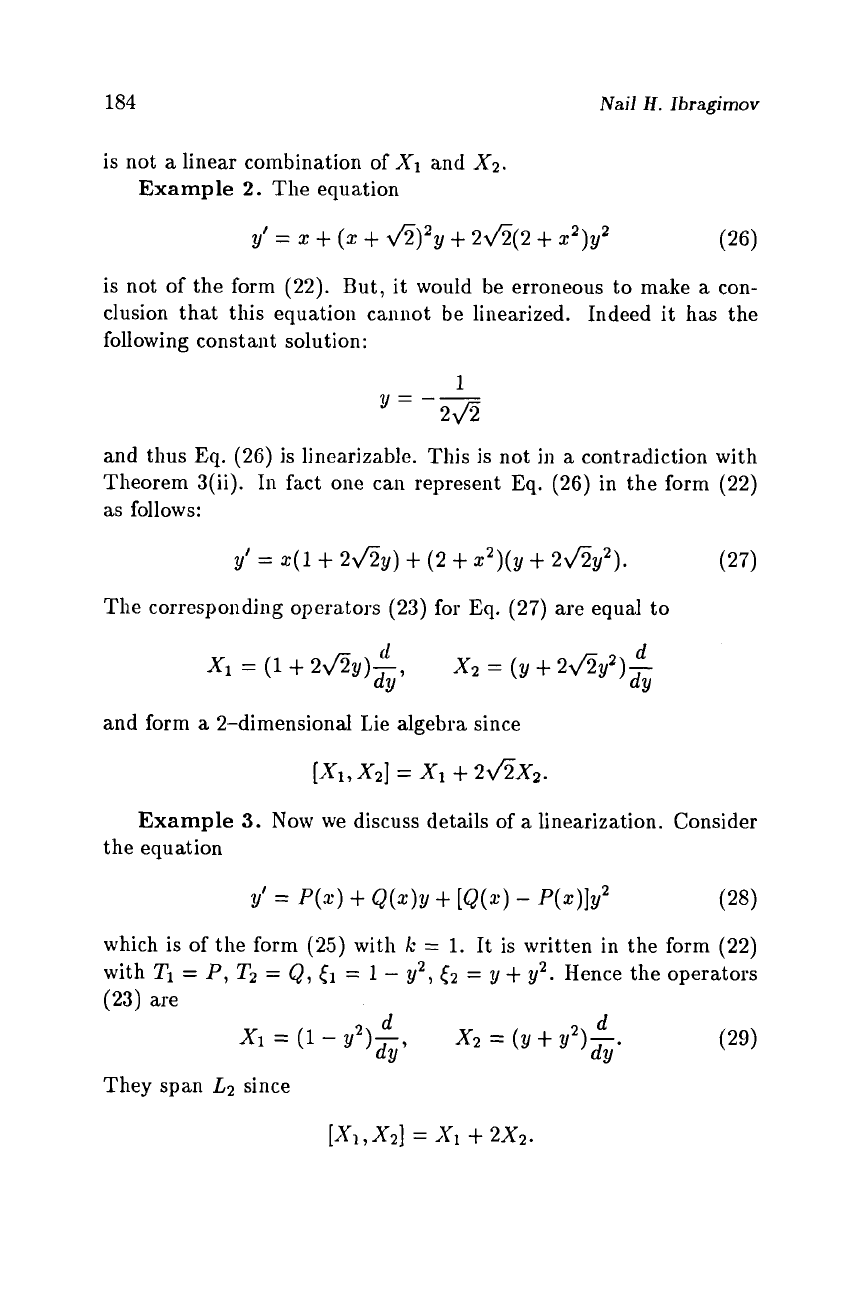
The two-dimensional vector space spanned by these operators is not
a
Lie algebra since the commutator
184
Nail
H.
lbragirnov
is not
a
linear combination of
X1
and
X2.
Example
2.
The equation
is not of the form
(22).
But, it would be erroneous to make
a
con-
clusion that this equation cannot be linearized. Indeed it has the
following constant solution:
and thus
Eq.
(26)
is linearizable. This is not in
a
contradiction with
Theorem 3(ii).
In
fact
one
can
represent
Eq.
(26)
in the form (22)
as follows:
Yl
=
x(1+
2Jzy)
+
(2
+
x2)(y
+
2Jzy2).
(27)
The corresponding operators
(23)
for
Eq.
(27)
are equal to
d
d
x1
=
(1
+
2JZY)-,
x2
=
(Y
+
2JzYZ);i;;
dY
and form
a
2-dimensional Lie algebra since
[Xl,
XZ]
=
x1
+
2hX2.
Example
3.
Now
we discuss details of
a
linearization. Consider
the equation
Y'
=
P(x)
t
Q(x)y
+
[Q(x)
-
P(x)]y2
(28)
which is of the form
(25)
with
k
=
1.
It
is written in the form
(22)
with
TI
=
P,
T2
=
Q,
(1
=
1
-
y2,
(2
=
y
+
y2.
Hence the operators
(23)
are
(29)
d
d
x1
=
(1
-
y2)-,
x2
=
(y
+
y2)-.
dY
dY
They span
L2
since
[X,
,
X2]
=
XI
+
2x2.
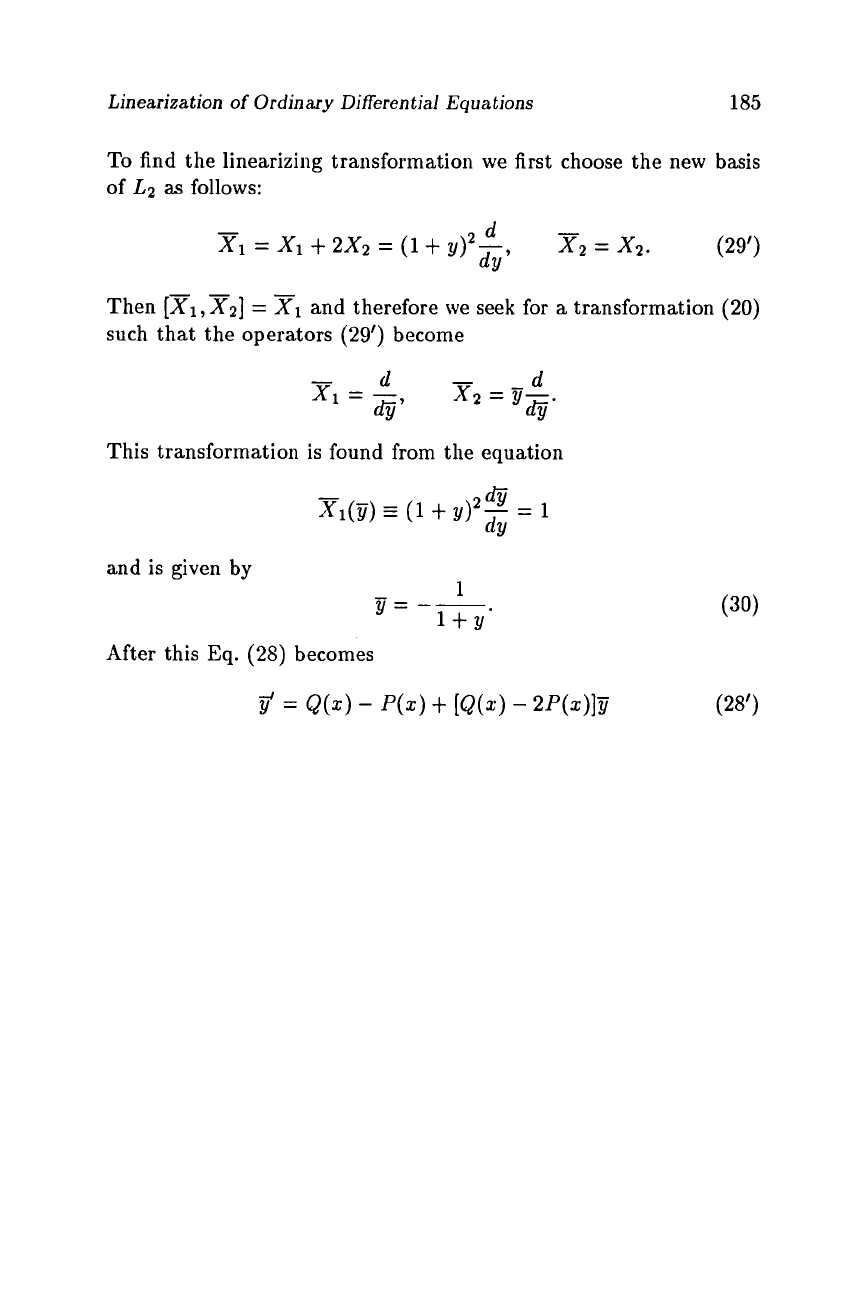
Linearization
of
Ordinary Differential Equations
185
To
find the linearizing transformation we first choose the new basis
of
L2
as
follows:
-
(29')
-
d
x1=
XI
+
2x2
=
(1
+
y)2-,
dY
x2
=
x2.
--
Then
[Xl,
X,]
=
XI and therefore we seek for
a
transformation
(20)
such
that the operators
(29')
become
This transformation is found from the equation
and
is
given by
1
After this
Eq.
(28)
becomes

186
Nail
H.
Ibragimov
Table
1.
Lie
Group
Classification
of
Second Order Equations
Group
G1
G2
G3
G8
Linearization
of
Ordinary Differential Equations
187
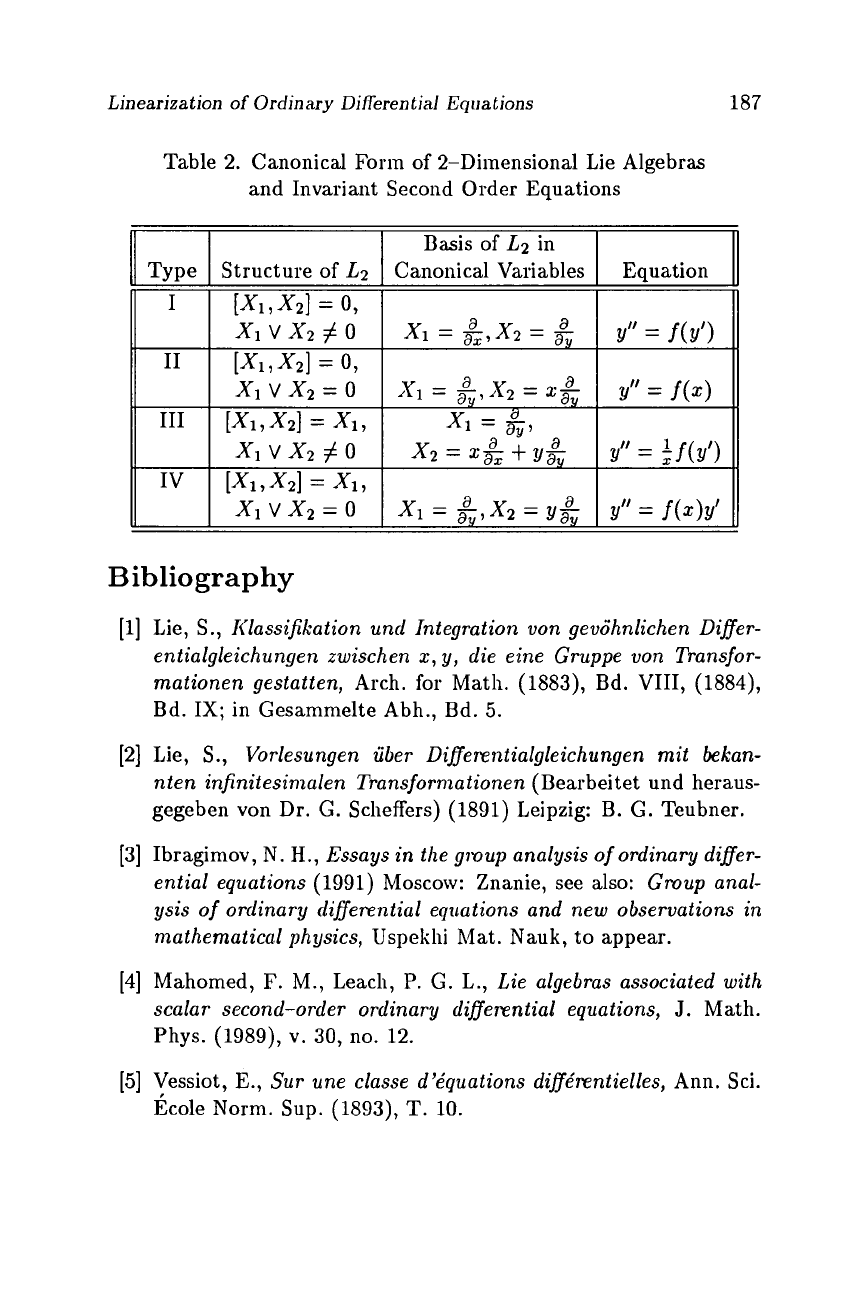
Table 2. Canonical
Form
of
2-Dimensional Lie Algebras
and Invariant Second
0
rd er Equations
Type
I
I1
I11
IV
Structure
of
L2
[Xl,
x21
=
0,
XI
v
x2
#
0
[Xl
,
x21
=
0,
x1
v
x2
=
0
[Xl,
X2]
=
x1,
x1
v
x2
#
0
[Xl,X21
=
x1,
x1
v
x2
=
0
Basis
of
L2
in
Canonical Variables
XI=
&,x2
=
-$
a
x1=
g,x2
a
=
yg
a
Equation
Ytl
=
f(Y')
Bibliography
[l]
Lie,
S.,
Klassifikation und Integration von gevo"hn1ichen Differ-
entialgleichungen zwischen x,
y,
die eine Gruppe von Transfor-
mationen gestatten,
Arch. for Math.
(1883),
Bd. VIII, (1884),
Bd.
IX;
in Gesammelte Abh., Bd.
5.
[2]
Lie,
S.,
Vorlesungen uber
Differe,ztialgleichungen
mit bekan-
nten infinitesinzalen Transfornzationen
(Bearbeitet und heraus-
gegeben von Dr.
G.
Scheffers) (1891) Leipzig: B.
G.
Teubner.
[3]
Ibragimov,
N.
H.,
Essays in the group analysis of ordinary differ-
ential equations
(1991) Moscow: Znanie, see also:
Group anal-
ysis
of
ordinary diflemntial equations and new observations in
nzathematicu.1 physics,
Uspelchi h4at. Nauk, to appear.
[4] Mahomed,
F.
M., Leach,
P.
G.
L.,
Lie algebras associated with
scalar
second-order ordinary differential equations,
J.
Math.
Phys. (1989), v. 30,
no.
12.
Ecole Norm. Sup. (1893),
T.
10.
[5]
Vessiot,
E.,
Sur une classe d'e'quations diffe'rentielles,
Ann. Sci.

188
Nail
H.
Ibragirnov
[6] Guldberg, A.,
Sur les e‘quations diffe’rentielles ordinaire qui
posstdent un systtnie fundamental d’integrules,
C.
R.
Paris
(1893),
T.
116.
[7] Lie,
S.,
Vorlesungen
2ikr
continuierliche Gruppen mit ge-
ometrischen and anderen Anwendungen,
(Bearbeitet and her-
ausgegeben von
Dr.
G.
Scheffers) (1893) Leipzig: B.
G.
Teubner.
[8] Ames,
W.
F.,
Nonlinear superposition
for
operator equations.
In
Nonlinear Equations
in
Abstract Spaces,
ed.
V.
Lakshmikan-
tham (1978) New York: Academic Press.
[9] Anderson,
R.
L.,
A nonlinear superposition principle admitted
by coupled Riccati equations
of
the projective type,
Lett.
Math.
Phys. (1989),
v.
4.
[lo]
Ibragimov,
N.
H.,
Primer on the group analysis
(1989) Moscow:
Znanie.

Expansion
of
Continuous
Spectrum Operators
in
Terms
of
Eigenprojections
Robert
M.
Kauffman
Dept.
of
Mathematics
University
of
Alabama
at
Birmingham
Birmingham,
AL
35294
1
Introduction
Eigenfunction expansions are at the hea.rt of the picture of quantum
mechanics which was developed by Dirac. The idea is to expand
states which change with time
as
they evolve under the Schrodinger
equation
dQ/dt
=
iH!P in terms of those which do not, in the sense
that they give the same expectation values
for
all
observables. How-
ever, in quantum mechanics, observables in the physical sense cor-
respond to operators in
a
Hilbert space. The operator which maps
the initial condition
9(0)
for the Schrodinger equation to the solu-
tion
Q(t)
at time t is denoted by
eiHt.
Since this is the fundamental
operator of quantum mechanics, it makes sense to expand it in terms
of simple operators; the most natural
way
of doing this is to expand
in terms of operators
of
the form
eiA'PA,
where
PA
is
a
projection
onto
a
one-dimensional space of eigenfunctions with eigenvalue
A.
This turns the operator exp(iHt) of time evolution into
a
diagonal
matrix; unfortunately, it in general has uncountably many entries.
For
many physical problems, such
as
those connected with scattering
DiRerentid Equations
with
Applications to Mathematical
Copyright
@
1993 by Academic Press, Inc.
All rights
of
reproduction in any
form
reserved.
Physics
ISBN
0-12-056740-7
189
