Accardi L., Freudenberg W., Obya M. (Eds.) Quantum Bio-informatics IV: From Quantum Information to Bio-informatics

This page intentionally left blankThis page intentionally left blank

Quantum

Bio-Informatics

IV

eds. L.

Accardi,

W.

Freudenberg

and

M.

Ohya

World

Scientific

Publishing

Co.

(pp.

41-50)

ON

A

GENERAL

FORM

OF

TIME

OPERATORS

OF

A

HAMILTONIAN

WITH

PURELY

DISCRETE

SPECTRUM

A. ARAI*

Department

of

Mathematics, Hokkaido University

Sapporo, Hokkaido

060-0810, Japan

* E-mail: arai@math.sci.hokudai.ac.jp

We review

some

results

on

determining

a

general

form

of

time

operators

of

a

Hamiltonian

with

purely

discrete

spectrum.

Keywords:

Canonical

commutation

relations;

Hamiltonian;

Spectrum;

Time

operator.

1.

Introduction

Let

1i

be

a complex

Hilbert

space

and

H

be

a self-adjoint

operator

on

1i.

A

symmetric

operator

T

on

1i

is called a

time

operator

of

H if

there

is a

subspace

V

=f.

{O}

(not

necessarily dense

in

1i) such

that

V c

D(T

H)

n

D(HT)-for

a linear

operator

A

on

1i,

D(A)

denotes

the

domain

of

A-

and

the

canonical

commutation

relation

(CCR)

on

V

[T,H]'lj; =

i'lj;,

V'lj;

E V (1.1)

holds, where

[T,

H]

:=

T H -

HT

and

i is

the

imaginary

unit.

The

name

"time

operator"

comes from

the

physical

context

where H is

the

Hamil-

tonian

of

a

quantum

system.

But

we use

this

terminology

in

the

general

mathematical

context

too.

We call

the

subspace

V a

CCR-domain

for

the

pair

(T, H).

From

purely

mathematical

point

of view,

the

pair

(T,

H)

is a

(not

nec-

essarily self-adjoint)

representation

of

the

CCR

with

one

degree

of

freedom.

A

study

from

this

point

of

view

has

been

made

by Dorfmeister 9

in

the

case

where

H is

bounded

and

purely

absolutely continuous.

There

is a

stronger

version

of

time

operator:

a

symmetric

operator

T

on

1i

is called a strong

time

operator

of

H if, for all t E

IR,

e-

itH

D(T)

c

D(T)

and

the

weak Weyl relation

Te-itH'lj; =

e-

itH

(T

+

t)'lj;,

t E

IR,

'lj;

E

D(T)

41

42

holds.

It

is easy

to

see

that

a

strong

time

operator

T

with

D(HT)

n

D(T

H)

#

{O}

is a

time

operator.

But

the converse is

not

true. As for

strong

time

operators,

there

have been studies 1-

6,

11-13.

One

of

the

fundamental

properties

of H which

has

a

strong

time

operator

is

that

H is

purely absolutely continuous. Hence

a self-adjoint operator with eigenvalues

cannot have a

strong

time

operator.

In

this

paper,

we

consider

time

operators

of

a self-adjoint

operator

H

whose

spectrum

consists

of

only discrete eigenvalues

and

their

possible ac-

cumulation

points.

It

follows from

the

fact mentioned

in

the

last sentence

of

the

preceding

paragraph

that

such

time

operators

cannot

be

strong

time

operators

of

H.

This

kind of

time

operators

was proposed by

Galapon

10

first (see also 8

and

references

therein

for specific examples).

Then

de-

tailed,

mathematically

rigorous analysis

on

the

Galapon

time

operator

has

been

made

by Arai-Matsuzawa

7.

It

is interesting

to

investigate

to

what

extent

a general form of such

time

operators

can

be

determined

under

a

suitable

condition.

In

the

5,

some results

on

this

aspect

were established. Below is a

summary

of

them.

2.

Time

Operators

of

a

Hamiltonian

with

Discrete

Eigenvalues

(I)

We

denote

the

inner

product

and

the

norm

of

1i

by (.,.) (linear in

the

second variable)

and

II

.

II

respectively.

Let

N =

{I,

2,

3,

...

}

be

the

set

of

natural

numbers. A basic

assumption

in

the

present

paper

is as follows:

Hypothesis

(H)

The

self-adjoint

operator

H

has

a complete

orthonormal

system

(CONS) {enaln E

N,a

=

1,···

,Mn}

C

1i

of

eigenvectors

with

discrete eigenvalues

{En

}nEN

(En

# Ern, n #

m,

n,

mEN):

(2.1)

(e

na

, e

m

(3)

=

DnmDa(3,

a =

1,···

,M

n

,

f3

=

1,···

,Mm,(2.2)

where

Dab

is

the

Kronecker

delta

and

Mn E N is

the

multiplicity of

eigenvalue

En,

obeying

(2.3)

We set

(2.4)

43

Hypothesis

(H) implies

that

the

spectrum

of

H,

denoted

CJ(H),

is given

by

(2.5)

where

the

right

hand

side is

the

closure

of

the

set

{En}

~=1'

and

CJ(

H)

\

{En}~=l

contains

no

eigenvalues

of

H.

For a

subset

5

of

H,

we

denote

by

1.h.(5)

the

linear

hull of

5,

i.e.,

the

subspace

algebraically

spanned

by

the

vectors

in

5.

Since

the

set

{enaln E N, a =

1""

,Mn}

is a

CONS

ofH,

the

subspace

Do

:=

1.h.(

{en<>ln

E

N,

a =

1""

,Mn})

is dense

in

H.

2.1.

A

general

class

of

time

operators

of

H

Suppose

that,

for some no

EN,

CXJ

1

L

-2

<00.

En

n=no

(2.6)

Then,

in

the

same

way as

in

7,

one

can

define a linear

operator

To

as

follows:

D(To)

:=

Do,

(2.7)

T, n/,

._

.

~ ~

(~

(e

rru

:

n

'Ij;)

)

O'f/

.-

Z L L L E _ E

eno:,

n=lo:=l

min

n m

'Ij;

E D(To).

(2.8)

It

is

easy

to

see

that

To

is a

symmetric

operator.

Remark

2.1.

Under

condition

(2.6), H is

unbounded,

since (2.6) implies

that

IEnl

-+

00

as n

-+

00.

Remark

2.2.

In

10

and

7,

it

is

assumed

that

H is

bounded

below

with

o < En < En+1' n E

N.

But,

in

the

present

paper,

we

do

not

assume

the

semi-boundedness

(boundedness

below

or

boundedness

above).

We

introduce

a subspace:

EM

:=

1.h.(

{en<>

-

emo:ln,

mEN,

a =

1"

..

,M}).

(2.9)

This

subspace

is

not

necessarily dense

in

H:

Lemma

2.1.

The

subspace

EM

is

dense

in

H

if

and

only

if

M =

Mn

for

all n E N.

44

The

shows

that

To

is a

time

operator

of H

with

EM

being

a

CCR-domain

for

(To

, H):

Theorem

2.1.

Und

er Hypothesis (H) with (2.6),

EM

c

D(ToH)nD(HTo)

and

(2.10)

Remark

2.3.

It

is easy

to

see

th

at

2:

%f

n

EUIE

k

- Enl

2

=

00

. Hence

it

follows

that

Toe

na

tf-

D(H).

Th

erefore Vo is not a

CCR-domain

for

(To

, H).

We

perturbation

of

To

by a

symmetric

oper

a

tor

T1

such

that

To

+

T1

is a

time

operator

of H.

Let

a:=

{a

n

(a,,8) ln E

N,a,,8

=

1,···

,Mn}

be

a set

of

complex num-

bers

su

ch

that

an(a,

,8)

* =

an

(,8,

a), n E

N,

a,,8 =

1,···

, Mn,

(2

.

11)

where a

n

(a,,8

)*

is

th

e complex

conjugat

e of an(a,,B).

Then

we define a

linear

operator

T1

(a)

on

1-{

as follows:

(2

.12)

(2

.13)

It

follows

that

with

Mn

T1

(a)e

na

= L

an

(,8,

a)e

n

,6, \/n E

N,

a =

1,···

, Mn- (2.14)

,6=

1

It

is easy

to

see

that

T1

(a)

is a

symmetric

operator.

Using (2.14),

we

see

that

Vo C D(HT1(a)) n D(T1(a)H)

and

T1(a)H

1jJ

=

HT

1

(a)1jJ,

\/1jJ

E Vo·

By

this

fact

and

Theorem

2.1 we obt

ain

th

e

45

Theorem

2.2.

Assume

Hypotheis (H) and (2.6). Let a

be

as

above and

T(a)

:=

To

+

Tl(a).

(2.15)

Then

T(a)

is a time operator

of

H with

EM

being a

CCR-domain

for

(T(a),

H).

Thus

(2.6) gives a sufficient condition for H

with

Hypothesis (H)

to

have

time

operators

of

the

form (2.15).

Remark

2.4.

Boundedness

or

unboundedness of

T(a)

can

be

investigated

in

the

same

way as in

7.

But

, here, we do

not

go into

the

details.

2.2.

Necessary

condition

for

H

to have

time

operators

and

the

general

form

of

them

We

to

have

time

operators

and

their

general form.

Theorem

2.3.

Let H

be

a self-adjoint operator satisfying Hypothesis (H)

and T

be

a time operator

of

H such that

EM

is a

CCR-domain

for (T,

H)

and e

na

E

D(T)

,

\:In

E

N,

a = 1" . . ,

M.

Then H is unbounded and there

is an no

E N such that (2.6) holds.

Moreover, the following (i) and (ii) hold:

(i) Let M

= M

n

,

\:In

E N. Then, for all

'l/J

E

D(T),

(2.16)

and

(2.17)

In

particular, one has

T

=

T(a(T))

=

To

+

Tl(a(T))

on V

o

,

(2.18)

where

46

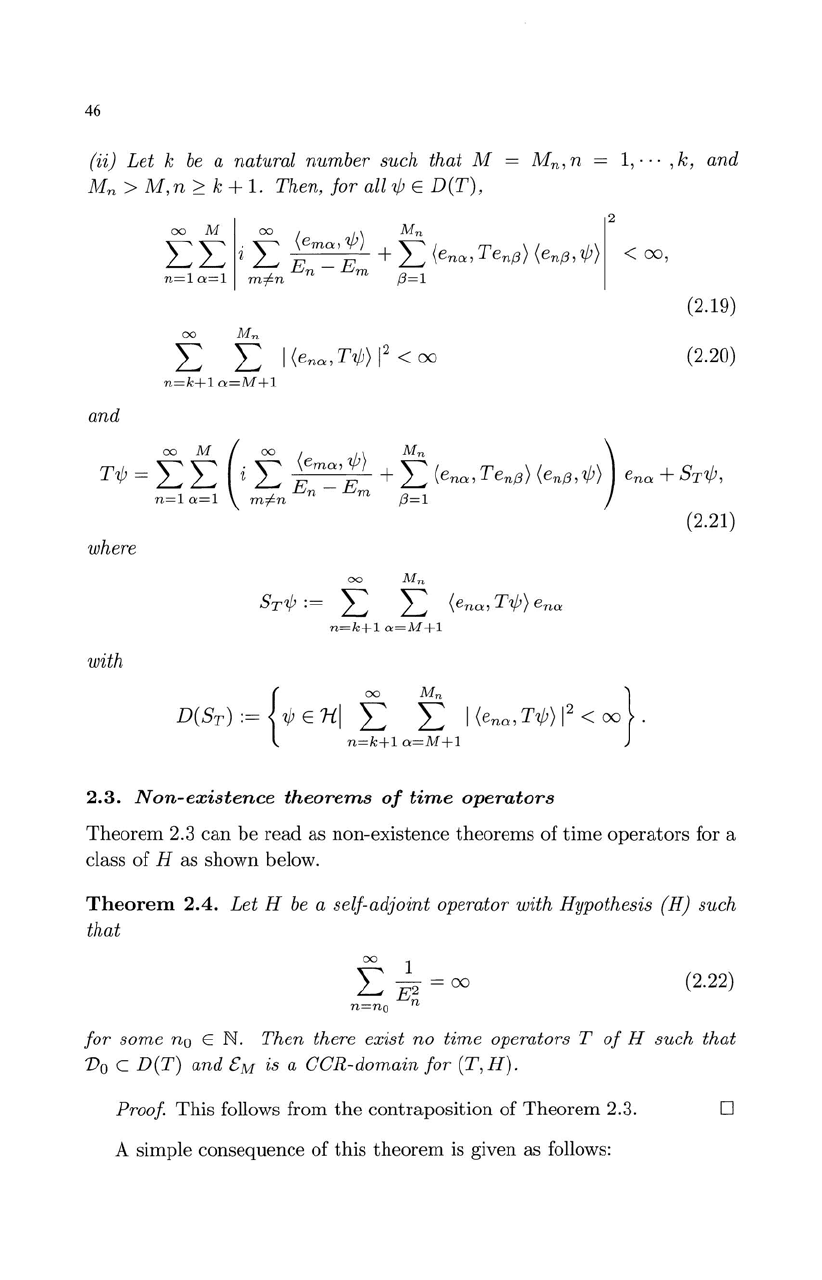
(ii) Let k

be

a natural number such that M

Mn

> M, n

;::::

k + 1. Then, for all

'Ij;

E

D(T),

M

n

,

n =

1,···

,k,

and

2

(2.19)

co

Mn

L L 1 (ena,T'Ij;)

12

<

00

(2.20)

n=k+l

a=M+l

and

where

co

Mn

ST'Ij;:= L L

(e

na

,

T'Ij;)

e

na

n=k+l

a=M+l

with

2.3.

Non-existence

theorems

of

time

operators

Theorem

2.3

can

be

read

as

non-existence

theorems

of

time

operators

for a

class

of

H as

shown

below.

Theorem

2.4.

Let H

be

a self-adjoint operator with Hypothesis (H) such

that

co

1

L

-=00

n=no

E;;

(2.22)

for some no

E N. Then there exist no time operators T

of

H such that

Vo c

D(T)

and

EM

is a

CCR-domain

for (T, H).

Proof.

This

follows from

the

contraposition

of

Theorem

2.3. D

A simple consequence

of

this

theorem

is given

as

follows:

47

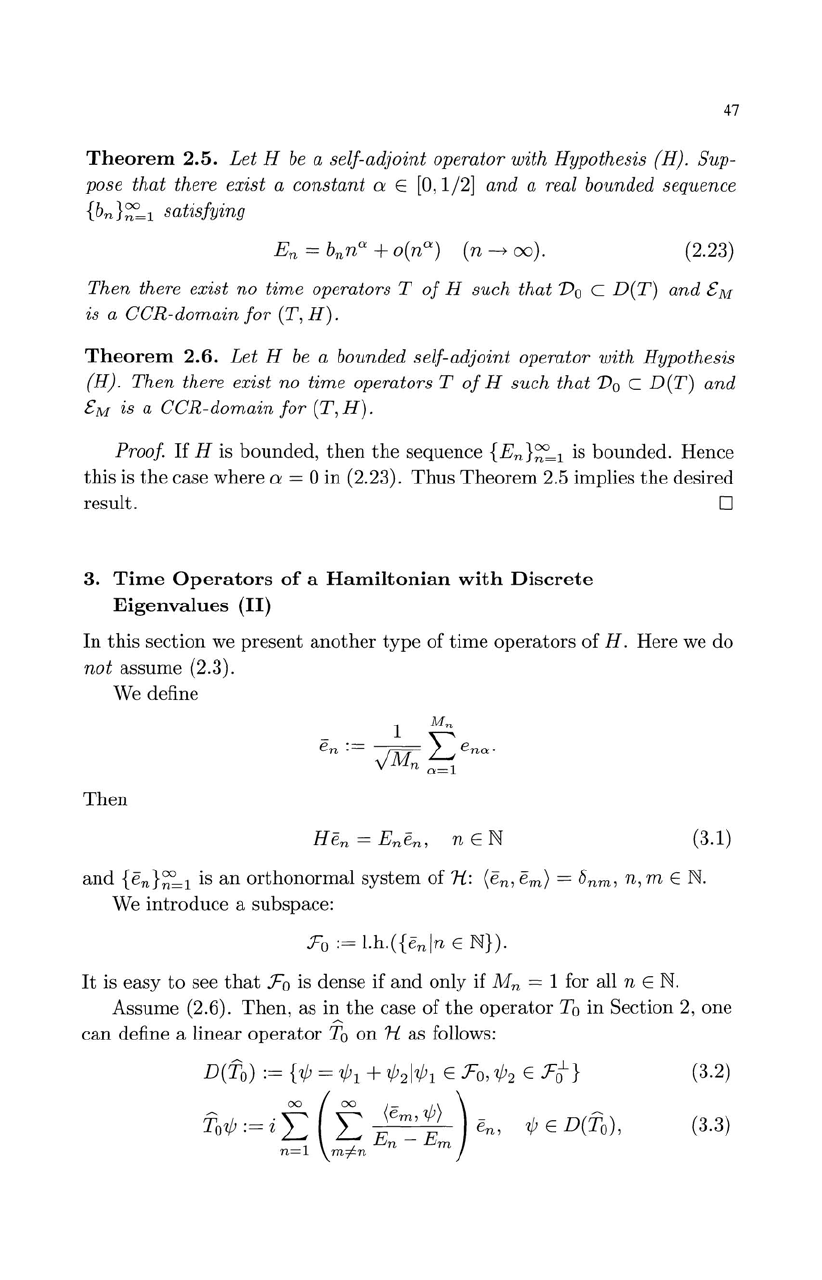
Theorem

2.5.

Let

H

be

a

self-adjoint

operator with Hypothesis (H). Sup-

pose

that

there

exist

a

constant

a E [0,1/2]

and

a real bounded sequence

{bn}~=l

satisfying

(2.23)

Then

there

exist

no

time

operators T

of

H

such

that

Vo

C

D(T)

and

EM

is a

CCR-domain

for

(T, H).

Theorem

2.6.

Let

H

be

a bounded

self-adjoint

operator

with

Hypothesis

(H).

Then

there

exist

no

time

operators T

of

H

such

that

Vo

C

D(T)

and

EM

is a

CCR-domain

for

(T, H).

Proof

If

H is

bounded,

then

the

sequence

{En}~=l

is

bounded.

Hence

this

is

the

case where a = 0 in (2.23).

Thus

Theorem

2.5 implies

the

desired

rewU. D

3.

Time

Operators

of

a

Hamiltonian

with

Discrete

Eigenvalues

(II)

In

this

section we

present

another

type

of

time

operators

of

H.

Here we do

not

assume (2.3).

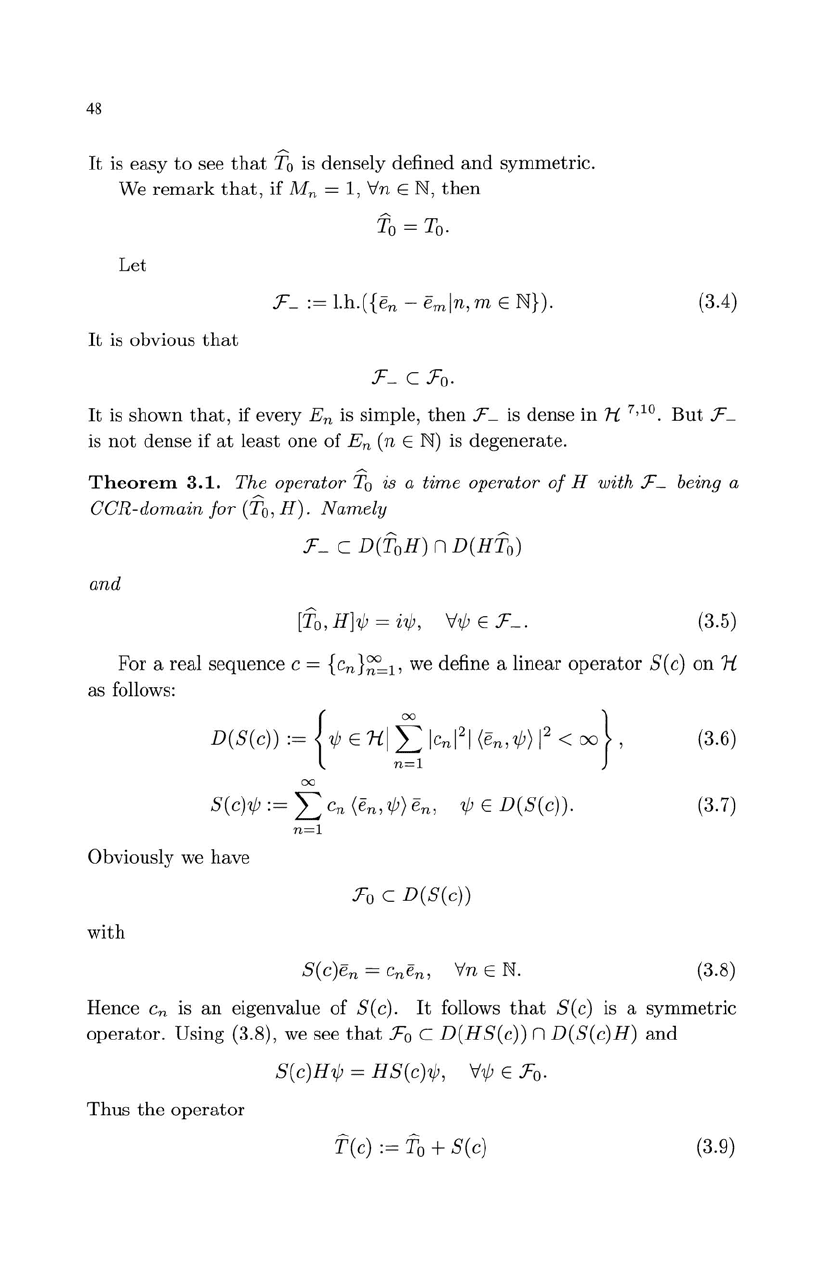
We define

Then

(3.1)

and

{en}~=l

is

an

orthonormal

system

of

H:

(en, em) = D

nm

,

n,

mEN.

We

introduce

a subspace:

:Fo:= l.h.({enin

EN}).

It

is easy

to

see

that

:Fo

is dense if

and

only

if

Mn

= 1 for all n E

N.

Assume (2.6).

Then,

as in

the

case

of

the

operator

To

in

Section

2,

one

can

define a linear

operator

To

on

H as follows:

~

D(To)

:=

{'lfJ

=

'lfJ

1

+

'lfJ2i'lfJl

E

:F

o

,

'lfJ2

E

:Fo

} (3.2)

To'lfJ

:=

if

(f

i~~

im)

en,

'lfJ

E

D(To),

(3.3)

n=l

mien

48

It

is

easy

to

see

that

To

is densely defined

and

symmetric.

We

remark

that,

if

Mn

= 1,

\:In

E

N,

then

To

= To·

Let

(3.4)

It

is obvious

that

It

is shown

that,

if

every

En is simple,

then

F_

is dense in H

7,lO.

But

F_

is

not

dense

if

at

least

one of En

(n

E N) is

degenerate.

Theorem

3.1.

The operator

To

is a time operator

of

H with

F_

being a

CCR-domain for

(To,

H).

Namely

F_

C D(ToH) n D(HTo)

and

(3.5)

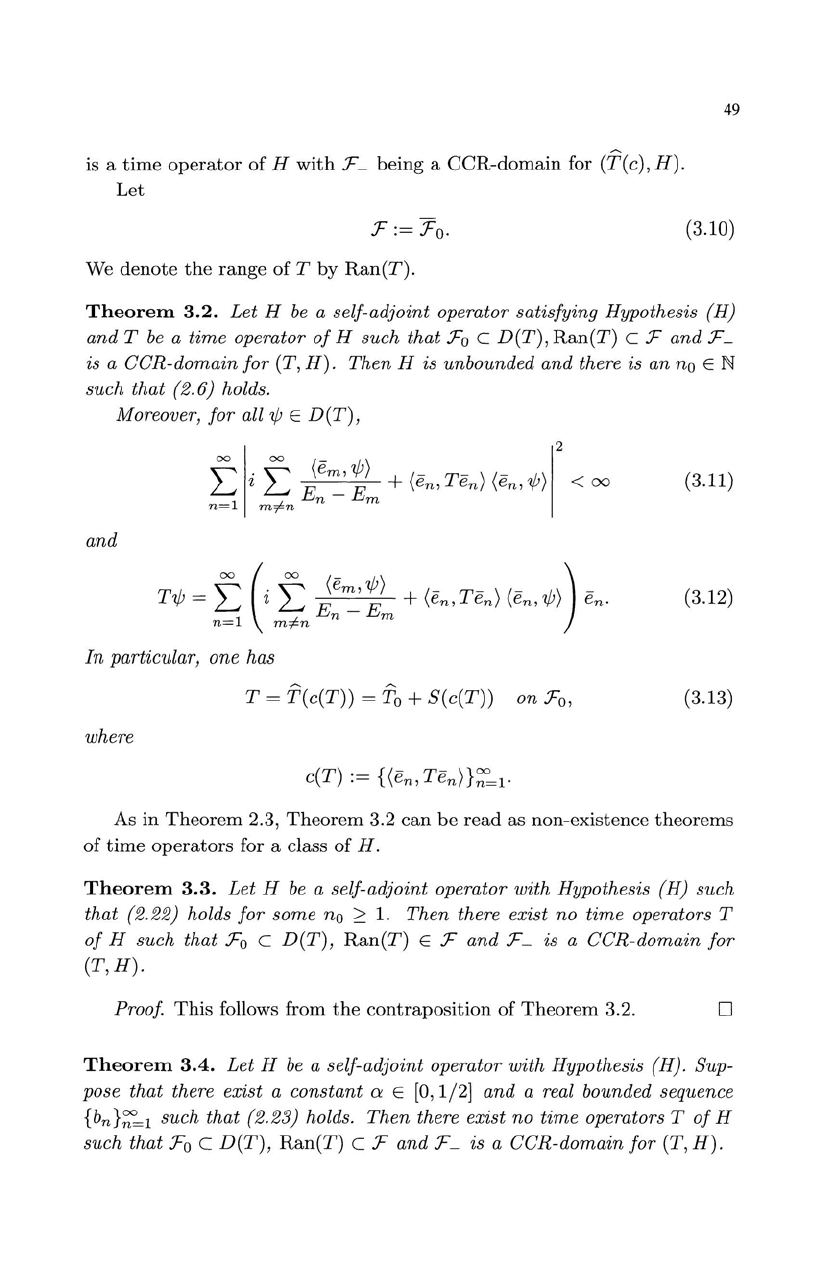
For a real sequence e = {en}

~=l'

we define a linear

operator

S

(e)

on

H

as follows:

00

(3.7)

n=l

Obviously we have

Fa

C D(S(e))

with

(3.8)

Hence

en

is

an

eigenvalue

of

S(c).

It

follows

that

S(e) is a

symmetric

operator.

Using (3.8), we see

that

Fa

C

D(HS(c))

n

D(S(e)H)

and

S(c)H'I/J

=

HS(e)'I/J,

\:I'I/J

E

Fa·

Thus the

operator

T(e)

:=

To

+ S(e)

(3.9)

49

is a

time

operator

of

H

with

F_

being a

CCR-domain

for (T(c) , H).

Let

F:=F

o

·

(3.10)

We

denote

the

range

of

T by

Ran(T).

Theorem

3.2.

Let H

be

a self-adjoint operator satisfying Hypothesis (H)

and T

be

a time operator

of

H such that Fo C

D(T),

Ran(T)

C F and

F_

is a CCR-domain for (T,

H).

Then H is unbounded and there is an no E

1"1

such that (2.6) holds.

Moreover, for

all7jJ

E

D(T),

2

<00

(3.11)

and

(3.12)

In

particular, one has

T

=

T(c(T))

=

To

+

S(c(T))

on F

o

, (3.13)

where

As

in

Theorem

2.3,

Theorem

3.2

can

be

read

as non-existence theorems

of

time

operators

for a class

of

H.

Theorem

3.3.

Let

H

be

a self-adjoint operator with Hypothesis (H) such

that

(2.22) holds for some no

;:::

1.

Then there exist no

time

operators T

of

H such that Fo C

D(T),

Ran(T)

E F and

F_

is a CCR-domain for

(T,H).

Froof

This

follows from

the

contraposition

of

Theorem

3.2. D

Theorem

3.4.

Let H

be

a self-adjoint operator with Hypothesis (H). Sup-

pose that there exist a constant a E [0,1/2] and a real bounded sequence

{bn}~=l

such that (2.23) holds. Then there exist no time operators T

of

H

such that

Fo

C

D(T),

Ran(T)

C F and

F_

is a

CCR-domain

for (T,

H).

Accardi L., Freudenberg W., Obya M. (Eds.) Quantum Bio-informatics IV: From Quantum Information to Bio-informatics

Подождите немного. Документ загружается.