Ablowitz M.A. Nonlinear Dispersive Waves: Asymptotic Analysis and Solitons

Подождите немного. Документ загружается.

6.8 Multidimensional water waves 165

Pulses with energy below E

exist globally (Weinstein, 1983). In many cases

when E > E

, even by a small amount, blow-up occurs. In the supercritical

case, the blow-up solution has a similarity form

ψ ∼

α



β



iλ ln t



with suitable constants α and β. But for the critical case the structure is

ψ ∼

√

L(t



)

√





√

L(t



)

√





iφ(t



)

where t



= t

− t, L(t) ∼ ln(ln(1/t



)) as t → t

is the blow-up or collapse time.

The stationary states, collapse and properties of the BR equations (6.59)

(sometimes referred to as Davey–Stewartson-type equations) as well as simi-

lar ones that arise in nonlinear optics were studied in detail by Papanicolaou

et al. (1994) and Ablowitz et al. (2005). More speciﬁcally, the equations

studied were

ΔU + |U|

U − ρUV

= 0, and

+ νV

= (|U|

)

The case ρ<0 corresponds to water waves, cf. Ablowitz and Segur (1979,

1981), and the case ρ>0 corresponds to χ

(2)

nonlinear optics (Ablowitz et al.,

1997, 2001a; Crasovan et al., 2003).

When ν>0 collapse is possible. That there is a singularity in ﬁnite time

can be shown by the virial theorem. In analogy with the Townes mode for

NLS, there are stationary states (ground states) that satisfy U = F(x, y)e

iμz

V = G(x, y)

−μF +

ΔF + |F|

F − ρFG

= 0

+ νG

= (|F|

)

The stationary states F, G can be obtained numerically (Ablowitz et al., 2005).

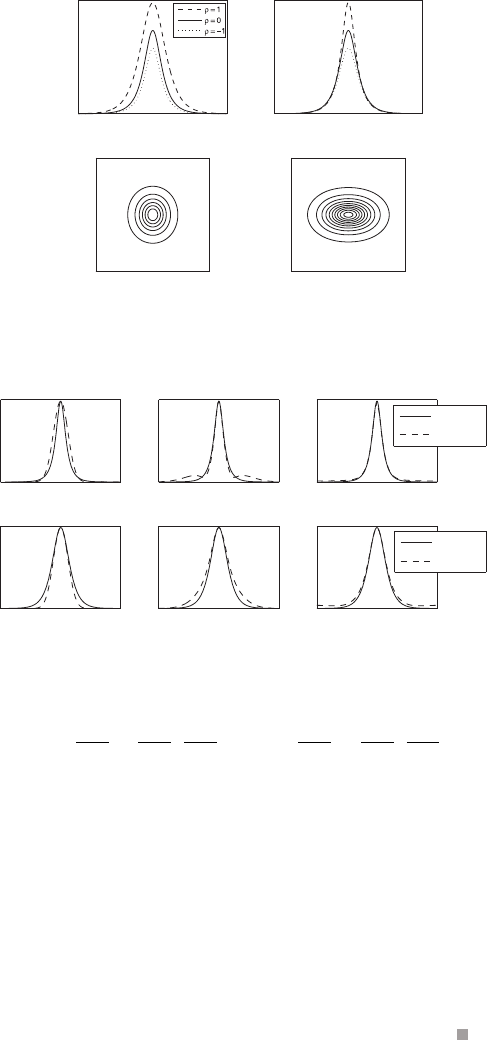
In Figure 6.3 slices of the modes along the y = 0, x = 0 axes, respectively, are

given for diﬀerent values of ρ. In Figure 6.3(c) and (d) contour plots of some

typical modes F are shown. One can see that the modes are elliptical in nature

when ρ  0.

Quasi-self-similar collapse

Papanicolaou et al. (1994) showed that as collapse occurs, i.e., as z → z

, then

U,V have a quasi-self-similar structure:

166 Nonlinear Schr¨odinger models and water waves

−5 5

|F(x,y = 0)|

(a)

−5 5

|F(x = 0,y)|

(b)

(c)

−3 3

−3

(d)

−3 3

−3

Figure 6.3 F(x, y); ν = 0.5; top (a), (b): “slices”; bottom: contour plots; (c)

ρ = −1, (d)ρ = 1.

−6 6

z = 0 L = 0.96

−6 6

z = 0.5 L = 0.56

−6 6

z = 0.94 L =0.22

F(x,0)

L|u(Lx,0)|

−6 6

F(0,y)

L|u(0,Ly)|

Figure 6.4 Wave collapse to the stationary state as z → z

U ∼

L(z)



L(z)



, V ∼

L(z)



L(z)



where L → 0. Further, one ﬁnds from direct simulation (Ablowitz

et al., 2005) that the stationary modes are good approximations of

collapse proﬁles. By taking typical initial conditions (of Gaussian

type) that collapse, we ﬁnd that the pulse structure is well approx-

imated by the stationary modes in the neighborhood of the collapse

point, where L(z) = F(0, 0)/U(0, 0, z) →0. In Figure 6.4 we compare

L(z)|U(Lx, Ly)| with F(x, y) where L(z) = F(0, 0)/U(0, 0, z). As collapse

occurs, L(z) →0 for typical values (ν, ρ) = (0.5, 1). In Figure 6.4 we pro-

vide snapshots of the solution compared to the stationary state F as the wave

Exercises 167

collapse begins to occur. Along the y = 0 axis (top) and the x = 0 axis (bottom)

at the edges of the solution, radiation is seen. But the behavior at the center of

the wave indicates that collapse is well approximated by the stationary state.

Finally, we mention that a similar, but more complicated blow-up or wave

collapse phenomenon occurs for the generalized KdV equation

+ u

xxx

= 0

when p ≥ 4(Ablowitz and Segur, 1981; Merle, 2001; Angulo et al., 2002).

Exercises

6.1 Derive the NLS equation from the following nonlinear equations.

(a) u

− u

+ sinu = 0 (sine–Gordon).

(b) u

− u

+ u

xxxx

+ (uu

)

= 0 (Boussinesq-type).

+ u

xxx

= 0 (mKdV) .

6.2 Given the equation

− u

xxxx

+ |u|

2(n+1)

u = 0

with n ≥ 1 an integer, substitute the mutli-scale and quasi-

monochromatic assumption:

u ∼ μe

(ikx−ωt)

A(

x,

t),

where μ, 

, and 

are all asymptotically small parameters, into the

equation. Choose a maximal balance between the small parameters to

ﬁnd a nonlinear Schr

odinger-type wave equation for the slowly varying

envelope A.

6.3 From the water wave equations with surface tension included, derive the

dispersion relation

= gκ tanh(κh)(1 +

T ),κ

= k

+ l

T =

ρg

in ﬁnite depth water waves with surface tension, where T

is the surface

tension coeﬃcient.

6.4 Beginning with the KP equation

∂

+ 6uu

+ u

xxx

) + 3σu

= 0,σ= ±1

derive the integrable Davey–Stewartson equation by employing the

slowly varying quasi-monochromatic wave expansion. Hint: see

Ablowitz et al. (1990).

168 Nonlinear Schr¨odinger models and water waves

6.5 Suppose we are given a “modiﬁed KP” equation

∂



n + 1

2(n+1)

)

+ u

xxx



+ 3σu

= 0,σ= ±1

where n ≥ 1, an integer. Following a similar analysis as in the previous

exercise, derive a “modiﬁed” Davey–Stewartson equation by employing

the slowly varying quasi-monochromatic wave expansion.

6.6 Suppose we are given the “damped NLS” equation

+ u

+ 2|u|

u = −iγu

where 0 <γ 1. Use the integral relation involving mass (power)



|u|

dx to derive an approximate evolution equation for the slowly

varying soliton amplitude η where the unperturbed soliton is given by

u = ηsech

(

x − x

)

iη

t+iθ

Hint: see Ablowitz and Segur (1981). Various perturbation problems

involving related NLS-type equations are also discussed in Chapter 10.

6.7 Discuss the stability associated with the special solution

u = ae

i(kx−(k

−2σ|a|

)t)

where k,σare constant, associated with the NLS equation

+ u

+ 2σ|u|

u = 0

on the inﬁnite interval.

6.8 Given the two-dimensional NLS equation

+ΔA + |A|

A = 0, x ∈ R

and associated “Townes” mode A = f (r)e

iλt

, where r

= x

+ y

,show

that the “virial” equation reduces to

= 0

where V(t) =



+ y

)|A|

dx. Hint: see the appendix in Ablowitz et al.

(2005).

Nonlinear Schr¨odinger models in nonlinear optics

An important and rich area of application of nonlinear wave propagation is the

ﬁeld of nonlinear optics. Asymptotic methods play an important role in this

ﬁeld. For example, since the scales are so disparate in Maxwell’s equations,

long-distance transmission in ﬁber optic communications depends critically on

asymptotic models. Hence the nonlinear Schr

odinger (NLS) equation is cen-

tral for understanding phenomena and detailed descriptions of the dynamics.

In this chapter we will outline the derivation of the NLS equation for electro-

magnetic wave propagation in bulk optical media. We also brieﬂy discuss how

the NLS equation arises as a model of spin waves in magnetic media.

7.1 Maxwell equations

We begin by considering Maxwell’s equations for electromagnetic waves with

no source charges or currents, cf. Landau et al. (1984) and Jackson (1998)

∇ × H =

∂D

∂t

(7.1a)

∇ × E = −

∂B

∂t

(7.1b)

∇ · D = 0 (7.1c)

∇ · B = 0 (7.1d)

where H is the magnetic ﬁeld, E is the electromagnetic ﬁeld, D is the

electromagnetic displacement and B is the magnetic induction.

We ﬁrst consider non-magnetic media so there is no magnetization term in

B. The magnetic induction B and magnetic ﬁeld H are then related by

B = μ

H. (7.2)

169

170 Nonlinear Schr¨odinger models in nonlinear optics

The constant μ

is the magnetic permeability of free space. We allow for

induced polarization of the media giving rise to the following relation

D = 

(E + P), (7.3)

where 

is the electric permittivity of free space, a constant. When we apply

an electric ﬁeld E to an ideal dielectric material a response of the material can

occur and the material is said to become polarized. Typically in these materials

the electrons are tightly bound to the nucleus and a displacement of these

electrons occurs. The macroscopic eﬀect (summing over all displacements)

yields the induced polarization P.

Since we are working with non-magnetic media, we can reduce Maxwell’s

equations to those involving only the electromagnetic ﬁeld E and the polar-

ization P. To that end, we take the curl of (7.1b) and use (7.2) and (7.1a)

to ﬁnd

∇ × (∇ × E) = −

∂

∂t

(∇ × B)

= −μ

∂

∂t

(∇ × H)

= −μ

∂

∂t

The vector identity

∇ × (∇ × E) = ∇(∇ · E) −∇

E, (7.4)

is useful here. With this, along with relation (7.3), we ﬁnd the following

equations for the electromagnetic ﬁeld

∇

E − ∇(∇ · E) =

∂

∂t

(E + P(E))

∇ · (E + P(E)) = 0,

(7.5)

where the constant c



is the square of the speed of light in a vacuum.

Notice that we have made explicit the connection between the polarization P

and the electromagnetic ﬁeld E.

Before we investigate polarizable, non-magnetic media in detail, let us also

write down the dual equation for magnetic media without polarization (P = 0)

satisfying

B = μ

(H + M(H)) (7.6)

where M is called the magnetization. We see that the magnetization vector

M plays a similar role to that of the polarization P, i.e., we are assuming

7.2 Polarization 171

that M = M(H). Namely, we assume that the magnetization is related to

the magnetic ﬁeld. The magnetization can be permanent for ferromagnetic

materials (permanent magnets such as iron). Taking the curl of (7.1a) then

for non-polarized media using D = 

E gives

∇ × (∇ × H) =

∂

∂t

(∇ × D)

= 

∂

∂t

(∇ × E)

= −

∂

∂t

Using (7.4) and (7.6)gives

∇

H − ∇(∇ · H) =

∂

∂t

(H + M(H))

∇ · (H + M(H)) = 0.

Later, in Section 7.4 we will discuss one way M can be coupled to H.

7.2 Polarization

In homogeneous, non-magnetic media, matter responds to intense electromag-

netic ﬁelds in a nonlinear manner. In order to model this, we use a well-known

relationship between the polarization vector P and the electromagnetic ﬁeld E

that is a good approximation to a wide class of physically relevant media:

P(E) =



(1)

∗ E +



(2)

∗ EE +



(3)

∗ EEE. (7.7)

The above equation involves tensors, so the operation ∗ is a special type of

convolution that will be deﬁned below.

For notational purposes, we will write the polarization vector as follows:

P =

⎛

⎜

⎝

⎞

⎟

⎠

≡

⎛

⎜

⎝

⎞

⎟

⎠

Notice that we can break up (7.7) into a linear and nonlinear part

P = P

+ P

In glass, and hence ﬁber optics, the quadratic term χ

(2)

is zero. This is cubically

nonlinear and is a so-called “centro-symmetric material”, cf. Agrawal (2001),

Boyd (2003) and also Ablowitz et al. (1997, 2001a) and included references

172 Nonlinear Schr¨odinger models in nonlinear optics

for more information. We write the linear and nonlinear components of the

polarization vector, and explicitly deﬁne the convolution mentioned above, as

follows:

L,i

= (χ

(1)

∗ E)



j=1



∞

−∞

(1)

(t − τ)E

(τ) dτ (7.8)

NL,i



j,k,l



∞

−∞

(3)

ijkl

(t − τ

, t−τ

, t − τ

(τ

) dτ

dτ

. (7.9)

In (7.9), the sums are over the indices {1, 2, 3} and the integration is over

all of R

. The matrix χ

(1)

is called the linear susceptibility and the tensor

(3)

is the third-order susceptibility. If the material is “isotropic”, χ

(1)

= 0,

i  j (exhibiting properties with the same values when measured along axes in

all directions), then the matrix χ

(1)

is diagonal. We will also usually identify

subscripts i = 1, 2, 3asi = x, y, z. In cubically nonlinear or “Kerr” materials

(such as glass), it turns out that the only important terms in the χ

(3)

tensor

correspond to χ

(3)

xxxx

, which is equal to χ

(3)

yyyy

and χ

(3)

zzzz

From now on, we will suppress the superscript in χ when context makes

the choice clear. For example χ

(1)

= χ

and χ

(3)

xxxx

= χ

xxxx

follow due to the

number of entries in the subscript.

We can now pose the problem of determining the electromagnetic ﬁeld E in

Kerr media as solving (7.5) subject to (7.8) and (7.9). Consider the asymptotic

expansion

E = εE

(1)

+ ε

(2)

+ ε

(3)

+ ···, |ε|1. (7.10)

Then, to leading order, we assume that the electromagnetic ﬁeld is initially

polarized along the x-axis; it propagates along the z-axis, and for simplicity

we assume no transverse y variations. So

(1)

⎛

⎜

⎝

(1)

⎞

⎟

⎠

, E

(1)

= A(X, Z, T)e

iθ

+ c.c., (7.11)

where θ = kz − ωt and the amplitude A isassumedtobeslowlyvaryinginthe

x, z, and t directions:

X = εx, Z = εz, T = εt.

In order to simplify the calculations, we assume E and hence A are independent

of y (i.e., Y = εy). Derivatives are replaced as follows

7.2 Polarization 173

∂

= ε∂

∂

= −ω∂

+ ε∂

∂

= k∂

+ ε∂

(7.12)

Substituting in the asymptotic expansion for E we note the important

simpliﬁcation that the assumption of slow variation has on the polarization P:

L,x



j=1



∞

−∞

(1)

(t − τ)E

(τ) dτ

= ε



∞

−∞

(t − τ)



A(X, Z, T )e

i(kz−ωτ)

+ c.c.



dτ + O(ε

)

= ε



∞

−∞

(t − τ)e

iω(t−τ)



A(X, Z, T )e

i(kz−ωt)

+ c.c.



dτ + O(ε

Now make the substitution t −τ = u to get

L,x

= ε



∞

−∞

(u)e

iωu



A(X, Z,εt − εu)e

i(kz−ωt)

+ c.c.



du + O(ε

We expand the slowly varying amplitude A around the point εt

L,x

=ε



∞

−∞

du χ

(u)e

iωu



1 − εu

∂

∂T

(εu)

∂

∂T

+ ···



A(X, Z, T )e

i(kz−ωt)

+ c.c.



+ O(ε

Recall that the Fourier transform of χ

, written as ˆχ

, and its derivatives are

ˆχ

(ω) =



∞

−∞

(u)e

iωu

ˆχ



(ω) =



∞

−∞

iuχ

(u)e

iωu

ˆχ



(ω) =



∞

−∞

−u

(u)e

iωu

du.

Then we can write the linear part of the polarization as

L,x

= ε



ˆχ

(ω) + ˆχ



(ω)iε∂

− ˆχ



(ω)

(ε∂

)

+ ···



(Ae

iθ

+ c.c.) + O(ε

)

= ε ˆχ

(ω + iε∂

)(Ae

iθ

+ c.c.) + O(ε

174 Nonlinear Schr¨odinger models in nonlinear optics

The last line is in a convenient notation where the term ˆχ

(ω + iε∂

)isan

operator that we can expand around ω to get the previous line.

In the nonlinear polarization equation (7.9), there will be interactions due

to the leading-order mode Ae

iθ

, for example, the nonlinear term includes E

which leads to terms such as A

3iθ

. Then we denote the corresponding linear

polarization term (containing interaction terms) as

interactions

L,x

= ˆχ

(ω

+ iε∂

)(B

imθ

+ c.c.),

where ω

= mω and B

contains the nonlinear terms generated by the

interaction (e.g. B

= A

Similarly there are nonlinear polarization terms. For example, a typical term

in the nonlinear polarization is

NL,x

= ε

ˆχ

xxxx

(ω

+ iε∂

,ω

+ iε∂

,ω

+ iε∂

)

× B

)

i(m+n+l)θ

+ c.c.

(7.13)

All other terms are of smaller order O(ε

7.3 Derivation of the NLS equation

So far, we have derived expressions for the polarization with a cubic nonlin-

earity (third-order susceptibility). Now we will use (7.5) to derive the NLS

equation. This will give the leading-order equation for the slowly varying

amplitude of the x-component of the electromagnetic ﬁeld E

We begin the derivation of the NLS equation by showing that E

 E

Recall (7.1c) with (7.3),

∇ · (E + P) = 0.

Since there is no y dependence, we get

∂

+ P

) + ∂

+ P

) = 0. (7.14)

We assume

= εA(X, Z, T )e

iθ

+ c.c. + O(ε

)

= εA

(X, Z, T )e

iθ

+ c.c. + O(ε

(7.15)

where as before c.c. denotes the complex conjugate of the preceding term. A

further note on notation. Because the x-component of the electromagnetic ﬁeld

appears so often, we label its slowly varying amplitude with A, whereas