Xing Zh., Zhou Sh. Neutrinos in Particle Physics, Astronomy and Cosmology
Подождите немного. Документ загружается.

266 7 Neutrinos
f
rom
S
upernovae
whether matter e
ff
ects pla
y
an important role in the
fl
avor conversions o
f
su
-
p
ernova neutrinos. Given two inde
p
endent neutrino mass-s
q
uared differences
|
Δ
m
2
3
1
|
≈
2
.4
×
10
−
3
e
V
2
an
d
Δ
m
2
21
≈
8
.
0
×
10
−
5
eV
2
,t
h
e question
b
ecomes
whether the M
SW
resonance conditions
f
or neutrino oscillations are satis
fi
e
d
i
n the supernova environment. Considerin
g
a
g
eneric neutrino mass-square
d
d
iff
e
r
e
n
ce
Δ
m
2
and the flavor mixing angle
θ
,
one finds that the matter den
-
s
ity on the MSW resonance is given by
(
Kuo and Pantaleone, 1989
)
ρ
∗
=
m
H
√
2
G
F
Y
e
YY
·
Δ
m
2
2
E
cos
2
θ
,
(
7.21
)
w
h
e
r
e
m
H
istheatomicmassunit
,
Y
e
Y
Y
denotes the electron number
f
raction
,
a
nd
E
r
epresents the neutrino beam energy. As mentioned before, the initial
n
eutrino
fl
uxes o
f
muon and tau
fl
avors are equal. Moreover, the weak inter-
act
i
o
n
sof
ν
μ
a
n
d
ν
τ
neutrinos are almost indistin
g
uishable when the ener
g
ie
s
a
re about tens of Me
V
. Hence the oscillation betwee
n
ν
μ
a
nd ν
τ
is irrelevant
h
ere, and it is convenient to work in the flavor basis
(
ν
e
,
ν
μ
,
ν
τ
)
which ren-
d
ers the effective Hamiltonian to be dia
g
onal in the
ν
μ
-
ν
τ
s
ector
(
Dighe an
d
Smirnov, 2000
)
. In view of the smallness of the neutrino mixing angle
θ
13
,
w
e
m
ay describe the system by two pairs of mixing parameters:
(
θ
1
3
,
Δ
m
2
31
)
an
d
(
θ
12
,
Δ
m
2
21
)
. A global analysis of current neutrino oscillation data actuall
y
yie
ld
s
θ
12
≈
3
4
◦
a
n
d
θ
1
3
<
12
◦
(
Gonzalez-Garci
a
e
ta
l.
,
2010
)
.Forthehig
h
n
eutrino mass-squared di
ff
erence, the resonance condition is
f
ul
fi
lled a
t
ρ
∗
H
=
3
.
4
×
10
3
g
c
m
−
3
|
Δ
m
2
31
|
2
.
4
×
1
0
−
3
e
V
2
1
0Me
V
E
0
.
5
Y
e
YY
,
(
7.22
)
where cos 2
θ
13
≈
1 has been taken; and for the low mass-s
q
uared difference
,
ρ
∗
L
=4
2
g
cm
−
3
Δ
m
2
21
8
.
0
×
1
0
−
5
eV
2
1
0Me
V
E
0
.
5
Y
e
YY
,
(
7.23
)
where cos
2
θ
12
≈
0
.37 has been input. So the MSW resonances ma
y
onl
y
occur in the outer layers of the mantle or envelope
(
i.e., the regions far fro
m
the supernova core and neutrino spheres
)
. Because o
f
|
Δ
m
2
3
1
|
Δ
m
2
2
1
,
t
he
two resonances are well se
p
arated in s
p
ace. Hence it is reasonable to treat the
whole three-
fl
avor system as a two-
fl
avor problem in each resonant re
g
ion.
A
s shown in Section 5.1, the resonant flavor conversion ma
y
be eithe
r
a
diabatic or non-adiabatic, or in-between, depending on the adiabaticity pa-
r
a
m
eter
γ
.G
iven the density pro
fi
l
e
ρ
(
r
)
=10
13
g
cm
−
3
(
10 k
m
/
r
)
3
≡
A
r
−
3
,
which is typical
f
or a supernova durin
g
the
fi
rst
f
ew seconds a
f
ter bounce
(
Brown et al.
,
1982; Bethe, 1990
)
, the transition probability between tw
o
m
ass ei
g
enstates can be calculated by usin
g
the Landau-Zener
f
ormula
P
LZ
PP
=
e
x
p
−
π
2
γ
=e
x
p
−
E
0
E
2
/
3
,
(
7.24
)

7.3 Matter E
ff
ects on
S
upernova Neutrinos 26
7
w
h
ere t
h
ecritica
l
ener
gy
E
0
is given by
(
Dighe and Smirnov, 2000
)
E
0
=
π
12
3
/
2
Δ
m
2
sin
3
2
θ
cos
2
2
θ
2
√
2
G
F
Y
e
YY
m
H
A
1
/
2
.
(
7.25
)
Note that
Δ
m
2
=
|
Δ
m
2
31
|
a
n
d
θ
=
θ
13
should be taken in Eq.
(
7.25
)
for the
h
igh-level resonance. More explicitly, we have
(
Lunardini and Smirnov, 2003
)
E
H
0
2
.
6
×
10
7
M
e
V
|
Δ
m
2
31
|
2
.
4
×
10
−
3
eV
2
s
i
n
3
θ
1
3
.
(
7.26
)
It is now evident tha
t
γ
∝
(
E
H
0
/E
)
2
/
3
d
e
p
ends on
θ
13
.
F
or si
n
2
θ
13
1
0
−
3
a
n
d
E
∼
10 MeV
,
one
h
a
s
P
H
PP
=
exp
[
−
(
E
H
0
/E
)
2
/
3
]
≈ 0w
h
ic
h
imp
l
ies a pure
ad
i
abat
i
cco
n
ve
r
s
i
o
n. F
o
r
s
in
2
θ
1
3
10
−
5
a
n
d
E
∼
1
0MeV,one
h
a
s
P
H
PP
≈
1
which stron
g
ly violates the adiabaticity. For an intermediate value of si
n
2
θ
13
,
P
H
P
P
i
ncreases wit
h
t
h
eneutrinoenerg
y
E
. After substitutin
g
Δ
m
2
=
Δ
m
2
21
a
n
d
θ
=
θ
1
2
i
nto Eq.
(
7.25
)
, we find that the low-level resonant conversion
i
s always adiabatic
(
i.e.,
E
L
0
E
a
nd thus
P
L
PP
≈
0)
. As for antineutrinos,
one may simi
l
ar
l
yeva
l
uate t
h
e transition pro
b
a
b
i
l
itie
s
P
H
a
n
d
P
L
i
f
t
h
e
resonances exist.
G
ive
n
Δ
m
2
31
>
0
,
b
ot
hh
i
gh
-an
dl
ow-
l
eve
l
resonances occur
i
n the neutrino sector. For
Δ
m
2
31
<
0,
the low-level resonance occurs in the
n
eutrino sector an
d
t
h
e
h
ig
h
-
l
eve
l
one ta
k
es p
l
ace in t
h
e antineutrino sector
.
B
ecause o
f
the hi
g
h matter density in a supernova, the e
ff
ective neu
-
trino mixing angles are suppressed and the flavor states
(
ν
e
,
ν
μ
,
ν
τ
)
coincid
e
with the effective mass eigenstates
(
ν
1m
,ν
2
m
,
ν
3
m
)
in matter. Hence the flavor
c
onversions can be treated as the propa
g
ation o
f
neutrino mass ei
g
enstates
a
nd the crossing among them. At the surface of a supernova one arrives a
t
the incoherent
fl
uxes o
f
neutrino mass ei
g
enstates, which will travel to th
e
s
urface of the Earth without further flavor conversions. Let us consider the
flavor conversions in the case of either
m
3
>m
2
>m
1
(
normal hierarchy
)
o
r
m
2
>m
1
>
m
3
(
inverted hierarchy
)
for both neutrinos and antineutrino
s
(
Dighe and Smirnov, 2000
).
(
1
)
T
h
enorma
l
neutrino mass
h
ierarc
h
y
.
In this case the original fluxes of
n
eutrino mass ei
g
enstates are
g
iven
b
y
F
0
ν
FF
1m
=
F
0
ν
FF
x
=
F
0
ν
FF
2m
a
n
d
F
0
ν
FF
3m
=
F
0
ν
FF
e
,
whe
r
e
F
0
ν
FF
x
=
F
0
ν
FF
μ
=
F
0
ν
FF
τ
h
o
ld
sasanexce
ll
ent approximation. T
h
epro
b
a
b
i
l
it
y
for
ν
3m
to be
ν
1
at t
h
esu
r
face
i
s
P
H
PP
P
L
PP
;
i.e., t
h
is
h
appens t
h
rou
gh b
ot
h
h
i
g
h- and low-level crossin
g
s. The probabilities o
f
ν
1m
→
ν
1
a
n
d
ν
2m
→
ν
1
transitions turn out to be
(
1
−
P
L
PP
)
an
d
P
L
PP
(
1
−
P
H
PP
)
, respectively. So the flux
o
f
the mass ei
g
enstat
e
ν
1
at the supernova sur
f
ace reads
F
0
ν
FF
1
=
P
H
PP
P
L
PP
F
0
ν
FF
e
+
(
1
−
P
H
PP
P
L
PP
)
F
0
ν
FF
x
.
(
7.27
)
O
ne may
fig
ure out the
fl
uxes o
f
ν
2
a
n
d
ν
3
in a simi
l
ar wa
y:
F
0
ν
F
F
2
=
P
H
P
P
(
1
−
P
L
P
P
)
F
0
ν
F
F
e
+(1
−
P
H
P
P
+
P
H
P
P
P
L
P
P
)
F
0
ν
F
F
x
,

268 7 Neutrinos
f
rom
S
upernovae
F
0
ν
FF
3
=
(1
−
P
H
PP
)
F
0
ν
FF
e
+
P
H
PP
F
0
ν
FF
x
.
(
7.28
)
The flux of electron neutrinos at the Earth is then obtained by projectin
g
the mass eigenstates onto the flavor eigenstates. Up to a factor
L
−
2
,
w
h
er
e
L
is t
h
e
d
istance to t
h
e Eart
h
,we
h
ave
F
E
ν
FF
e
=
i
|
V
ei
VV
|
2
F
0
ν
FF
i
=
pF
0
ν
FF
e
+
(
1 −
p
)
F
0
ν
FF
x
(
7.29
)
to
g
ether with the survival probability
p
=
|
V
e
V
V
1
|
2
P
H
P
P
P
L
P
P
+
|
V
e
V
V
2
|
2
P
H
P
P
(
1
−
P
L
P
P
)+
|
V
e
V
V
3
|
2
(
1
−
P
H
P
P
)
,
(
7.30
)
whe
r
e
V
ei
VV
(
fo
r
i
=
1
,
2
,
3
)
denotes an element of the neutrino mixing matri
x
V
.
The total flux of
ν
μ
a
nd
ν
τ
c
an be derived from the conservation of neutrin
o
fluxes:
F
E
ν
FF
μ
+
F
E
ν
FF
τ
=(
1
−
p
)
F
0
ν
FF
e
+
(
1+
p
)
F
0
ν
FF
x
.
As for su
p
ernova antineutrinos,
their mass eigenstates coincide with their flavor eigenstates
(
i.e.
,
ν
1m
=
ν
e
,
ν
2m
=
ν
μ
an
d
ν
3
m
=
ν
τ
)
. As mentioned above, there is no level crossing i
n
the antineutrino sector
f
or the normal mass hierarchy. Hence antineutrino
s
c
an always keep in the mass ei
g
enstates until they reach the Earth. It is the
n
s
traightforward to calculate the flux of electron antineutrinos at the Earth
:
F
E
ν
FF
e
=
i
|
V
ei
VV
|
2
F
0
ν
FF
i
=
pF
0
ν
FF
e
+(1
−
p
)
F
0
ν
FF
x
(
7.31
)
to
g
ether with the survival probabilit
y
p
=
|
V
e
VV
1
|
2
.Th
esu
m
of
ν
μ
a
n
d
ν
τ
flu
x
es
c
an similarly be fixed by the conservation of antineutrino fluxes
.
(
2
)
Th
e inverte
d
neutrino mass
h
ierarc
h
y.Inthere
g
ion o
f
hi
g
h matter
d
ensities, the neutrino mass ei
g
enstates are identified with their flavor ei
g
en
-
s
tates as follows
:
ν
1m
=
ν
μ
,
ν
2
m
=
ν
e
an
d
ν
3
m
=
ν
τ
f
or neutrinos
;
ν
1m
=
ν
τ
,
ν
2m
=
ν
μ
a
n
d
ν
3m
=
ν
e
for antineutrinos
(
Dighe and Smirnov, 2000
)
.Re-
c
all that the hi
g
h-level resonance exists in the antineutrino sector and th
e
l
ow-level one occurs in the neutrino sector. In view of Eqs.
(
7.29
)
and
(
7.31
)
,
we
fi
nd that it is onl
y
needed to calculate the survival probabilities o
f
elec
-
tron neutrinos
(
p
)
and electron antineutrinos
(
p
)
. Since the initial electro
n
neut
rin
o
ν
e
i
sint
h
e mass eigenstate
ν
2
m
,
the probabilities o
f
ν
2
m
→
ν
1
a
n
d
ν
2m
→
ν
2
t
r
a
n
sitio
n
sa
r
e
P
L
PP
a
nd
(
1
−
P
L
PP
)
, respectively. So we hav
e
p
=
|
V
e
V
V
1
|
2
P
L
PP
+
|
V
e
VV
2
|
2
(1
−
P
L
PP
)
.
(
7.32
)
A
s for antineutrinos
,
ν
e
initia
ll
y coinci
d
es wit
h
ν
3
m
.Itco
n
ve
r
ts
in
to
ν
1
v
i
a
t
h
e
h
i
gh
-
l
eve
l
resonance wit
h
t
h
epro
b
a
b
i
l
ity
P
H
,
an
d
sta
y
si
n
ν
3
w
i
th the
probability
(1
−
P
H
)
. The survival probability of electron antineutrinos turns
out to be
p
=
|
V
e
V
V
1
|
2
P
H
+
|
V
e
V
V
3
|
2
1
−
P
H
.
(
7.33
)
7.3 Matter E
ff
ects on
S
upernova Neutrinos 26
9
E
qs.
(
7.29
)
and
(
7.31
)
together with Eqs.
(
7.32
)
and
(
7.33
)
give rise to the
fluxes of electron neutrinos and electron antineutrinos at the Earth.
Finally, we summarize the matter effects on neutrino oscillations in th
e
s
upernova mant
l
ean
d
enve
l
ope
:
p
=
|
V
e
VV
2
|
2
P
H
P
P
+
|
V
e
V
V
3
|
2
(
1
−
P
H
P
P
)
,
p
=
|
V
e
VV
1
|
2
,
(
7.34
)
f
or the normal neutrino mass hierarchy; and
p
=
|
V
e
VV
2
|
,
p
=
|
V
e
VV
1
|
2
P
H
PP
+
|
V
e
V
V
3
|
2
(
1
−
P
H
PP
)
,
(
7.35
)
f
or the inverted neutrino mass hierarch
y
.Notethatwehaveset
P
L
PP
=
0an
d
P
H
P
P
=
P
H
. Althoug
h
P
H
PP
r
e
l
ies on sin
2
θ
13
, one may simplify Eqs.
(
7.34
)
an
d
(
7.35
)
in two interesting scenarios:
(
A
)
for sin
2
θ
1
3
10
−
3
a
n
dthus
P
H
PP
≈
0
,
one arr
i
ves a
t
p
=0an
d
p
=
s
in
2
θ
12
f
or the normal mass hierarch
y
o
r
p
=
sin
2
θ
1
2
an
d
p
= 0 for the inverted mass hierarchy;
(
B
)
for si
n
2
θ
13
10
−
5
a
n
dthus
P
H
PP
≈
1
,oneo
b
tains
p
=si
n
2
θ
1
2
a
n
d
p
=
cos
2
θ
1
2
fo
r
bot
hm
ass
h
ierarchies. The terms of si
n
2
θ
13
a
re omitted in either scenario. We conclude
that a measurement of the supernova neutrino fluxes has the great potentia
l
to pro
b
et
h
esma
ll
est neutrino mixin
g
an
gl
e
θ
13
a
n
d
pin
d
own t
h
eneutrin
o
m
ass hierarchy
(
Lunardini and Smirnov, 2003; Raffelt, 2005
).
7.3.3 Matter E
ff
ects in the Eart
h
We have discussed the supernova neutrino
fl
uxes at the sur
f
ace o
f
the Earth
.
D
ependin
g
on the location of a supernova and the time of a day, supernov
a
n
eutrinos may traverse the Earth before arriving at the detector. Hence a
c
omparison between the neutrino si
g
nals in two detectors located at di
ff
er
-
ent places could reveal the matter effects induced by the Earth
(
Dighe an
d
Smirnov, 2000; Dighe et a
l
.
,
2003
;M
irizzi
e
ta
l
., 2006
).
If
neutrinos do not cross the core o
f
the Earth, one ma
y
assume that th
e
a
verage matter density is a constant. The density profile changes abruptl
y
f
r
o
m
t
h
evacuu
m
to t
h
e
E
a
r
t
h. In
t
hi
scaseo
n
e
h
as to ca
l
cu
l
ate t
h
et
r
a
n
s
i
t
i
on
a
mplitude o
f
ν
(
1
)
i
→
ν
(
2
)
j
ν
,w
h
ere
ν
(
1
)
i
a
n
d
ν
(
2
)
j
ν
d
enote t
h
eneutrinomassei
g
en
-
s
tates in media “1” and “2”, respectively. Since the neutrino flavor eigenstates
c
ross the interface continuously, the transition amplitudes are given by
(
Kuo
a
nd Pantaleone, 1989
)
A
(
ν
(
1
)
i
→
ν
(
2
)
j
ν
)
=
cos
θ
(
2
)
−
sin
θ
(
2
)
s
i
n
θ
(
2
)
cos
θ
(
2
)
cos
θ
(
1
)
si
n
θ
(
1
)
−
s
in
θ
(
1
)
cos
θ
(
1
)
=
cos
θ
(
1
)
−
θ
(
2
)
si
n
θ
(
1
)
−
θ
(
2
)
−
s
i
n
θ
(
1
)
−
θ
(
2
)
cos
θ
(
1
)
−
θ
(
2
)
,
(
7.36
)
i
n which the two-flavor oscillation has been assumed
,
θ
(
1
)
an
d
θ
(
2
)
are th
e
c
orrespon
d
ing mixing ang
l
es in me
d
ia. Now we ca
l
cu
l
ate t
h
epro
b
a
b
i
l
ity t
h
at

270 7 Neutrinos
f
rom
S
upernovae
a
mass e
ig
enstat
e
ν
i
enterin
g
t
h
e Eart
h
arrives at t
h
e
d
etector as
ν
α
.
First
,
ν
i
c
onverts into the mass ei
g
enstates
ν
1m
an
d
ν
2m
in matter with the am
p
litude
s
given in Eq.
(
7.36
)
. Second, the propagation of mass eigenstates in a constant
m
atter pro
fi
le induces a phase di
ff
erenc
e
Φ
m
=
Δ
˜
m
2
L
/
(
2
E
)
betwee
n
ν
1m
a
n
d
ν
2m
,
wher
e
Δ
˜
m
2
s
tands for the effective neutrino mass-s
q
uared difference in
m
atter
,
L
is t
h
e
d
istance trave
l
e
db
y neutrinos, an
d
E
de
n
otes the
n
eut
rin
o
b
eam ener
g
y. Fina
ll
y
,
ν
1m
a
n
d
ν
2
m
reach the detecto
r
a
n
da
r
e
m
easu
r
ed as
ν
α
w
ith the amplitudes given by the neutrino mixing matrix. Put all thes
e
together, we obtain the probability o
f
ν
i
→
ν
α
as
(
Digh
e
et al
., 2003
)
P
iα
P
P
=
cos
θ
m
−
s
in
θ
m
sin
θ
m
cos
θ
m
10
0
e
i
Φ
m
cos
Δ
θ
s
i
n
Δ
θ
−
sin
Δ
θ
cos
Δ
θ
α
i
2
,
(
7.37
)
where
Δ
θ
≡
θ
m
−
θ
w
ith
θ
m
(
or
θ
)
being the mixing angle in matter
(
o
r
vacuum
)
. Takin
g
ν
1
→
ν
e
f
or example, we explicitly
g
et
P
1
PP
e
=cos
2
θ
12
−
si
n
2
θ
m
12
s
in 2
(
θ
m
12
−
θ
12
)
sin
2
Δ
˜
m
2
21
L
4
E
.
(
7.38
)
In addition, we can obtai
n
P
2
PP
e
=1
−
P
1
P
P
e
due to the probabilit
y
conservation.
We
p
roceed to consider the terrestrial matter effects on su
p
ernova neutrinos
.
G
iven the
fl
uxes o
f
neutrino mass ei
g
enstates
F
0
ν
FF
i
,
the
fl
ux o
f
ν
e
at the detecto
r
turns out to b
e
F
D
ν
FF
e
=
i
F
0
ν
FF
i
P
ie
PP
,
(
7.39
)
w
h
e
r
e
P
ie
PP
can be read off from Eq.
(
7.37
)
. In the case of a normal neutrin
o
m
ass hierarchy, the fluxe
s
F
0
ν
FF
i
havebeengiveninEqs.
(
7.27
)
and
(
7.28
).
These equations allow us to rewrite Eq.
(
7.39
)
as
F
D
ν
FF
e
=
p
D
F
0
ν
FF
e
+(
1
−
p
D
)
F
0
ν
FF
x
,
w
h
ere t
h
epro
b
a
b
i
l
ity p
D
rea
ds
p
D
=
P
1
PP
e
P
H
PP
P
L
PP
+
P
2
PP
e
P
H
PP
(1
−
P
L
PP
)
+
P
3
PP
e
(
1 −
P
H
PP
)
.
(
7.40
)
I
f
there are two detectors and onl
y
one o
f
them is shadowed b
y
the Earth,
then the terrestrial matter effects are characterized by the difference between
F
D
ν
FF
e
a
n
d
F
E
ν
FF
e
.
More exp
l
icit
ly
,
F
D
ν
FF
e
−
F
E
ν
FF
e
=
p
D
−
p
F
0
ν
FF
e
−
F
0
ν
FF
x
.
(
7.41
)
Note t
h
at t
h
e
m
atte
r
effects wou
l
dva
ni
s
hi
f
F
0
ν
FF
e
=
F
0
ν
FF
x
e
xactly held.
S
ince the
i
nitial fluxes of supernova neutrinos are energy-dependent,
(
F
0
ν
FF
e
−
F
0
ν
FF
x
)
is in
g
eneral positive at low ener
g
iesandbecomesne
g
ative at hi
g
her ener
g
ies.
So
i
t may change its sign at the critical energ
y
E
C
,
where
F
0
ν
FF
e
(
E
C
)
=
F
0
ν
FF
x
(
E
C
)
h
olds. Furthermore, Eqs.
(
7.30
)
and
(
7.40
)
lead us t
o
7.3 Matter E
ff
ects on
S
upernova Neutrinos 27
1
p
D
−
p
=
P
H
PP
(
1
−
2
P
L
PP
)
P
2
P
P
e
−
|
V
e
VV
2
|
2
,
(
7.42
)
wherewehavene
g
lected the contribution fro
m
ν
3
oscillations because it i
s
s
uppresse
db
y
b
ot
h
si
n
2
θ
1
3
and sin 2
(
θ
m
1
3
−
θ
1
3
)
. In the most general case, two
d
i
ff
erent detectors “D1” and “D2” are both shadowed b
y
the Earth. Then we
h
ave
(
Dighe and Smirnov, 2000
)
F
D
1
ν
FF
e
−
F
D2
ν
FF
e
=
P
H
PP
(
1
−
2
P
L
PP
)
P
D
1
2
PP
e
−
P
D2
2
PP
e
F
0
ν
FF
e
−
F
0
ν
FF
x
,
(
7.43
)
whe
r
e
P
D
1
2
P
P
e
a
n
d
P
D2
2
PP
e
denote the probabilities o
f
ν
2
→
ν
e
fo
r
two d
i
ffe
r
e
n
t
d
etectors. Some comments on Eq.
(
7.43
)
are in order:
(
1
)
the contributions
f
rom the initial supernova neutrino fluxes
(
F
0
ν
FF
e
−
F
0
ν
FF
x
)
, the flavor conversions
i
nthesu
p
ernova envelo
pe
P
H
P
P
(
1
−
2
P
L
P
P
) and the propagation in the Earth
e
x
(
P
D1
2
PP
e
−
P
D
2
2
P
P
e
)
are clearly factorized;
(
2
)
a difference between the initial ν
e
a
nd
ν
x
fl
uxes is required for significant matter effects;
(
3
)
the high-level resonanc
e
n
eeds to be non-adiabatic
(
i.e.,
P
H
PP
≈
1)
, otherwise the matter effects would
b
e suppresse
dby
P
H
PP
.
One may analogously consider the matter effects o
n
electron antineutrinos and obtain the counterpart of Eq.
(
7.43
)
as follows
:
F
D1
ν
FF
e
−
F
D2
ν
FF
e
=
1
−
2
P
L
P
D1
1
e
−
P
D2
1
e
F
0
ν
FF
e
−
F
0
ν
FF
x
.
(
7.44
)
N
ote that the facto
r
P
H
d
oes not appear in Eq.
(
7.44
)
, because the high-level
resonance is absent in the antineutrino sector. As for the inverted neutrino
m
ass hierarchy, a straightforward analysis yields
(
Dighe and Smirnov, 2000
)
F
D
1
ν
FF
e
−
F
D2
ν
FF
e
=
(
1
−
2
P
L
PP
)
P
D1
2
e
−
P
D2
2
e
F
0
ν
FF
e
−
F
0
ν
FF
x
(
7.45
)
f
or neutrinos
;
and
F
D1
ν
FF
e
−
F
D2
ν
FF
e
=
P
H
1
−
2
P
L
P
D1
1
PP
e
−
P
D2
1
PP
e
F
0
ν
FF
e
−
F
0
ν
FF
x
(
7.46
)
f
or antineutrinos. In li
g
ht o
f
current neutrino oscillation data, the low-level
resonance is always adiabatic and thus
P
L
PP
=
P
L
=
0holds.SoEq.
(
7.45
)
is
obtainable from Eq.
(
7.44
)
with the replacement
s
ν
e
→
ν
e
a
n
d
ν
x
→
ν
x
;
an
d
E
q.
(
7.46
)
can be obtained from Eq.
(
7.43
)
with the replacement
s
ν
e
→
ν
e
,
ν
x
→
ν
x
a
n
d
P
H
P
P
→
P
H
.
Table
7
.4 summarizes the matter effects both in
t
h
e supernova enve
l
ope an
d
in t
h
e Eart
h
. For t
h
e
l
atter, we on
ly l
ist t
h
e
c
hannels in which the terrestrial matter effects may be si
g
nificant. Note tha
t
t
h
es
h
oc
k
wave wi
ll
pass t
h
e
d
ense region, w
h
ere t
h
e
h
ig
h
-
l
eve
l
resonanc
e
takes place, and ma
y
break the adiabaticit
y
o
f
the resonance. In this case th
e
s
urvival probabilities have to be modified
(
Schirato and Fuller, 2002; Fogli
et al
.
,
2003, 2005
)
. The channels sensitive to the shock-wave effects are als
o
l
iste
d
in Ta
bl
e7.4.

272 7 Neutrinos
f
rom
S
upernovae
Table 7.4 The survival probabilitie
s
p
(
neutrinos
)
an
d
p
(
antineutrinos
)
, terres
-
trial matter effects and shock-wave effects in three scenarios
(
Raffelt, 2005. With
permission from the Institute of Physics
)
Mass
h
ierarc
h
ysi
n
2
θ
13
p
p
E
arth matter
S
hock wave
No
rm
al
10
−
3
0
cos
2
θ
12
ν
e
ν
e
In
ve
r
ted
10
−
3
s
in
2
θ
12
0
ν
e
ν
e
A
n
y
10
−
5
s
in
2
θ
12
cos
2
θ
12
ν
e
a
n
d
ν
e
–
7
.4
C
ollective Neutrino Flavor
C
onversions
S
oon a
f
ter the discover
y
o
f
the M
S
W matter e
ff
ects on solar neutrino
fl
avor
c
onversions, it was realized that the coherent forward scattering of neutrinos
on a
d
ense neutrino
b
ac
kg
roun
d
s
h
ou
ld b
e important in t
h
e core-co
ll
apse
s
upernovae and in the early Universe
(
Fulle
r
et al.
, 1987; N¨otzold and Ra
f
-
f
elt, 1988
)
. In contrast with the MSW effects in ordinary matter, the neu-
trino sel
f
-interaction potential has the o
ff
-dia
g
onal elements in the
fl
avor basis
(
Pantaleone, 1992a, 1992b
)
. Due to this neutrino self-interaction potential,
n
eutrinos mig
h
t
h
ave un
d
ergone co
ll
ective osci
ll
ations in t
h
eear
l
yUniverse
(
i.e., a large fraction of neutrinos with different energies oscillated coherently
)
(
Samuel, 1993, 1996; Kosteleck´
y
e
tal.
,
1993; Kosteleck´
y
and Samuel, 1993,
1
994
,
1995
,
1996
;
Panta
l
eone
,
1998
;
Pastor
e
ta
l
., 2002
).
I
n the context o
f
core-collapse supernovae, the s
y
nchronized and bipolar
n
eutrino oscillations are the main features of the collective phenomena
(
Dua
n
a
n
d
Kne
ll
er, 2009; Dua
n
et al
.
,
2010
)
. Such collective effects may give rise t
o
the almost complete
fl
avor conversions and dramaticall
y
a
ff
ect the neutrin
o
energy spectra. In this section we shall introduce collective neutrino oscil
-
l
ations an
d
try to un
d
erstan
d
t
h
ep
h
ysics
b
e
h
in
d
t
h
em. It s
h
ou
ld b
e
k
ept
i
nmin
d
t
h
at t
h
istopicisnowun
d
er active stu
d
ies, an
d
it remains unc
l
ear
whether collective neutrino oscillations will be significantly changed in a real
-
i
stic supernova environment inc
l
u
d
in
g
t
h
e non-sp
h
erica
lg
eometry, convective
effects and magnetic fields
(
Dua
n
et al
., 2010
)
. Hence we shall concentrat
e
on the generic features of collective neutrino oscillations and some analytical
u
nderstandin
g
o
f
them by assumin
g
the standard delayed-explosion scenario.
7.4.1 Equations of Motion
In Section 5.3 we have formulated neutrino oscillations in the language o
f
the density matrix, presented the equations of motion for the flavor polar-
i
z
atio
n
vecto
r
s
P
a
n
d
P
, and illustrated the nonlinear behavior o
ffl
avo
r
c
onversions in the simplest case with the isotropic neutrino gases, monochro
-
m
atic neutrino energy spectra and equal number densities of neutrinos and
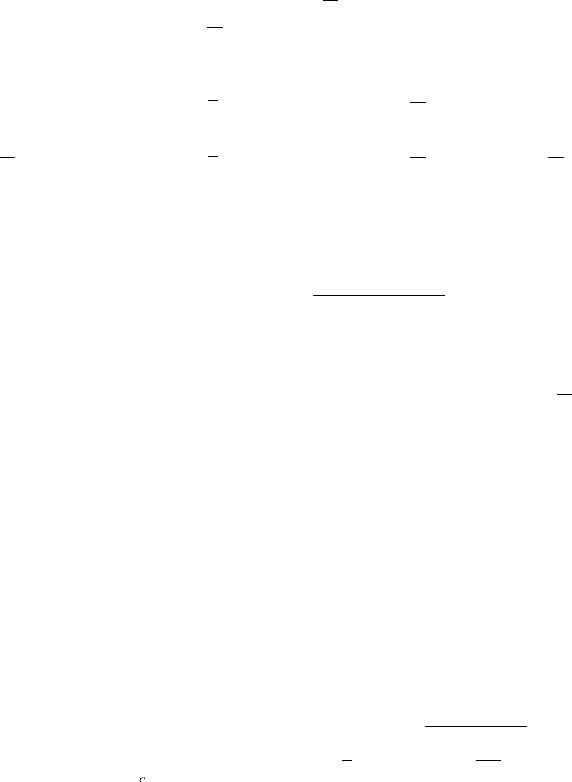
7
.4
C
ollective Neutrino Flavor
C
onversions 2
73
a
ntineutrinos. To
b
emorerea
l
istic, we intro
d
uce t
h
eso-ca
ll
e
d
neutrino
b
u
lb
m
odel which accounts for the
g
eometry of the neutrino emission from a proto
-
n
eutron star
(
Dua
n
et a
l
., 2006b, 2010
)
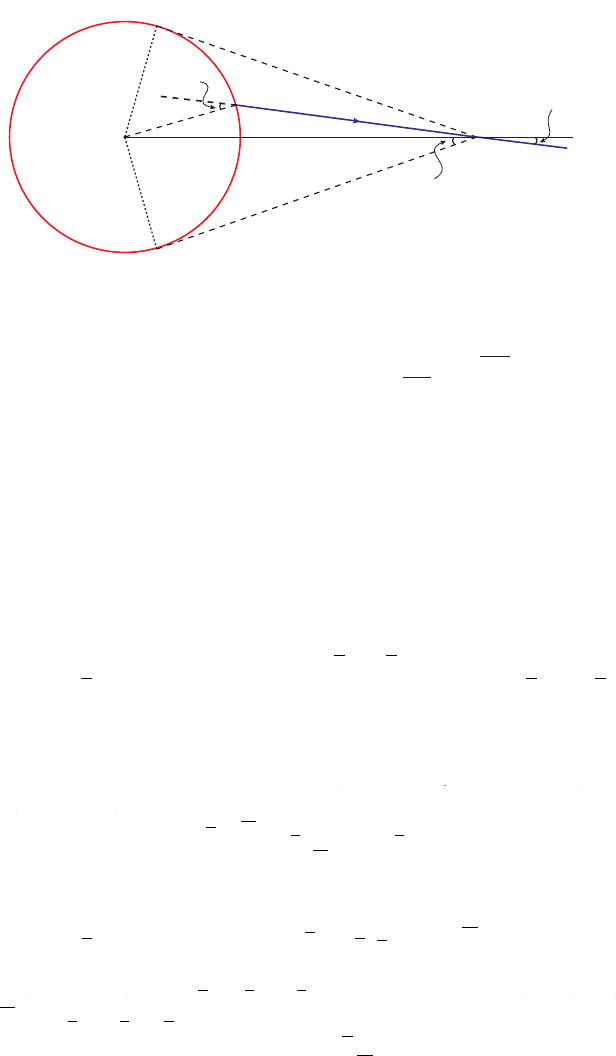
. Fig. 7.3 shows the correspondin
g
g
eometrical layout. The test neutrinos with di
ff
erent emission an
g
les
ϑ
R
m
a
y
experience different flavor conversions, because the
y
have distinct histories
of interactions when traveling from the surface of the proto-neutron star
to po
i
nt
A
w
i
th a
r
ad
i
us
r
. The back
g
round neutrinos come
f
rom a spe-
c
ial part of the neutrino sphere, which is within the cone with an open angle
ϑ
ma
x
= arcsin
(
R
ν
/
r
)
. Given the geometry in Fig. 7.3, the equations of motio
n
f
or the polarization vectors
P
p
(
t
)
an
d
P
p
(
t
)
in Eq.
(
5.146
)
can be rewritte
n
as t
h
ose fo
r
P
ϑ
(
E,
t
)
and
P
ϑ
(
E
,t
)
,wher
e
ϑ
is the polar an
g
le o
f
the neutrin
o
m
omen
t
u
m
p
an
d
E
=
|
p
|
is the neutrino energy:
˙
P
ϑ
=
+
ω
B
+
λ
L
+
2
√
2
π
G
F
d
c
ϑ
d
E
(
P
ϑ
−
P
ϑ
)
g
(
ϑ
,
ϑ
)
×
P
ϑ
,
˙
P
ϑ
=
−
ω
B
+
λ
L
+
2
√
2
π
G
F
d
c
ϑ
d
E
(
P
ϑ
−
P
ϑ
)
g
(
ϑ,
ϑ
)
×
P
ϑ
,
(
7.47
)
whe
r
e
g
(
ϑ
,
ϑ
)
≡
1
−
c
ϑ
c
ϑ
,
c
ϑ
≡
cos
ϑ
a
n
d
c
ϑ
≡
cos
ϑ
w
i
th
ϑ
b
ein
g
t
h
e
polar angle of the momentu
m
q
of the background neutrinos. Note that
ϑ
∈
[0
,
ϑ
max
]
and thus co
s
ϑ
∈
[
co
s
ϑ
m
ax
,
1]
. In view of Fig. 7.3, one find
s
r sin
ϑ
=
R
ν
si
n
ϑ
R
,t
=
r
2
−
R
2
ν
sin
2
ϑ
R
−
R
ν
cos
ϑ
R
,
(
7.48
)
a
nd thus there exists a one-to-one correspondence between
(
t,
ϑ
)
and
(
r,
ϑ
R
)
.
With the help of the second expression in Eq.
(
7.48
)
,weget
d
t
=d
r/
cos
ϑ
.
S
o the polarization vectors can also be expressed a
s
P
ϑ
R
(
E,r
)
and
P
ϑ
R
(
E
,r
)
.
These observations imply that the evolution of the polarization vectors wit
h
t
i
s equiva
l
ent to t
h
at wit
h
r
.
T
h
e
l
atter is more convenient to stu
dy
t
h
e
flavor conversions of su
p
ernova neutrinos.
I
n the multi-angle calculations, the equations of motion are numericall
y
so
l
ved for
ϑ
R
a
n
d
E
(
Duan
et al
., 2006
b
;Fo
gl
i
et al.
,
2007
)
. Since such simu
-
l
ations are very time-consuming and too complicated to make the underlying
p
h
ysics transparent, it ma
k
es sense to resort to some ana
l
ytica
l
approxima
-
tions in t
h
esin
gl
e-an
gl
e
l
imit
b
y assumin
g
a
ll
t
h
epo
l
arization vectors t
o
behave as the ones at
ϑ
R
=
0. The corresponding equations of motion ca
n
be derived from Eq.
(
7.47
)
by setting
ϑ
=
ϑ
R
=0an
dd
iscar
d
in
g
t
h
ean
g
u
l
a
r
d
ependence o
f
the polarization vectors. In this case the inte
g
ration over th
e
polar angle
ϑ
y
ields a geometrical function
D
(
r
)
≡
1
cos
ϑ
max
d
c
ϑ
(1
−
c
ϑ
)=
1
2
⎡
⎣
1
−
!
1
−
R
ν
r
2
⎤
⎦
2
.
(
7.49
)
We hav
e
D
(
r
)
∝
r
−
4
fo
r
r
R
ν
.Inthe
f
ollowin
g
discussions, we shal
l
only consider the single-angle approximation in order to analytically revea
l
274 7 Neutrinos
f
rom
S
upernovae
O
D
B
A
C
ϑ
ϑ
m
ax
ϑ
R
r
R
ν
Neutrino
t
Sp
here
Fig. 7.3 A sketch of the neutrino emission from the neutrino sphere in the neutrino
bulb model
(
Duan
et al.
, 2006b, 2010. With permission from the American Physica
l
Society
)
. The test neutrinos emitted from the neutrino sphere at point
D
w
it
h
t
h
e
emission angl
e
ϑ
R
a
rrive at point
A
, and the elapsed time i
s
t
=
D
A. The distance
f
r
om
A
t
o the center o
f
the proto-neutron star is
r
=
O
A
,
and the polar angle o
f
t
h
eneutrinotra
j
ector
y
i
s
ϑ
,
which also characterizes the direction o
f
the neutrino
m
o
m
e
n
tum
ˆ
p
≡
p
/
|
p
|
. The background neutrinos come from the special part of the
neutrino sp
h
ere
l
yin
g
in t
h
econewit
h
an open an
gl
e
ϑ
ma
x
≡
a
rcsin
(
R
ν
/r
)
, where
R
ν
d
enotes the radius o
f
the neutrino s
p
her
e
the salient
f
eatures o
f
collective neutrino oscillations. This approximation is
reasonably
g
ood, althou
g
h the multi-an
g
le effects may cause the kinemati
c
d
ecoherence among the flavor conversions of neutrinos with different emissio
n
a
ngles
(
Esteban-Prete
l
et al.
,
2007; Fo
gl
i
et al
., 2007
).
A
s mentioned in Chapter 5, the three-flavor oscillations ma
y
approximate
to t
h
etwo
-fl
avo
r
osc
ill
at
i
o
n
s
ν
e
↔
ν
x
a
n
d
ν
e
↔
ν
x
due to
|
Δ
m
2
31
|
Δ
m
2
21
,
whe
r
e
ν
x
(
or
ν
x
)
stands for the superposition of
ν
μ
a
n
d
ν
τ
(
or
ν
μ
a
n
d
ν
τ
)
a
nd the relevant mixing parameters ar
e
Δ
m
2
31
a
nd
θ
13
. Some comments o
n
the conventions in the equations of motion are in order
.
(
1
)
Taking account of the definitions of the flavor polarization vectors in
E
q.
(
5.142
)
,wehaveT
r
ρ
p
=
f
ν
f
f
e
(
p
)
+
f
ν
f
f
x
(
p
)
and
P
z
p
PP
=
f
ν
f
f
e
(
p
)
−
f
ν
f
f
x
(
p
)
w
i
th
f
ν
ff
e
(
p
)
and
f
ν
ff
x
(
p
) being the distribution functions of
e
x
ν
e
a
n
d
ν
x
, respec
-
tivel
y
. Similar relations for
ρ
p
,
P
p
,
f
ν
ff
e
(
p
)
an
d
f
ν
ff
x
(
p
)
can be obtained for
a
ntineutrinos. One may redefine
P
p
an
d
P
p
b
y factoring out the distribution
f
unctions such that the
y
onl
y
measure the
fl
avor composition o
f
the s
y
stem
:
ρ
p
=
1
2
f
ν
ff
(
p
)
1+
P
p
·
σ
,
ρ
p
=
1
2
f
ν
f
f
(
p
)
1+
P
p
·
σ
,
(
7.50
)
where
f
ν
f
f
=
f
ν
ff
e
+
f
ν
ff
x
a
nd
f
ν
f
f
=
f
ν
f
f
e
+
f
ν
f
f
x
.
Thus we have
P
z
=[
f
ν
ff
e
−
f
ν
ff
x
]
/f
ν
ff
a
nd
P
z
=[
f
ν
f
f
e
−
f
ν
f
f
x
]
/f
ν
f
f
, where the momentum de
p
endence is im
p
lied. Fo
r
a
system w
h
ic
h
initia
ll
y
h
as pur
e
ν
e
a
n
d
ν
e
,t
h
epo
l
arization vectors are
therefore normalized to unity
(
i.e.
,
|
P
p
|
=
|
P
p
|
=
1
)
. Note that different

7
.4
C
ollective Neutrino Flavor
C
onversions 2
75
n
ormalization schemes have been used in the literature
(
Hannestad
et al
.
,
2
006; Fo
g
li et al.
,
2007
;
Duan et al., 2010
)
.
(
2
)
The neutrino-antineutrino system under discussion is often assume
d
to be isotropic, so the direction o
f
the neutrino momentum is irrelevant. I
n
this case the flavor polarization vectors depend on the neutrino energy
E
≈
p
=
|
p
|
. If the frequenc
y
ω
=
|
Δ
m
2
31
|
/
(2
E
)
is defined, then the dependenc
e
o
n
E
ca
n
be co
n
ve
r
ted
in
to the o
n
eon
ω
.Thatiswh
y
the
fl
avor polarization
vectors are somet
i
mes wr
i
tten a
s
P
ω
an
d
P
ω
f
or each energy mode
.
(
3
)
If the strength of neutrino-neutrino interactions is defined as
μ
≡
√
2
G
F
n
ν
w
i
th
n
ν
b
ein
g
t
h
e
l
oca
l
neutrino num
b
er
d
ensity, t
h
en t
h
e equations
of motion can be rewritten as
P
ω
=[
+
ω
B
+
λ
L
+
μ
D
]
×
P
ω
,
P
ω
=[
−
ω
B
+
λ
L
+
μ
D
]
×
P
ω
,
(
7.51
)
whe
r
e
D
≡
P
−
P
w
i
th
P
≡
7
∞
0
77
P
ω
d
ω
a
n
d
P
≡
7
∞
0
77
P
ω
d
ω
b
ein
g
t
h
e
gl
o
b
a
l
p
olarization vectors. Note tha
t
n
ν
∝
D
(
r
)
L
ν
/
E
ν
,
wher
e
D
(
r
)
is the geo
-
m
etrical function given in Eq.
(
7.49
)
in the single-angle approximation
,
L
ν
d
enotes the total neutrino luminosit
y
an
d
E
ν
i
stheavera
g
eneutrinoener
g
y
.
In the region far from the neutrino sphere we hav
e
n
ν
(
r
)
∝
r
−
4
,
and the elec-
tron
d
ensity is approximate
l
y
n
e
(
r
)
∝
r
−
3
.
Just a
b
ove t
h
eneutrinosp
h
ere,
the neutrino density is so hi
g
h that the neutrino-neutrino sel
f
-interaction
becomes larger than the neutrino interaction with ordinary matter.
Numerical simulations with the
g
eneral equations o
f
motion have show
n
three salient features of collective neutrino oscillations
(
Duan
e
tal
.
,
2006a
,
2
006
b
, 2010; Fog
li
et a
l.
,
2007
)
:
(
1
)
sync
h
ronize
d
osci
ll
ations
—
neutrinos wit
h
d
ifferent energies
(
and thus different intrinsic oscillation frequencies
)
oscillat
e
c
oherently;
(
2
)
bi
p
olar oscillations
—
neutrinos and antineutrinos oscillate i
n
opposite directions and form two separate groups;
(
3
)
energy spectra
l
sp
l
it
s
—inthe
fi
nal neutrino and antineutrino ener
g
y spectra, a critical ener
gy
E
c
s
plits the spectra sharpl
y
into parts of almost pure different flavors. Thes
e
f
eatures will be described in detail in the following subsections
.
7.4.2
S
ynchronized Neutrino
O
scillations
L
et us recall the neutrino oscillations in vacuum, whose e
q
uation o
f
motio
n
rea
ds
˙
P
=
ω
B
×
P
w
it
h
ω
=
Δ
m
2
/
(
2
p
)
. This is just the equation of motion for
t
h
ean
g
u
l
ar-momentum
P
p
recessin
g
around the ma
g
netic
fi
el
d
B
,
an
d
t
h
e
c
orrespondin
g
ma
g
netic dipole moment is
g
iven by
M
=
ω
P
.
So ω
=
|
M
|
/
|
P
|
plays the role of gyromagnetic ratio and determines the rate of precession
.
N
ext, we consider an ensemble o
f
homo
g
eneous and isotropic neutrino
g
as
.
The total number of neutrinos i
s
N
ν
NN
,
and the ensemble has a lar
g
evolum
e
V
.
D
enoting the polarization vector of each neutrino as
P
j
a
n
d
t
h
e correspon
d
ing
mo
m
e
n
tu
m
as
p
j
=
|
p
j
|
(
fo
r
j
=
1
,
2
,
···
,N
ν
N
N
)
, one may define the total
p
olarization vector
J
=
P
1
+
P
2
+
···
+
P
N
ν
.Thee
q
uation of motion for the
i
ndividual polarization vector turns out to be
(
Pasto
r
et al
., 2002
)
