Spohn H. Dynamics of Charged Particles and their Radiation Field

Подождите немного. Документ загружается.

9.2 Some applications 113

magnetron

cyclotron

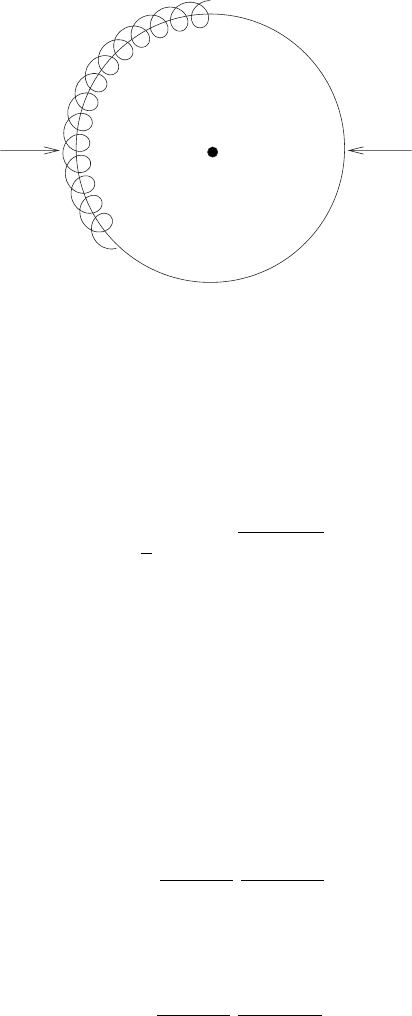
Figure 9.1: Orbit of an electron in a Penning trap seen from above.

with ψ = (r, u) and A

= 0, A

= 1l, A

= ω

1l, A

= iω

, V

= 0,

= 0, V

= iω

, V

= (ω

− ω

)1l, where σ

is the Pauli spin matrix

with eigenvectors χ

,σ

=±χ

. The unperturbed motion is governed by the

4 × 4 matrix A.Ithas the eigenvectors ψ

+,±

= (±i(1/ω

)χ

∓

,χ

∓

) with eigenval-

ues ±iω

and ψ

−,±

= (±i(1/ω

−

)χ

∓

,χ

∓

) with eigenvalues ±iω

−

, where





− 2ω



. (9.34)

The mode with frequency ω

is called the cyclotron mode and that with ω

−

called the magnetron mode. Experimentally ω

 ω

and therefore ω

 ω

−

The orbit is then an epicycle with rapid cyclotron and slow magnetron motion,

as shown in ﬁgure 9.1. The adjoint matrix A

∗

has eigenvectors orthogonal to the

ψ’s. They are given by ϕ

+,±

= (∓i(ω

/ω

)χ

∓

,χ

∓

) with eigenvalues ±iω

and

−,±

= (−(ω

/ω

−

)χ

∓

,χ

∓

) with eigenvalues ∓iω

−

Since β is small, the eigenfrequencies of A + βV can be computed in ﬁrst-order

perturbation. The cyclotron mode attains a negative real part corresponding to the

friction coefﬁcient

6πc

− ω

−

(9.35)

and the magnetron mode attains a positive real part corresponding to the antifric-

tion coefﬁcient

−

6πc

−

− ω

. (9.36)

As the electron radiates, it lowers its potential energy by increasing the magnetron

radius.

114 The Lorentz–Dirac equation

Experimentally B = 6 × 10

gauss and the voltage drop across the trap is 10 V.

This corresponds to ω

= 4 × 10

Hz,ω

= 1.1 × 10

Hz,ω

−

= 7.4 × 10

Hz.

The conditions (9.28), (9.29) are easily satisﬁed. For the lifetimes (1/γ

) = 5 ×

s, (1/γ

) = 8 ×10

−2

s, and (1/γ

−

) =−2 × 10

sare obtained. Thus the

magnetron motion is stable, as observed through keeping a single electron trapped

over weeks. The cyclotron motion decays within fractions of a second. The axial

motion is in fact damped by coupling to the external circuit and decays also within

a second.

The variation with the magnetic ﬁeld can be more clearly discussed in terms of

the dimensionless ratio (ω

/ω

) = λ. Then

= ω



λ ±



− 2



,γ

=±βω



λ ±



− 2





− 2.

(9.37)

For large λ, ω

∼

λ, ω

−

∼

−1

, while γ

∼

, γ

−

∼

−4

.Asλ →

√

2, we

have ω

= ω

−

= ω

√

2. However, the friction coefﬁcients diverge as (λ −

√

−1/2

. Let us call B

the critical ﬁeld at which the mechanical motion becomes

unstable. For B > B

, one still has periodic motion with frequency ω

√

2, but

the onsetting instability is revealed through the vanishing lifetime. In the men-

tioned experiment λ = 2.7 × 10

and for ﬁxed ω

the critical ﬁeld strength is

= 30 gauss.

9.3 Experimental status of the Lorentz–Dirac equation

Energy loss through radiation is a well-established phenomenon. Indeed, in syn-

chrotron sources electrons slow down because of radiation losses, and energy has

to be supplied to maintain a steady electron current. The supplied power is com-

puted on the basis of Larmor’s formula, and synchrotron sources are one promi-

nent example to conﬁrm its validity. On the other hand, the Lorentz–Dirac equation

goes way beyond mere energy balancing and claims to predict the orbit of an elec-

tron. Here synchrotron sources provide no test, since the modiﬁcation of the orbit

due to radiation damping is lost in the noise of experimental uncertainties. As a

fair summary, thus we can say that while qualitative aspects of radiation damping

are well tested, there is no single experiment which probes quantitatively the pre-

dictions of the electron motion by the Lorentz–Dirac equation. We propose and

discuss here two experiments which are within the reach of present-day techni-

ques.

To cope with the smallness of the radiation reaction, in essence, only two

approaches seem feasible. The ﬁrst one is to wait long enough until the effects

9.3 Experimental status of the Lorentz–Dirac equation 115

accumulate to something which may be detected, a route followed in the Penning-

trap experiment. The other option is to use ultrastrong ﬁelds. In either case, there

is no way to monitor directly the electron orbit and one has to rely on indirect

evidence, like lifetimes or emission spectra.

(i) Penning trap. In the previous section we discussed the electron orbits for the

Penning trap with the quadrupole potential in the quadratic approximation. The

Lorentz–Dirac equation predicts, in particular, the lifetime of the cyclotron mode.

For the ﬁeld strengths used in the high-precision measurement of the g-factor, this

lifetime is measured to 0.8 s in good agreement with the theoretical result. To

have a more stringent test what would be needed is a systematic determination

of how the lifetime depends on the magnetic ﬁeld strength. Another option of

interest is to turn the B-ﬁeld out off the symmetry axis. For this case we have not

computed the cyclotron lifetime, but could have done so by the scheme explained,

with the welcome complication that all three modes couple. The dependence of

the cyclotron lifetime on the orientation of the B-ﬁeld would be a valuable test of

the validity of the Lorentz–Dirac equation.

(ii) Ultrastrong laser pulse. A strong laser pulse hits a bound electron. Since the

atom ionizes instantaneously, the electron is subject only to the time-dependent

laser ﬁeld. Thus we set q

= 0, v

= 0, and for the external ﬁelds

E(x, t) = h(ωt − k · x)E

cos(ωt − k · x),

B(x, t ) = h(ωt − k · x)B

cos(ωt − k · x),

|=|B

|, E

· k = 0 = B

· k, E

· B

= 0. (9.38)

h is a shape function. The motion of the electron is governed by the Landau–

Lifshitz equation (9.7) augmented by the term

6πc

∂

∂t

(E + c

−1

v × B) (9.39)

because of the time dependence of the external ﬁelds. Our dynamical problem is

in fact two dimensional with the motion of the electron lying in the plane spanned

by E

and k.Nevertheless one has to rely on numerical integration, and we discuss

the example from Keitel et al. (1998).

The ultra-intense laser ﬁeld has an intensity of 10

Wcm

−2

. The frequency is

chosen to be ω = 3.54 ×10

−1

,which is in the near-infrared regime. We fol-

low the motion of the electron up to 3000 laser cycles, i.e. up to the ﬁnal time

t = 3000(2π/3.54 × 10

) s = 0.53 ×10

−11

s. Over that time span the shape

function is assumed to interpolate linearly between zero and the full ﬁeld strength.

116 The Lorentz–Dirac equation

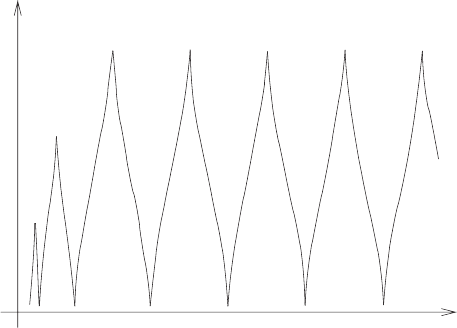
Figure 9.2: Orbit of an electron when hit by an ultrastrong laser pulse.

The electron motion is highly relativistic, as can be seen from the strong redshift

corresponding to only the seven electron cycles displayed in ﬁgure 9.2. The elec-

tron is displaced by 0.1586 cm in the propagation direction and has a maximal

amplitude of 0.795 × 10

−3

cm in the electric ﬁeld direction.

The effects of radiation damping are minute. In the propagation direction the

distance is increased by the fraction 7 × 10

−7

and in the electric ﬁeld direction it

is decreased by the fraction 10

−2

. Thus a direct veriﬁcation of the radiation re-

action is out of reach. However, in the emission spectrum the radiation damping

results in a roughly 1% change as compared to the frictionless solution with the

Lorentz force from the external ﬁelds of (9.38). In an experiment the radiation

spectrum has to be measured with such precision that, after the theoretical spec-

trum, computed without radiation reaction, has been subtracted, there is still a sig-

niﬁcant background which allows for a quantitative comparison with the emission

spectrum predicted by the Lorentz–Dirac equation.

Notes and references

Section 9

The name Lorentz–Dirac is standard but historically inaccurate. Some authors,

e.g. Rohrlich (1997), therefore propose Abraham–Lorentz–Dirac instead. The

radiation reaction term was originally derived in Abraham (1905); compare with

chapters 7 and 8. Von Laue (1909) realized its covariant form. In the Pauli

(1921) encyclopaedia article on relativity the equation is stated as in (9.1). Dirac’s

Notes and references 117

contribution is explained in section 3.3. Plass (1961) is a summary of exact solu-

tions of the Lorentz–Dirac equation.

Section 9.1

Detailed case studies of the Lorentz–Dirac equation, including its center manifold,

are listed in the Notes to section 8.2. Baylis and Huschilt (2002) critically explore

the relation to the Landau–Lifshitz equation. The substitution trick seems to have

been common knowledge. For example, without further comment it is used by

Pauli (1929) and Heitler (1936) in the particular case of a harmonic oscillator. In

its full generality the Landau–Lifshitz equation (9.10) appears already in the ﬁrst

edition of Volume II: The Classical Theory of Fields of the Landau–Lifshitz Course

in Theoretical Physics.Atnopoint is the reader given a hint on the geometrical pic-

ture of the solution ﬂow and on the errors involved in the approximation. To me it

is rather surprising that the contribution of Landau and Lifshitz is ignored in essen-

tially all discussions of radiation reaction, one notable exception being Teitelbom

et al. (1980). Spohn (1998, 2000a) uses singular perturbation theory to rederive the

Landau–Lifshitz equation. The appearance of singular perturbation theory is difﬁ-

cult to track. For a particular application it is clearly stated by Burke (1970). There

have been attempts to replace the Lorentz–Dirac equation by a second-order equa-

tion (Mo and Papas 1971; Shen 1972b; Bonnor 1974; Parrot 1987; Valentini 1988;

Ford and O’Connell 1991, 1993). Based on Ford and O’Connell (1991), Jackson

(1999) uses the substitution trick for a radiation damped harmonic oscillator and

discusses several applications. In the case of arbitrary time-dependent potentials,

only Landau and Lifshitz provide the correct center manifold equation. The struc-

ture discussed here reappears whenever a low-dimensional system is coupled to a

wave equation; for an application in acoustics see Templin (1999).

Section 9.2

Uniform acceleration is discussed in Fulton and Rohrlich (1960) and Rohrlich

(1990). A constant magnetic ﬁeld is of importance for synchrotron sources. Since

the electron is maintained on a circular orbit, Larmor’s formula is precise enough.

Landau and Lifshitz (1959) give a brief discussion. The power law for the ultra-

relativistic case is noted in Spohn (1998). Shen (1972a, 1978) discusses at which

ﬁeld strengths quantum corrections will become important. His results are only

partially reliable, since his starting point is not the Landau–Lifshitz equation. The

Penning trap is reviewed by Brown and Gabrielse (1986), which includes a discus-

sion of the classical orbits and their lifetimes. They state the results (9.35), (9.36)

118 The Lorentz–Dirac equation

as based on a quantum resonance computation. Since the ﬁnal answer does not

contain

,itmust follow from the Landau–Lifshitz equation (Spohn 2000a). In

the classical framework, general trap potentials can be handled through numerical

integration routines for ordinary differential equations. The self-force in the case

of synchroton radiation is studied by Burko (2000).

Section 9.3

The Penning-trap experiment is proposed in Spohn (2000a). The numerical results

on ultrastrong laser pulses are taken from Keitel et al. (1998). Another proposal,

which apparently never received the proper funding, is to measure the mega-gauss

magnetic bremsstrahlung for ultrarelativistic electrons (Erber 1971; Shen 1970).

Spinning charges

The Lorentz model includes by necessity the inner rotation of charges and, beyond

the translational degrees of freedom, one has to determine its effective dynamics.

This will lead to a derivation of the Bargmann–Michel–Telegdi (BMT) equation

from a microscopic basis including an expression for the gyromagnetic ratio. We

will also discuss the Abraham model with spin, a little-explored territory, since it

is more easily controlled mathematically and it teaches us how the BMT equation

is modiﬁed when Lorentz invariance is no longer available.

10.1 Effective spin dynamics of the Lorentz model

Let us recall the equations of motion for an extended charge, where for the moment

the interaction with the self-ﬁeld is ignored,

p = f,

s + Ω

· s = t . (10.1)

Here the external force f , respectively the external torque t, are deﬁned through

(2.92), respectively (2.95). Equation (10.1) must be supplemented by

p = m

u, s = I

w, (10.2)

which deﬁne the bare gyrational mass m

and the bare moment of inertia I

. Both

depend on |

We assume now that the external ﬁeld tensor is slowly varying, by replacing

F(q) by the scaled ﬁeld tensor εF(εq) in (2.92), (2.95). Note that this prescription

automatically includes slow variation in time. f and t simplify in the limit of small

ε and, on the macroscopic scale, (10.1) becomes

p = eF · u,

s + Ω

· s = µ(F · w)

⊥

(10.3)

119

120 Spinning charges

with the magnetic moment

µ =



xϕ(x)x

(10.4)

and a

⊥

= (g + u ⊗ u) · a.Since |w| is conserved, the translational motion is au-

tonomous, whereas the spin follows the local ﬁelds as they are encountered.

As a next step we have to include the coupling to the self-ﬁeld. In principle the

scheme of chapter 7 has to be repeated, but we prefer to take the static short-cut.

The energy–momentum relation for the Lorentz model was computed in chapter 4.

Thus we stipulate that the bare gyrational mass m

is renormalized to m

+ m

and

the bare moment of inertia to I

+ I

;see (4.43), (4.45), respectively (4.49), (4.51).

This means that instead of (10.2) we have

p = (m

+ m

)u, s = (I

+ I

)w . (10.5)

Equation (10.3) together with (10.5) is the effective dynamics in the adiabatic limit

on the Hamiltonian level neglecting radiation damping.

We want to compare our spin dynamics with the BMT equation which reads

w + Ω

· w =

(F · w)

⊥

, (10.6)

where m is the experimental mass and g the gyromagnetic ratio, which like the

charge is an intrinsic property of the particle. Using the fact that Ω

is determined

by Newton’s translational equations of motion one arrives at the perhaps more

familiar three-vector form for the angular velocity,

˙ω =

ω ×



− 1 +



−



− 1



1 + γ

−2

(v · B

−



−

1 + γ



−1

v × E



. (10.7)

Here v, E

, B

are to be evaluated along the given orbit. To compare (10.6) with

(10.3) one uses (10.6) and notes that, since |w| is a constant of motion,

w + Ω

· w =

+ I

(F · w)

⊥

. (10.8)

Therefore the gyromagnetic ratio of the Lorentz model is given by

g =

2µ

+ m

+ I

. (10.9)

The magnetic moment µ depends on the charge distribution, all other terms in

(10.9) on the mass distribution. Through their variation any value of g can be real-

ized, unless the charge and mass form factors are equal to each other, as assumed

already. In the case of a uniformly charged sphere [ball] of radius R the integrals

10.2 The Abraham model with spin 121

in (10.9) can be evaluated with the result (the ﬁrst term refers to a sphere and

[ ... ]toaball)

µ =





, (10.10)

= m

ωR

arctanhωR ,



= m

2(ωR)



ωR − (1 − (ωR)

)arctanhωR





(10.11)

4π R



1 +

(ωR)





4π R



(ωR)



, (10.12)

= m

2ω



− 1 +

1 + (ωR)

ωR

arctanhωR





= m

2ω

4(ωR)



3ωR − (ωR)

+ (−3 + 2(ωR)

+(ωR)

)arctanhωR





(10.13)

4π R



4π R



. (10.14)

In the limit e → 0, g

sphere

decreases from 1 to 2/3 and g

ball

from 1 to2/5asωR

increases from 0 to 1. In the opposite limit m

→ 0, one obtains

sphere

(ωR)

, g

ball

(ωR)

. (10.15)

10.2 The Abraham model with spin

Abraham models the charge as a nonrelativistic rigid body with mass distribution

ϕ and charge distribution eϕ, which for notational simplicity we take to be

proportional to each other. A complete mechanical description must specify both

the center of mass, q(t), and the angular velocity, ω(t) ∈

,relative to the center.

The spinning charge generates the current

j(x, t) =



v(t) + ω(t) × (x − q(t))



eϕ(x − q(t)) , (10.16)

which satisﬁes charge conservation, since ϕ is radial. Therefore the Maxwell equa-

tions have a modiﬁed source term and read

∂

B(x, t ) =−∇×E(x, t ),

∂

E(x, t) =∇×B(x, t) −



v(t) + ω(t) × (x − q(t))



eϕ(x − q(t)) ,

∇·E(x, t ) = eϕ(x − q(t)), ∇·B(x, t) = 0. (10.17)

122 Spinning charges

The momentum of the center of mass is m

v(t) and the angular momentum

relative to q(t) is

s = I

ω with I



xϕ(x)x

. (10.18)

Therefore Newton’s equations of motion for the translational degrees of freedom

become

v(t) =



xeϕ(x − q(t))



E(x, t) +



v(t) + ω(t) × (x − q(t))



× B(x, t)



(10.19)

and for the rotational degrees of freedom

ω(t) =



xeϕ(x − q(t))(x − q(t))



E(x, t) +



v(t) + ω(t) × (x − q(t))



× B(x, t)



. (10.20)

If in addition there are external forces acting on the charge, then E and B in

(10.19), (10.20) would have to be replaced by E + E

and B + B

,respectively.

The Abraham model of section 2.4 is obtained by formally setting ω(t) = 0.

Note that this is not consistent with Newton’s torque equation (10.20), since

˙ω(t) = 0, in general, even for ω(t) = 0.

The Abraham model with spin conserves the energy

E =



x(E

+ B

), (10.21)

the linear momentum

P = m

v +



x E × B , (10.22)

and in addition the total angular momentum

J = q × m

v + I

ω +



x x × (E × B). (10.23)

Of course, also the spinless Abraham model is invariant under rotations and there

must exist a correspondingly conserved quantity, only it does not have the standard

form of a total angular momentum, which from a somewhat different perspective

indicates that inner rotations must be included.

In the by now established tradition, we assume that the external forces are

slowly varying and want to derive in this adiabatic limit an effective equation of

motion for the particle including its spin. As a ﬁrst step of this program we have