Skiadas C.H., Dimotikalis I. (editors) Chaotic Systems: Theory and Applications
Подождите немного. Документ загружается.

18
1.
Awrejcewicz and G. Kudra
insight into dynamics
of
the real pendulum, the corresponding mathematical
model is required.
In
the work
[1]
the suitable mathematical modeling and
numerical analysis have been performed, where the viscous damping in the
pendulum joints (constructed by the use
of
rolling bearings) has been assumed.
In
the next step [2],
we
have also taken into account the dry friction in the joints
with many details and variants. Here
we
present the model
of
friction taking into
account only essential details.
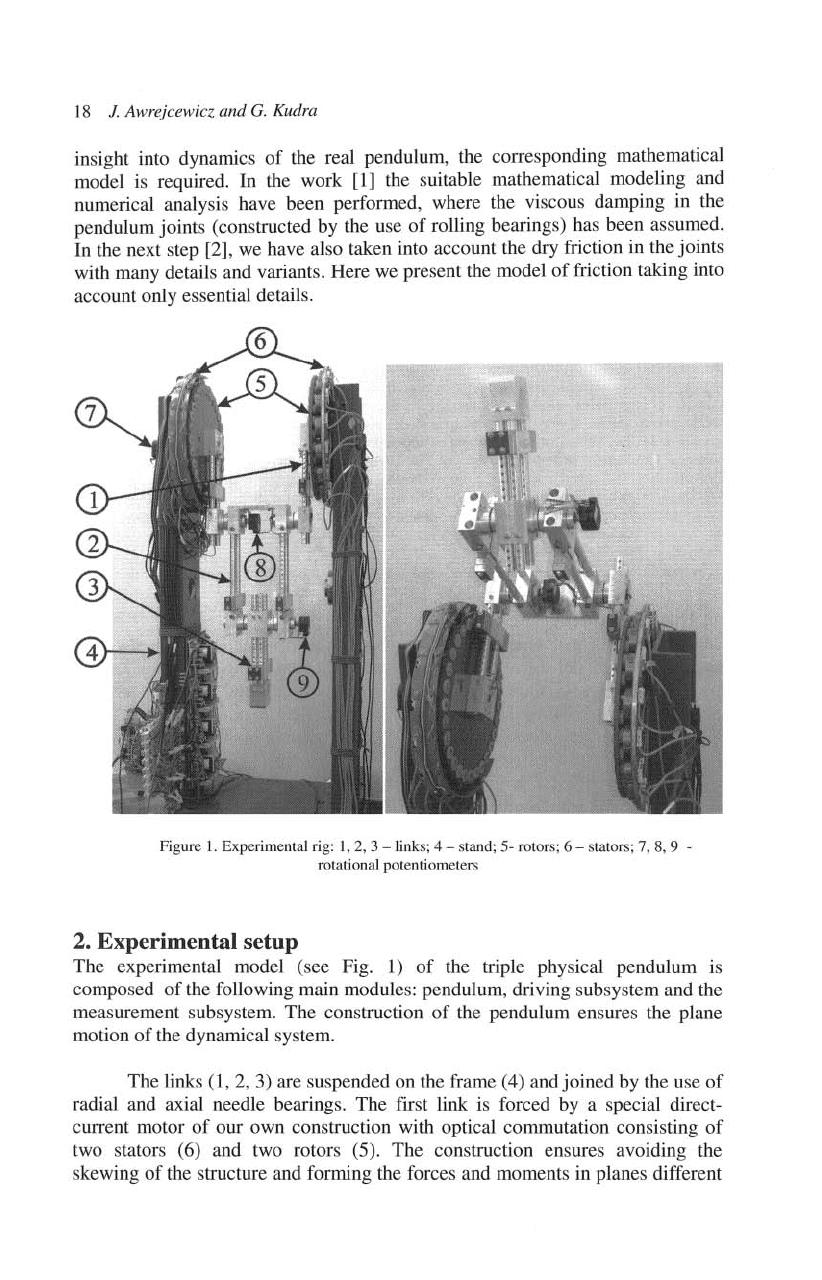
Figure
1.
Experimental rig: 1,
2,3
- links; 4 - stand; 5- rotors; 6 - stators; 7, 8, 9 -
rotational potentiometers
2. Experimental setup
The experimental model (see Fig.
1)
of
the triple physical pendulum is
composed
of
the following main modules: pendulum, drivin·g subsystem and the
measurement subsystem. The construction
of
the pendulum ensures the plane
motion
of
the dynamical system.
The links
(1
,
2,
3) are suspended on the frame (4) and joined by the use
of
radial and axial needle bearings. The fust link is forced by a special direct-
current motor
of
our own construction with optical commutation consisting
of
two stators (6) and two rotors (5). The construction ensures avoiding the
skewing
of
the structure and forming the forces and moments in planes different
Deterministic Chaos Machine
19
that the plane
of
the assumed pendulum motion. On the other hand the
construction allows the full rotations
of
all the links
of
the pendulum.
The voltage conveyed to the DC motor inductors is controlled by the use
of
special digital system
of
our own construction together with precise signal
generator HAMEG. As a result the square-shape in time
of
the voltage signal
(but with some asymmetry - see the next sections) with adjustable frequency
is
obtained. The measurement
of
the angular position
of
the three links is realized
by the use
of
the precise rotational potentiometers (7,
8,
9). Then the LabView
measure-programming system is used for experimental data acquisition and
presentation on a computer.
y
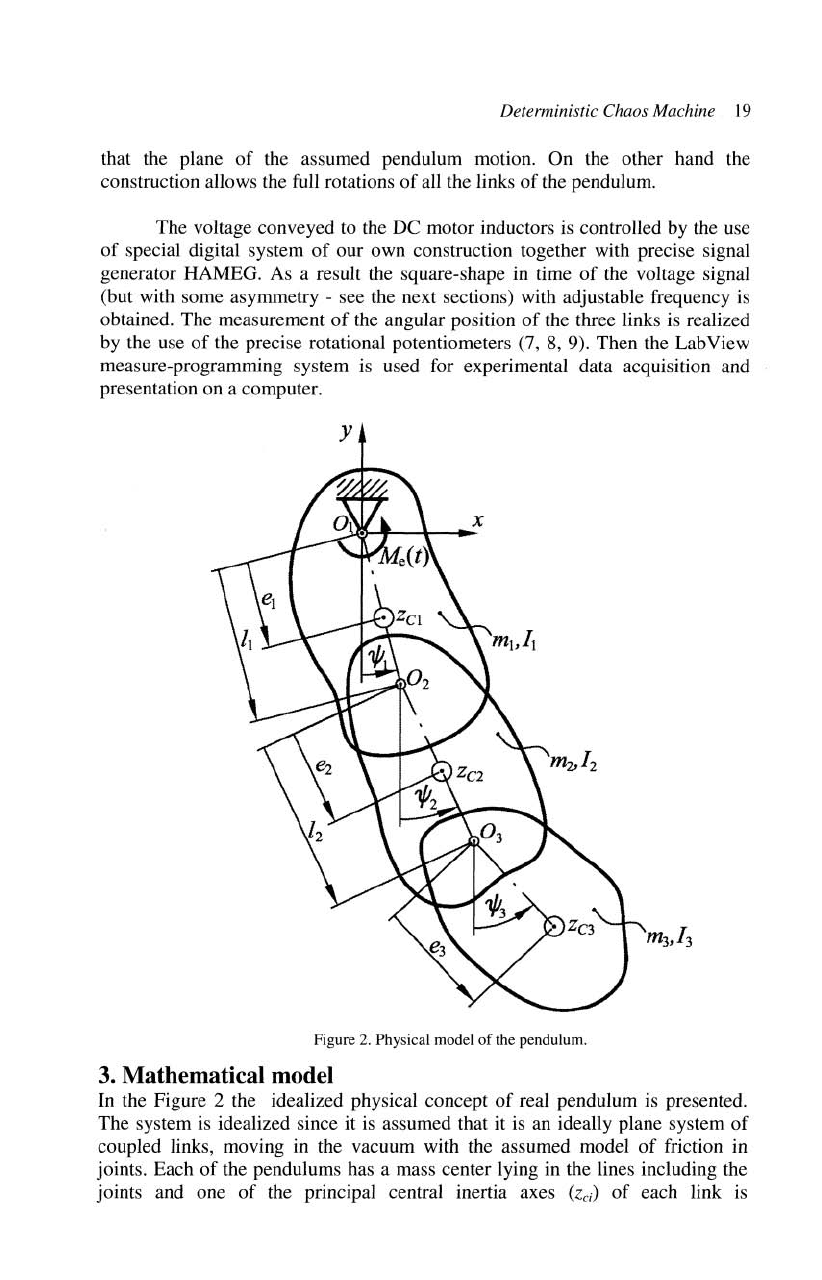
Figure
2.
Physical model
of
the pendulum.
3. Mathematical model
In the Figure 2 the idealized physical concept
of
real pendulum is presented.
The system is idealized since it is assumed that it is an ideally plane system
of
coupled links, moving in the vacuum with the assumed model
of
friction in
joints. Each
of
the pendulums has a mass center lying in the lines including the
joints and one
of
the principal central inertia axes
(Zei)
of
each link
is
20
1.
Awrejcewicz and
G.
Kudra
perpendicular to the movement plane. The system
is
governed by the following
set
of
differential equations:
where the pendulum position is described by the use
of
three angles
If/i
(i
=
1,2,
3),
Mit)
is
the external forcing
of
the first link,
MRi
(i
=
1,
2,
3) are friction
torques generated in the joints and where the following notation is used
M, =m,ge, +(m2
+m
3
)gl, '
M
2 = m
2
ge
2
+ m
3
g1
2
,
M3
= m
3
ge
3
,
B,
= I,
+e,2
m
,
+1,2
(m2
+m3)'
2 2
B2
=
12
+ e
2
m
2
+
12
m3
'
B3
=13
+e
3
2m
3 '
N'2
= m
2
e
2
1,
+ mi,12'
N'3
=
m3
e
3
1
, '
N
23
= m
3
e
3
1
2
,
where mj and
Ii
(i
=
1,
2,
3)
are the masses and inertia moments with respect to
the central axes perpendicular to the movement plane
of
the corresponding
links and
g is gravitational acceleration. Other geometrical parameters are seen
in the Figure
2.
Note that there
is
the following dependence between introduced
quantities

Deterministic Chaos
Ma
chine
21
and
The resistance torques in the pendulum joints are modeled with the
assumption that they are the functions
of
the relative velocities
of
the
corresponding links only (among others they are not dependent on the bearing
loadings):
M RI = I;
3..
arctan
(£I'ifl)
+
CI'if1
'
Jr
M
R2
=
T2
3..
arctan
(£2
('if2 -
'ifl))
+ C
2
('if2
-
'ifl)
,
Jr
M
R3
= T3
3..
arctan ( £ 3 ( 'if3 -
'if2
) ) + C
3
( 'if3 -
'if2
) ,
Jr
and they are composed
of
two parts: linear (viscous) part and nonlinear part in
the form
of
arctan
function
as
seen above. The
arctan
(or
tanh)
function is
usually used in modeling
of
dry friction with high value
of
the c parameter and
its classical objective is to obtain a smooth approximation
of
the sign function.
Although our initial goal was just a smooth approximation
of
sign function, the
parameter estimation results (see the next section) have been shown, that smaller
values
of
the c parameter can give better results
of
modeling
of
the investigated
triple pendulum.
The external input to the system
is
the voltage signal u controlling the
direction
of
the constant voltage applied to the DC motor
of
the pendulum. The
signal u in the pendulum model can be an arbitrary function
of
time, and
in
particular, it can be the same function as applied (and recorded
in
a file) in real
system (it
is
useful
in
the parameter estimation process). On the other hand, it is
possible to apply the signal u due to the following mathematical description:
U(f)={1
-I
for
mod
(
lOt
+ 90'
2Jr)
::;
2Jr a
for mod(lOt+90,2Jr»2Jra'
which imitates the signal applied
in
the real pendulum, with adjustable angular
velocity
w, initial phase
Wo
and coefficient a (in the investigated experimental
pendulum there is a = 0.4927 which means an asymmetry in the forcing, as
mentioned in section 2).

22
1.
Awrejcewicz and
C.
Kudra
The relation between the control signal u and torque Me acting on the first
link
is
modeled by the following equation
Me
(t)
+
TM
dM
e = q U
(t)
,
dt
where q is the amplitude
of
the external torque corresponding to the constant
voltage applied in DC motor and
T M
is
the time-constant
of
the DC motor.
4. Parameter estimation and experimental vs. numerical
results
Although some parameters
of
the system (for example masses, some
geometrical lengths) can be obtained from direct measurements or calculated
from such measurements, it can be difficult, labour-consuming or inaccurate for
some
of
them. Because
of
that we have used only values that can be got easily
and accurately, that is g
= 9.812
rnIs
2
,
II
= 0.17418 m and
12
= 0.22405
m.
Other
model parameters are obtained from identification process, that is from
experimental data
of
input and output signals, where the input signal is the
voltage signal
u controlling the direction
of
the constant voltage applied to the
DC motor
of
the pendulum and the outputs are the angular positions
of
the
pendulum links
lfIi
(i =
1,
2,
3). One can also note that some other special
experiments might be performed in order to identify particular model
parameters, but here we assume that only above mentioned experimental data
from the triple pendulum is available.
The model parameters are estimated by the global minimum searching
of
the error-function
of
the model and real system matching. The matching
of
model and real system is understood as the matching
of
the corresponding
output signals
lfIi
(i
=
1,
2,
3) from model integrated numerically and from the
real pendulum, assuming the same inputs to both the model and real system. The
sum
of
squares
of
deviations between corresponding samples
of
signals from
model and experiment, for few different solutions, serves as a criterion function.
A minimum
is
searched by applying the simplex method. In order to avoid the
local minima, the simplex method is stopped from time to time and a random
searching
is
then applied. After random searching the simplex method is
restarted again.
If
we divide final value
of
criterion-function by the number
of
samples used
in
calculation
of
the error -function,
we
obtain average square
of
deviation between two signals (obtained from the model and the experiment) -
let
us
denote this parameter as
Fer-
Now this parameter can be used for
comparison
of
matching
of
different sets
of
experimental data and corresponding
numerical solutions.
Deterministic Chaos Machine
23
Since the amplitude
of
the forcing is assumed to be known before
identification process
(q = 1.718 Nm), the full vector
of
the parameters to be
estimated is
and other parameters are dependent since
and
0.2
I
0.103
0.1
~
0.05
eel
~
0
--------
--l
r
....
_
...................
_
...................
........
\
..
-
..
-
,-<
-0.05
-~
-0.1
-0.15
J .
f
. \
~
\..
I
-0.2
o
0.2
0.4 0.6 0.8
1.2
t
[s]
experiment
simulation
forcing
1.5
,----,---,--,---,----,-"-------,
~
0.5
eel
~
0
""
.~
-0.5
-1
-1..5
0
0.2 0.4 0.6 0.8
1.2
t
[]
experiment
S
simulation
forcing
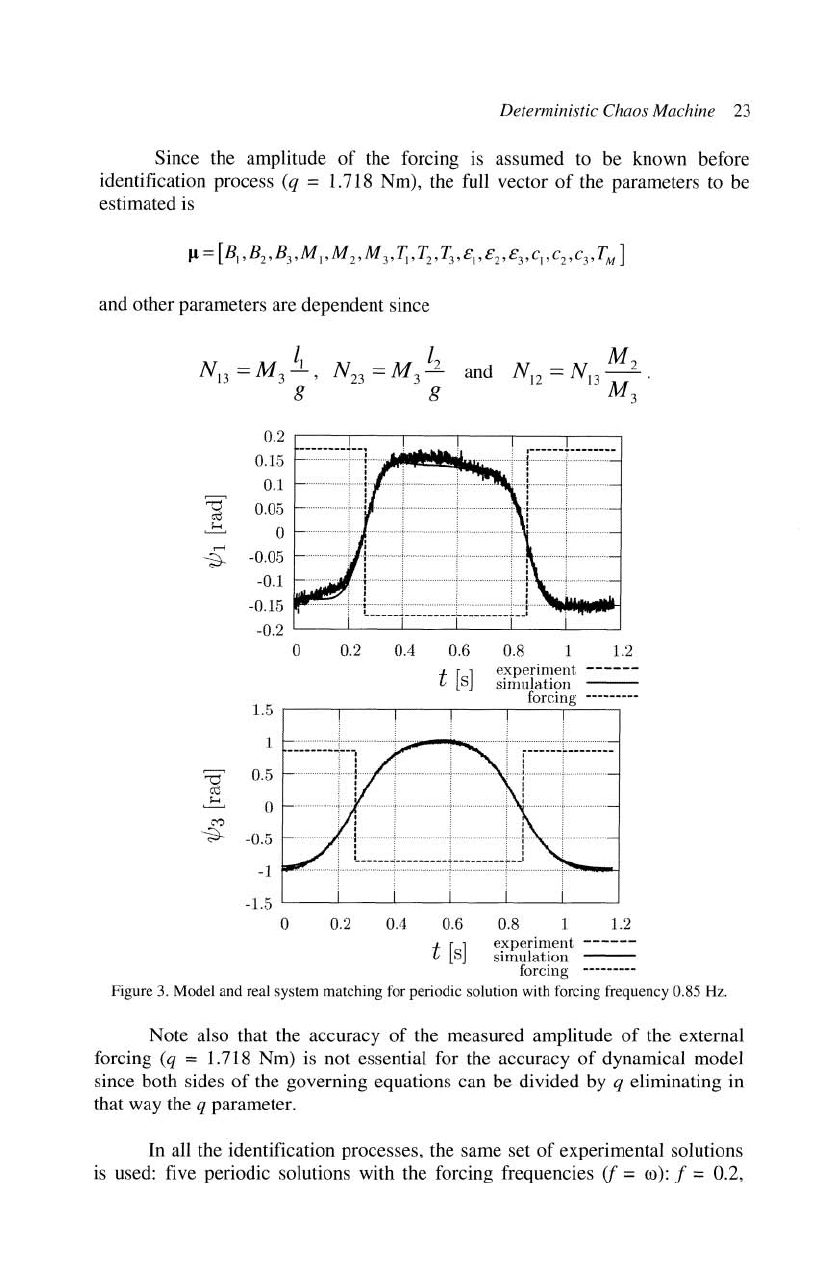
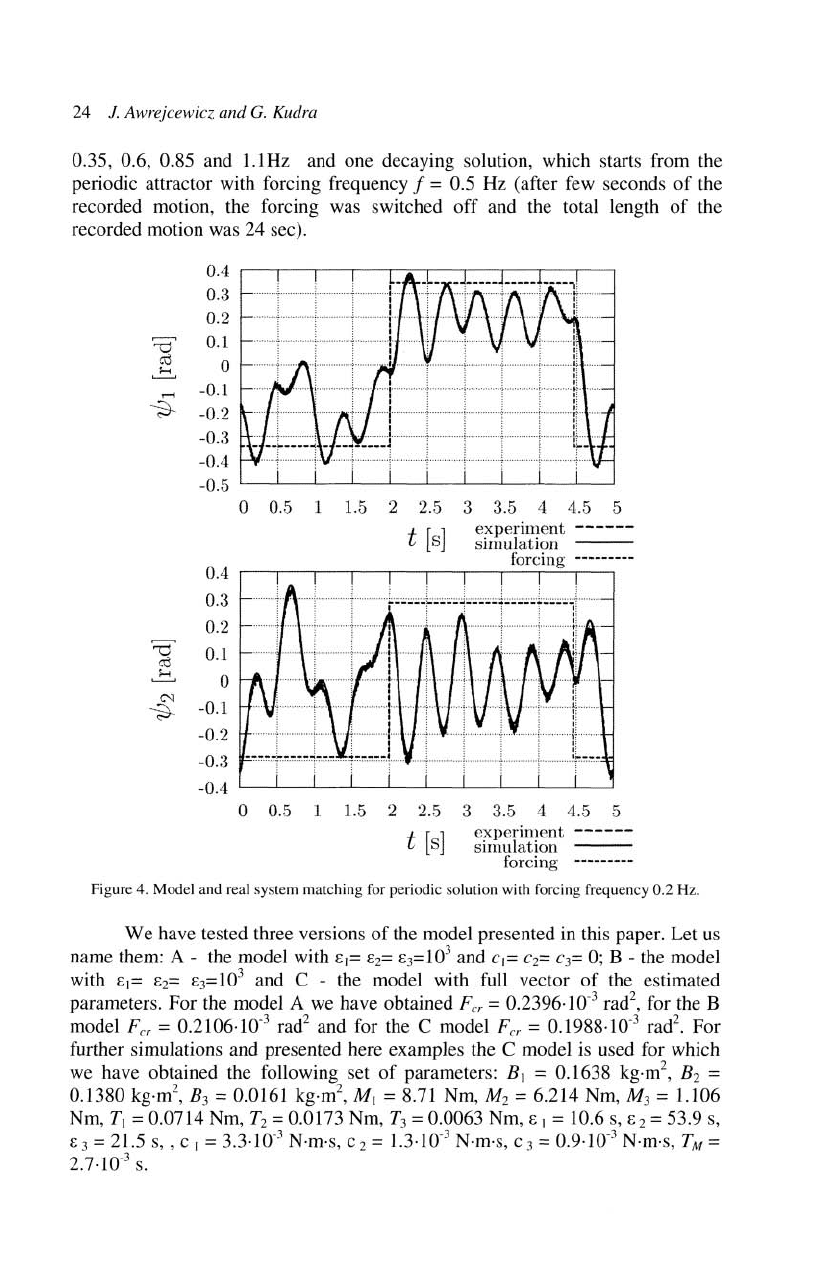
Figure
3.
Model and real system matching for periodic solution with forcing frequency 0.85 Hz.
Note also that the accuracy
of
the measured amplitude
of
the external
forcing (q = 1.718 Nm) is not essential for the accuracy
of
dynamical model
since both sides
of
the governing equations can be divided by q eliminating in
that way the q parameter.
In
all the identification processes, the same set
of
experimental solutions
is used: five periodic solutions with the forcing frequencies
(j
=
co):
f = 0.2,
24
1.
Awrejcewicz and
G.
Kudra
0.35, 0.6, 0.85 and
l.IHz
and one decaying solution, which starts from the
periodic attractor with forcing frequency
f = 0.5 Hz (after few seconds
of
the
recorded motion, the forcing was switched off and the total length
of
the
recorded motion was 24 sec).
0.4
0.3
0.2
~
0.1
cd
0
~
...-<
-0.1
~
-0.2
-0.3
-0.4
-0.5
0.4
0.3
0.2
~
0.1
cc
~
0
C'1
-0.1
~
-0.2
-0.3
-0.4
0 0.5
1
0
0.
5
1.5 2 2.5 3 3.5 4 4.5 5
t
[sJ
experiment
simula
tion
forcing
---------
1.5 2 2.5 3 3.5 4 4.5 5
t
[SJ
experiment
simulation
forcing
---------
Figure 4. Model and real system matching for periodic solution with forcing frequency 0.2
Hz.
We
have tested three versions
of
the model presented in this paper. Let
us
name them: A - the model with
£1= £2=
£3=10
3
and
CI=
C2=
C3=
0;
B - the model
with
£1=
£2=
£3=10
3
and C - the model with full vector
of
the estimated
parameters. For the model A we have obtained
Fer
= 0.2396.10-
3
rad
2
, for the B
model Fcr =
0.2106.10-
3
rad
2
and for the C model
Fe
r = 0.1988.10-
3
rad
l
.
For
further simulations and presented here examples the C model is used for which
we have obtained the following set
of
parameters: BI = 0.1638 kg·m
l
,
Bl
=
0.1380 kg·m
2
,
B3
= 0.0161 kg·m
2
,
MI
= 8.71 Nm,
M2
= 6.214 Nm,
M3
= 1.106
Nm,
TI
= 0.0714 Nm,
T2
= 0.0173 Nm,
T3
= 0.0063 Nm, £ I = 10.6
s,
£ 2 = 53.9
s,
£ 3 =
2l.5
s,
, c I = 3.3.10-
3
N·m·s, c 2 = l.3·10-
3
N·m·s, c 3 = 0.9.10-
3
N·m·s, T M =
2.
7·1O-
3
s.
Deterministic Chaos Machine
25
5
00
500
400
400
300 300
200 200
1/)3
100
100
0
-lOO
-100
-200
-21l0
<100
-:lOO
- .lrr
·
17r
8"
1.
2rr
0
10
20
30
40
.50
60
l!h
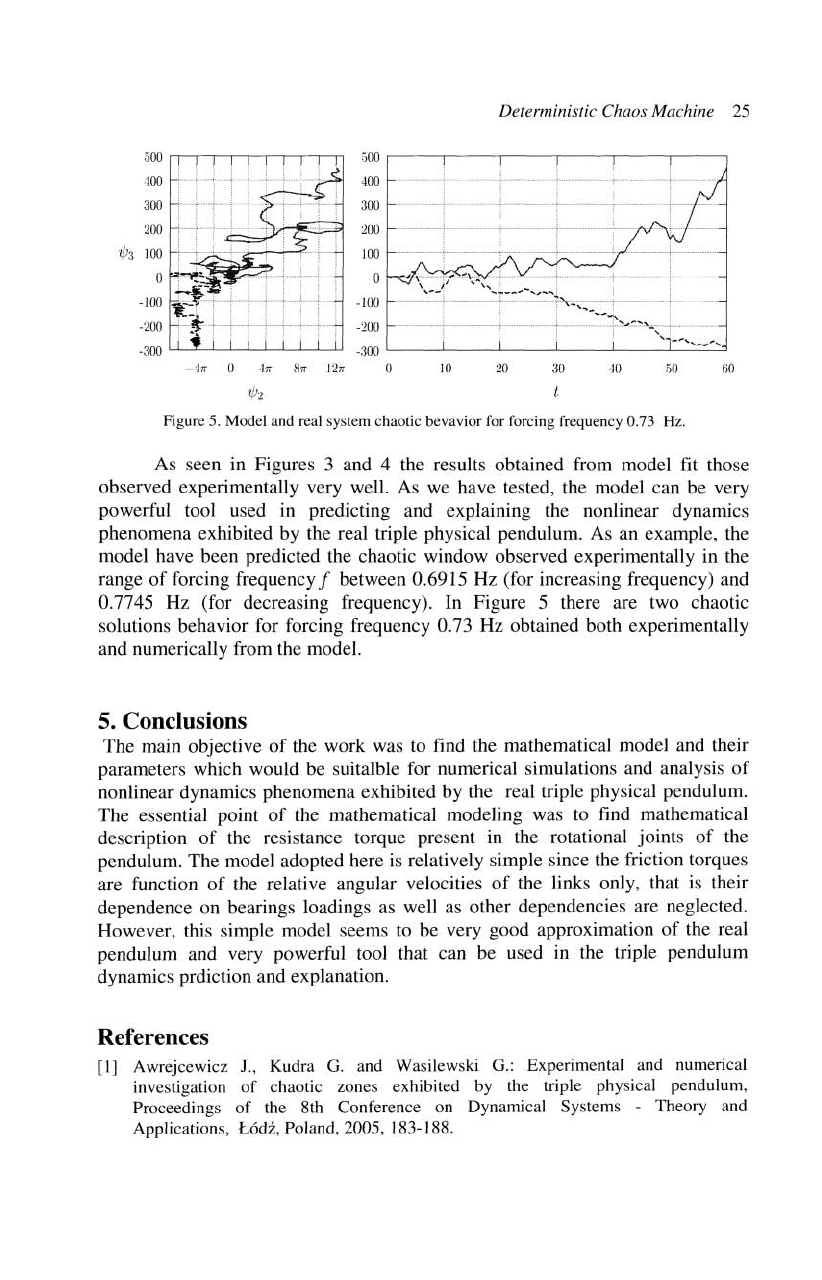
Figure
5.
Model and real system chaotic be
va
vi
or for forcing frequency 0.73 Hz.
As seen in Figures 3 and 4 the results obtained from model fit those
observed experimentally very well.
As
we have tested, the model can be very
powerful tool used in predicting and explaining the nonlinear dynamics
phenomena exhibited by the real triple physical pendulum. As an example, the
model have been predicted the chaotic window observed experimentally in the
range
of
forcing frequency f between 0.6915 Hz (for increasing frequency) and
0.7745 Hz (for decreasing frequency).
In
Figure 5 there are two chaotic
solutions behavior for forcing frequency 0.73 Hz obtained both experimentally
and numerically from the model.
5. Conclusions
The main objective
of
the work was
to
find the mathematical model and their
parameters which would be suitalble for numerical simulations and analysis
of
nonlinear dynamics phenomena exhibited by the real triple physical pendulum.
The essential point
of
the mathematical modeling was to find mathematical
description
of
the resistance torque present in the rotational joints
of
the
pendulum. The model adopted here is relatively simple since the friction torques
are function
of
the relative angular velocities
of
the links only, that
is
their
dependence on bearings loadings as well as other dependencies are neglected.
However, this simple model seems to be very good approximation
of
the real
pendulum and very powerful tool that can be used in the triple pendulum
dynamics prdiction and explanation.
References
[I] Awrejcewicz J., Kudra G. and Wasilewski G.: Experimental and numerical
investigation
of
chaotic zones exhibited by the triple physical pendulum,
Proceedings
of
the 8th Conference on Dynamical Systems - Theory and
Applications,
L6dz, Poland, 2005, 183-188.

26
1.
Awrejcewicz
and
G.
Kudra
[2] Awrejcewicz J., Supel, Kudra G., Wasilewski G. and Olejnik P.: Numerical and
experimental study
of
regular and chaotic motion
of
triple physical pendulum,
International Journal
of
Bifurcation and Chaos, 18(10), 2008
[3] Galan J
.,
Fras
er
W.B
.,
Acheson
OJ.
and Champneys A.R.: The parametrically
excited upside-down rod: an elastic jointed pendulum model, Journal
of
Sound and
Vibration,
280, 200S, 3S9-377.
[4]
Baker G.
L.
and Blackburn J.A.: The Pendulum. A Case Study in Physics, Oxford
University
Press, 200S.
[S]
Bishop S.R. and Sudor D.J
.:
The
"not
quite" inverted pendulum, International
Journal
of
Bifurcation and Chaos, 9(1), 1998, 273-28S.
[6] Blackburn J.A., Zhou-Jing Y., Vik S., Smith H.J.T. and Nerenberg M.A.H.:
Experimental study
of
chaos in a driven pendulum, Physica,
026(1-3),
1987, 38S-
39S.
[7]
Zhu Q. and Ishitobi M.: Experimental study
of
chaos in a driven triple pendulum,
Journal
of
Sound and Vibration,
227(1),1999
, 230-238.

Some
Issues
and
Results
on
the
EnKF
and
Particle
Filters
for
Meteorological
Models
Christophe
Baehr
1
,2
and
Olivier
Pannekoucke
1
1 Meteo-France / CNRS
CNRM
/ GAME URA1357
42
Avenue
G.
Coriolis, 31057 Toulouse Cedex
1,
France
(e-mail:
christophe.
baehr@meteo.fr,
olivier
.pannekoucke@meteo.fr)
2 Universite de Toulouse Paul Sabatier
Institut
de Mathematiques
118
route de Narbonne, 31062 Toulouse Cedex
9,
France
27
Abstract:
In this paper
we
examine the links between Ensemble Kalman Filters
(EnKF) and Particle Filters (PF).
EnKF
can be seen as a mean-field process with
a
PF
approximation.
We
explore
the
problem of dimensionality on a toy model.
To by-pass this difficulty,
we
suggest using Local Particle Filters (LPF)
to
catch
nonlinearities and feed larger scale EnKF.
To
go
one step forward
we
conclude with a
real application and present the filtering of
perturbed
measurements of atmospheric
wind in the domain of turbulence. This example
is
the cornerstone of
the
LPF
for
the assimilation of atmospheric turbulent wind. These local representation
techniques will be used in further works
to
assimilate singular
data
of turbulence
linked parameters in non-hydrostatic models.
Keywords:
Ensemble Kalman Filter, Particle Filter,
data
assimilation, mean-field
process.
1
Introduction
The
major
problems
in
data
assimilation
for
geophysical
models
come
from
nonlinearity
of
dynamics,
non-gaussianity
of
perturbations
and
high
dimen-
sions
of
state
space.
Ensemble
Kalman
Filters
(EnKF)
was
a first
answer
to
these
difficulties.
For
a few
years
some
authors
have
tried
to
use
Particle
Filters
(PF)
roughly
to
propose
an
alternative
strategy.
But
directly
applied
this
new
approach
stumbles
across
the
problem
of
the
dimensionality.
In
this
paper,
we
present
the
links
between
EnKF
and
PF,
we also
remind
that
the
EnKF
converges
but
tends
to
a
particular
process
and
we
describe
the
dynamical
system
of
the
nonlinear
filter
distribution.
In
the
case
of
the
PF,
with
a modified
selection
step,
we
investigate
the
effect
of
an
increasing
state
space
dimension
for a
constant
number
of
particles.
Then
we
suggest
to
cou-
ple
EnKF
and
Local
Particle
Filter
(LPF)
to
propose
solutions
in
the
vicinity
of
strong
uncertainties.
The
next
step
is
the
use
of
LPF
with
a
stochastic
representation
of
the
medium
and
we
present
some
results
on
the
filtering
