Povh B., Rith K., Scholz Ch., Zetsche F. Particles and Nuclei: An Introduction to the Physical Concepts
Подождите немного. Документ загружается.

Solutions to Chapter 13 373
Chapter 11
1. The total width Γ
tot
of Z
0
may be written as
Γ
tot
= Γ
had
+3Γ
+ N
ν
Γ
ν
and Γ
ν
/Γ
=1.99 (see text). From (11.9) it follows that
σ
max
had
=
12π(c)
2
M
2
Z
Γ
e
Γ
had
Γ
tot
.
Solving for Γ
tot
and inserting it into the above formula yields from the
experimental results N
ν
=2.96. Varying the experimental results inside
the errors only changes the calculated value of N
ν
by about ±0.1.
Chapter 13
1. The reduced mass of positronium is m
e
/2. From (13.4) we thus find the
ground state (n = 1) radius to be
a
0
=
2
αm
e
c
=1.1 · 10
−10
m .
The range of the weak force may be estimated from Heisenberg’s uncer-
tainty relation:
R ≈
M
W
c
=2.5 · 10
−3
fm.
At this separation the weak and electromagnetic couplings are of the same
order of magnitude. The masses of the two particles, whose bound state
would have the Bohr radius R,wouldthenbe
M ≈
2
αRc
≈ 2 · 10
4
GeV/c
2
.
This is equivalent to the mass of 4 · 10
7
electrons or 2 · 10
4
protons. This
vividly shows just how weak the weak force is.
2. From (18.1) the transition probability obeys 1/τ ∝ E
3
γ
|r
fi
|
2
.Ifm is the
reduced mass of the atomic system, we have |r
fi
| ∝ 1/m and E
γ
∝ m.
1/τ = m/m
e
· 1/τ
H
implies τ = τ
H
/940 for protonium.
3. The transition frequency in positronium f
e
+
e
−
is given by
f
e
+
e
−
f
H
=
7
4
g
e
g
p
m
p
m
e
|ψ(0)|
2
e
+
e
−
|ψ(0)|
2
H
Using (13.4) one finds |ψ(0)|
2
∝ m
3
red
=[(m
1
· m
2
)/(m
1
+ m
2
)]
3
.Oneso
obtains f
e
+
e
−
= 204.5 GHz. One can analogously find f
µ
+
e
−
=4.579 GHz.
374 Solutions to Chapter 14
The deviations from the measured values (0.5 % and 2.6 % respectively)
are due to higher order QED corrections to the level splitting. These are
suppressed by a factor of the order α ≈ 0.007.
4. a) The average decay length is s = vτ
lab
= cβγτ where γ = E
B
/m
B
c
2
=
0.5 m
Υ
/m
B
and βγ =
γ
2
− 1. One thus obtains s =0.028 mm.
b) From 0.2mm = cβγτ = τ ·|p
B
|/m
B
we obtain |p
B
| =2.3 GeV/c.
c) From the assumption, m
B
=5.29 GeV/c
2
= m
Υ
/2, the B-mesons do
not have any momentum in the centre of mass frame. In the laboratory
frame, |p
B
| =2.3 GeV/c and thus |p
Υ
| =2|p
B
|. We obtain from this
E
Υ
=
m
2
Υ
c
4
+ p
2
Υ
c
4
=11.6 GeV.
d) From four-momentum conservation p
Υ
= p
e
+
+ p
e
−
we obtain (setting
m
e
=0)E
Υ
= E
e
+
+ E
e
−
and p
Υ
c = E
e
+
+ E
e
−
from this we get
E
e
+
=8.12 GeV and E
e
−
=3.44 GeV (or vice-versa).
Chapter 14
1. Angular momentum conservation requires = 1, since pions are spin 0. In
the ( = 1) state, the wave function is antisymmetric, but two identical
bosons must have a totally symmetric wave function.
2. The branch in the denominator is Cabibbo-suppressed and from (10.21)
we thus expect: R ≈ 20.
3. a) From the decay law N(t)=N
0
e
−t/τ
we obtain the fraction of the
decaying particles to be F =(N
0
− N )/N
0
=1−e
−t/τ
. In the laboratory
frame we have t
lab
= d/(βc)andτ
lab
= γτ
∗
,whereτ
∗
is the usual
lifetime in the rest frame of the particle. We thus obtain
F =1− exp
−
d
βcγτ
∗
=1− exp
⎛
⎝
−
d
1 −
m
2
c
4
E
2
c
E
mc
2
τ
∗
⎞
⎠
,
and from this we find F
π
=0.9 % und F
K
=6.7%.
b) From four momentum conservation we obtain, e.g., for pion decay p
2
µ
=
(p
π
− p
ν
)
2
and upon solving for the neutrino energy get
E
ν
=
m
2
π
c
4
− m
2
µ
c
4
2(E
π
−|p
π
|c cos θ)
.
At cos θ = 1 we have maximal E
ν
, while for cos θ = −1 it is minimal.
We can so obtain E
max
ν
≈ 87.5 GeV and E
min
ν
≈ 0 GeV (more precisely:
11 keV) in pion decay. In the case of kaon decay, we obtain E
max
ν
≈
191 GeV and E
min
ν
≈ 0 GeV (more precisely: 291 keV).
Solutions to Chapter 15 375
Chapter 15
1. b) All of the neutral mesons made out of u- and d-quarks (and similarly
the s
s(φ)meson)areveryshortlived;cτ < 100 nm. The dilatation
factor γ that they would need to have in order to traverse a distance of
several centimetres in the laboratory frame is simply not available at
these beam energies. Since mesons with heavy quarks (c, b) cannot be
produced, as not enough energy is available, the only possible mesonic
decay candidate is the K
0
S
. Similarly the only baryons that come into
question are the Λ
0
and the Λ
0
. The primary decay modes of these
particles are K
0
S
→ π
+
π
−
,Λ
0
→ pπ
−
and Λ
0
→ pπ
+
.
c) We have for the mass M
X
of the decayed particle from (15.1)
M
2
X
= m
2
+
+ m
2
−
+2
p
2
+
/c
2
+ m
2
+
p
2
−
/c
2
+ m
2
−
−
2
c
2
|p
+
||p
−
|cos <) (p
+
, p
−
)
where the masses and momenta of the decay products are denoted
by m
±
and p
±
respectively. Consider the first pair of decay products:
thehypothesisthatwehaveaK
0
S
→ π
+
π
−
(m
±
= m
π
±
) decay leads
to M
X
=0.32 GeV/c
2
which is inconsistent with the true K
0
mass
(0.498 GeV/c
2
). The hypothesis Λ
0
→ pπ
−
(m
+
= m
p
, m
−
= m
π
−
)
leads to M
X
=1.11 GeV/c
2
which is in very good agreement with the
mass of the Λ
0
.TheΛ
0
possibility can, as with the K
0
hypothesis, be
confidently excluded. Considering the second pair of decay particles
we similarly find: K
0
hypothesis, M
X
=0.49 GeV/c
2
;Λ
0
hypothesis,
M
X
=2.0 GeV/c
2
;theΛ
0
hypothesis also leads to a contradiction. In
this case we are dealing with the decay of a K
0
.
d) Conservation of strangeness in the strong interaction means that as
well as the Λ
0
, which is made up of a uds quark combination, a fur-
ther hadron with an
s-quark must be produced. The observed K
0
S
decay
means that this was a K
0
(sd).
1
Charge and baryon number conserva-
tion now combine to imply that the most likely total reaction was
p+p→ K
0
+Λ
0
+p+π
+
.
We cannot, however, exclude additional, unobserved neutral particles
or very short lived intermediate states (such as a ∆
++
).
2. Let us consider the positively charged Σ particles |Σ
+
= |u
↑
u
↑
s
↓
and
|Σ
+∗
= |u
↑
u
↑
s
↑
. Since the spins of the two u-quarks are parallel, we
have
3
i,j=1
i<j
σ
i
· σ
j
m
i
m
j
=
σ
u
· σ
u
m
2
u
+2
σ
u
· σ
s
m
u
m
s
.
1
Both the K
0
and the K
0
can decay as K
0
S
(cf. Sec. 14.4).

376 Solutions to Chapter 15
We first inspect
2 σ
u
· σ
s
=
3
i,j=1
i<j
σ
i
· σ
j
− σ
u
· σ
u
.
We already know the first term on the r.h.s. from (15.10). It is −3for
S =1/2 baryons and +3 for S =3/2 baryons. The second term is +1.
This yields
∆M
ss
=
⎧
⎪
⎪
⎪
⎪
⎪
⎨
⎪
⎪
⎪
⎪
⎪
⎩
4
9
3
c
3
πα
s
|ψ(0)|
2
)
1
m
2
u,d
−
4
m
u,d
m
s
*
for the Σ states ,
4
9
3
c
3
πα
s
|ψ(0)|
2
)
1
m
2
u,d
+
2
m
u,d
m
s
*
for the Σ
∗
states .
The average mass difference between the Σ and Σ
∗
baryons is about 200
MeV/c
2
. With the mass formula (15.12) we have
M
Σ
∗
− M
Σ
= ∆M
ss
(Σ
∗
) − ∆M
ss
(Σ) =
4
9
3
c
3
πα
s
|ψ(0)|
2
6
m
u,d
m
s
≈ 200 MeV/c
2
,
where we assume that ψ(0) is the same for both states. We thus obtain
(m
u,d
= 363 MeV/c
2
, m
s
= 538 MeV/c
2
)
α
s
|ψ(0)|
2
=0.61 fm
−3
.
Inserting a hydrogen atom-type wave function, |ψ(0)|
2
=3/4πr
3
,and
α
s
≈ 1, yields a rough approximation for the average separation r of the
quarks in such baryons: r ≈ 0.8fm.
3. The Λ is an isospin singlet (I = 0). To a first approximation the decay is
just the quark transition s → u, which changes the isospin by 1/2. Thus the
pion-nucleon system must be a I =1/2 state. Charge conservation implies
that the third component is I
N
3
+ I
π
3
= −1/2. The matrix elements of the
decay of the Λ
0
are proportional to the squares of the Clebsch-Gordan
coefficients:
σ(Λ
0
→ π
−
+p)
σ(Λ
0
→ π
0
+n)
=
(
1
1
2
−1 +
1
2
|
1
2
−
1
2
)
2
(1
1
2
0 −
1
2
|
1
2
−
1
2
)
2
=
(−
√
2/3)
2
(
√
1/3)
2
=2.
4. The probability that a muon be captured from a 1s state into a
12
C nucleus
is
1
τ
µC
=
2π
12
Be
ip
ν
r
i
g
A
σ
i
I
−
12
Cψ
µ
(r)
(r=0)
2
p
2
ν
dp
ν
dΩ
(2π)
3
dE
ν
.
Solutions to Chapter 15 377
Since carbon has J
P
=0
+
and boron J
P
=1
+
, this is a purely axial
vector transition. We further have dp
ν
/dE
ν
=1/c,
dΩ =4π and
|ψ
µ
(r =0)|
2
=3/(4πr
3
µ
). The radius of the
12
C muonic atomic is found to
be
r
µ
=
a
Bohr
m
e
Zm
µ
=42.3fm,
and the energy is
E
ν
= m
µ
c
2
− 13.3MeV≈ 90 MeV .
This yields the absorption probability
1
τ
µC
=
2π
c
4πcE
2
ν
(2π)
3
(c)
3
12
B
i
g
A
σ
i
I
−
12
C
2
|ψ(0)|
2
.
These are all known quantities except for the matrix element. This may
be extracted from the known lifetime of the
12
B →
12
C+e
−
+ ν
e
decay:
1
τ
12
B
=
1
2π
3
7
c
6
12
C
i
g
A
σ
i
I
+
12
B
2
E
5
max
.
We thus finally obtain
1
τ
µC
≈ 1.5 · 10
4
s
−1
.
The total decay probability of the muon decay in
12
C is the sum of the
probabilities of the free muon decaying and of its being captured by the
nucleus:
1
τ
=
1
τ
µ
+
1
τ
µC
.
5. These branching ratios depend primarily upon two things: a) the phase
space and b) the fact that the strangeness changes in the first case
(Cabibbo suppression) but not in the latter. A rough estimate may be
obtained by assuming that the matrix elements are, apart from Cabibbo
suppression, identical. From (10.21) and (15.49) one finds
W (Σ
−
→ n)
W (Σ
−
→ Λ
0
)
≈
sin
2
θ
C
cos
2
θ
C
·
E
1
E
2
5
=
1
20
·
257 MeV
81 MeV
5
≈ 16 .
This agreement is not bad at all, considering the coarseness of our approx-
imation. In the decay Σ
+
→ n+e
+
+ ν
e
we would need two quarks to
change their flavours; (suu) → (ddu).

378 Solutions to Chapter 16
6. a) Baryon number conservation means that baryons can neither be anni-
hilated nor created but rather only transformed into each other. Hence
only the relative parities of the baryons have any physical meaning.
b) The deuteron is a ground state p-n system, i.e., = 0. Its parity is
therefore η
d
= η
p
η
n
(−1)
0
= +1. Since quarks have zero orbital angular
momentum in nucleons, the quark intrinsic parities must be positive.
c) The downwards cascade of pions into the ground state may be seen
from the characteristic X rays.
d) Since the deuteron has spin 1, the d-π system is in a state with total
angular momentum J = 1. The two final state neutrons are identical
fermions and so must have an antisymmetric spin-orbit wave function.
Only
3
P
1
of the four possible states with J =1,
3
S
1
,
1
P
1
,
3
P
1
and
3
D
1
fulfills this requirement.
e) From
nn
= 1, we see that the pion parity must be η
π
= η
2
n
(−1)
1
/η
d
=
−1.
f) The number of quarks of each individual flavour (N
q
−N
q
)issepa-
rately conserved in parity conserving interactions. The quark pari-
ties can therefore be separately chosen. One could thus choose, e.g.,
η
u
= −1,η
d
= +1, giving the proton a positive and the neutron a neg-
ative parity. The deuteron would then have a negative parity and the
charged pions a positive one. The π
0
as a uu/dd mixed state would
though keep its negative parity. Particles like (π
+
,π
0
,π
−
)or(p, n)
although inside the same isospin multiplets would then have distinct
parities – a rather unhelpful convention. For η
n
= η
p
= −1, on the other
hand, isospin symmetry would be fulfilled. The parities of nucleons and
odd nuclei would then be the opposite of the standard convention, while
those of mesons and even nuclei would be unchanged. The Λ and Λ
c
parities are just those of the s- and c-quarks and may be chosen to be
positive.
Chapter 16
1. The ranges, λ ≈ c/mc
2
,are:1.4fm (1π), 0.7fm (2π), 0.3fm (, ω). Two
pion exchange with vacuum quantum numbers, J
P
=0
+
,I = 0, generates
a scalar potential which is responsible for nuclear binding. Because of its
negative parity, the pion is emitted with an angular momentum, =1.
The spin dependence of this component of the nuclear force is determined
by this. Similar properties hold for the and ω. The isospin dependence
is determined by the isospin of the exchange particle; I = 1 for the π and
and I = 0 for the ω. Since isospin is conserved in the strong interac-
tion, the isospin of interacting particles is coupled, just as is the case with
angular momentum.
2. Taking (16.1), (16.2) and (16.6) into account we obtain
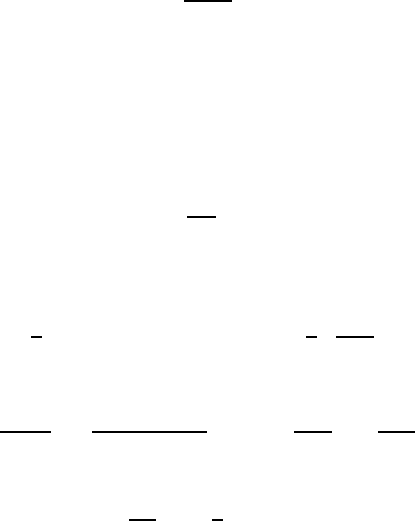
Solutions to Chapter 17 379
σ =4π
sin kb
k
2
.
At low energies, where the = 0 partial wave dominates, we obtain in the
k → 0 limit, the total cross-section, σ =4πb
2
.
Chapter 17
1. At constant entropy S the pressure obeys
p = −
∂U
∂V
S
,
where V is the volume and U is the internal energy of the system. In the
Fermi gas model we have from (17.9):
U =
3
5
AE
F
and hence p = −
3
5
A
∂E
F
∂V
.
From (17.3) we find for N = Z = A/2:
A =2
Vp
3
F
3π
2
3
=2
V (2ME
F
)
3/2
3π
2
3
=⇒
∂E
F
∂V
= −
2E
F
3V
.
The Fermi pressure is then
p =
2A
5V
E
F
=
2
5
N
E
F
,
where
N
is the nucleon density. This implies for
N
=0.17 nucleons/fm
3
and E
F
≈ 33 MeV
p =2.2MeV/fm
3
=3.6 · 10
27
bar.
2. a) We only consider the odd nucleons. The even ones are all paired off in
the ground state. The first excited state is produced either by (I) the
excitation of the unpaired nucleon into the next subshell or (II) by the
pairing of this nucleon with another which is excited from a lower lying
subshell.
7
3
Li
23
11
Na
33
16
S
41
21
Sc
83
36
Kr
93
41
Nb
Ground state 1p
1
3/2
1d
3
5/2
1d
1
3/2
1f
1
7/2
1g
−3
9/2
1g
1
9/2
Excited (I)1p
1
1/2
2s
1
1/2
(1f
1
7/2
)(2p
1
3/2
)(1g
1
7/2
)(1g
1
7/2
)
Excited (II)(1s
−1
1/2
)1p
−1
1/2
2s
−1
1/2
1d
−1
3/2
2p
−1
1/2
2p
−1
1/2
J
P
0
experiment 3/2
−
3/2
+
3/2
+
7/2
−
9/2
+
9/2
+
J
P
0
model 3/2
−
5/2
+
3/2
+
7/2
−
9/2
+
9/2
+
J
P
1
experiment 1/2
−
5/2
+
1/2
+
3/2
+
7/2
+
1/2
−
J
P
1
case (I)1/2
−
1/2
+
(7/2
−
)(3/2
−
)(7/2
+
)(7/2
+
)
J
P
1
case (II)(1/2
+
)1/2
−
1/2
+
3/2
+
1/2
−
1/2
−
380 Solutions to Chapter 17
Those states whose excitation would be beyond a “magic” boundary
are shown here in brackets. This requires a lot of energy and so is only
to be expected for higher excitations. As one sees, the predictive powers
of the shell model are good for those nuclei where the unfilled subshell
is only occupied by a single nucleon.
b) The (p-1p
1
3/2
;n-1p
1
3/2
)in
6
3
Li implies J
P
=0
+
, 1
+
, 2
+
, 3
+
.
40
19
K has from
(p-1d
−1
3/2
;n-1f
1
7/2
) a possible coupling to 2
−
, 3
−
, 4
−
, 5
−
.
3. a) An
17
O nucleus may be viewed as being an
16
O nucleus with an ad-
ditional neutron in the 1f
5/2
shell. The energy of this level is thus
B(
16
O) − B(
17
O). The 1p
1/2
shell is correspondingly at B(
15
O) −
B(
16
O). The gap between the shells is thus
E(1f
5/2
) − E(1p
1/2
)=2B(
16
O) − B(
15
O) − B(
17
O) = 11.5MeV.
b) One would expect the lowest excitation level with the “right” quantum
numbers to be produced by exciting a nucleon from the topmost, occu-
pied shell into the one above. For
16
O this would be the J
P
=3
−
state,
whichisat6.13 MeV, and could be interpreted as (1p
−1
1/2
, 1d
5/2
). The
excitation energy is, however, significantly smaller than the theoretical
result of 11.5 MeV. It seems that collective effects (state mixing) are
making themselves felt. This is confirmed by the octupole radiation
transition probability, which is an order of magnitude above what one
would expect for a single particle excitation.
c) The 1/2
+
quantum numbers make it natural to interpret the first ex-
cited state of
17
Oas2s
1/2
. The excitation energy is then the gap be-
tween the shells.
d) Assuming (more than a little naively) that the nuclei are homogenous
spheres with identical radii, one finds from (2.11) that the difference
in the binding energies implies the radius is (3/5) · 16αc/3.54 MeV =
3.90 fm, which is much larger than the value of 3.1 fm, which follows
from (5.56). In the shell model one may interpret each of these nuclei
as an
16
O nucleus with an additional nucleon. The valence nucleon in
the d
5/2
shell thus has a larger radius than one would expect from the
above simple formula which does not take shell effects into account.
e) The larger Coulomb repulsion means that the potential well felt by
the protons in
17
F is shallower than that of the neutrons in
17
O. As
a result the wave function of the excited, “additional” proton in
17
F
is more spread out than that of the equivalent “additional” neutron
in
17
O and the nuclear force felt by the neutron is stronger than that
acting upon the proton. This difference is negligible for the ground state
since the nucleon is more strongly bound.
4. At the upper edge of the closed shells which correspond to the magic
numbers 50 and 82 we find the closely adjacent 2p
1/2
,1g
9/2
and the 2d
3/2
,
1h
11/2
,3s
1/2
levels respectively. It is thus natural that for nuclei with

Solutions to Chapter 17 381
nucleon numbers just below 50 or 82 the transition between the ground
state and the first excited state is a single particle transition (g
9/2
↔ p
1/2
andr h
11/2
↔ d
3/2
, s
1/2
respectively). Such processes are 5th order (M4 or
E5) and hence extremely unlikely [Go51].
5. a) The spin of the state is given by the combination of the unpaired nu-
cleons which are in the (p-1f
7/2
,n-1f
7/2
) state.
b) The nuclear magnetic moment is just the sum of the magnetic moments
of the neutron in the f
7/2
shell −1.91 µ
N
and of the proton in the f
7/2
shell +5.58 µ
N
. From (17.36) we would expect a g factor of 1.1.
6. a) In the de-excitation i → f of an Sm nucleus at rest the atom receives
a recoil energy of p
2
Sm
/2M where |p
Sm
| = |p
γ
|≈(E
i
− E
f
)/c.Inthe
case at hand this is 3.3eV.Thesameamountofenergyislostwhen
the photon is absorbed by another Sm nucleus.
b) If we set the matrix element in (18.1) to one, this implies a lifetime
of τ =0.008 ps, which is equivalent to Γ = 80 meV. In actual mea-
surements one finds τ =0.03 ps, i.e., Γ = 20 meV [Le78], which is
of a similar size. Since the width of the state is much smaller than
the energy shift of 2 · 3.3 eV, no absorption can take place. Thermal
motion will change |p
Sm
| by roughly ±
√
M · kT . At room temperature
this corresponds to smearing the energy by ±0.35 eV, which is also
insufficient.
c) If the Sm atom emits a neutrino before the deexcitation, then |p
Sm
| is
changed by ±|p
ν
| = ±E
ν
/c. If the emission directions of the neutrino
and of the photon are opposite to each other, then the energy of the
radiated photon is 3.12 eV larger than the excitation energy E
i
− E
f
.
This corresponds to the classical Doppler effect. In this case resonant
fluorescence is possible for the γ radiation. The momentum direction of
the neutrino can be determined in this fashion (for details, see [Bo72]).
7. The three lowest proton shells in the
14
O nucleus, the 1s
1/2
,1p
3/2
and
1p
1/2
, are fully occupied as are the two lowest neutron shells. The 1p
1/2
shell is, however, empty (sketched on p. 273). Thus one of the two valence
nucleons (one of the protons in their 1p
1/2
shell) can transform into a
neutron at the equivalent level and with the same wave function (super
allowed β-decay). We thus have
ψ
∗
n
ψ
p
=1.Thisisa0
+
→ 0
+
transition,
i.e., a pure Fermi decay. Each of the two protons contributes a term to the
matrix element equal to the vector part of (15.39). The total is therefore
|M
fi
|
2
=2g
2
V
/V
2
. Equation (15.47) now becomes
ln 2
t
1/2
=
1
τ
=
m
5
e
c
4
2π
3
7
· 2g
2
V
· f(E
0
) .
Using the vectorial coupling (15.56) one finds the half-life is 70.373 s –
which is remarkably close to the experimental value. Note: the quantum
numbers and definite shell structure here means that this is one of the few
382 Solutions to Chapter 18
cases where a nuclear β-decay can be calculated exactly. In practice this
decay is used to determine the strength of the vectorial coupling.
Chapter 18
1. a) In the collective model of giant resonances we consider Z protons and
N neutrons whose mutual vibrations are described by a harmonic os-
cillator. The Hamiltonian may be written as
H =
p
2
2m
+
mω
2
2
x
2
where ω =80A
−1/3
MeV,
and m = A/2M
N
is the reduced mass. The solution of the Schr¨odinger
equation yields the lowest lying oscillator states [Sc95]
ψ
0
=
1
4
√
π
√
x
0
· e
−(x/x
0
)
2
/2
where x
0
=
/mω,
ψ
1
=
1
4
√
π
√
x
0
·
√
2
x
x
0
e
−(x/x
0
)
2
/2
.
The average deviation is
x
01
:= ψ
0
|x|ψ
1
=
√
2
√
π
x
0
x
x
0
2
e
−(x/x
0
)
2
d
x
x
0
=
√
2
√
π
x
0
.
For
40
Ca we have x
0
=0.3fmand x
01
=0.24 fm.
b) The matrix element is Zx
01
. Its square is therefore 23 fm
2
.
c) The single particle excitations have about half the energy of the giant
resonance, i.e., ω ≈ 40A
−1/3
MeV. The reduced mass in this case is
approximately the nucleon mass, since the nucleon moves in the mean
field of the heavy nucleus. This increases x
0
, and thus x
01
,byafactor
of
√
40. The 24 nucleons in the outermost shell each contribute to the
square of the matrix element with an effective charge e/2. The square
of the matrix element is so seen to be 27.6fm
2
. The agreement with the
result of b), i.e., the model where the protons and neutrons oscillate
collectively, is very good.
2. See Sec. 17.4 (17.38ff) and (18.49f).
From
δ =
a − b
R
, R = ab
2
1/3
.
and the nucleon density, which in the nucleus is roughly
N
≈
0.17 nucleons/fm
3
, it follows that a ≈ 8fm, b ≈ 6fm.
