Poto?nik P. (Ed.) Natural Gas
Подождите немного. Документ загружается.

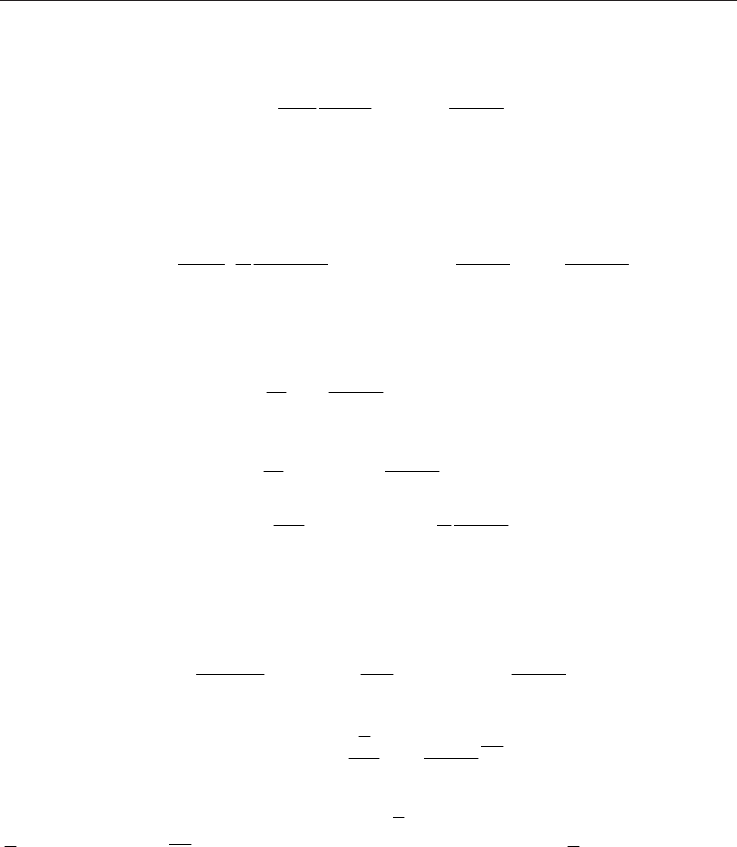
Rareed natural gas transport 541
2
2
2
2
2
2
2
3
1
y
u
yx
u
y
u
x
u
y
p
y
u
u
x
u
u
y
x
yy
r
y
y
y
x
(32)
The corresponding slip boundary conditions for a stationary unheated channel (Eq., 28) are
gw
x
m
m
sl
y
u
u
2
(33)
Here, we have neglected the body force for simplicity. By using a perturbation analysis
(Weng & Chen, 2008a), the momentum conservation equations (31) & (32) for the flow
through a sufficiently long channel can be reduced to the form:
2
2
y
u
dx
dp
x
r
(34)
Proceeding with the analysis, we introduce the following dimensionless parameters:
r
cr
r
coir
c
cc
y
c
x
cc
lTRlpp
l
p
p
P
u
u
V
u
u
U
l
y
Y
l
x
X
2/
ˆ
,We,Kn
,,,,,
2
2
(35)
where
We is the pressure drop from the entrance to the exit,
is a material constant, and
the subscript
c denotes the characteristic values. Here, the characteristic length
c
l , velocity
c
u , and pressure
c
p are, respectively, defined as
2
2
,,
cr
r
c
r
coi
cc
l
p
lpp
uwl
(36)
Substituting Eq. (35) into Eqs. (30), (33), (34) gives
0
Y
PV
X
PU
(37)
2
2
We
Y
U
dX
dP
(38)
gw
m
m
c
sl
Y
U
u
u
Kn
2
(39)
Solving momentum conservation equation (38) subject to the slip boundary conditions (39)
gives
)(Kn
2
)(
We2
1
),(
2
XYY
dX
XdP
YXU
m
m
(40)
Substituting Eq. (40) into mass conservation equation (37) and integrating once in
Y , we can
derive an equation for the cross-flow velocity:
Y
dX
XPd
XYY
dX
XPd
P
YXV
m
m
2
2
23
2
22
)(
)(Kn
2
1232
)(1
We24
1
),(
(41)
Evaluating this at
1Y , where V must vanish, we can derive an equation for the pressure:
01
2
2
12
1
CXCPP
m
m
(42)
where
We
2
1
We2We
12
1
,We
2
We
12
1
2
1
2
0
m
m
o
o
m
m
o
L
P
L
C
PPC
(43)
The corresponding mass flow rate is
m
m
o
ccc
w
x
P
L
UdYP
lup
dyup
M
2
62We
24
1
1
0
0
(44)
or
Kn
2
61
12
m
m
L
P
M
(45)
where
L
is the dimensionless channel length,
P
is the dimensionless average pressure,
2/
oi
PPP , and Kn is the average Knudsen number calculated at
P
.
4.2 Buoyancy-driven flow
If the driving mechanism is buoyancy, as shown in Fig. 2(b), then the wall temperature
w
T
is greater than the ambient temperature (that is,
01
TT and
0
TT
w
) and the discharge-area
pressure
1
p is equal to the reservoir pressure
0
p . Modeling the flow as a two-dimensional
steady incompressible flow, the field equations under the Boussinesq approximation
(Boussinesq, 1903) are given by (Eqs., 9, 14, 20)
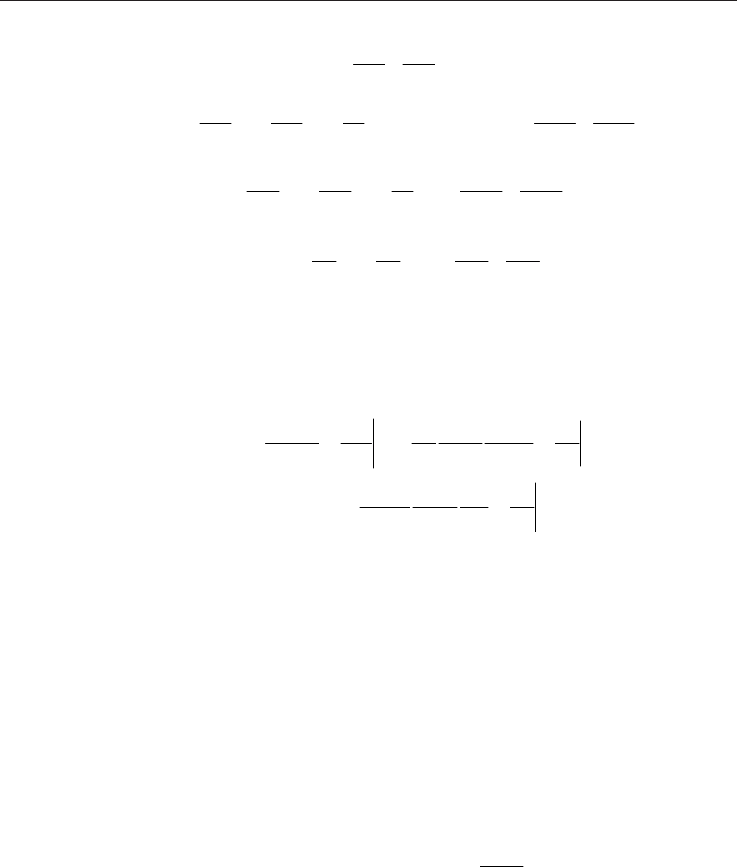
Natural Gas542
0
y
u
x
u
y
x
(46)
2
2
2
2
ˆ
y
u
x
u
TTg
x
p
y
u
u
x
u
u
xx
rrrr
x
y
x
xr
(47)
2
2
2
2
ˆ
y
u
x
u
y
p
y
u
u
x
u
u
yy
r
y
y
y
xr
(48)
2
2
2
2
y
T
x
T
k
y
T
u
x
T
uc
ryxprr
(49)
where
p
ˆ
is the pressure defect, related to p by
h
ppp
ˆ
, where
h
p is the hydrostatic
pressure. The corresponding slip and jump boundary conditions for a stationary
isothermally heated channel (Eqs., 28 & 29) are
gw
r
r
rpr
r
r
gw
x
r
m
m
sl
x
T
c
y
u
u
2
1
2
3
2
(50)
gw
r
rr
r
e
e
wju
y
T
TT
Pr
1
1
2
2
(51)
Here, we have neglected the internal heat generation for simplicity. It should be noted that
assuming a small temperature difference between the wall and the ambient gas supports the
constant-property assumption (Weng & Chen 2008b) and that considering the low-speed
flow of a low-Prandtl-number fluid supports the neglect of viscous dissipation in the energy
equation (Chen & Weng 2005; Weng & Chen 2008b).
We can think of the fully developed region as the flow section situated far from the entrance
such that
y
u is negligible. Based on this characterization, the mass conservation equation
(46) requires that
0/ xu
x
. In most treatments, 0
y
u and 0/ xu
x
are taken as a
starting point in the analysis of fully developed flow. The momentum conservation
equations (47) & (48) then become
2
2
0
dy
ud
TTg
x
rrrr
(52)
A solution of Eq. (52) in the form
)(Yu
x
is only possible if T is a function of y position
only, i.e.,
0/ XT . It implies that the assumption of a hydrodynamically fully developed
flow necessarily means that the flow is also thermally fully developed. The energy
conservation equations (49) and the slip boundary conditions (50) then be reduced to
2
2
0
dy
Td
(53)
gw
x
r
m
m
sl
y
u
u
2
(54)
Proceeding with the analysis, we introduce the following dimensionless parameters:
rw
r
c
x
cc
TT
TT
u
u
U
l
y
Y
l
x
X
,,,
(55)
Here, the characteristic length
c
l and velocity
c
u are, respectively, defined as
r
crwrr
cc
lTTg
uwl
2
,
(56)
Substituting Eq. (55) into Eqs. (51)–(54) gives
2
2
dY
Ud
(57)
0
2
2
dY
d
(58)
gw
r
m
m
c
sl
dY
dU
u
u
Kn
2
(59)
gw
r
rr
r
e
e
rw
rju
dY
d
TT
TT
Kn
Pr
1
1
2
2
1
(60)
Equations (57) & (58) subject to (59) & (60) have the following velocity and temperature
analytical solutions:
r
m
m
YYYU Kn
2
2
1
)(
2
(61)
1)(
Y
(62)
The corresponding mass flow rate is
,
6
1
Kn
2
2
1
1
0
0
m
m
cc
w
x
UdY
lu
dyu
M
(63)

Rareed natural gas transport 543
0
y
u
x
u
y
x
(46)
2
2
2
2
ˆ
y
u
x
u
TTg
x
p
y
u
u
x
u
u
xx
rrrr
x
y
x
xr
(47)
2
2
2
2
ˆ
y
u
x
u
y
p
y
u
u
x
u
u
yy
r
y
y
y
xr
(48)
2
2
2
2
y
T
x
T
k
y
T
u
x
T
uc
ryxprr
(49)
where
p
ˆ
is the pressure defect, related to p by
h
ppp
ˆ
, where
h
p is the hydrostatic
pressure. The corresponding slip and jump boundary conditions for a stationary
isothermally heated channel (Eqs., 28 & 29) are
gw
r
r
rpr
r
r
gw
x
r
m
m
sl
x
T
c
y
u
u
2
1
2
3
2
(50)
gw
r
rr
r
e
e
wju
y
T
TT
Pr
1
1
2
2
(51)
Here, we have neglected the internal heat generation for simplicity. It should be noted that
assuming a small temperature difference between the wall and the ambient gas supports the
constant-property assumption (Weng & Chen 2008b) and that considering the low-speed
flow of a low-Prandtl-number fluid supports the neglect of viscous dissipation in the energy
equation (Chen & Weng 2005; Weng & Chen 2008b).
We can think of the fully developed region as the flow section situated far from the entrance
such that
y
u is negligible. Based on this characterization, the mass conservation equation
(46) requires that
0/
xu
x
. In most treatments, 0
y
u and 0/
xu
x
are taken as a
starting point in the analysis of fully developed flow. The momentum conservation
equations (47) & (48) then become
2
2
0
dy
ud
TTg
x
rrrr
(52)
A solution of Eq. (52) in the form
)(Yu
x
is only possible if T is a function of y position
only, i.e.,
0/
XT . It implies that the assumption of a hydrodynamically fully developed
flow necessarily means that the flow is also thermally fully developed. The energy
conservation equations (49) and the slip boundary conditions (50) then be reduced to
2
2
0
dy
Td
(53)
gw
x
r
m
m
sl
y
u
u
2
(54)
Proceeding with the analysis, we introduce the following dimensionless parameters:
rw
r
c
x
cc
TT
TT
u
u
U
l
y
Y
l
x
X
,,,
(55)
Here, the characteristic length
c
l and velocity
c
u are, respectively, defined as
r
crwrr
cc
lTTg
uwl
2
,
(56)
Substituting Eq. (55) into Eqs. (51)–(54) gives
2
2
dY
Ud
(57)
0
2
2
dY
d
(58)
gw
r
m
m
c
sl
dY
dU
u
u
Kn
2
(59)
gw
r
rr
r
e
e
rw
rju
dY
d
TT
TT
Kn
Pr
1
1
2
2
1
(60)
Equations (57) & (58) subject to (59) & (60) have the following velocity and temperature
analytical solutions:
r
m
m
YYYU Kn
2
2
1
)(
2
(61)
1)( Y
(62)
The corresponding mass flow rate is
,
6
1
Kn
2
2
1
1
0
0
m
m
cc
w
x
UdY
lu
dyu
M
(63)

Natural Gas544
4.3 Thermocreep-driven flow
If the driving mechanism is thermocreep, as shown in Fig. 2(c), then the reservoir
temperature
0
T is less than the discharge-area pressure
1
T and the discharge-area pressure
1
p is equal to the reservoir pressure
0
p . Modeling the flow as a two-dimensional steady
incompressible constant-property flow, the momentum and energy equations under the
fully developed flow limit (
0
y
u and 0/ xu
x
) are given by (Eqs., 9, 14, 20)
2
2
0
dy
ud
dx
dp
x
r
(64)
2
2
2
dy
du
y
T
k
x
T
uc
x
rrxprr
(65)
Here, we have used the Prandtl boundary layer theory (Prandtl, 1904) to omit nonessential
terms shown in field equations. It should be noted that assuming a small temperature
difference between the reservoir and the discharge area supports the constant-property
assumption.
Proceeding with the analysis, we introduce the following dimensionless parameters:
01
2
2
3
01
2
01
Ec,Gr
,,,,,
Gr
TTc
ulTTg
p
p
P
TT
TT
u
u
U
l
y
Y
l
x
X
pr
c
r
crr
c
r
c
x
cc
(66)
Here, the characteristic length
c
l , velocity
c
u , and pressure
c
p are, respectively, defined as
2
2
01
,,
crc
r
crr
cc
up
lTTg
uwl
(67)
Substituting Eq. (66) into Eqs. (64), (65), and (50) gives
0
2
2
dY
Ud
dX
dP
(68)
2
2
2
EcPrPr
dY
dU
Y
X
U
rr
(69)
gw
r
r
r
gw
r
m
m
c
sl
XdY
dU
u
u
2
Kn
Ec
1
1
2
3
Kn
2
(70)
From Eqs. (68) and (70), a solution of Eq. (68) in the form
)(YU
is only possible if dXdP /
and
X / are constants (let
0
C and
1
C , respectively). The constant value in temperature
gradient implies that the flow under the assumption of hydrodynamically fully developed
flow is also thermally fully developed.
The momentum equation (68) can be integrated twice to obtain the streamwise velocity:
32
2
0
2
1
)(
CYCYCYU
(71)
Substituting Eq. (71) into the energy equation (69) and integrating the resultant with respect
to
Y twice and the thermally fully developed condition
1
/ CX
with respect to
X
once, we obtain the temperature:
.
2
1
3
1
12
1
EcPr
2
1
6
1
24
1
Pr),(
154
22
2
3
20
42
0
2
3
3
2
4
01
XCCYCYCYCCYC
YCYCYCCYX
(72)
Integrating the pressure gradient
0
/ CdXdP
with respect to
X
once, we obtain the
pressure:
XCCPXP
060
)(
(73)
By applying the boundary conditions given in Eq. (70), the inlet condition
2/)0(
2
0
MPP
(Chen & Weng, 2006), the outlet conditions
0
)( PLP
, the midline condition
0/)2/1,( YX , and the edge conditions 1)0,(
L and 0)0,0(
, the six unknown
constants
,,,,,,
543210
CCCCCC and
6
C can be obtained as
,
2
1
,0
,Ec1261
24
Pr
,
2
1
,
2
1
,
1
,
2
1
2
65
2
0
2
1104
10302
1
2
0
MCC
CCCCC
CCCCC
L
C
L
M
C
mcm
r
mcm
(74)
where
1
0
2
,
Gr
,Kn
Ec
11
2
3
,Kn
2
UdY
u
u
M
l
l
L
c
i
c
mc
m
m
m
(75)
By using the flow-rate expression
1
0
UdYM
, the channel length
L
can be obtained as
M
ML
mc
m
61
24
1
(76)
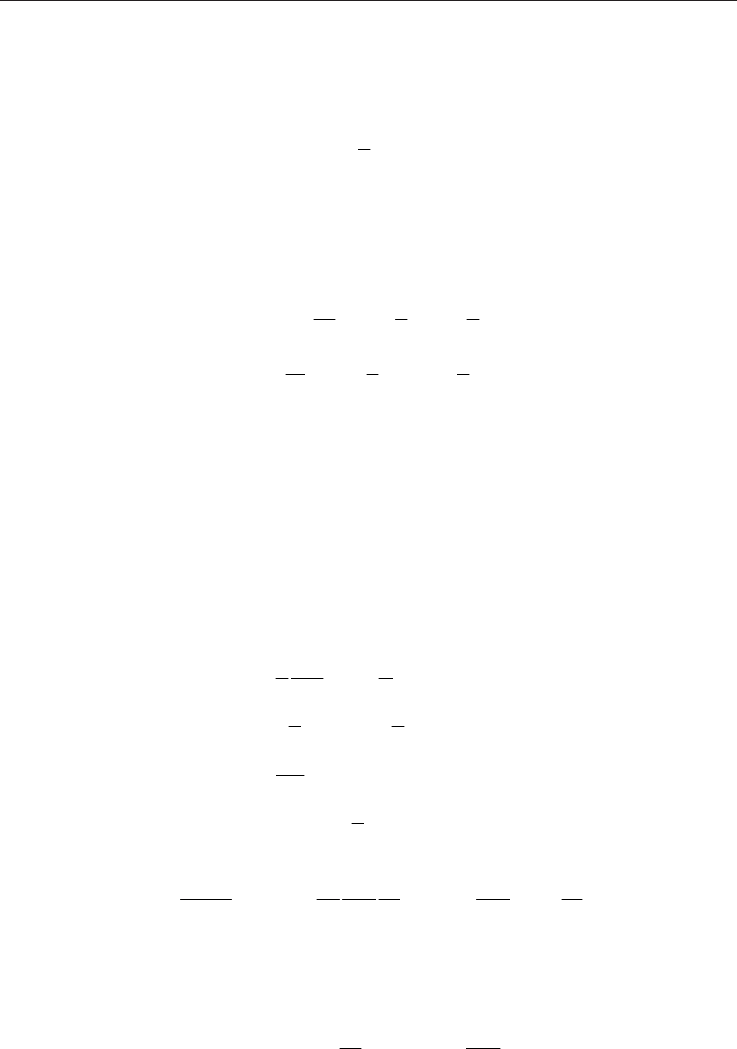
Rareed natural gas transport 545
4.3 Thermocreep-driven flow
If the driving mechanism is thermocreep, as shown in Fig. 2(c), then the reservoir
temperature
0
T is less than the discharge-area pressure
1
T and the discharge-area pressure
1
p is equal to the reservoir pressure
0
p . Modeling the flow as a two-dimensional steady
incompressible constant-property flow, the momentum and energy equations under the
fully developed flow limit (
0
y
u and 0/
xu
x
) are given by (Eqs., 9, 14, 20)
2
2
0
dy
ud
dx
dp
x
r
(64)
2
2
2
dy
du
y
T
k
x
T
uc
x
rrxprr
(65)
Here, we have used the Prandtl boundary layer theory (Prandtl, 1904) to omit nonessential
terms shown in field equations. It should be noted that assuming a small temperature
difference between the reservoir and the discharge area supports the constant-property
assumption.
Proceeding with the analysis, we introduce the following dimensionless parameters:
01
2
2
3
01
2
01
Ec,Gr
,,,,,
Gr
TTc
ulTTg
p
p
P
TT
TT
u
u
U
l
y
Y
l
x
X
pr
c
r
crr
c
r
c
x
cc
(66)
Here, the characteristic length
c
l , velocity
c
u , and pressure
c
p are, respectively, defined as
2
2
01
,,
crc
r
crr
cc
up
lTTg
uwl
(67)
Substituting Eq. (66) into Eqs. (64), (65), and (50) gives
0
2
2
dY
Ud
dX
dP
(68)
2
2
2
EcPrPr
dY
dU
Y
X
U
rr
(69)
gw
r
r
r
gw
r
m
m
c
sl
XdY
dU
u
u
2
Kn
Ec
1
1
2
3
Kn
2
(70)
From Eqs. (68) and (70), a solution of Eq. (68) in the form
)(YU
is only possible if dXdP /
and
X / are constants (let
0
C and
1
C , respectively). The constant value in temperature
gradient implies that the flow under the assumption of hydrodynamically fully developed
flow is also thermally fully developed.
The momentum equation (68) can be integrated twice to obtain the streamwise velocity:
32
2
0
2
1
)(
CYCYCYU
(71)
Substituting Eq. (71) into the energy equation (69) and integrating the resultant with respect
to
Y twice and the thermally fully developed condition
1
/ CX
with respect to
X
once, we obtain the temperature:
.
2
1
3
1
12
1
EcPr
2
1
6
1
24
1
Pr),(
154
22
2
3
20
42
0
2
3
3
2
4
01
XCCYCYCYCCYC
YCYCYCCYX
(72)
Integrating the pressure gradient
0
/ CdXdP
with respect to
X
once, we obtain the
pressure:
XCCPXP
060
)(
(73)
By applying the boundary conditions given in Eq. (70), the inlet condition
2/)0(
2
0
MPP
(Chen & Weng, 2006), the outlet conditions
0
)( PLP , the midline condition
0/)2/1,( YX , and the edge conditions 1)0,( L and 0)0,0( , the six unknown
constants
,,,,,,
543210
CCCCCC and
6
C can be obtained as
,
2
1
,0
,Ec1261
24
Pr
,
2
1
,
2
1
,
1
,
2
1
2
65
2
0
2
1104
10302
1
2
0
MCC
CCCCC
CCCCC
L
C
L
M
C
mcm
r
mcm
(74)
where
1
0
2
,
Gr
,Kn
Ec
11
2
3
,Kn
2
UdY
u
u
M
l
l
L
c
i
c
mc
m
m
m
(75)
By using the flow-rate expression
1
0
UdYM
, the channel length
L
can be obtained as
M
ML
mc
m
61
24
1
(76)

Natural Gas546
5. Summary
In this chapter, the property formulas of natural gases are provided in power-law form. To
simply predict the physical properties of natural gases, the physical properties of methane at
the standard reference state are presented. The basic flows are analyzed by using important
principles including conservation of mass, Newton’s second law of motion, and the first and
second laws of thermodynamics.
The following checklist provides a study guide for this chapter. When your study of the
entire chapter has been completed, you should be able to
use the property formulas of gases to present further properties of other hydrocarbons,
such as ethane, propane, butane, etc.
use the physical properties of methane, in conjunction with the properties of other
gases as necessary, to calculate further physical properties of natural gases in most
common operating states.
use the property formulas of gases and the physical properties of methane at the
standard reference state to conduct further analyses of theoretical and experimental
researches.
use the mass conservation equation to solve further problems involving mass or
volume flow rate.
use the momentum conservation equation subject to the slip boundary conditions to
solve further problems involving force related to momentum change.
use the energy conservation equation subject to the jump boundary conditions to solve
further problems involving losses due to friction and energy input by compressors or
extraction by turbine.
use the analytical procedure shown in basic transport problems to conduct further
analyses of theoretical researches.
apply the analytical solutions of basic transport problems to determine further flow
(or/and thermal) characteristics, predict and analyze further transport behavior of
rarefied natural gas in pipelines, and understand why gas rarefaction in natural gas
transport is so important.
6. Acknowledgment
The author would like to acknowledge financial support from the National Science Council
in Taiwan as grant NSC 98-2218-E-033-003 and the CYCU Distinctive Research Area project
as grant CYCU-98-CR-ME.
7. References
Arkilic, E. B.; Schmidt, M. A. & Breuer, K. S. (1997). Gaseous slip flow in long microchannels.
J. Microelectromech. Systems, 6, 167–178.
Bejan, A., (2004). Convection heat transfer, John Wiley & Sons, 0471271500, 3rd edition.
Beskok, A. & Karniadakis, G. E. (1999). A model for flows in channels, pipes, and ducts at
micro and nano scales. Microscale Thermophys. Eng., 3, 43–77.
Boussinesq, J. (1903). Theorie analytique de la chaleur heat dissipation of parallel plates by free
convection, Gauthier-Villars, Paris.
Burgdorfer, A. (1959). The influence of molecular mean free path on the performance of
hydrodynamic gas lubricated bearings. ASME J. Basic Eng., 81, 94–100.
Carr, N. L. (1954). Viscosity of hydrocarbon gases under pressure. J. Pet. Technol., 6, 47–55.
Chen, C.-K. & Weng, H. C. (2005). Natural convection in a vertical microchannel. ASME J.
Heat Transfer, 127, 1053–1056.
Chen, C.-K. & Weng, H. C. (2006). Developing natural convection with thermal creep in a
vertical microchannel. J. Phys. D, 39, 3107–3118.
Clarke, A. G. & Smith, E. B. (1969). Low‐temperature viscosities and intermolecular forces of
simple gases. J. Chem. Phys., 51, 4156–4161.
Ewart, T.; Perrier, P.; Graur, I. & Méolans, J. G. (2007). Mass flow rate measurements in a
microchannel, from hydrodynamic to near free molecular regimes. J. Fluid Mech.,
584, 337–356.
Friend, D. G.; Ely, J. F. & Ingham, H. (1989). Thermophysical properties of methane. J. Phys.
Chem. Ref. Data, 18, 583–638.
Gammon, B. E. & Douslin, D. R. (1976). The velocity of sound and heat capacity in methane
from near‐critical to subcritical conditions and equation‐of‐state implications. J.
Chem. Phys., 64, 203–218.
GPSA (1998). Engineering Data Book, Section 23–Physical properties, Gas Processors
Suppliers Association, Tulsa, Oklahoma, 11th edition.
Haberman, W. L. & John, J. E. A. (1980) Engineering thermodynamics, Allyn and Bacon, Inc.,
Boston.
Hurly, J. J.; Gillis, K. A.; Mehl, J. B. & Moldover, M. R. (2003). The viscosity of seven gases
measured with a greenspan viscometer. Int. J. Thermophys., 24, 1441–1474.
Hsia, Y. T. & Domoto, G. A. (1983). An experimental investigation of molecular rarefaction
effects in gas lubricated bearings at ultra-low clearances. ASME J. Lubrication Tech.,
105, 120–130.
Ivanov, M. S. & Gimelshein, S. F. (1998). Computational hypersonic rarefied flows. Ann. Rev.
Fluid Mech., 30, 469–505.
Ivings, M. J.; Lea, C. J. & Ledin, H. S. (2003). Outstanding safety questions concerning the
analysis of ventilation and gas dispersion in gas turbine enclosures: best practice
guidelines for CFD, Technical Report CM/03/12, Health and Safety Laboratory.
Jansoone, V.; Gielen, H. & de Boelpaep, J. (1970). The pressure-temperature-volume
relationship of methane near the critical point. Physica., 46, 213–221.
Johnston, H. L. & McCloskey, K. E. (1940). Viscosities of Several Common Gases between
90°K. and Room Temperature. J. Phys. Chem., 44, 1038–1058.
Jin, G. X.; Tang, S. & Sengers, J. V. (1992). Thermodynamic properties of methane in the
critical region. Int. J. Thermophys., 13, 671–684.
Kennard, E. H. (1938) Kinetic theory of gasses, McGraw-Hill, New York.
Kerley, G. I. (1980). A theoretical equation of state for methane,” J. Appl. Phys., 51, 5368–5374.
Kleinrahm, R. & Wagner, W. (1986). Measurement and correlation of the equilibrium liquid
and vapour densities and the vapour pressure along the coexistence curve of
methane. J. Chem. Thermodynam., 18, 739–760.
Kleinrahm, R.; Duschek, W. & Wagner, W. (1986) (Pressure, density, temperature)
measurements in the critical region of methane. J. Chem. Thermodynam., 18, 1103–
1114.

Rareed natural gas transport 547
5. Summary
In this chapter, the property formulas of natural gases are provided in power-law form. To
simply predict the physical properties of natural gases, the physical properties of methane at
the standard reference state are presented. The basic flows are analyzed by using important
principles including conservation of mass, Newton’s second law of motion, and the first and
second laws of thermodynamics.
The following checklist provides a study guide for this chapter. When your study of the
entire chapter has been completed, you should be able to
use the property formulas of gases to present further properties of other hydrocarbons,
such as ethane, propane, butane, etc.
use the physical properties of methane, in conjunction with the properties of other
gases as necessary, to calculate further physical properties of natural gases in most
common operating states.
use the property formulas of gases and the physical properties of methane at the
standard reference state to conduct further analyses of theoretical and experimental
researches.
use the mass conservation equation to solve further problems involving mass or
volume flow rate.
use the momentum conservation equation subject to the slip boundary conditions to
solve further problems involving force related to momentum change.
use the energy conservation equation subject to the jump boundary conditions to solve
further problems involving losses due to friction and energy input by compressors or
extraction by turbine.
use the analytical procedure shown in basic transport problems to conduct further
analyses of theoretical researches.
apply the analytical solutions of basic transport problems to determine further flow
(or/and thermal) characteristics, predict and analyze further transport behavior of
rarefied natural gas in pipelines, and understand why gas rarefaction in natural gas
transport is so important.
6. Acknowledgment
The author would like to acknowledge financial support from the National Science Council
in Taiwan as grant NSC 98-2218-E-033-003 and the CYCU Distinctive Research Area project
as grant CYCU-98-CR-ME.
7. References
Arkilic, E. B.; Schmidt, M. A. & Breuer, K. S. (1997). Gaseous slip flow in long microchannels.
J. Microelectromech. Systems, 6, 167–178.
Bejan, A., (2004). Convection heat transfer, John Wiley & Sons, 0471271500, 3rd edition.
Beskok, A. & Karniadakis, G. E. (1999). A model for flows in channels, pipes, and ducts at
micro and nano scales. Microscale Thermophys. Eng., 3, 43–77.
Boussinesq, J. (1903). Theorie analytique de la chaleur heat dissipation of parallel plates by free
convection, Gauthier-Villars, Paris.
Burgdorfer, A. (1959). The influence of molecular mean free path on the performance of
hydrodynamic gas lubricated bearings. ASME J. Basic Eng., 81, 94–100.
Carr, N. L. (1954). Viscosity of hydrocarbon gases under pressure. J. Pet. Technol., 6, 47–55.
Chen, C.-K. & Weng, H. C. (2005). Natural convection in a vertical microchannel. ASME J.
Heat Transfer, 127, 1053–1056.
Chen, C.-K. & Weng, H. C. (2006). Developing natural convection with thermal creep in a
vertical microchannel. J. Phys. D, 39, 3107–3118.
Clarke, A. G. & Smith, E. B. (1969). Low‐temperature viscosities and intermolecular forces of
simple gases. J. Chem. Phys., 51, 4156–4161.
Ewart, T.; Perrier, P.; Graur, I. & Méolans, J. G. (2007). Mass flow rate measurements in a
microchannel, from hydrodynamic to near free molecular regimes. J. Fluid Mech.,
584, 337–356.
Friend, D. G.; Ely, J. F. & Ingham, H. (1989). Thermophysical properties of methane. J. Phys.
Chem. Ref. Data, 18, 583–638.
Gammon, B. E. & Douslin, D. R. (1976). The velocity of sound and heat capacity in methane
from near‐critical to subcritical conditions and equation‐of‐state implications. J.
Chem. Phys., 64, 203–218.
GPSA (1998). Engineering Data Book, Section 23–Physical properties, Gas Processors
Suppliers Association, Tulsa, Oklahoma, 11th edition.
Haberman, W. L. & John, J. E. A. (1980) Engineering thermodynamics, Allyn and Bacon, Inc.,
Boston.
Hurly, J. J.; Gillis, K. A.; Mehl, J. B. & Moldover, M. R. (2003). The viscosity of seven gases
measured with a greenspan viscometer. Int. J. Thermophys., 24, 1441–1474.
Hsia, Y. T. & Domoto, G. A. (1983). An experimental investigation of molecular rarefaction
effects in gas lubricated bearings at ultra-low clearances. ASME J. Lubrication Tech.,
105, 120–130.
Ivanov, M. S. & Gimelshein, S. F. (1998). Computational hypersonic rarefied flows. Ann. Rev.
Fluid Mech., 30, 469–505.
Ivings, M. J.; Lea, C. J. & Ledin, H. S. (2003). Outstanding safety questions concerning the
analysis of ventilation and gas dispersion in gas turbine enclosures: best practice
guidelines for CFD, Technical Report CM/03/12, Health and Safety Laboratory.
Jansoone, V.; Gielen, H. & de Boelpaep, J. (1970). The pressure-temperature-volume
relationship of methane near the critical point. Physica., 46, 213–221.
Johnston, H. L. & McCloskey, K. E. (1940). Viscosities of Several Common Gases between
90°K. and Room Temperature. J. Phys. Chem., 44, 1038–1058.
Jin, G. X.; Tang, S. & Sengers, J. V. (1992). Thermodynamic properties of methane in the
critical region. Int. J. Thermophys., 13, 671–684.
Kennard, E. H. (1938) Kinetic theory of gasses, McGraw-Hill, New York.
Kerley, G. I. (1980). A theoretical equation of state for methane,” J. Appl. Phys., 51, 5368–5374.
Kleinrahm, R. & Wagner, W. (1986). Measurement and correlation of the equilibrium liquid
and vapour densities and the vapour pressure along the coexistence curve of
methane. J. Chem. Thermodynam., 18, 739–760.
Kleinrahm, R.; Duschek, W. & Wagner, W. (1986) (Pressure, density, temperature)
measurements in the critical region of methane. J. Chem. Thermodynam., 18, 1103–
1114.

Natural Gas548
Kurumov, D. S.; Olchowy, G. A. & Sengers, J. V. (1988). Thermodynamic properties of
methane in the critical region. Int. J. Thermophys., 9, 73–84.
Mann, W. B. & Dickins, B. G. (1931). The Thermal Conductivities of the Saturated
Hydrocarbons in the Gaseous State. Proc. R. Soc. Lond. A, 134, 77–96.
Maxwell, J. C. (1879). On stress in rarefied gases from inequalities of temperature. Philos.
Trans. R. Soc. London, 170, 231–256.
Pátek, J. & Klomfar, J. (2002). Measurement of the thermal conductivity of argon and
methane: a test of a transient hot-wire apparatus. Fluid Phase Equilib., 198, 147–163.
Pfahler, J.; Harley, J. C.; Bau, H. & Zemel, J. N. (1991). Gas and liquid flow in small channels,
Winter Annual Meeting of the American Society of Mechanical Engineers, 32, 49–60,
0791808637, Atlanta, December 1991, ASME, New York.
Pong, K. C.; Ho, C. M.; Liu, J. & Tai, Y. C. (1994). Nonlinear pressure distribution in uniform
microchannels, Proceedings of the 1994 International Mechanical Engineering Congress
and Exposition, 197, pp. 51–56, Chicago, November 1994, ASME, New York.
Prandtl, L. (1904) On fluid motions with very small friction (in German), Proceedings of the
3rd International Mathematical Congress, pp. 484–491, Heidelberg, Germany.
Schaaf, S. A. & Chambre, P. L. (1961). Flow of rarefied gases, Princeton University Press, New
Jersey.
Schley, P.; Jaeschke, M.; Kuchenmeister, C. & Vogel, E. (2004). Viscosity measurements and
predictions for natural gas.
Sonntag, R. E.; Borgnakke, C. & Wylen, G. J., (1998). Fundamentals of thermodynamics, John
Wiley & Sons, 047118361X, New York, 5th edition.
Tsuboi, N. & Matsumoto, Y. (2005). Experimental and numerical study of hypersonic
rarefied gas flow over flat plates. AIAA Journal, 43, 1243–1255.
Vennix, A. J.; Leland Jr., T. W. & Kobayashi, R. (1970). Low-temperature volumetric
properties of methane. J. Chem. Eng. Data, 15, 238–243.
Viswanathan, A. (2007). Viscosities of natural gases at high pressures and high temperatures, MS
thesis, Texas A&M University, College. Station, Texas.
Weng, H. C. & Chen, C.-K. (2008a). A challenge in Navier–Stokes-based continuum
modeling: Maxwell–Burnett slip law. Phys. Fluids, 20, 106101.
Weng, H. C. & Chen, C.-K. (2008b). Variable physical properties in natural convective gas
microflow. ASME J. Heat Transf., 130, 082401.
Weng, H. C. & Chen, C.-K. (2008c). Fully developed thermocreep-driven gas microflow.
Appl. Phys. Lett., 92, 094105.
Younglove, B. A. (1974). The specific heats cp and cv of compressed and liquified methane. J.
Res. Natl. Bur. Stand., 78A, 401–410.

Consequence analysis of large-scale liqueed natural gas spills on water 549
Consequence analysis of large-scale liqueed natural gas spills on water
Hideyuki Oka
X
Consequence analysis of large-scale
liquefied natural gas spills on water
Hideyuki Oka
National Maritime Research Institute
Japan
1. Introduction
1.1 Background
As public concerns increase over global warming caused by the burning of fossil fuels,
natural gas is gaining a lot of attention for the lowest emission of carbon dioxide among the
fossil fuels. Thus, governments implementing national or regional plans to reduce
greenhouse gas emissions may encourage its use to displace other fossil fuels. According to
the Energy Information Administration, the worldwide natural gas consumption in 2030
will increase by about one and a half times as much as in 2006 (EIA, 2009), so that the
number and frequency of seaborne transportation of liquefied natural gas (LNG) are
expected to increase significantly around the world. In fact, there are a lot of projects to
build new receiving terminals in the United States. Also, natural gas consumption is
expected to rise rapidly in China and India. With such a growing global demand, recent
LNG carriers (LNGCs) become larger up to a 266,000 m
3
cargo capacity, which are referred
to as Q-Max vessels.
Due to the above change in the situation, there has recently been considerable interest
concerning possible risks involved in the LNG carrier operations, though seaborne
transportation of LNG has been conducted with a very good safety record since 1959.
Hence, public authorities have raised their awareness of concern about the possibility of
large-scale LNG spill hazards caused by accidental events or intentional attacks. As a result,
a number of consequence analyses have been carried out in recent years in order to propose
models and approaches or to assess hazards resulting from an unconfined LNG spill over
water (Luketa-Hanlin, 2006). However, these studies showed a broad range of results due to
their differences in models, approaches and assumptions, since the physics involved in such
LNG spills and related phenomena is very complicated. In addition, because of the lack of
experimental data for a large-scale LNG spill and subsequent combustion and/or dispersion
events, there are many theoretical and experimental gaps related to understanding of the
dynamics and limitations in predicting the associated hazards. Therefore, consequence
assessment methods based on a combination of theoretical formulations and empirical
relationships derived from laboratory and small-scale field experiments are the only
practical measure to predict the hazards associated with large-scale LNG spills on water.
23

Natural Gas550
On the other hand, a broad range of results of these studies indicates how important it is to
use appropriate assumptions, data, and models in trying to make an accurate assessment of
hazards from an LNG spill. Although the results of recent consequence studies were
compared in a few publications (Hightower et al., 2004), there was no comparison study on
consequence models under the same scenarios in terms of LNG properties, release
assumptions and weather conditions. Therefore, the current author compared and evaluated
consequence models for pool fire hazards involving an LNG spill on water in order to
clarify their characteristics (Oka & Ota, 2008).
In the above comparison study, attention was paid to thermal radiation hazards from pool
fires, because there is a high possibility that an ignition source immediately after breaching a
tank will be available (Hightower et al., 2004). Hence, the sensitivity analysis of a spill and
the subsequent pool fire hazards to the hole size breached in a membrane-type tank of a
conventional size LNGC (125,000 m
3
cargo capacity) were carried out using three major
consequence assessment methods developed by the Federal Energy Regulatory Commission
(FERC) (FERC, 2004), Sandia National Laboratories (SNL) (Hightower et al., 2004) and Fay
(Fay, 2003). These methods were chosen based on an in-depth review of the recent literature
available to the public. Through the sensitivity analysis, it was found that the FERC method
was most appropriate for practical consequence analyses of incidents involving large-scale
LNG spills on water from the practical viewpoint of applicability to any breach size.
Recent LNGCs are designed to have as much as a 266,000 m
3
cargo capacity, so that it is
important to evaluate how much the extent of the hazard impact would increase due to the
enlarged size and capacity of such carriers. Thus, thermal radiation hazards from pool fires
involving spills from one of the latest and largest LNGCs (250,000m
3
cargo capacity) were
assessed using the recommended FERC method, and the results were discussed in
comparison with those for the conventional size LNGC. As a result, it was found that the
maximum thermal hazard distance was only about 24 % longer than that for the
conventional LNGC, while the spill volume was twice as much (Oka & Ota, 2008).
When the author focused on estimating LNG spill hazards from the latest LNGC, similar
hazard assessments had not been covered at least in the publicly available literature.
However, in almost the same period the U.S. Department of Energy requested that SNL
conduct analyses of possible spill hazards from a breach of the latest LNGC (Luketa et al.,
2008). The results of both studies were published at the same time. This updated SNL study
presented somewhat different results in that the thermal hazard distances increased by
approximately 7–8 % due to the increase in hydrostatic head and tank volume for the new,
larger LNGC. In the scenarios used in the SNL studies (Luketa et al., 2008), the nominal
breach size and the total spill volume from a single tank were determined as 5 m
2
and 41,000
m
3
, respectively, so that a smaller breach size and a larger spill volume were used than those
in the other study (Oka & Ota, 2008). Hence, for quantitative comparison, the current author
carried out consequence analyses of pool fire hazards following an LNG spill from a
breached tank of the conventional and latest LNGCs under the same scenarios as in the SNL
studies (Oka, 2009). It was found that, as a whole, the thermal hazard consequences by the
SNL method were in fairly good agreement with those by the FERC method.
1.2 Scope of the present study
The principal LNG hazards of interest for the present study are those posed by thermal
radiation and flammable vapor dispersion resulting from unconfined LNG spills on water.
Cryogenic burns and asphyxiation are typically localized to LNG transport and storage
areas, so that such secondary hazards are outside the scope of this study.
The two previous studies for the latest LNGC by the FERC method (Oka & Ota, 2008; Oka,
2009) were carried out under the following scenarios. In the first study (Oka & Ota, 2008),
predicted consequences were compared only when the hole diameters were 1, 3 and 5 m as
typical hole sizes, which were chosen from the recent literature on the assessment of the
impacts of large-scale release from the conventional type LNGC. In the second study (Oka,
2009), two breach sizes of 5 and 12 m
2
were used as nominal tank breaches for near-shore
and offshore LNG marine import operations, respectively, so as to compare the updated
SNL study (Luketa et al., 2008). Therefore, no sensitivity analysis of pool fire hazards to the
hole size has been carried out for the latest LNGC.
As for flammable vapor dispersion hazards, as far as the author knows, there is no study to
assess consequences predicted by the FERC method for the latest LNGC. Though the
sensitivity analysis of spills and the subsequent dispersion hazards to the breach size were
conducted for the conventional size LNGC using the FERC method (Qiao et al., 2006), the
averaging time used to estimate flammable gas concentrations was much larger than the
recommended value in the FERC method. Thus, it is interesting to evaluate the sensitivity
using the FERC method composed of all the recommended models and assumptions for the
latest LNGC.
The present work considers the sensitivity of the flammable vapor and thermal radiation
hazards to the hole diameter under release scenarios that a hole can develop just above the
waterline level in the event of a breach of a single tank on the conventional and latest
LNGCs. Under current circumstances, from the practical viewpoint of applicability to any
breach size, the FERC method has been recommended in the previous studies (Oka & Ota,
2008; Oka, 2009), so that the present consequence analyses are carried out using the same
method.
2. Overview of potential consequences
Currently, the potential for the dynamics and dispersion of a large spill and the associated
hazards are not fully understood. As will be shown in Fig. 1 later, existing experimental data
on LNG spill dynamics, dispersion, and burning over water cover only small amount of spill
volumes that are two to three orders of magnitude less than those postulated in the recent
literature (Luketa-Hanlin, 2006).
2.1 Brief description on major hazards of an LNG spill on water
The potential hazards associated with LNG spills include cryogenic damage caused by
direct contact, pressure increase due to rapid phase transition (RPT), flash fires, pool fires,
deflagrations and detonations. Because of its extremely low temperature, direct contact with
LNG will result in brittle fracture of the ship's structure, which may cause cascading
damage to additional LNG tanks. When LNG comes in contact with water at a significantly
higher temperature than the boiling point of LNG, there is the possibility of RPT, which is a
nearly instantaneous transition from the liquid to vapor phases and produces an associated
rapid pressure increase. The impacts of RPT will be localized near the spill source and
should not cause extensive structural damage.
