Parinov I.A. Microstructure and Properties of High-Temperature Superconductors
Подождите немного. Документ загружается.

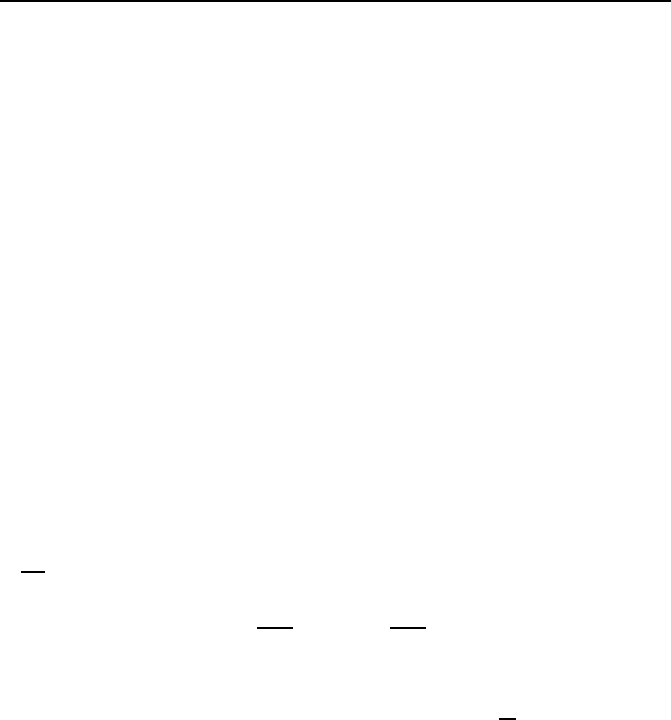
C
Macrostructure Modeling of Heat Conduction
C.1 Method of Summary Approximation
for Quasi-Linear Equation of Heat Conduction
For macrostructure modeling of the heat front propagation during sintering
and cooling of HTSC ceramic, the method of summary approximation (MSA)
is used as a method for construction of economic schemes for quasi-linear
non-stationary equations in the case of arbitrary region and any number of
measurements p [916].
The quasi-linear equation of heat conduction without heat sources (heat
capacity, c
V
, and material density, ρ, are suggested to be constant) has the
form:
∂u
∂t
= Lu; x =(x
1
,x
2
,...x
p
) ∈ G, t > 0;
Lu =
p
#
α=1
L
α
u; L
α
u =
∂
∂x
α
k
α
(u, x
α
)
∂u
∂x
α
; k
α
≥ C
α
> 0(C.1)
with boundary and initial conditions:
u|
Γ
= μ(x, t); u(x, 0) = u
0
(x); x ∈ G, (C.2)
where u is the temperature; x
α
are the spatial coordinates; t is the time and
α is the fixed coordinate direction. A boundary Γ of a region G is sufficiently
smooth, which is necessary for the existence of smooth solution, u = u(x, t).
It is assumed that smooth derivations are required.
As it is made in all economic schemes, the process of approximate solution
for multidimensional problem is divided into several stages. Simple problem
is solved at every stage. The operator L is presented by the operator sum of
more simple structures:
L =
p
#
α=1
L
α
. (C.3)
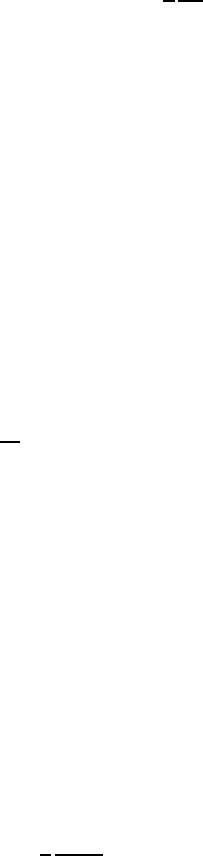
516 C Macrostructure Modeling of Heat Conduction
We consider multidimensional equation (C.1) and compare to problem
(C.1), (C.2) a “chain” of equations:
p
#
α=1
P
α
u =0; P
α
u =
1
p
∂u
∂t
− L
α
u. (C.4)
At the interval, 0 ≤ t ≤ t
0
, an uniform lattice,
τ
= {t
j
= jτ; j =
0, 1,...,j
0
} with a step, τ = t
0
/j
0
, is introduced. Every interval is divided into
p parts, introducing points t
j+α/p
= t
j
+ατ/p; α =1, 2,...,p−1. Successively
(at α =1, 2,...,p),
P
α
V
(α)
=0; x ∈ G; t ∈ (t
j+(α−1)/p
; t
j+α/p
]; α =1, 2 ...,p , (C.5)
are solved suggesting
V
(1)
(x, 0) = u
0
(x); V
(α)
(x, t
j+(α−1)/p
)=V
(α−1)
(x, t
j+(α−1)/p
) . (C.6)
Also, it is assumed that the boundary condition of first type is given at Γ.
The solution of this problem is named
V (x, t
j
)=V
(p)
(x, t
j
); j =0, 1,...,j
0
. (C.7)
Every of the equations, P
α
V
(α)
= 0, it is substituted by the difference
scheme (approximating
∂u
∂t
and L
α
by corresponding difference relations at
uniform lattice ω
h
with steps h
1
,h
2
,...,h
p
)
Π
α
y
(α)
=0; α =1, 2,...,p. (C.8)
The scheme (C.8) approximates the equation P
α
V
(α)
= 0 in usual sense,
that is
Π
α
u
j+α/p
− (P
α
u)
j+α/p
→ 0, at τ → 0andh
α
→ 0 . (C.9)
The summary approximation of additive scheme (C.8) is attained due
to the “chain” of differential equations (C.5), and (C.6) approximates corre-
sponding equations (C.5) in usual sense.
Then, it is assumed that L
α
consists of derivations only on variable x
α
.
Therefore, L
α
is the one-dimensional operator, P
α
V
(α)
= 0 are the one-
dimensional equations and additive scheme (C.8) is the local one-dimensional
scheme (LOS). We write LOS, and with this aim the multidimensional equa-
tion is replaced by “chain” of one-dimensional equations:
1
p
∂V
(α)
∂t
= L
α
V
(α)
, (C.10)
at
t
j+(α−1)/p
<t≤ t
j+α/p
; α =1, 2 ...p; x ∈ G; t
j+α/p
=(j + α/p)τ,
(C.11)
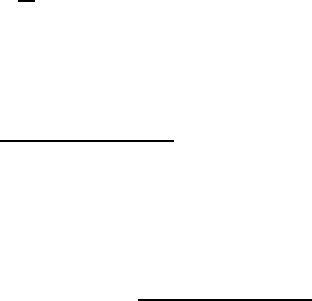
C.1 Method of Summary Approximation for Quasi-Linear Equation 517
with conditions
V
(1)
(x, 0) = u
0
(x); V
(α)
(x, t
j+(α−1)/p
)=V
(α−1)
(x, t
j+(α−1)/p
);
V
(α)
= μ(x, t), at x ∈ Γ
α
. (C.12)
For difference approximation of the operator L
α
in node x
i
, a three-point
templet is used, consisting of the points: x
(−1α)
i
,x
i
,x
(+1α)
i
where x
(±1α)
i
=
[x
(i
1
)
1
,...,x
(i
α
)
α
±h
α
,...,x
(i
P
)
p
]; x
(i
α
)
α
= h
α
i
α
; h
α
is the step of the lattice ω
h
in
α-direction. A number of internal nodes of the lattice ω
h
consists of the points
x =(x
1
,x
2
,...,x
p
) ∈ G of crossing of the hyper-planes x
α
= i
α
h
α
; i
α
=
0, ±1, ±2,...; α =1, 2,...,p, but a number of boundary nodes γ
h
consists of
the points of crossing of the straight lines C
α
, passing through all internal
nodes x ∈ ω
h
, with boundary Γ . Also introduced are the next designations:
γ
h,α
is the number of boundary nodes in direction x
α
; γ
h
is the number of all
boundary nodes x ∈ Γ .
Let
G = { 0 ≤ x
α
≤ l
α
} is the parallelepiped, then Γ
α
consists of the
facets: x
α
=0andx
α
= l
α
. Approximating every heat conduction equation
of number α at semi-interval (t
j+(α−1)/p
; t
j+α/p
] by a two-layer scheme with
weights, a “chain” of p one-dimensional schemes is stated that is called LOS:
y
j+α/p
− y
j+(α−1)/p
τ
= Λ
α
[σ
α
y
j+α/p
+(1− σ
α
)y
j+(α−1)/p
] , (C.13)
where α =1, 2,...,p; x ∈ ω
h
; σ
α
∈ [0, 1].
In regular nodes, Λ
α
has second order of approximation, Λ
α
u − L
α
u =
O(h
2
α
), and in non-regular nodes, Λ
α
u−L
α
u = O(1). Consider purely implicit
LOS (σ
α
≡ 1):
y
j+α/p
− y
j+(α−1)/p
τ
= Λ
α
y
j+α/p
, (C.14)
and join to this equation the boundary condition:
y
j+α/p
= μ
j+α/p
, at x ∈ γ
h,α
; j =0, 1,...,j
0
; α =1, 2,...,p, (C.15)
and initial condition:
y(x, 0) = u
0
(x) . (C.16)
Let us assume y
j
is known. In order to define y
j+1
at new layer from (C.14)
and (C.15), the p equations (C.14) are required to solve together with the
boundary condition (C.15), successively suggesting α =1, 2,...,p.Inorder
to define y
j+α/p
, we have the boundary-value problem:
A
i
α
y
j+α/p
i
α
−1
− C
i
α
y
j+α/p
+ A
i
α
+1
y
j+α/p
i
α
+1
=0, at x ∈ ω
h
; (C.17)
y
j+α/p
= μ
j+α/p
, at x ∈ γ
h,α
; α =1, 2,...,p. (C.18)
Here, the lower indexes are pointed only, which change in calculations. The
difference equation is written along the section of the straight line, the ends
518 C Macrostructure Modeling of Heat Conduction
of which coincide with the nodes γ
h,α
. Equation (C.17) is solved by the run
method at fixed α-direction along corresponding sections. Successively sug-
gesting α =1, 2,...,pand changing the run directions, y
j+1/p
,y
j+2/p
,...,y
j+1
are calculated, expending O(1) operations per lattice node. Thus, the LOS
(C.14)–(C.16) is economic [916]. Moreover, it can be shown that the LOS ap-
proximation error tends to zero at τ → 0andh
α
→ 0, and from summary
approximation, uniform convergence of the LOS with rate, O
τ +max
1≤α≤p
h
2
α
is followed [916].
C.2 Heat Conduction of Heterogeneous Systems
A study of heat conduction of the heterogeneous systems is a sufficiently
complex problem. In the case of HTSC ceramics, it is even more complicated
due to significant porosity and different, compared to metal, mechanism of
heat conduction. In metal, demonstrating a small porosity and high heat con-
duction of crystallites, the main mechanism of heat conduction is the convec-
tion. In oxide superconductors, possessing relatively greater porosity, together
with heat transfer on solid component, the main sources of heat transfer are
molecular and radiant components of heat conduction.
C.2.1 Effective Heat Conduction of Mixes
and Composites
Existing mixes and composites can be presented by one of the models, depicted
in Fig. C.1. A process of ceramic sintering can be described by using structure
with mutual-penetrating components (see Fig. C.1b) and the loose granu-
lar material (see Fig. C.1e). All components of the structure with mutual-
penetrating components are continuous in any direction and geometrically
equivalent relative to effective heat conduction:
λ
ef
= f
1
(λ
1
,λ
2
)=f
2
(λ
2
,λ
1
), at m
1
= m
2
, (C.1)
where λ
i
and m
i
(i =1, 2) are the heat conduction and concentration of the
components.
Granular materials consist of monolithic particles (1) (see Fig. C.1e) and
occupy an intermediate state between the structures with impregnation and
the structures with mutual-penetrating components. The contacting particles
and pores (2), disposed between them, form continuous extent of solid com-
ponents and cavities in any direction.
Following [234], define heat conduction of granular system. First, consider
a structure with mutual-penetrating components. In order to take into account
the distortion of the heat flux lines, we use the following formulae for effective
C.2 Heat Conduction of Heterogeneous Systems 519
1
2
(a)
(c)
(d)
(e)
1
2
1
(b)
2
Fig. C.1. Heterogeneous systems with different structure: (a) impregnated struc-
ture; (b) structure with mutual-penetrating components; (c, d) combined structures
with mutual-penetrating components and impregnation; (e) loose granular material
heat conduction in the cases of adiabatic and isothermal division of elementary
cell, respectively:
λ
ad
= λ
1
c
2
+ ν(1 − c)
2
+
2c(1 − c)ν
1+c(ν − 1)
,ν= λ
2
/λ
1
;(C.2)
λ
is
= λ
1
1 − c
c
2
+ ν(1 − c
2
)
+
c
c(2 − c)+ν(1 − c)
2
−1
. (C.3)
here c is the solution of the equation:
m
2
=2c
3
− 3c
2
+1;m
2
=1− m
1
. (C.4)
520 C Macrostructure Modeling of Heat Conduction
Hence
c =0.5+A cos(ϕ/3) , (C.5)
where
A =
−1; ϕ = arccos (1 − 2m
2
), at 0 ≤ m
2
≤ 0.5;
1; ϕ = arccos (2m
2
− 1), at 0.5 ≤ m
2
≤ 1 ,
(C.6)
and 3π/2 ≤ ϕ ≤ 2π. Then, effective heat conduction is defined as
λ
ef
=(λ
ad
+ λ
is
)/2 . (C.7)
The approximate solution (C.7) gives values, which are near to numerical
results and preserves property of component invariability.
C.2.2 Polystructural Model of Granular Material
A heat transfer into pores occurs due to molecular collisions and radiation.
At the same time, convection is absent, as a rule. The molecular transfer of
heat takes place due to interchange by kinetic energy at collisions of moving
molecules with one another and with surface of solid or liquid component,
limiting pores (grain and liquid surfaces). The heat transfer due to radiation
occurs on account of the absorption, emission and dissipation of radiant en-
ergy. Both mechanisms can exist together and influence each other mutually.
The granular structure of ceramic powder consists of “frame” (1) (see
Fig. C.1e), formed by the chaotic, but relatively dense package of continuously
contacting grains (the structure of first order) and spatial lattice of more larger
cavities (2), penetrating the powder, which together with the frame form the
structure of second order with mutual-penetrating continuous components.
Not destroying generality, it may be assumed that the structure of second
order occurs at m
2
≥ 0, 4 [235].
First, a dependence of coordination number, N
c
(i.e., number of contacts
per one particle) on the porosity m
2
is defined. For this, a granular system is
considered, consisting of convex rounded particles. In all points of the particle
contacts, we depict tangential planes. Then, volume of the system is divided
into polyhedrons, circumscribed around any particle. In this case, the facet
number of every polyhedron is equal to the number of contacts for given
particle (Fig. C.2a).
The porosity of the system is presented through ratio of the difference be-
tween volumes of all polyhedrons and particles to volume of the polyhedrons.
Determination of required dependence for the parameter N
c
is carried out, us-
ing arbitrary polyhedron, circumscribed around particle with mean radius, r.
Then, N
c
is the mean coordination number for all polyhedrons of the system.
For chaotic actual structure, it is assumed that all contacts are uniformly
distributed on particle surface. Then, it is suggested that the polyhedron con-
sists of N
c
identical pyramids with tops at the particle center (Fig. C.2b). In
this case, mean porosity of pyramid is equal to mean porosity of the granular
system. For simplicity, the pyramid is substituted by cone with the same solid

C.2 Heat Conduction of Heterogeneous Systems 521
O
(a)
O
(b)
O
C
A
B
D
2r
c
(c)
Fig. C.2. Substitution procedure used for definition of coordination number as
function of porosity: (a) polyhedron formed due to crossing the planes tangential
to particle in contact points; (b) pyramid is the element of polyhedron; (c) straight
circular cone
angle and around-contact surfaces are replaced by spherical ones (Fig. C.2c).
Then, the porosity of this system is equal to
m
2
=1− V
bs
/V
c
; V
c
=0.33πr
2
c
r, (C.8)
where V
c
is the cone volume; V
bs
is the ball sector in the cone; r
c
is the
radius of the cone base: and r is the radius of the spherical surface.
In order to define r
c
, we use equalities: F
b
/N
c
= F
s
=2πrh
s
. Hence
h
s
=2r/N
c
, (C.9)
where F
b
,F
s
are the surface squares of the ball and ball segment and h
s
is
the segment height. Because ΔOAB is similar to ΔOCD, then from (C.9), we
obtain
r
c
=2r(N
c
− 1)
1/2
/(N
c
− 2) . (C.10)
The volume of the ball sector is smaller by N
c
times than the volume of
the ball
V
bs
=4πr
3
/3N
c
. (C.11)
By using the formula for N
c
and (C.8), (C.10) and (C.11), we obtain finally
N
c
=[m
2f
+3+(m
2
2f
− 10m
2f
+9)
1/2
]/2m
2f
. (C.12)
Then, effective heat conduction of the structure of second order with
mutual-penetrating components is calculated by using (C.2), reduced to the
form
λ = λ
f
c
2
2
+ ν
f
(1 − c
2
)
2
+
2ν
f
c
2
(1 − c
2
)
ν
f
c
2
+1− c
2
; ν
f
= λ
22
/λ
f
. (C.13)
Here, the geometrical parameter c
2
characterizes volume concentration of
frame and is connected with the porosity, m
22
(in the second order structure),
throughanequationoftype(C.4):
m
22
=2c
3
2
− 3c
2
2
+1. (C.14)

522 C Macrostructure Modeling of Heat Conduction
The volume concentration of pores, m
2
, in granular system with volume
V and pore volume V
2
is equal to m
2
= V
2
/V , but the porosities of the frame,
m
2f
, and the second order structure, m
22
, are found as
m
2f
= V
2f
/(V
1
+ V
2f
); m
22
= V
22
/V , (C.15)
where V
1
,V
2f
and V
22
are the volumes of particles, pores in the frame and
pores in the second order structure.
Moreover, there are next relations:
V = V
22
+ V
2f
+ V
1
; V
2
= V
22
+ V
2f
. (C.16)
Then, we obtain from (C.15) and (C.16)
m
22
=(m
2
− m
2f
)/(1 − m
2f
) . (C.17)
In order to calculate heat conduction, using (C.13), it is necessary to know
the heat conduction of component, filling pores of the second order structure,
λ
22
, and heat conduction of the frame, λ
f
.Thevalueofλ
22
is the sum of
molecular and radiant components. It depends both on the physical proper-
ties of gas and on the geometrical and physical parameters of the pores. For
structure of granular material, the molecular component is calculated as [234]
λ
2m
=
λ
g
1+B/(Hδ
2c
)
, (C.18)
where δ
2c
=3d(1 − c
2
)/c
2
is the mean size of great cavities; d =2r is the
grain diameter;
B =
4γ(2 − a)Λ
c
H
(γ +1)aPr
;
where λ
g
is the heat conduction factor of gas in the infinite space at pressure
H and temperature T ; a is the accommodation factor of gas at uniform walls;
Λ
c
is the mean length of the gas molecule run; γ = c
p
/c
V
is the adiabatic
index, being a ratio of isobaric heat capacity to isochoric; Pr = ν/k is Prandtl’s
criterion, being the ratio of kinematic viscosity, ν, to temperature conductivity
of gas, k.
Relation for radiant component of the heat conduction factor in pores of
the second order structure has the form [234]:
λ
2r
≈ 0.23(T/100)
3
Yd
c
2
2
(1 − c
2
)(2 − ε)
, (C.19)
where Y = f(τ, ε) is the function, taking into account influence of the optic
thickness of sample, τ = βl
l
, and of the blackness degree, ε, limiting surfaces
(walls); for “gray” approximation, β = α
λ
+ γ
λ
is the spectrum factor of
weakening; α
λ
and γ
λ
are the volume spectrum factors of absorption and
scattering; l
l
is the thickness of filling layer. The value Y ≈ 1 for granular

C.2 Heat Conduction of Heterogeneous Systems 523
systems with porosity m
2
< 0.95 [234]. We obtain from (C.18) and (C.19)
relation for the heat conduction of gas component in pores of the second
order structure:
λ
22
= λ
g
1+
Bc
2
3Hd(1 − c
2
)
−1
+0.23(T/100)
3
Yd
c
2
2
(1 − c
2
)(2 − ε)
. (C.20)
C.2.3 Model of Granular System with Chaotic Structure
In order to model the granular system with chaotic structure, consider a sys-
tem consisting of rounded absolute solid particles with heat conduction fac-
tors, that are greater than corresponding parameter of component, occupying
pores. Main fraction of heat flux passes through the regions, surrounding point
contacts of particles (the sizes of near-contact regions are much smaller than
grain diameter). Then, we divide the heat flux into single flux tubes, so that
the tube axis in every particle passes successively the near-contact regions at
entry and exit of the flux (Fig. C.3a).
Assumption 1. Heat conduction of any tube is equal to effective heat con-
duction of all granular system.
It is assumed that the tube length is much more greater than the cross-
section length of particles with non-elongated shape, which fill the granular
system volume chaotically. We divide the tube into elements: i −1,i,i+1,....
Every element is limited by two planes perpendicular to the heat flow,
namely the plane in contact point and the plane δ–δ, dividing particle in
half (Fig. C.3). The lateral surface of the tube is formed by adiabatic surface.
Thermal resistance of the tube is equal to the sum of thermal resistance
of its elements, which are divided into two types. The elements without
(first type) and with (second type) through pores are shown respectively in
Fig. C.3b and c. The through pores are present in only those elements of the
tube (second type) for which a–a plane (Fig. C.3c) contacts with δ–δ plane
within tube.
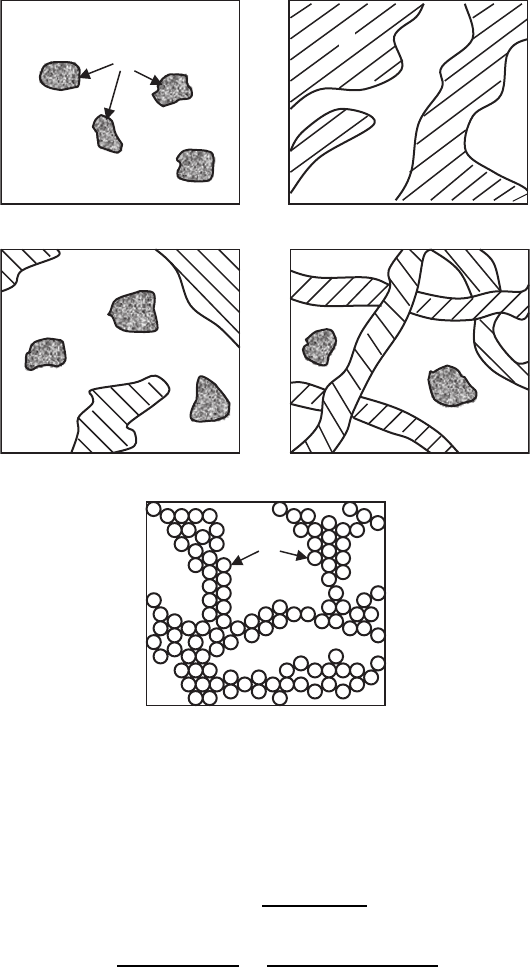
The averaged element for case of the ordered cubic package of balls is
shown in Fig. C.4a. Any ball contacts with six other balls in the points K, L,
M, N, O, P. Four contacts (points M, N, O, P) belong to through pores; the
cross-section area of the through pores is shaded.
First, consider a heat transfer in the elements of first type (with distorted
boundaries of elements). Let the thermal resistance of the distorted element
(A
2
A
1
A
0
B
0
B
1
B
2
) (see Fig. C.3b) be the resistance of “straightened” element
(A
0
A
1
ABB
1
B
0
) with the lateral adiabatic surfaces parallel to the direction
of general heat flux, but cross-section square is the same as initial element
square. Then, the distortion of flux lines in the “straightened” element occurs
only in the plane of near-contact region.
Assumption 2. The near-contact regions of particle in any element of flux
tube are formed by spherical surfaces with mean radius r.
524 C Macrostructure Modeling of Heat Conduction
i
i
+ 1
i + 2
c
c
Q
Q
(a)
i – 1
i
– 3
i
– 2
q
c
c
r
(c)
a
a
•
•
h
c
r
q
B
2
BA
2
A
0
B
1
B
0
A
1
A
(b)
c
a
c
a
α
α
δ
δ
δδ
δδ
Fig. C.3. Account of chaotic character of particle package in frame: (a) flux tubes
in the frame; (b) element of the flux tube of 1st type; (c) element of the flux tube
of 2nd type
The square of spherical surface per contact, S
c
, is the equal to ratio of
total square of the particle surface, S =4πr
2
, to the coordination number,
N
c
,thatis,S
c
=4πr
2
/N
c
. A cross-section of grain part in the flux tube is
presented in the form of circle with radius r
1
(Fig. C.4c). Dependency between
parameters r
1
and N
c
is stated, taking into account the following relations:
r
2
1
= h
bs
(2r − h
bs
); S
c
=2πrh
bs
, (C.21)
where h
bs
is the height of ball segment. Hence
y
1
= r
1
/r =2(N
c
− 1)
1/2
/N
c
. (C.22)
