Marathe K. Topics in Physical Mathematics

Подождите немного. Документ загружается.

28 1Algebra

j(z) −744. The ﬁrst few terms in the Fourier expansion of the function J are

given by

J = q

−1

+ 196,884q +21,493,760q

+ 864,299,970q

+ ··· . (1.33)

We note that the quotient space H/Γ under the action of Γ on H is

a surface of genus zero. In general, we deﬁne the genus of a congruence

subgroup as the genus of the surface obtained as indicated above for Γ .If

G is a congruence subgroup such that the quotient space H/G has genus

zero then it can be shown that every holomorphic function on H/G can

be expressed as a rational function of a single function j

. This function

is called a Hauptmodul for G. The fact that the coeﬃcients of the J-

function are integers has had several arithmetic applications, for example

in the theory of complex multiplication and class ﬁeld theory. The p-adic

properties and congruences of these coeﬃcients have been studied extensively.

But their positivity brings to mind the following classical folk principle of

representation theory: If you meet an interesting natural number, ask if it is

the character degree of some representation. This is exactly the question that

John McKay asked about the number 196, 884. He sent his thoughts about it

to Thompson in November 1978, and this led to the McKay–Thompson series

and the monstrous moonshine. Correspondences of this type are also known

for some other ﬁnite and inﬁnite groups. For example, McKay had found a

relation between the character degrees of irreducible representations of the

complexiﬁed exceptional Lie group E

and the q-coeﬃcients of the cube root

of the J-function.

1.6.3 The monster and the Moonshine Conjectures

Before discussing the relation of the monster with the modular functions

we give some numerology related to this group. This largest of the sporadic

groups is denoted by M,anditsordero(M)equals

808,017,424,794,512,875,886,459,904,961,710,757,005,754,368 ×10

The prime factorization of o(M)isgivenby

o(M)=2

· 3

· 5

· 7

·11

·13

· 17 · 19 ·23 · 29 · 31 · 41 · 47 · 59 · 71.

It has more elements (about 10

) than number of atoms in the earth. We

call the prime factors of the monster the monster primes.Wedenoteby

, the set of all monster primes. Thus,

= {2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 41, 47, 59, 71}.

1.6 Monstrous Moonshine 29

The set π

contains 15 of the ﬁrst 19 primes. The primes 37, 43, and 67

also occur as divisors of the order of some sporadic groups. The only prime

that does not divide the order of any of the sporadic groups among the ﬁrst

19 primes is 61. Thus, 61 can be characterized as the smallest prime that

does not divide the order of any of the sporadic groups. There are a number

of characterizations of the set π

of all monster primes. The theorem below

gives an indication of some deep connection between the monster and the

modular groups.

Theorem 1.6 The group Γ

(p)+ has genus zero if and only if p is a monster

prime, i.e., p divides o(M). Moreover, if the element g ∈ M has order p then

its McKay–Thompson series T

is a Hauptmodul for the group Γ

(p)+.

Remark 1.1 The 15 monster primes are singled out in other situations. For

example, the primes p dividing the o(M) are exactly the primes for which

every supersingular elliptic curve in characteristic p is deﬁned over the ﬁeld

. They also appear in the proof of the Hecke conjecture by Pizer [318].

In 1940 Hecke made a conjecture concerning the representation of modular

forms of weight 2 on the congruence group Γ

(p)(p a prime) by certain

theta series determined by the quaternionic division algebra over the ﬁeld of

rational numbers, ramiﬁed at p and ∞. Pizer proved that the conjecture is

true if and only if p is a monster prime. The 15 monster primes also arise

in Ogg’s work on modular groups. Andrew Ogg was in the audience at a

seminar on the monster given by Jacques Tits in 1975. When he wrote down

the prime factorization of o(M), Ogg was astounded. He knew this list of 15

primes very well through his work on modular groups. He oﬀered a small

prize for anyone who could explain this remarkable coincidence. In spite of a

great deal of work on the properties of the monster and its relations to other

parts of mathematics and physics, this prize remains unclaimed.

Recall that the quantum dimension of a graded vector space was deﬁned

as a power series in the formal variable q.Ifwewriteq =exp(2πiz),z ∈ C

then dim

V can be regarded as the Fourier expansion of a complex func-

tion. A spectacular application of this occurs in the study of ﬁnite groups.

The study of representations of the largest of these groups has led to the

creation of a new ﬁeld of mathematics called vertex algebras; these turn out

to be closely related to the chiral algebras in conformal ﬁeld theory. These

and other ideas inspired by string theory have led to a proof of Conway

and Norton’s moonshine conjectures (see, for example, Borcherds [48], and

the book [139] by Frenkel, Lepowski, and Meurman). The monster Lie al-

gebra is the simplest example of a Lie algebra of physical states of a chiral

string on a 26-dimensional orbifold. This algebra can be deﬁned by using the

inﬁnite-dimensional graded representation V of the monster simple group.

Its quantum dimension is related to Jacobi’s SL(2, Z) Hauptmodul (elliptic

modular function of genus zero) j(q), where q = e

2πiz

,z ∈ H,by

dim

V =J(q):=j(q) −744 = q

−1

+196,884q +21,493,760q

+ 864,299,970q

+ ... .

30 1Algebra

The coeﬃcient 196, 884 in the above formula attracted John McKay’s at-

tention, it being very close to 196,883, the character degree of the smallest

nontrivial irreducible representation of the monster. McKay communicated

his observation to Thompson. We summarize below Thomson’s observations

on the numerology between the monster and the Jacobi modular function J.

Let c(n) denote the coeﬃcient of the nth term in J(q)andletχ

be the nth

irreducible character of the monster group. Then the character degree χ

(1)

is the dimension of the nth irreducible representation of M. We list the ﬁrst

few values of c(n)andχ

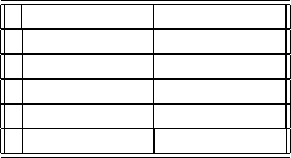
(1) in Table 1.4.

Tab le 1. 4 A strange correspondence

n c(n) χ

(1)

1 1 1

2 196,884 196,883

3 21,493,760 21,296,876

4 864,299,970 842,609,326

5 20,245,856,256 18,538,750,076

A short calculation using the values in Table 1.4 gives the following for-

mulas for the ﬁrst few coeﬃcients of the J-function in terms of the character

degrees of the monster:

c(1) = χ

(1)

c(2) = χ

(1) + χ

(1)

c(3) = χ

(1) + χ

(1)

c(4) = 2χ

(1) + 2χ

(1) + χ

(1)

c(5) = 3χ

(1) + 3χ

(1) + χ

(1) + 2χ

(1) + χ

(1).

These relations led Thompson to ask the following questions:

1. Is there a function theoretic description where the coeﬃcients c(n)equal

the dimension of some vector space V

2. Is there a group G containing the monster as a subgroup that admits V

as a representation space such that the restriction of this representation

to the group M provides the explanation of the entries in Table 1.4.

These two questions are a special case of Conway and Norton’s monstrous

moonshine, the moonshine conjectures, which we now state.

1. For each g ∈ M there exists a function T

(z) with normalized Fourier series

expansion given by

1.6 Monstrous Moonshine 31

(z)=q

−1

∞



(n)q

. (1.34)

There exists a sequence H

of representation of M called the head rep-

resentations such that

(n)=χ

(g), (1.35)

where χ

is the character of H

2. For each g ∈ M, there exists a Hauptmodul J

for some modular group of

genus zero such that T

= J

.Inparticular,

a. T

= J

= J, the Jacobi Hauptmodul for the modular group Γ .

b. If g is an element of prime order p,thenT

is a Hauptmodul for the

modular group Γ

(p)+.

3. Let [g] denote the set of all elements in M that are conjugate to g

,i∈ Z.

Then T

depends only on the class [g]. Note that from equation (1.35), it

follows that T

is a class function in the usual sense. However, [g]isnot

the usual conjugacy class. There are 194 conjugacy classes of M but only

171 distinct McKay–Thompson series.

Conway and Norton calculated all the functions T

and compared their ﬁrst

few coeﬃcients with the coeﬃcients of known genus zero Hauptmoduls. Such

a check turns out to be part of Borcherds’s proof, which he outlined in his

lecture at the 1998 ICM in Berlin [48]. The ﬁrst step was the construction

of the moonshine module. The entire book [139]byFrenkel,Lepowskyand

Meurman is devoted to the construction of this module, denoted by V



.It

has the structure of an algebra called the Moonshine vertex operator

algebra (also denoted by V



). Frenket et al. proved that the automorphism

group of the inﬁnite-dimensional graded algebra V



is the largest of the ﬁnite,

sporadic, simple groups, namely, the monster. We now describe some parts

in the construction of the graded algebra V



which can be written as



∞



i=−1

All the V

are ﬁnite dimensional complex vector spaces with V

−1

one-

dimensional and V

= 0 (corresponding to zero constant term in the Haupt-

modul J). There are two distinguished homogeneous elements:

i) the vacuum vector, denoted by 1 in V

−1

, is the identity element of the

algebra;

ii) the conformal vector (also called the Virasoro vector), denoted by ω ∈

For each v ∈ V



there is given a linear map Y (v, z) with formal parameter z

deﬁned by

Y (v, z)=



n∈Z

−n−1

∈ End(V



32 1Algebra

The operator Y (v, z) is called the vertex operator associated with v.Themap

v → Y (v, z) is called the state-ﬁeld correspondence. These operators

are subject to a number of axioms. For example, the endomorphisms ω

appearing in the operator Y (ω,z) corresponding to the Virasoro vector ω

generate a copy of the Virasoro algebra with central charge 24, acting on V



The magical number 24 appearing here is the same as the dimension of the

Leech lattice. It also plays an important role in number theory and in the

theory of modular forms.

The second step was the construction by Borcherds of the monster Lie

algebra using the moonshine vertex operator algebra V



.Heusedthisal-

gebra to obtain combinatorial recursion relations between the coeﬃcients

(n) of the McKay–Thompson series. It was known that the Hauptmoduls

satisﬁed these relations and that any function satisfying these relations is

uniquely determined by a ﬁnite number of coeﬃcients. In fact, checking the

ﬁrst ﬁve coeﬃcients is suﬃcient for each of the 171 distinct series. The j-

function belongs to a class of functions called replicable functions.This

class also includes the three classical functions, the exponential (q), the sine

((q − 1/q)/(2i)) and the cosine ((q +1/q)/2). McKay has called these three

functions the modular ﬁctions. Modular ﬁctions are known to be the only

replicable functions with ﬁnite Laurent series. All modular functions given

by the 171 distinct series are replicable functions. Thus, all the monstrous

moonshine conjectures are now parts of what we can call the “Moonshine

Theorem” (for more details, see [91], [151], [152]). Its relation to vertex op-

erator algebras, which arise as chiral algebras in conformal ﬁeld theory and

string theory has been established. In spite of the great success of these new

mathematical ideas, many mysteries about the monster are still unexplained.

We end this section with a comment which is a modiﬁcation of the remarks

made by Ogg in [305], when the existence of the monster group and its

relation to modular functions were still conjectures (strongly supported by

computational evidence). Its deep signiﬁcance for theoretical physics is still

emerging; so mathematicians and physicists young and old may ﬁnd exciting

emergence of a new subject, guaranteed to be rich and varied and deep, with

many new questions to be asked and many of the conjectured results yet to be

proved. It is indeed quite extraordinary that new light should be shed on the

theory of modular functions, one of the most beautiful and extensively studied

areas of classical mathematics, by the largest and the most exotic sporadic

group, the monster. That its interaction goes beyond mathematics, into areas

of theoretical physics such as conformal ﬁeld theory, chiral algebras,

and

string theory, may indicate that the ﬁeld of physical mathematics is rich and

worthy of deeper study.

A discussion of chiral algebras from a mathematical point of view may be found in the

book by Beilinson and Drinfeld [35]. For vertex operator algebras and their relation to

CFT see, for example, [138, 197, 250].

Chapter 2

Topology

2.1 Introduction

Several areas of research in modern mathematics have developed as a result

of interaction between two or more specialized areas. For example, the sub-

ject of algebraic topology associates with topological spaces various algebraic

structures and uses their properties to answer topological questions. An el-

egant proof of the theorem that R

and R

with their respective standard

topologies, are not homeomorphic for m = n is provided by computing the

homology of the one point compactiﬁcation of these spaces. Indeed, the prob-

lem of classifying topological spaces up to homeomorphism was fundamental

in the creation of algebraic topology. In general, however, the knowledge of

these algebraic structures is not enough to decide whether two topological

spaces are homeomorphic. The equivalence of algebraic structures follows

from a weaker relation among topological spaces, namely, that of homotopy

equivalence. In fact, homotopy equivalent spaces have isomorphic homotopy

and homology structures. Equivalence of algebraic structures associated to

two topological spaces is a necessary but not suﬃcient condition for their

homeomorphism. Thus, one may think of homotopy and homology as provid-

ing obstructions to the existence of homeomorphisms. As we impose further

structure on a topological space such as piecewise linear, diﬀerentiable, or

analytic structures other obstructions may arise.

Forexample,itiswellknownthatR

,n= 4, with the standard topology

admits a unique compatible diﬀerential structure. On the other hand, as a

result of the study of the moduli spaces of instantons by Donaldson and the

classiﬁcation of four-dimensional topological manifolds by Freedman, it fol-

lows that R

admits an uncountable number of non-diﬀeomorphic structures.

In the case of the standard sphere S

⊂ R

n+1

, the generalized Poincar´econ-

jecture states that a compact n-dimensional manifold homotopically equiv-

alent to S

is homeomorphic to S

. This conjecture is now known to be

true for all n and is one of the most interesting recent results in algebraic

K. Marathe, Topics in Physical Mathematics, DOI 10.1007/978-1-84882-939-8 2, 33

 Springer-Verlag London Limited 2010

34 2 Topology

topology. The case n = 2 is classical. For n>4 it is due to Stephen Smale.

Smale (b. 1930) received a Fields Medal at the ICM 1966 held in Moscow

for his contributions to various aspects of diﬀerential topology and, in par-

ticular, to his novel use of Morse theory, which led him to his solution of the

generalized Poincar´e conjecture for n>4. Smale has extensive work in the

application of dynamical systems to physical processes and to economic equi-

libria. His discovery of strange attractors led naturally to chaotic dynamical

systems. The result for n = 4 is due to Michael Hartley Freedman (b. 1951),

who received a Fields Medal at ICM 1986

held in Berkeley for his complete

classiﬁcation of all compact simply connected topological 4-manifolds, which

leads to his proof of the Poincar´e conjecture. The original Poincar´e conjecture

was recently proved by Grigory Yakovlevich Perelman (b. 1966). Perelman

received a Fields Medal at ICM 2006 held in Madrid for his fundamental con-

tributions to geometry and for his revolutionary insights into the analytical

and geometric structure of the Ricci ﬂow. He studied the geometric topology

of 3-manifolds by extending Hamilton’s Ricci ﬂow ideas. While he did not

publish his work in a ﬁnal form, it contains all the essential ingredients of

a proof of the Thruston geometrization conjectures and in particular of the

original Poincar´e conjecture (the case n = 3). This problem is one of the

seven, million dollar Clay Prize problems. As of this writing, it is not known

if and when he will get this prize. We will discuss the topology of 3- and

4-manifolds later in this chapter.

In the category of diﬀerentiable manifolds, it was shown by John Willard

Milnor that S

admits an exotic diﬀerential structure, i.e., a structure not

diﬀeomorphic to the standard one. This work ushered in the new ﬁeld of

diﬀerential topology. Milnor was awarded a Fields Medal at the ICM 1962

held in Stockholm for his fundamental work in diﬀerential geometry and

topology. Using homotopy theory, Kervaire and Milnor proved the striking

result that the number of distinct diﬀerentiable structures on S

is ﬁnite for

any n =4.Forn =1, 2, 3, 5, 6, there is a unique diﬀerential structure on the

standard n-sphere. As of this writing (May 2010) there is no information on

the number of distinct diﬀerentiable structures on S

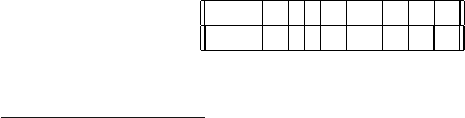
. The following table

gives a partial list of the number of diﬀeomorphism classes [S

]ofn-spheres.

Tab le 2. 1 Number of diﬀeomorphism classes of n-spheres

n 7 8 9 10 11 12 13 14

#[S

] 28 2 8 6 992 1 3 2

The year was the 50th anniversary of the inception of Fields medals. However, several

mathematicians including invited speakers were denied U.S. visas. Their papers were read

by other mathematicians in a show of solidarity.

2.2 Point Set Topology 35

We note that the set of these equivalence classes can be given a structure

of a group denoted by θ

. Milnor showed that θ

is cyclic group of order

28. Brieskorn has constructed geometric representatives of the elements of θ

as 7-dimensional Brieskorn spheres Σ(6m − 1, 3, 2, 2, 2), 1 ≤ m ≤ 28, by

generalizing the Poincar´e homology spheres in three dimensions. Thus the

mth sphere is the intersection of S

⊂ C

with the space of solutions of the

equation

6m−1

+ z

=0, 1 ≤ m ≤ 28,z

∈ C, 1 ≤ i ≤ 5.

Until recently, such considerations would have seemed too exotic to be of

utility in physical applications. However, topological methods have become

increasingly important in classical and quantum ﬁeld theories. In particular,

several invariants associated to homotopy and homology of a manifold have

appeared in physical theories as topological quantum numbers. In the re-

maining sections of this chapter and in the next chapter is a detailed account

of some of the most important topics in this area.

2.2 Point Set Topology

Point set topology is one of the core areas in modern mathematics. How-

ever, unlike algebraic structures, topological structures are not familiar to

physicists. In this appendix we collect some basic deﬁnitions and results con-

cerning topological spaces. Topological concepts are playing an increasingly

important role in physical applications, some of which are mentioned here.

Let X be a set and P(X)thepower set of X, i.e., the class of all subsets

of X. T⊂P(X) is called a topology on X if the following conditions are

satisﬁed:

1. {∅,X}⊂T;

2. if A, B ∈T,thenA ∩B ∈T;

3. if { U

| i ∈ I}⊂T,then



i∈I

∈T,whereI is an arbitrary indexing

set.

The pair (X,T ) is called a topological space. It is customary to refer to X

as a topological space when the topology T is understood. An element of T

is called an open set of (X, T ). If W ⊂ X,thenT

:= {W ∩A | A ∈T}is a

topology on W called the relative topology on W induced by the topology

T on X.

Example 2.1 Let T = {∅,X}.ThenT is called the indiscrete topology on

X.IfT = P(X),thenT is called the discrete topology on X.If{T

| i ∈ I}

is a family of topologies on X,then



| i ∈ I} is also a topology on X.

36 2 Topology

The indiscrete and the discrete topologies are frequently called trivial

topologies. An important example of a nontrivial topology is given by the

metric topology.

Example 2.2 Let R

denote the set of nonnegative real numbers. A metric

or a distance function on X,isafunctiond : X × X → R

satisfying,

∀x, y, z ∈ X, the following properties:

1. d(x, y)=d(y,x), symmetry;

2. d(x, y)=0if and only if x = y, non-degeneracy;

3. d(x, y) ≤ d(x, z)+d(z,y), triangle inequality.

The pair (X, d) is called a metric space. If (X, d) is a metric space, we can

make it into a topological space with topology T

deﬁned as follows. T

is the

class of all subsets U ⊂ X such that

∀x ∈ U, ∃>0, such that B(, x):={y ∈ X | d(x, y) <}⊂U.

The set B(, x) is called an -ball around x and is itself in T

. R

with the

usual Euclidean distance function is a metric space. The corresponding topol-

ogy is called the standard topology on R

. The relative topology on S

n−1

⊂ R

is called the standard topology on S

n−1

A topological space (X, T ) is said to be metrizable if there exists a distance

function d on X such that T = T

. It is well known that Riemannian mani-

folds are metrizable. It is shown in [256] that pseudo-Riemannian manifolds,

and in particular, space-time manifolds, are also metrizable.

Let (X, T

)and(Y,T

) be topological spaces. A function f : X → Y is

said to be continuous if ∀V ∈T

−1

(V ) ∈T

.Iff is a continuous bijection

and f

−1

is also continuous, then f is called a homeomorphism between X

and Y . Homeomorphism is an equivalence relation on the class of topological

spaces. A property of topological spaces preserved under homeomorphisms

is called a topological property. For example, metrizability is a topological

property.

Let (X

, T

),i∈ I, be a family of topological spaces and let X =



i∈I

be the Cartesian product of the family of sets {X

| i ∈ I}.Letπ

: X → X

be the canonical projection. Let {S

| j ∈ J} be the family of all topologies

on X such that π

is continuous for all i ∈ I.IfS =



| j ∈ J}, then

S is called the product topology on X. We observe that it is the smallest

topology on X such that all the π

are continuous.

Let (X, T ) be a topological space, Y aset,andf : X → Y a surjection.

The class T

deﬁned by

:= {V ⊂ Y | f

−1

(V ) ∈T}

is a topology on Y called the quotient topology on Y deﬁned by f . T

the largest topology on Y with respect to which f is continuous. We observe

that if ρ is an equivalence relation on X, Y = X/ρ is the set of equivalence

2.2 Point Set Topology 37

classes and π : X → Y is the canonical projection, then Y with the quotient

topology T

is called quotient topological space of X by ρ.

Let (X, T ) be a topological space and let A, B, C denote subsets of X. C

is said to be closed if X \ C is open. The closure

A or cl(A)ofA is deﬁned

A :=



{F ⊂ X | F is closed and A ⊂ F }.

Thus,

A is the smallest closed set containing A. It follows that C is closed

if and only if C =

C.Letf : X → Y be a function. We deﬁne supp f,the

support of f to be the set cl{x ∈ X | f (x) =0}. A subset A ⊂ X is said to

be dense in X if

A = X. X is said to be separable if it contains a countable

dense subset. A is said to be a neighborhood of x ∈ X if

there exists U ∈T

suc

h that x ∈ U ⊂ A.WedenotebyN

the class of neighborhoods of x.A

subclass B⊂T∩N

is called a local base at x ∈ X if for each neighborhood

A of x there exists U ∈Bsuch that U ⊂ A. X is said to be ﬁrst countable if

each point in X admits a countable local base. A subclass B⊂T is called a

base for T if ∀A ∈T, ∀x ∈ A, there exists U ∈Bsuch that x ∈ U ⊂ A. X is

said to be second countable if its topology has a countable base. A subclass

S⊂T is called a subbase for T if the class of ﬁnite intersections of elements

of S is a base for T . Any metric space is ﬁrst countable but not necessarily

second countable. First and second countability are topological properties.

We now give some further important topological properties.

X is said to be a Hausdorﬀ space if ∀x, y ∈ X,thereexistA, B ∈T

such that x ∈ A, y ∈ B and A ∩ B = ∅. Such a topology is said to separate

points and the Hausdorﬀ property is one of a family of separation axioms for

topological spaces. The Hausdorﬀ property implies that ﬁnite subsets of X

areclosed.Ametricspace is a Hausdorﬀ space.

A family U = {U

| i ∈ I} of subsets of X is said to be a cover or a

covering of A ⊂ X if A ⊂



U.Acover{V

| j ∈ J} of A ⊂ X is called

a reﬁnement of U if, for all j ∈ J, V

⊂ U

for some i ∈ I.Acoveringby

open sets is called an open covering. A ⊂ X is said to be compact if every

open covering of A has a ﬁnite reﬁnement or, equivalently, if it has a ﬁnite

subcovering. The continuous image of a compact set is compact. It follows

that compactness is a topological property. The Heine–Borel theorem

asserts that a subset of R

is compact if and only if it is closed and bounded. A

consequence of this is the extreme value theorem, which asserts that every

continuous real-valued function on a compact space attains its maximum and

minimum values. A Hausdorﬀ space X is said to be paracompact if every

open covering of X has a locally ﬁnite open reﬁnement, i.e., each point has

a neighborhood that intersects only ﬁnitely many sets of the reﬁnement. A

family F = {f

: X → R | i ∈ I} of functions is said to be locally ﬁnite if

each x ∈ X has a neighborhood U such that f

(U) = 0, for all but a ﬁnite

subset of I. A family F of continuous functions is said to be a partition of

unity if it is a locally ﬁnite family of nonnegative functions and