Jost J. Partial Differential Equations
Подождите немного. Документ загружается.


306 12. Moser Iteration Method and Regularity Theorem of de Giorgi and Nash
−
Ω
ϕdx =
1
|Ω|
Ω
ϕdx.
In order to familiarize ourselves with the notions of sub- and supersolutions,
we shall demonstrate the following useful lemma.
Lemma 12.1.1: (i) Let u be a subsolution, i.e. u ∈ C
2
(Ω),Lu≥ 0,and
let f ∈ C
2
(R) be convex with f
≥ 0. Then f ◦ u is a subsolution as
well.
(ii) Let u be a supersolution, f ∈ C
2
(R) concave with f
≥ 0. Then f ◦u is
a supersolution as well.
(iii) Let u be a solution, and f ∈ C
2
(R) convex. Then f ◦u is a subsolution.
Proof:
L(f ◦ u)=
i,j
∂
∂x
j
a
ij
f
(u)
∂u
∂x
i
= f
(u)
i,j
a
ij
∂u
∂x
i
∂u
∂x
j
+ f
(u)Lu,
(12.1.3)
which implies all the inequalities claimed.
We now wish to verify that the assertions of Lemma 12.1.1 continue to hold
for weak (sub-, super-)solutions. We assume that f
(u)andf
(u) satisfy
approximate integrability conditions to make the chain rules for weak deriva-
tives
D
i
(f ◦ u)=f
(u)D
i
(u)
and
D
i
(f
◦ u)=f
(u)D
i
u for i =1, ..., d
valid. (By Lemma 8.2.3 this holds if, for example,
sup
y∈R
|f
(y)| +sup
y∈R
|f
(y)| < ∞.)
We obtain
Ω
i,j
a
ij
D
i
(f ◦ u)D
j
ϕ =
i,j
a
ij
f
(u)D
i
uD
j
ϕ
=
i,j
a
ij
D
i
uD
j
(f
(u)ϕ)
−
i,j
a
ij
D
i
uf
(u)D
j
uϕ.
The last integral is nonnegative because of the ellipticity condition, if f is
convex, i.e., f
(u) ≥ 0, and ϕ ≥ 0, and consequently yields a nonpositive

12.1 The Moser–Harnack Inequality 307
contributionbecauseoftheminussigninfrontofit,ifu is a weak subsolution
and f
(u) ≥ 0. Therefore, under those assumptions
Ω
i,j
a
ij
D
i
(f ◦ u)D
j
ϕ ≤ 0,
and f ◦ u is a weak subsolution.
In the same manner, one treats the weak versions of the other assertions
of Lemma 12.1.1 to obtain the following result:
Lemma 12.1.2: Under the corresponding assumptions, the assertions of
Lemma 12.1.1 hold for weak (sub-,super-)solutions, provided that the chain
rule for weak derivatives is satisfied for f ∈ C
2
(R).
From Lemma 12.1.2 we derive the following result:
Lemma 12.1.3: Let u ∈ W
1,2
(Ω) be a weak subsolution of L,andk ∈ R.
Then
v(x) := max(u(x),k)
is a weak subsolution as well.
Proof: We consider the function
f : R → R,
f(y) := max(y,k).
Then
v = f ◦ u.
We approximate f by a sequence (f
n
)
n∈N
of convex functions of class C
2
with
f
n
(y)=f(y)fory ∈
k −
1
n
,k+
1
n
and
|f
n
(y)|≤1 for all y.
Then, as in the proofs of Lemmas 8.2.2 and 8.2.3, by an approximation ar-
gument, f
n
◦ u converges to v = f ◦ u in W
1,2
. Therefore,
Ω
i,j
a
ij
D
i
vD
j
ϕ = lim
n→∞
Ω
i,j
a
ij
D
i
(f
n
◦ u)D
j
ϕ
≤ 0forϕ ∈ H
1,2
0
(Ω),ϕ ≥ 0
by Lemma 12.1.2.
308 12. Moser Iteration Method and Regularity Theorem of de Giorgi and Nash
Remark: Of course, we also have a result analoguous to Lemma 12.1.3 for
weak supersolutions. For k ∈ R,ifu ∈ W
1,2
(Ω) is a weak supersolution, then
so is
min(u(x),k).
We now come to the fundamental estimates of J. Moser:
Theorem 12.1.1: Let u be a subsolution in the ball B(x
0
, 4R) ⊂ R
d
(R>
0), and assume p>1. Then
sup
B(x
0
,R)
u ≤ c
1
p
p − 1
2
p
−
B(x
0
,2R)
(max(u(x), 0))
p
dx
1
p
, (12.1.4)
with a constant c
1
depending only on d and
Λ
λ
.
Remark: If u is positive, then obviously max(u, 0) = u in (12.1.4), and this
case will constitute our main application of this result.
Theorem 12.1.2: Let u be a positive supersolution in B(x
0
, 4R) ⊂ R
d
.For
0 <p<
d
d−2
,andifd ≥ 3, then
−
B(x
0
,2R)
u
p
dx
1
p
≤
c
2
d
d−2
− p
2
inf
B(x
0
,R)
u, (12.1.5)
with c
2
again depending on d and
Λ
λ
only. If d =2, this estimate holds
for any 0 <p<∞, with a constant c
2
depending on p, d,
Λ
λ
in place of
c
2
/
d
d−2
− p
2
.
Remark: In order to see the necessity of the condition p<
d
d−2
,weletL be
the Laplace operator Δ and
u(x)=min
|x|
2−d
,k
for some k>0.
According to the remark after Lemma 12.1.3, because |x|
2−d
is harmonic on
R
d
\{0}, this is a weak supersolution on R
d
.Ifwethenletk increase, we see
that the L
d
d−2
-norm can no longer be controlled by the infimum.
From Theorems 12.1.1 and 12.1.2 we derive Harnack-type inequalities for
solutions of Lu = 0. These two theorems directly yield the following corollary:
Corollary 12.1.1: Let u be a positive (weak) solution of Lu =0in the ball
B(x
0
, 4R) ⊂ R
d
(R>0). Then
sup
B(x
0
,R)
u ≤ c
3
inf
B(x
0
,R)
u, (12.1.6)
with c
3
depending on d and
Λ
λ
only.

12.1 The Moser–Harnack Inequality 309
For general domains, we have the following result:
Corollary 12.1.2: Let u be a positive (weak) solution of Lu =0in a domain
Ω of R
d
,andletΩ
0
⊂⊂ Ω. Then
sup
Ω
0
u ≤ c inf
Ω
0
u, (12.1.7)
with c depending on d, Ω, Ω
0
,and
Λ
λ
.
Proof: This Harnack inequality on Ω
0
follows by the standard ball chain
argument: Since
¯
Ω
0
is compact, it can be covered by finitely many balls B
i
:=
B(x
i
,R)withB(x
i
,R) ⊂ Ω (we choose, for example, R<
1
4
dist(∂Ω,Ω
0
)),
i =1,...,N.Nowlety
1
,y
2
∈ Ω
0
; without loss of generality y
1
∈ B
k
, y
2
∈
B
k+m
for some m ≥ 1, and the balls are enumerated in such manner that
B
j
∩ B
j+1
= ∅ for j = k,...,k+ m − 1. By applying Corollary 12.1.1 to the
balls B
k
,B
k+1
,..., we obtain
u(y
1
) ≤ sup
B
k
u(x) ≤ c
3
inf
B
k
u(x)
≤ c
3
sup
B
k+1
u(x)(sinceB
k
∩ B
k+1
= ∅)
≤ c
2
3
inf
B
k+1
u(x) ≤ ...
≤ c
m+1
3
inf
B
k+m
u(x) ≤ c
m+1
3
u(y
2
).
Since y
1
and y
2
are arbitrary, and m ≤ N, it follows that
sup
Ω
0
u(x) ≤ c
N+1
3
inf
Ω
0
u(x). (12.1.8)
We now start with the preparations for the proofs of Theorems 12.1.1 and
12.1.2. For positive u and a point x
0
, we put
φ(p, R):=
−
B(x
0
,R)
u
p
dx
1
p
.
Lemma 12.1.4:
lim
p→∞
φ(p, R)= sup
B(x
0
,R)
u =: φ(∞,R), (12.1.9)
lim
p→−∞
φ(p, R)= inf
B(x
0
,R)
u =: φ(−∞,R). (12.1.10)
Proof: By H¨older’s inequality, φ(p, R) is monotonically increasing with re-
spect to p. Namely, for p<p
and u ∈ L
p
(Ω),
310 12. Moser Iteration Method and Regularity Theorem of de Giorgi and Nash
1
|Ω|
Ω
u
p
1
p
≤
1
|Ω|
1
p
Ω
1
p
−p
pp
Ω
(u
p
)
p
p
1
p
=
1
|Ω|
Ω
u
p
1
p
.
Moreover,
φ(p, R) ≤
1
|B(x
0
,R)|
B(x
0
,R)
(sup u)
p
1
p
= φ(∞,R). (12.1.11)
On the other hand, by the definition of the essential supremum, for any ε>0
there exists some δ>0with
x ∈ B(x
0
,R):u(x) ≥ sup
B(x
0
,R)
u − ε
>δ.
Therefore,
φ(p, R) ≥
1
|B(x
0
,R)|
u(x)≥sup u−ε
x∈B(x
0
,R)
u
p
1
p
≥
δ
|B(x
0
,R)|
1
p
(sup u − ε),
and hence
lim
p→∞
φ(p, R) ≥ sup u − ε
for any ε>0, and thus also
lim
p→∞
φ(p, R) ≥ sup u. (12.1.12)
Inequalities (12.1.11) and (12.1.12) imply (12.1.9), and (12.1.10) is derived
similarly (or, alternatively, by applying the preceding argument to
1
u
).
Lemma 12.1.5: (i) Let u be a positive subsolution in Ω,andforq>
1
2
,
assume
v := u
q
∈ L
2
(Ω).
For any η ∈ H
1,2
0
(Ω), we then have
Ω
η
2
|Dv|
2
≤
Λ
2
λ
2
2q
2q − 1
2
Ω
|Dη|
2
v
2
. (12.1.13)
(ii) If u is a supersolution instead, this inequality holds for q<
1
2
.
Proof: The claim is trivial for q = 0. We put
f(u)=u
2q
for q>0,
f(u)=−u
2q
for q<0.
12.1 The Moser–Harnack Inequality 311
By Lemma 12.1.2, f(u) then is a subsolution in case (i), and a supersolution
in case (ii). The subsequent calculations are based on that fact. (In the course
of the proof there will also arise integrability conditions implying the needed
chain rules. For that purpose, the proof of Lemma 8.2.3 requires a slight
generalization, utilizing varying Sobolev exponents, the H¨older inequality,
and the Sobolev embedding theorem. We leave this as an exercise for the
reader.) As a test function in (12.1.2) (or in the corresponding inequality in
case (ii), we then use
ϕ = f
(u) · η
2
. (12.1.14)
Then
Ω
ij
a
ij
(x)D
i
uD
j
ϕ
=
Ω
i,j
a
ij
D
i
uD
j
uf
(u)η
2
+
Ω
i,j
a
ij
D
i
uf
(u)2ηD
j
η
=
Ω
2 |q|(2q − 1)
i,j
a
ij
D
i
uD
j
uu
2q−2
η
2
+
Ω
4 |q|
i,j
a
ij
D
i
uu
2q−1
ηD
j
η.
(12.1.15)
In case (i), this is ≤ 0. Applying Young’s inequality to the last term, for all
ε>0, we obtain
2 |q|(2q − 1)λ
|Du|
2
u
2q−2
η
2
≤ 2 |q|Λε
|Du|
2
u
2q−2
η
2
+
2 |q|Λ
ε
u
2q
|Dη|
2
.
With
ε =
2q − 1
2
λ
Λ
,
we thus obtain
|Du|
2
u
2q−2
η
2
≤
4
(2q − 1)
2
Λ
2
λ
2
u
2q
|Dη|
2
,
i.e.,
|Dv|
2
η
2
≤
Λ
2
λ
2
2q
2q − 1
2
v
2
|Dη|
2
.
In case (ii), (12.1.15) is nonnegative, and since in that case also 2q − 1 ≤ 0,
one can proceed analoguously and put
ε =
1 − 2q
2
λ
Λ
,
to obtain (12.1.13) in that case as well.

312 12. Moser Iteration Method and Regularity Theorem of de Giorgi and Nash
We now begin the proofs of Theorems 12.1.1 and 12.1.2. Since the stated
inequalities are invariant under scaling, we may assume, without loss of gen-
erality, that
R = 1 and x
0
=0.
We shall employ the abbreviation
B
r
:= B(0,r).
Let
0 <r
<r≤ 2r
, (12.1.16)
and let η ∈ H
1,2
0
(B
r
) be a cutoff function satisfying
η ≡ 1onB
r
,
η ≡ 0onR
d
\ B
r
,
|Dη|≤
2
r − r
.
(12.1.17)
For the proof of Theorem 12.1.1, we may assume without loss of generality
that u is positive, since otherwise, by Lemma 12.1.3, we may consider the
positive subsolutions
v
k
(x)=max(u(x),k)
for k>0 (or the approximating subsolutions from the proof of that lemma),
perform the subsequent reasoning for positive subsolutions, apply the result
to the v
k
, and finally let k tend to 0.
We consider once more
v = u
q
and assume that v ∈ L
2
(Ω). By the Sobolev embedding theorem (Corol-
lary 9.1.3), for d ≥ 3, we obtain
−
B
r
v
2d
d−2
d−2
d
≤ c
4
r
2
−
B
r
|Dv|
2
+ −
B
r
v
2
. (12.1.18)
If d = 2 instead of
2d
d−2
, we may take an arbitrarily large exponent p and
proceed analogously. We leave the necessary modifications for the case d =2
to the reader and henceforth treat only the case d ≥ 3. With (12.1.13) and
(12.1.17), (12.1.18) yields
−
B
r
v
2d
d−2
d−2
d
≤ ¯c −
B
r
v
2
(12.1.19)
12.1 The Moser–Harnack Inequality 313
with
¯c ≤ c
5
r
r − r
2
2q
2q − 1
2
+1
. (12.1.20)
Thus, we get v ∈ L
2d
d−2
(Ω). We shall iterate that step and realize that higher
and higher powers of u are integrable.
We put s =2q and assume
|s|≥μ>0,
choosing an appropriate value for μ later on. Because of r ≤ 2r
,then
¯c ≤ c
6
r
r − r
2
s
s − 1
2
, (12.1.21)
with c
6
also depending on μ. Thus, by (12.1.19) and (12.1.21), since v = u
s
2
,
we get for s ≥ μ,
φ
ds
d − 2
,r
=
−
B
r
v
2d
d−2
d−2
ds
≤ c
7
r
r − r
2
s
s
s − 1
2
s
φ(s, r)
(12.1.22)
with c
7
= c
1
s
6
.Fors ≤−μ, analogously,
φ
ds
d − 2
,r
≥
1
c
7
r
r − r
−
2
|s|
φ(s, r) (12.1.23)
(we may omit the term
s
s−1
−
2
|s|
here, since it is greater than or equal to
1).
We now wish to complete the proof of Theorem 12.1.1, and therefore, we
return to (12.1.22). The decisive insight obtained so far is that we can control
the integral of a higher power of u by that of a lower power of u.Wenow
shall simply iterate this estimate to control even higher integral norms of u
and from Lemma 12.1.4 then also the supremum of u. For that purpose, let
s
n
=
d
d − 2
n
p for p>1,
r
n
=1+2
−n
,
r
n
= r
n+1
>
r
n
2
.
Then (12.1.22) implies
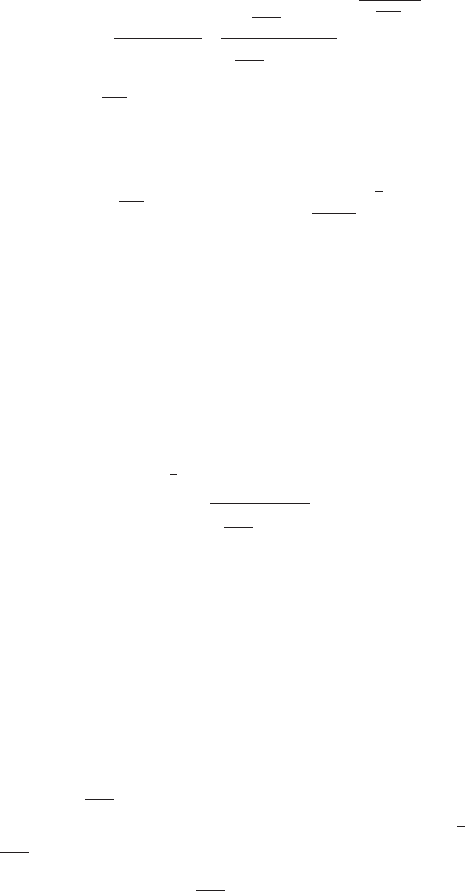
314 12. Moser Iteration Method and Regularity Theorem of de Giorgi and Nash
φ (s
n+1
,r
n+1
) ≤ c
7
⎛
⎝
1+2
−n−1
2
−n−1
·
d
d−2
n
p
d
d−2
n
p − 1
⎞
⎠
2
p
(
d
d−2
)
n
φ(s
n
,r
n
)
= c
n
(
d
d−2
)
−n
8
φ(s
n
,r
n
),
and iteratively,
φ(s
n+1
,r
n+1
) ≤ c
n
ν=1
ν
(
d
d−2
)
−ν
8
φ(s
1
,r
1
) ≤ c
9
p
p − 1
2
p
φ(p, 2). (12.1.24)
(Since we may assume u ∈ L
p
(Ω), therefore φ(s
n
,r
n
) is finite for all n ∈ N,
and thus any power of u is integrable.) Using Lemma 12.1.4, this yields
Theorem 12.1.1.
In order to prove Theorem 12.1.2, we now assume u>ε>0, in order
to ensure that φ(σ, r) is finite for σ<0. This does not constitute a serious
restriction, because once we have proved Theorem 12.1.2 under that assump-
tion, then for positive u, we may apply the result to u + ε. In the resulting
inequality for u + ε, namely
−
B(x
0
,2R)
(u + ε)
p
1
p
≤
c
2
d
d−2
− p
2
inf
B(x
0
,R)
(u + ε),
we then simply let ε → 0 to deduce the inequality for u itself.
Carrying out the above iteration analogously for s ≤−μ with r
n
=2+
2
−n
, we deduce from (12.1.23) that
φ(−μ, 3) ≤ c
10
φ(−∞, 2) ≤ c
10
φ(−∞, 1). (12.1.25)
By finitely many iteration steps, we also obtain
φ(p, 2) ≤ c
11
φ(μ, 3). (12.1.26)
(The restriction p<
d
d−2
in Theorem 12.1.2 arises because according to
Lemma 12.1.5, in (12.1.19) we may insert v = u
q
only for q<
1
2
.There-
lation p =2q
d
d−2
that is needed to control the L
p
-norm of u with (12.1.19),
by (12.1.20) also yields the factor
d
d−2
− p
−2
in (12.1.15).)
The only missing step is
φ(μ, 3) ≤ c
12
φ(−μ, 3). (12.1.27)
Inequalities (12.1.25), (12.1.26), (12.1.27) imply Theorem 12.1.2. For the
proof of (12.1.27), we shall use the theorem of John–Nirenberg (Theo-
rem 9.1.2). For that purpose, we put
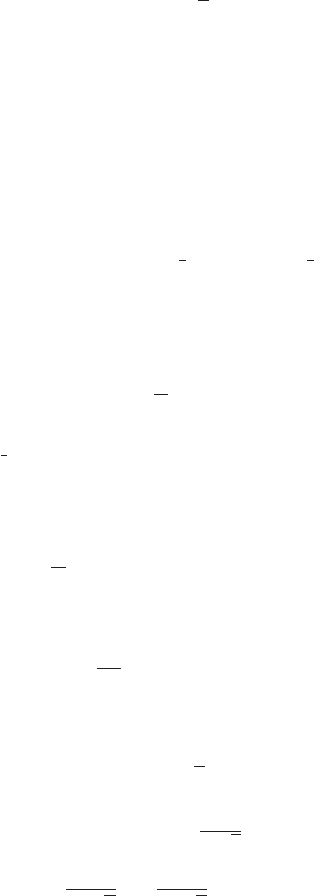
12.1 The Moser–Harnack Inequality 315
v =logu, ϕ =
1
u
η
2
with some cutoff function η ∈ H
1,2
0
(B
4
). Then
B
4
i,j
a
ij
D
i
ϕD
j
u = −
B
4
η
2
a
ij
D
i
vD
j
v +
B
4
2η
a
ij
D
i
ηD
j
v.
Since u is a supersolution, the left-hand side is nonnegative; hence
λ
B
4
η
2
|Dv|
2
≤
B
4
η
2
a
ij
D
i
vD
j
v ≤ 2
B
4
η
a
ij
D
i
ηD
j
v
≤ 2Λ
B
4
η
2
|Dv|
2
1
2
B
4
|Dη|
2
1
2
by the Schwarz inequality, and thus
B
4
η
2
|Dv|
2
≤ 4
Λ
λ
2
B
4
|Dη|
2
. (12.1.28)
If now B(y, R) ⊂ B
3+
1
2
is any ball, we choose η satisfying
η ≡ 1onB(y,R),
η ≡ 0 outside of B(y,2R) ∩ B
4
,
|Dη|≤
6
R
.
Withsuchanη, we obtain from (12.1.28)
−
B(y,R)
|Dv|
2
≤ γ
1
R
2
with some constant γ.
Thus,byH¨older’s inequality
B(y,R)
|Dv|≤ω
d
√
γR
d−1
.
Now let α be as in Theorem 9.1.2. With μ =
α
ω
d
√
γ
, applying that theorem to
w =
1
ω
d
√
γ
v =
1
ω
d
√
γ
log u,
we obtain
B
3
u
μ
B
3
u
−μ
≤ β
2
,
and hence
