Jost J. Partial Differential Equations

Подождите немного. Документ загружается.

11.1 C

-Regularity Theory for the Poisson Equation 287

(a) If f ∈ C

(Ω), then u ∈ C

1,α

(Ω),and

u

1,α

(Ω

)

≤ c



f

(Ω)

+ u

(Ω)



. (11.1.20)

(b) If f ∈ C

(Ω), then u ∈ C

2,α

(Ω),and

u

2,α

(Ω)

≤ c



f

(Ω)

+ u

(Ω)



. (11.1.21)

Remark: The restriction 0 <α<1 is essential for Theorem 11.1.2, as well

as for the subsequent results. For example, in some neighborhood of 0, the

function







log







satisﬁes the inequality

|u| + |Δu|≤const,

while the mixed second derivative

∂

∂x

behaves like

log







Consequently, the C

1,1

-norm of u cannot be controlled by pointwise bounds

for f := Δu and u.

Proof: We demonstrate the estimates (11.1.20) and (11.1.21) ﬁrst under the

assumption u ∈ C

2,α

(Ω). We may cover Ω

by ﬁnitely many balls that are

contained in Ω. Therefore, it suﬃces to verify the estimates for the case

= B(0,r),

Ω = B(0,R), 0 <r<R<∞.

Let 0 <R

<R. We choose some η ∈ C

∞

(B(0,R

)) with 0 ≤ η ≤ 1,

η(x)=1for|x|≤R

,and

η

k,α

(B(0,R

))

≤ c

− R

)

−k−α

. (11.1.22)

We put

φ := ηu. (11.1.23)

Then φ vanishes outside of B(0,R

), and by (1.1.7),

φ(x)=



Γ (x, y)Δφ(y)dy. (11.1.24)

Here,

288 11. Existence Techniques IV

Δφ = ηΔu +2Du · Dη + uΔη, (11.1.25)

and so

Δφ

≤Δu

+ c

η

·u

, (11.1.26)

and by Lemma 11.1.1

Δφ

≤ c

η

2,α

(Δu

+ u

1,α

) , (11.1.27)

where all norms are computed on B(0,R

). From Theorem 11.1.1 and

(11.1.26) and (11.1.27), we obtain

φ

1,α

≤ c

(Δu

+ η

u

) (11.1.28)

and

φ

2,α

≤ c

η

2,α

(Δu

+ u

1,α

) , (11.1.29)

respectively. Since u(x)=φ(x)for|x|≤R

, and recalling (11.1.22), we

obtain

u

1,α

(B(0,R

))

≤ c



Δu

(B(0,R

))

− R

)

u

(B(0,R

))



(11.1.30)

and

u

2,α

(B(0,R

))

≤ c

− R

)

2+α



Δu

(B(0,R

))

+ u

1,α

(B(0,R

))



(11.1.31)

respectively.

We now interrupt the proof for some auxiliary results:

Lemma 11.1.2:

a) There exists a constant c

such that for every ρ>0 and any function

v ∈ C

(B(0,ρ))

v

(B(0,ρ))

≤Dv

(B(0,ρ))

+ c

v

(B(0,ρ))

. (11.1.32)

b) There exists a constant c

such that for every ρ>0 and any function

v ∈ C

1,α

(B(0,ρ))

v

(B(0,ρ))

≤|Dv|

(B(0,ρ))

+ c

v

(B(0,ρ))

(11.1.33)

(here, |Dv|

is the H¨older seminorm deﬁned in (11.1.2)).

11.1 C

-Regularity Theory for the Poisson Equation 289

Proof: If a) did not hold, for every n ∈ N, we could ﬁnd a radius ρ

and a

function v

∈ C

(B(0,ρ

)) with

1=v



(B(0,ρ

))

≥Dv



(B(0,ρ

))

+ nv



(B(0,ρ

))

. (11.1.34)

We ﬁrst consider the case where the radii ρ

stay bounded for n →∞in

which case we may assume that they converge towards some radius ρ

and

we can consider everything on the ﬁxed ball B(0,ρ

Thus, in that situation, we have a sequence v

∈ C

(B(0,ρ

)) for which

v



(B(0,ρ

))

is bounded. This implies that the v

are equicontinuous. By

the theorem of Arzela-Ascoli, after passing to a subsequence, we can as-

sume that the v

converge uniformly towards some v

∈ C

(B(0,ρ)) with

v



(B(0,ρ

))

= 1. But (11.1.34) would imply v



(B(0,ρ

))

= 0, hence

v ≡ 0, a contradiction.

It remains to consider the case where the ρ

tend to ∞. In that case, we use

(11.1.34) to choose points x

∈ B(0,ρ

)with

)|≥

v



(B(0,ρ

))

. (11.1.35)

We then consider w

(x):=v

(x + x

)sothatw

(0) ≥

while (11.1.34)

holds for w

on some ﬁxed neighborhood of 0. We then apply the Arzela-

Ascoli argument to the w

to get a contradiction as before.

b) is proved in the same manner. The crucial point now is that for a sequence

for which the norms v



1,α

are uniformly bounded, both the v

and their

ﬁrst derivatives are equicontinuous. 

Lemma 11.1.3:

a) For ε>0, there exists M(ε)(< ∞) such that for all u ∈ C

(B(0, 1))

u

(B(0,1))

≤ ε u

(B(0,1))

+ M(ε) u

(B(0,1))

(11.1.36)

for all u ∈ C

1,α

.Forε → 0,

M(ε) ≤ const. ε

−d

. (11.1.37)

b) For every α ∈ (0, 1) and ε>0, there exists N (ε)(< ∞) such that for all

u ∈ C

1,α

(B(0, 1))

u

(B(0,1))

≤ ε u

1,α

(B(0,1))

+ N(ε) u

(B(0,1))

(11.1.38)

for all u ∈ C

1,α

.Forε → 0,

N(ε) ≤ const. ε

−

d+1

. (11.1.39)

c) For every α ∈ (0, 1) and ε>0, there exists Q(ε)(< ∞) such that for all

u ∈ C

2,α

(B(0, 1))

290 11. Existence Techniques IV

u

1,α

(B(0,1))

≤ ε u

2,α

(B(0,1))

+ Q(ε) u

(B(0,1))

(11.1.40)

for all u ∈ C

1,α

.Forε → 0,

Q(ε) ≤ const. ε

−d−1−α

. (11.1.41)

Proof: We rescale:

(x):=u(

),u

: B(0,ρ) → R. (11.1.42)

(11.1.36) then is equivalent to

u



(B(0,ρ))

≤ ερ u



(B(0,ρ))

+ M(ε)ρ

−d

u

(B(0,ρ))

. (11.1.43)

We choose ρ such that ερ =1,thatis,ρ = ε

−1

and apply a) of Lemma 11.1.2.

This shows (11.1.43), and a) follows.

For b), we shall show

Du

(B(0,1))

≤ ε |Du|

(B(0,1))

+ N(ε) u

(B(0,1))

. (11.1.44)

Combining this with a) then shows the claim. We again rescale by (11.1.42).

This transforms (11.1.44) into

Du



(B(0,ρ))

≤ ερ

|Du|

(B(0,ρ))

+ N(ε)ρ

−d−1

u

(B(0,ρ))

. (11.1.45)

We cho ose ρ such that ερ

=1,thatis,ρ = ε

−

andapplya)ofLemma

11.1.2. This shows (11.1.45) and completes the proof of b).

c) is proved in the same manner. 

We now continue the proof of Theorem 11.1.2:

For homogeneous polynomials p(t),q(t), we deﬁne

:= sup

0≤r≤R

p(R − r) u

1,α

(B(0,r))

:= sup

0≤r≤R

q(R − r) u

2,α

(B(0,r))

For the proof of (a), we choose R

such that

≤ 2p(R − R

) u

1,α

(B(0,R

))

, (11.1.46)

and for (b), such that

≤ 2q(R − R

) u

2,α

(B(0,R

))

. (11.1.47)

(In general, the R

of (11.1.46) will not be the same as that of (11.1.47).)

Then (11.1.30) and (11.1.38) imply

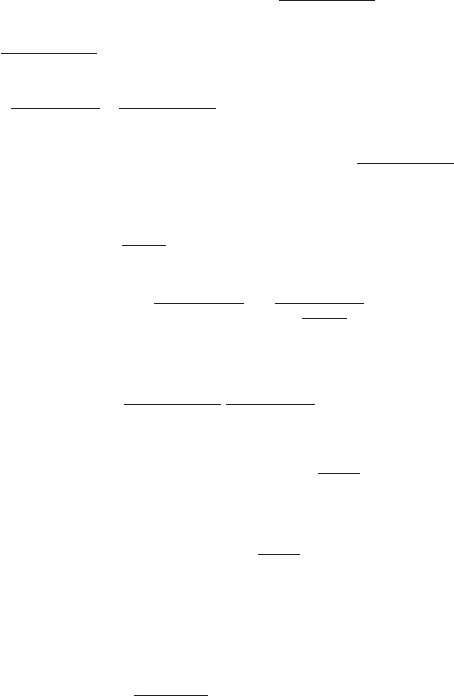
11.1 C

-Regularity Theory for the Poisson Equation 291

≤ c

p(R − R

)



Δu

(B(0,R

))

− R

)

u

1,α

(B(0,R

))

− R

)

N(ε) u

(B(0,R

))



≤ c

p(R − R

)

p(R − R

)

− R

)

· A

+ c

p(R − R

) Δu

(B(0,R

))

+ c

N(ε)

p(R − R

)

− R

)

u

(B(0,R

))

(11.1.48)

We choose R

R+R

∈ (R

,R). Then, because the polynomial p is

homogeneous,

p(R − R

)

p(R − R

)

p(R − R

)

R−R

)

is independent of R and R

. Therefore,

ε =

− R

)

p(R − R

)

p(R − R

)

∼ (R − R

)

and

N(ε) ∼ (R − R

)

−

2(d+1)

by Lemmma 11.1.2 b). Thus, when we choose

p(t)=t

2(d+1)

the coeﬃcient of u

(B(0,R

))

in (11.1.48) is controlled.

Thus, ﬁnally

u

1,α

(B(0,r))

≤

p(R − r)

≤ c



Δu

(B(0,R))

+ u

(B(0,R))



(11.1.49)

with a constant that now also depends on the radii occurring.

In the same manner, from (11.1.31) and (11.1.40), we obtain

u

2,α

(B(0,r))

≤ c



Δu

(B(0,R))

+ u

(B(0,R))



(11.1.50)

for 0 <r<R.SinceΔu = f, we have thus proved (11.1.20) and (11.1.21)

for u ∈ C

2,α

(Ω).

For u ∈ W

1,2

(Ω) we consider the molliﬁcations u

as in Lemma A.2 of

the Appendix. Let 0 <h<dist(Ω

,∂Ω). Then



· Dv = −



v for all v ∈ H

1,2

(Ω),

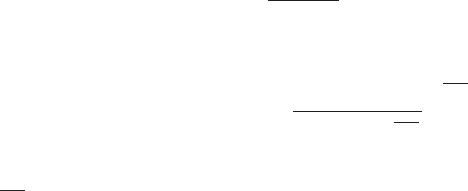
292 11. Existence Techniques IV

and since u

∈ C

∞

,also

Δu

= f

Moreover, by Lemma A.2,

f

− f

→ 0,

and with an analogous proof, if f ∈ C

(Ω),

f

− f

→ 0.

For h → 0, the f

therefore constitute a Cauchy sequence in C

(Ω)orC

(Ω).

Applying (11.1.20) and (11.1.21) to u

− u

, we obtain

u

− u



1,α

(Ω

)

≤ c



f

− f



(Ω)

+ u

− u



(Ω)



(11.1.51)

u

− u



2,α

(Ω

)

≤ c



f

− f



(Ω)

+ u

− u



(Ω)



(11.1.52)

The limit function u thus is contained in C

1,α

(Ω

)orC

2,α

(Ω

), and satisﬁes

(11.1.20) or (11.1.21). 

Part (a) of the preceding theorem can be sharpened as follows:

Theorem 11.1.3: Let u be a weak solution of Δu = f in Ω (Ω abounded

domain in R

), f ∈ L

(Ω) for some p>d, Ω

⊂⊂ Ω. Then u ∈ C

1,α

(Ω) for

some α that depends on p and d,and

u

1,α

(Ω

)

≤ const



f

(Ω)

+ u

(Ω)



Proof: Again, we consider the Newton potential

w(x):=



Γ (x, y)f (y)dy,

and

(x):=



− y

(x − y)

f(y)dy.

Using H¨older’s inequality, we obtain



(x)



≤f

(Ω)





|x − y|

(d−1)

p−1



p−1

and this expression is ﬁnite because of p>d. In this manner, one also veriﬁes

that

∂

∂x

w =constv

and obtains the H¨older estimate as in the proof of

Theorem 11.1.1(a) and Theorem 11.1.2(a). 

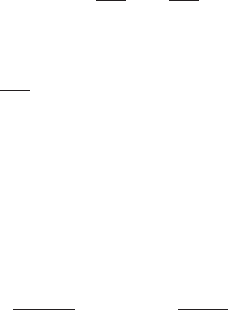
11.2 The Schauder Estimates 293

Corollary 11.1.1: If u ∈ W

1,2

(Ω) is a weak solution of Δu = f with f ∈

k,α

(Ω), k ∈ N, 0 <α<1, then u ∈ C

k+2,α

(Ω),andforΩ

⊂⊂ Ω,

u

k+2,α

(Ω

)

≤ const



f

k,α

(Ω)

+ u

(Ω)



If f ∈ C

∞

(Ω),soisu.

Proof: Since u ∈ C

2,α

(Ω) by Theorem 11.1.2, we know that it weakly solves

∂

∂x

u =

∂

∂x

Theorem 11.1.2 then implies

∂

∂x

u ∈ C

2,α

(Ω)(i ∈{1,...,d}),

and thus u ∈ C

3,α

(Ω). The proof is concluded by induction. 

11.2 The Schauder Estimates

In this section, we study diﬀerential equations of the type

Lu(x):=



i,j=1

(x)

∂

u(x)

∂x



i=1

(x)

∂u(x)

∂x

+ c(x)u(x)=f(x) (11.2.1)

in some domain Ω ⊂ R

. We make the following assumptions:

(A) Ellipticity: There exists λ>0 such that for all x ∈ Ω, ξ ∈ R



i,j=1

(x)ξ

≥ λ |ξ|

Moreover, a

(x)=a

(x) for all i, j, x.

(B) H¨older continuous coeﬃcients: There exists K<∞ such that

)

(Ω)

)

(Ω)

, c

(Ω)

≤ K

for all i, j.

The fundamental estimates of J. Schauder are the following:

Theorem 11.2.1: Let f ∈ C

(Ω), and suppose u ∈ C

2,α

(Ω) satisﬁes

Lu = f (11.2.2)

in Ω (0 <α<1). For any Ω

⊂⊂ Ω, we then have

u

2,α

(Ω

)

≤ c



f

(Ω)

+ u

(Ω)



, (11.2.3)

with a constant c

depending on Ω,Ω

,α,d,λ,K.

294 11. Existence Techniques IV

For the proof, we shall need the following lemma:

Lemma 11.2.1: Let the symmetric matrix (A

)

i,j=1,...,d

satisfy

λ |ξ|

≤



i,j=1

≤ Λ |ξ|

for all ξ ∈ R

(11.2.4)

with

0 <λ<Λ<∞.

Let u satisfy



i,j=1

∂

∂x

= f (11.2.5)

with f ∈ C

(Ω) (0 <α<1). For any Ω

⊂⊂ Ω, we then have

u

2,α

(Ω

)

≤ c



f

(Ω)

+ u

(Ω)



. (11.2.6)

Proof: We shall employ the following notation:

A := (A

)

i,j=1,...,d

u :=



∂

∂x



i,j=1,...,d

If B is a nonsingular d × d–matrix, and if y := Bx,v := u ◦B

−1

, i.e., v(y)=

u(x), we have

u(x)=AB

v(y)B,

and hence

Tr(AD

u(x)) = Tr(BAB

v(y)). (11.2.7)

Since A is symmetric, we may choose B such that B

AB is the unit matrix.

In fact, B can be chosen as the product of the diagonal matrix

D =

⎛

⎜

⎝

−

⎞

⎟

⎠

(λ

,...,λ

being the eigenvalues of A) with some orthogonal matrix R.In

this way we obtain the transformed equation

Δv(y)=f



−1



. (11.2.8)

Theorem 11.1.2 then yields C

2,α

-estimates for v, and these can be trans-

formed back into estimates for u = v ◦ B. The resulting constants will also

depend on the bounds λ, Λ for the eigenvalues of A, since these determine

the eigenvalues of D and hence of B. 

11.2 The Schauder Estimates 295

Proof of Theorem 11.2.1: We shall show that for every x

∈

there exists

some ball B(x

,r) on which the desired estimate holds. The radius r of this

ball will depend only on dist(Ω

,∂Ω) and the H¨older norms of the coeﬃcients

,c.Since

is compact, it can be covered by ﬁnitely many such balls,

and this yields the estimate in Ω

Thus, let x

∈

. We rewrite the diﬀerential equation Lu = f as



i,j

)

∂

u(x)

∂x



i,j



) − a

(x)



∂

u(x)

∂x

−



(x)

∂u(x)

∂x

− c(x)u(x)+f(x)

=: ϕ(x).

(11.2.9)

If we are able to estimate the C

-norm of ϕ, putting A

:= a

)and

applying Lemma 11.2.1 will yield the estimate of the C

2,α

-norm of u.The

crucial term for the estimate of ϕ is



)−a

(x))

∂

∂x

.LetB(x

,R) ⊂

Ω. By Lemma 11.1.1





i,j



) − a

(x)



∂

u(x)

∂x



(B(x

,R))

≤ sup

i,j,x∈B(x

,R)



) − a

(x)



(B(x

,R))



i,j



(B(x

,R))

sup

B(x

,R)



. (11.2.10)

Thus, also

)





) − a

(x)



∂

∂x

)

(B(x

,R))

≤ sup



) − a

(x)



u

2,α

(B(x

,R))

+ c

u

(B(x

,R))

, (11.2.11)

where c

in particular depends on the C

-norm of the a

Analogously,

)



(x)

∂u

∂x

(x)

)

(B(x

,R))

≤ c

u

1,α

(B(x

,R))

, (11.2.12)

c(x)u(x)

(B(x

,R))

≤ c

u

(B(x

,R))

. (11.2.13)

Altogether, we obtain

ϕ

(B(x

,R))

≤ sup

i,j,x∈B(x

,R)



) − a

(x)



u

2,α

(B(x

,R))

+ c

u

(B(x

,R))

+ f

(B(x

,R))

. (11.2.14)

296 11. Existence Techniques IV

By Lemma 11.2.1, from (11.2.9) and (11.2.14) for 0 <r<R, we obtain

u

2,α

(B(x

,r))

≤ c

sup

i,j,x∈B(x

,R)



) − a

(x)



u

2,α

(B(x

,R))

+ c

u

(B(x

,R))

+ c

f

(B(x

,R))

. (11.2.15)

Since the a

are continuous on Ω,wemaychooseR>0sosmallthat

sup

i,j,x∈B(x

,R)



) − a

(x)



≤

. (11.2.16)

With the same method as in the proof of Theorem 11.1.2, the corresponding

term can be absorbed in the left-hand side. We then obtain from (11.2.15)

u

2,α

(B(x

,R))

≤ 2c

u

(B(x

,R))

+2c

f

(B(x

,R))

. (11.2.17)

By (11.1.40), for every ε>0, there exists some Q(ε)with

u

(B(x

,R))

≤ ε u

2,α

(B(x

,R))

+ Q(ε) u

(B(x

,R))

. (11.2.18)

With the same method as in the proof of Theorem 11.1.2, from (11.2.18) and

(11.2.17) we deduce the desired estimate

u

2,α

(B(x

,R))

≤ c



f

(B(x

,R))

+ u

(B(x

,R))



. (11.2.19)



We may now state the global estimate of J. Schauder for the solution of the

Dirichlet problem for L:

Theorem 11.2.2: Let Ω ⊂ R

be a bounded domain of class C

2,α

(anal-

ogously to Deﬁnition 9.3.1, we require the same properties as there, except

that (iii) is replaced by the condition that φ and φ

−1

are of class C

2,α

). Let

f ∈ C

(

Ω), g ∈ C

2,α

(

Ω) (as in Deﬁnition 9.3.2), and let u ∈ C

2,α

(

Ω) satisfy

Lu(x)=f(x) for x ∈ Ω,

u(x)=g(x) for x ∈ ∂Ω.

(11.2.20)

Then

u

2,α

(Ω)

≤ c



f

(Ω)

+ g

2,α

(Ω)

+ u

(Ω)



, (11.2.21)

with a constant c

depending on Ω,α,d, λ,andK.

The Proof essentially is a modiﬁcation of that of Theorem 11.2.1, with

modiﬁcations that are similar to those employed in the proof of Theo-

rem 9.3.3. We shall therefore provide only a sketch of the proof. We start

with a simpliﬁed model situation, namely, the Poisson equation in a half-

ball, from which we shall derive the general case.