Johnson R.S. A Modern Introduction to the Mathematical Theory of Water Waves

Подождите немного. Документ загружается.

294

Weakly nonlinear dispersive waves

-3(H

)

+ H

xxx

= -U

[See Hirota (1973).]

Q3.41 Conserved quantities

and the

N-soliton solution.

Use the

results

obtained

Q3.40,

and

described

Section 3.3.4,

show

principle

how the

amplitudes

of the

solitons

of the

Boussinesq

equation

can be

determined from given initial data;

see

Q3.38.

Give

example

of the

method

for the

2-soliton solution.

(The

solitary-wave solution

the Boussinesq equation

discussed

Q3.8.)

Q3.42 Shallow water equations: conservation laws

Show that

\-u

+hu

+u m\ +

umr

- h

hm\

2 )

-u*

+ hu

+-h

\w\

conservation

law for the

shallow water equations, (3.101).

Hence obtain

the

corresponding conserved quantity.

Q3.43 Shallow water equations: conservation laws

II. See

Q3.42; show

that

(tu)

{t(u

- xu}

{(tu

- x)w}

= 0

and

{t(u

- xu}

{t(u

+2h + m

) - x(u

A)},

{[t(u

+ 2A) -

XM]W}

= 0

are also conservation laws.

Q3.44 Reduction

to the

classical

KdV

equations. Show that

the KdV

equation

for

shear flow, (3.128), together with

the

Burns condi-

tion, (3.117), lead

to the

classical

KdV

equations ((3.28),

Q3.2)

for right-

and

left-going waves, respectively, when

U(z) = 0.

Q3.45

KdV

equation

for

linear shear. Obtain

the

form

of the KdV

equation, (3.128), when

the

shear flow

see (3.113).

Q3.46 Burns condition.

For the two

shear profiles

(a) U(z)

-U

)z;

Exercises 295

(b) U(z)=U

(2z-z

), 0<z<l,

show that no critical level exists.

[Hint: assume that a critical level does exist, use the definition

(3.129) and then show that the only solutions are not critical.]

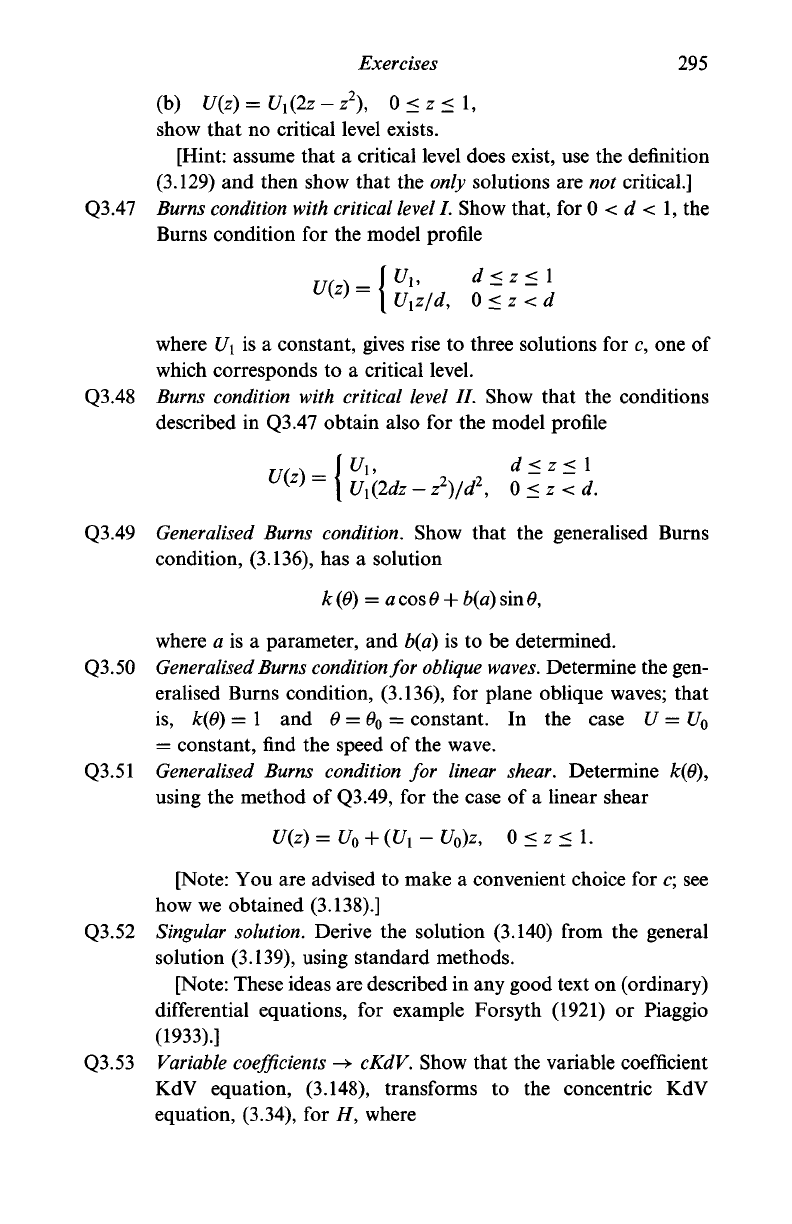
Q3.47

Burns condition with critical level

I. Show that, for 0 < d < 1, the

Burns condition for the model profile

d<z< 1

0 <z < d

where U\ is a constant, gives rise to three solutions for c, one of

which corresponds to a critical level.

Q3.48 Burns

condition

with critical level II. Show that the conditions

described in Q3.47 obtain also for the model profile

d<z<l

{

(2dz-z

)/d\

0<z<d.

Q3.49

Generalised

Burns

condition.

Show that the generalised Burns

condition, (3.136), has a solution

k(0) = a

cos 0

b(a)

sin 0,

where a is a parameter, and b(a) is to be determined.

Q3.50

Generalised Burns

condition for

oblique

waves. Determine the gen-

eralised Burns condition, (3.136), for plane oblique waves; that

is,

k(0) =

and 0 =

= constant. In the case U = U

= constant, find the speed of the wave.

Q3.51

Generalised

Burns

condition

for linear shear. Determine k(0),

using the method of Q3.49, for the case of a linear shear

U(z) =U

+ (U

{

0 < z < 1.

[Note: You are advised to make a convenient choice for

see

how we obtained (3.138).]

Q3.52 Singular

solution.

Derive the solution (3.140) from the general

solution (3.139), using standard methods.

[Note: These ideas are described in any good text on (ordinary)

differential equations, for example Forsyth (1921) or Piaggio

(1933).]

Q3.53

Variable coefficients

cKdV.

Show that the variable coefficient

KdV equation, (3.148), transforms to the concentric KdV

equation, (3.34), for //, where

296 3 Weakly nonlinear dispersive waves

provided that a special choice of D(X) is made. What is this

Q3.54 Conservation laws. Show that the variable coefficient KdV

equation, with D = D(X),

r\ TT I O

7"\—7/4

TT TT I

TAI/2

TT f\

"•"

u n

$$ "5 °^

= u

has a conserved density

HQ.

Also investigate the form of the next

equation in this sequence (which involves

(HQ)

; cf. equation

(3.96)).

Q3.55 Variable depth solitary wave. Obtain the most general solitary-

wave solution of the equation given in Q3.54, where D is treated

as a variable parameter. Now impose the conservation law asso-

ciated with HQ (also in Q3.54) and hence obtain the form of

see equation (3.150).

Q3.56 Oblique plane wave. Obtain, at leading order as e -> 0, the KdV

equation, (3.158), from equations (3.155) and (3.156), by seeking

a solution which is a function of £ = kx + ly

—

t, r = st.

Q3.57 Oblique waves: weak interaction. Obtain the expression for the

surface wave, (3.166), correct at O(s), from the solution for/,

(3.165).

Slow modulation of

dispersive

waves

'But let me tell thee now another tale'

The Coming of Arthur

In ever climbing up the climbing wave

The Lotos-Eaters: Choric song IV

The Korteweg-de Vries equation, members of

its

family and the applica-

tions to more realistic situations, cover only one general area of interest

in the modern theory of nonlinear water waves. In particular, all our

discussions in Chapter 3 have been based on the requirement that the

waves are long; this was accomplished by the condition

-> 0 or, rather,

by the rescaling

8 8

—>

—

—> —

£2 £2

with s -> 0; see equation (3.10). In this discussion we shall now allow the

wave to be of any wavelength, so that the wave number (k) plays the role

of a parameter in our calculations. The amplitude parameter, £, is then

used to describe the slow evolution of an harmonic wave of wave number

k; the wave is thus slowly modulated as described by £ -> 0. The

approach that we adopt is to be found in Section 1.4.2 (equation

(1.103) et seq.) where the appropriate multiple-scale technique is used

there to obtain the asymptotic solution of a partial differential equation.

We shall follow a similar route to that developed in Chapter

namely,

a presentation of the derivation of the basic evolution equation together

with the application of these ideas to more realistic situations. It turns out

that the fundamental equation (the

Nonlinear Schrodinger equation)

- and

some of its relations - are again special equations of the completely

integrable (soliton) type. We shall describe a few properties of these

equations, and how solutions can be readily obtained. Not surprisingly,

the long-wave limit of these various problems that we present here

recovers the essential features of the KdV description; we shall show

how this comes about.

297

298

4 Slow modulation of dispersive waves

4.1 The evolution of wave packets

We shall present two derivations that lead to a description of the evolu-

tion of

wave

packets (for gravity waves) on the surface of water of finite

depth. First we examine the problem of the propagation of a plane wave

and then, just as in Chapter 3, we construct a two-dimensional version of

this problem that incorporates a suitable (weak) dependence on the coor-

dinate that is transverse to the predominant direction of propagation; cf.

the 2D KdV equation. This two-dimensional surface wave is described by

a pair of equations: the Davey-Stewartson (DS) equations.

4.1.1 Nonlinear Schrodinger (NLS) equation

In keeping with much that has gone before, we shall start with an exam-

ination of gravity waves (moving in one direction) on stationary water of

constant depth (b = 0). The most direct approach is to formulate the

problem in terms of the equations for irrotational flow (although we

shall not always be able to follow this route). Thus, from equations

(2.132) with d/dy = 0, we have

(j)

+ 8 (j)

= 0;

on z =

srj

and

(4.1)

= 0 on z = 0.

In these equations we have retained the shallowness parameter,

and we

shall consider e -> 0 for

fixed.

The solution that we seek is a harmonic

wave with wave number k - a solution of the linear equations (e = 0) -

which is allowed to evolve slowly on scales determined by s. We have

already seen (equation (1.103) et

seq.)

that the relevant scales would seem

to be associated with both e and s

. Here, therefore, we introduce

= x

—

t, f = s(x

—

t), x — s

(4.2)

where c

(k) and c

(k) are to be determined (but the notation should be

suggestive!). The justification for this choice is, ultimately, that it pro-

duces a consistent solution of the equations; a simple argument based on

The evolution of wave packets

299

the Fourier integral representation of a general plane wave also confirms

this choice (Q4.1).

The governing equations, (4.1), under the transformation (4.2), become

<t>z

0r " '

- 0;

(j)

on z =

srj

and

= 0 on z = 0.

We seek an asymptotic solution of these equations in the form

oo oo

which is to be periodic in £. The leading-order problem is clearly

with

and

and -

= 0 on z =

= 0 on z = 0.

The solution of interest to us takes the form

= A

E + c.c; 0

(4.3)

(4.4)

(4.5)

(4.6)

(4.7)

where E = exp(ifc£), A

= A

(S, r), F

= F

(z, f, r), /

(f,

and c.c.

denotes the complex conjugate of the terms in E. The real

term/

(f,

r) is

required in order to accommodate the mean drift component; see Section

2.5.

This solution describes a single harmonic wave, of wave number k,

which is propagating at speed c

. We see that Laplace's equation, (4.4),

with (4.7), becomes

Ozz

= 0,

so the solution which satisfies the bottom boundary condition, (4.6), is

300 4 Slow modulation of dispersive waves

where G

(f, r) is yet to be determined. The two surface boundary

conditions, (4.5), yield

8kG

sinh 8k = —i8

and ikc

cosh 8k = A

from which we obtain

tanhSA;

P=-

thus we may write

—— and G

= -T-^sech5A:;

8k kc

cosh

8kz

]

sinh 8k '

(4.8)

(4.9)

all of which is familiar; see equation (2.4) et seq., equation (2.13) and

Q2.5.

At this order, the amplitude function

(t;,

r) is unknown; we now

proceed to the problem given by the O(^) terms.

Equations (4.3), upon collecting the terms of

O(e)

and expanding about

z =

in the surface boundary conditions, yield

= 0;

(4.10)

with

f ^2

(4.11)

on z = 1

= 0

and

and z = 0.

(4.12)

(4.13)

It is clear that these equations will produce terms E

, E~

and Ep (from

) by virtue of the nonlinearity of the surface boundary conditions.

The contributions E

(with E~

, the complex conjugate) are the first of

the higher harmonics that are generated by the nonlinear interaction; the

fundamental is E

(with E~

), introduced in (4.7). Since we are seeking a

solution which is periodic in £, we choose to build in this requirement at

this stage. We do this by imposing a periodic structure on the form of

solution that we seek hereafter. An alternative approach is to solve at

each O(e

) and determine the various functions that are available, in such

a fashion as to remove all those terms that contribute to the non-periodic

The evolution of

wave

packets 301

(or secular) terms; this is how we tackled the problem in Section 1.4.2

(equations (1.103) et

seq.).

The two methods produce, eventually, exactly

the same result, but the former presents us with a more straightforward

calculation, as we shall now demonstrate.

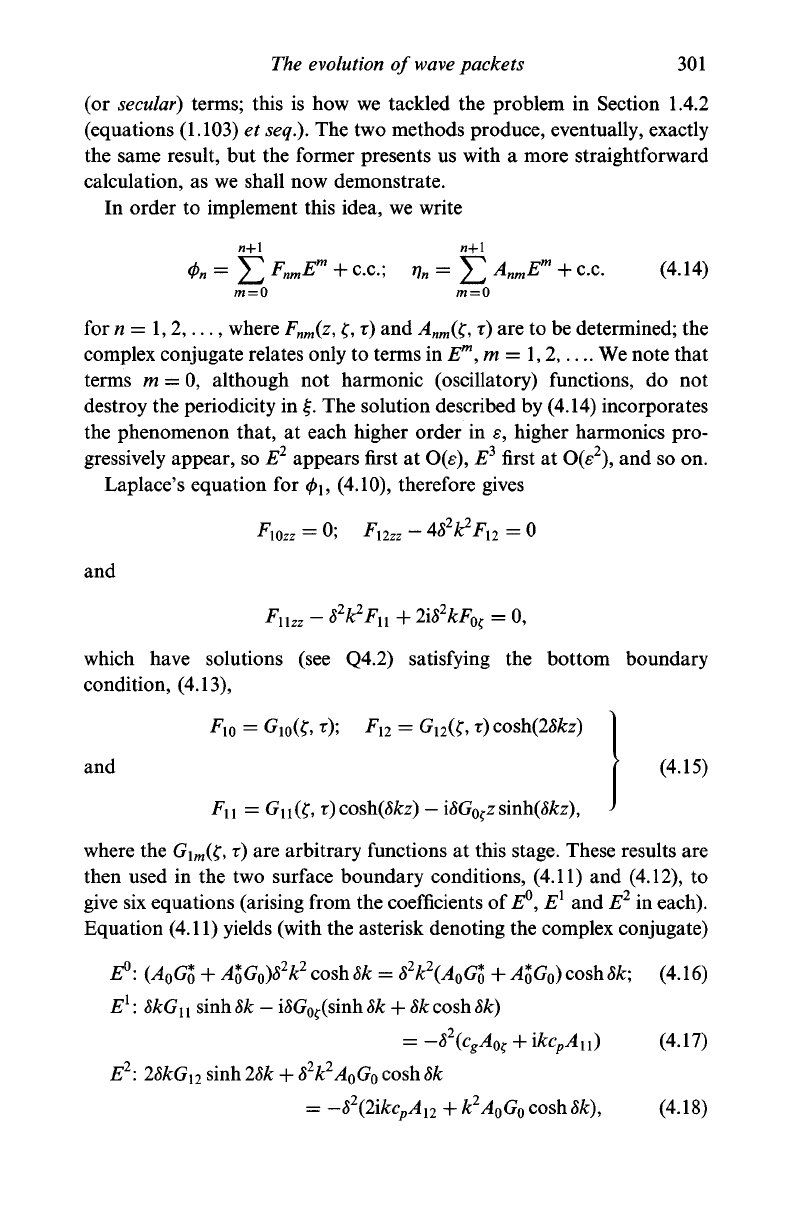
In order to implement this idea, we write

w+l

w+1

4>n

= J^

c.c;

rin

= £

c.c.

(4.14)

= 0

for

1,2,...,

where F

(z, f, r) and A

(t;, r) are to be determined; the

complex conjugate relates only to terms in E

, m = 1, 2, We note that

terms m

—

0, although not harmonic (oscillatory) functions, do not

destroy the periodicity in £. The solution described by (4.14) incorporates

the phenomenon that, at each higher order in e, higher harmonics pro-

gressively appear, so E

appears first at O(e), E

first at O(£

), and so on.

Laplace's equation for 0

(4.10), therefore gives

*io« = O; F

l2zz

-48

= 0

and

which have solutions (see Q4.2) satisfying the bottom boundary

condition, (4.13),

);

n =

((,

r) cosh(28kz)

and I (4.15)

= Gn(f, r)cosh(8kz) -

where the

(£,

r) are arbitrary functions at this stage. These results are

then used in the two surface boundary conditions, (4.11) and (4.12), to

give six equations (arising from the coefficients of E°, E

and E

in each).

Equation (4.11) yields (with the asterisk denoting the complex conjugate)

E°:

A%G

cosh 8k

^o^o)

cosh8k;

(4.16)

8kG

sinh 8k

i^G

^(sinh

8k cosh

8k)

= -8

A^ + ikc

)

(4.17)

28kG

sinh28k

+ 8

cosh 8k

-8

(2ikc

cosh

8k),

(4.18)

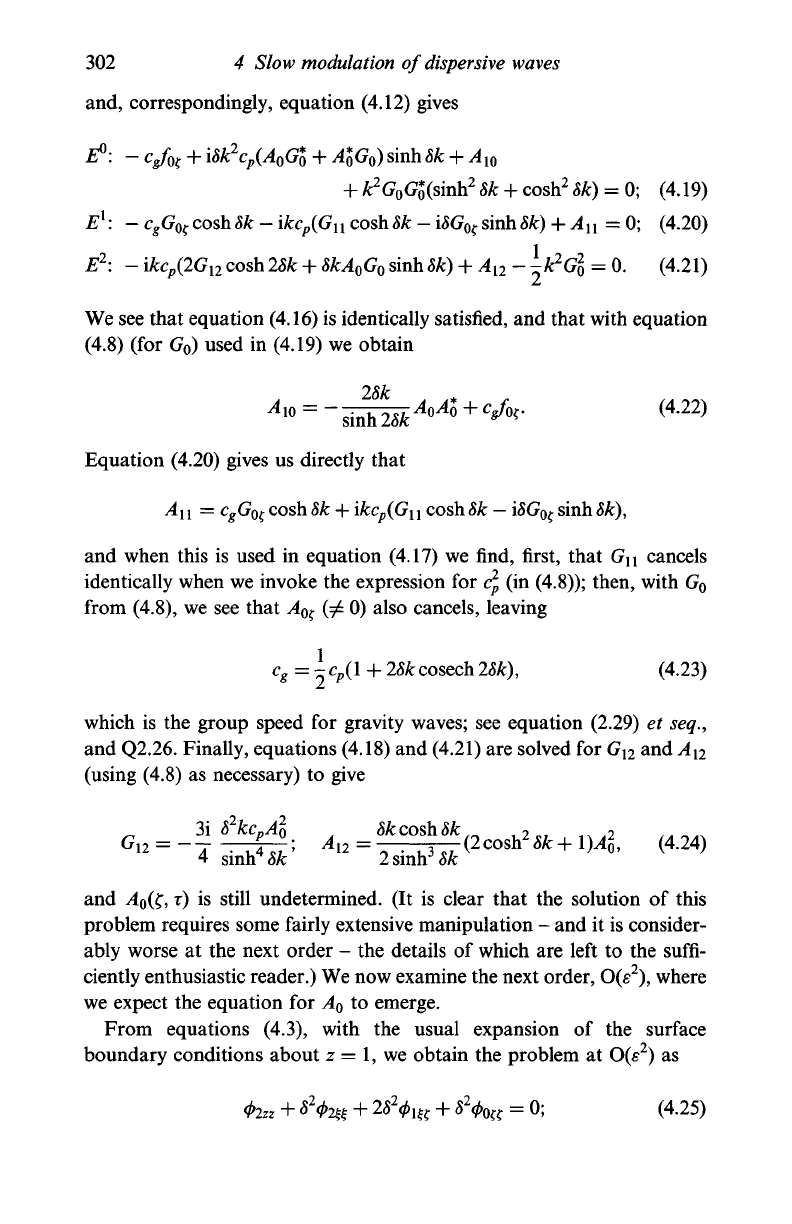
302 4 Slow modulation of dispersive waves

and, correspondingly, equation (4.12) gives

E°'. - c/ot; + i8k

)

sinh 8k + A

+ ^GoG^sinh

8k + cosh

8k) = 0; (4.19)

: - c

G^ cosh 8k - ikc

cosh 8k -

i8G

sinh 8k) + A

=0; (4.20)

: - i^(2G

cosh28k + 5fc^

^o sinh8k) +

- )-&G\ = 0. (4.21)

We see that equation (4.16) is identically satisfied, and that with equation

(4.8) (for G

) used in (4.19) we obtain

28k

A A

Equation (4.20) gives us directly that

cosh8k + ikc

cosh8k

—

iSG^ sinh8k),

and when this is used in equation (4.17) we find, first, that G

cancels

identically when we invoke the expression for

(in (4.8)); then, with G

from (4.8), we see that A^ (^ 0) also cancels, leaving

g = \

28k cosech m

(

)

which is the group speed for gravity waves; see equation (2.29) et seq.,

and Q2.26. Finally, equations (4.18) and (4.21) are solved for G

and A

(using (4.8) as necessary) to give

3i 8

Al 8kcosh8k^ ,

2 of iX j2

,^^

i2 = —7 -VI77; A

=-—j—(2cosh

8k+l)Al (4.24

4 sinh fifc 2smh 5A:

and

(f,

r) is still undetermined. (It is clear that the solution of this

problem requires some fairly extensive manipulation - and it is consider-

ably worse at the next order - the details of which are left to the suffi-

ciently enthusiastic reader.) We now examine the next order,

O(s

where

we expect the equation for A

to emerge.

From equations (4.3), with the usual expansion of the surface

boundary conditions about z = 1, we obtain the problem at O(£

) as

<^ott

= 0; (4.25)

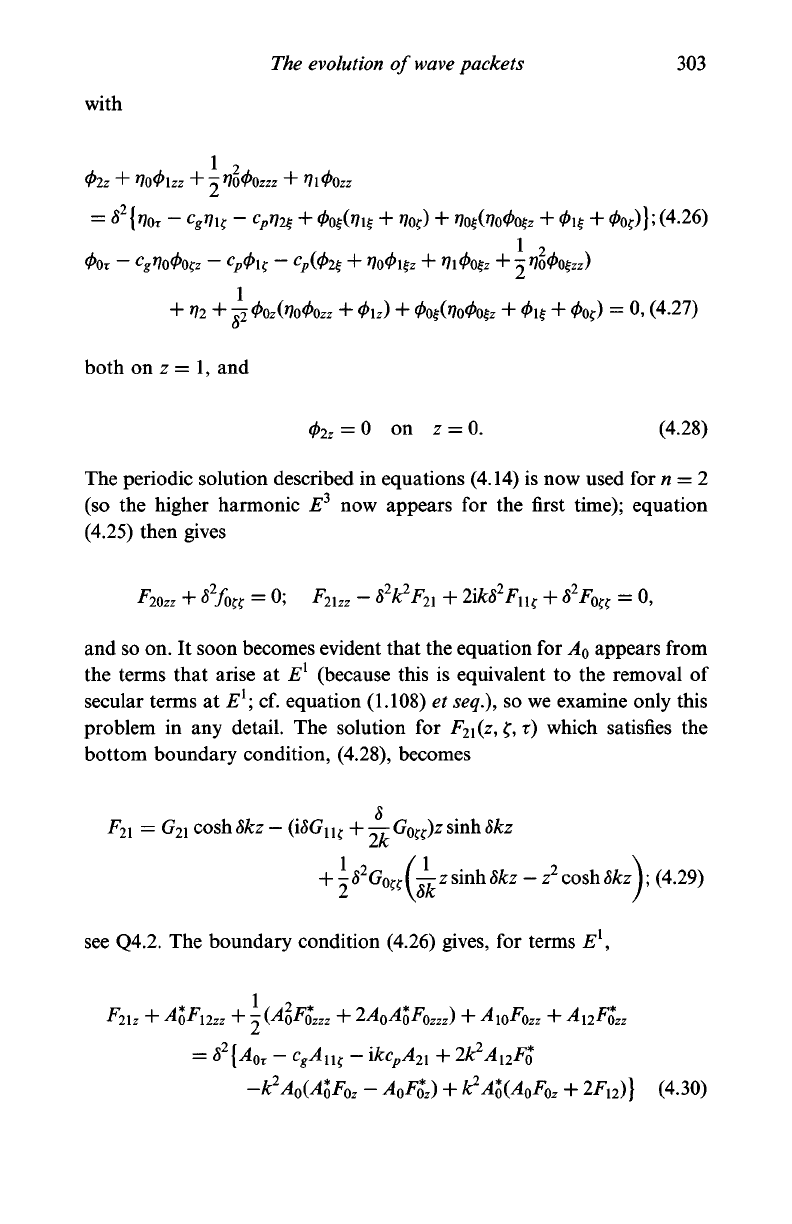
The evolution of wave packets 303

with

02z j

= ^faor ~ c

ri2i:

+ 0o^(^7i^ + W + *to$foo0O*z + 0i| +

00^)};

(4.26)

0Or - <^O0O£z ~

Cp<t

both on z = 1, and

= 0 on z = 0. (4.28)

The periodic solution described in equations (4.14) is now used for n = 2

(so the higher harmonic E

now appears for the first time); equation

(4.25) then gives

FTOZZ

+ S

/^ = 0; F

izz -

+ 2ik8

= 0,

and so on. It soon becomes evident that the equation for A

appears from

the terms that arise at E

(because this is equivalent to the removal of

secular terms at E

; cf. equation (1.108) et

seq.),

so we examine only this

problem in any detail. The solution for F

\(z

f, r) which satisfies the

bottom boundary condition, (4.28), becomes

= G

cosh8kz - (i8G

+ — G

^)zsinh8kz

-8

(-—

z sinh 8kz - z

cosh 8kz\ (4.29)

see Q4.2. The boundary condition (4.26) gives, for terms E

1 2

\-~J(AQFQ

ZZZ

+ 2AQAQF

0ZZZ

) +

QFQ

= 8

\kc

+ 2fc

-k

(A*

)

+ /^(^oz +

)}

(4.30)