Johnson R.S. A Modern Introduction to the Mathematical Theory of Water Waves

Подождите немного. Документ загружается.

284 3 Weakly nonlinear dispersive waves

how the equations can arise in many different situations and, in particu-

lar, how they are relevant in configurations that model physical phenom-

ena more closely (such as variable depth and shear

flows).

There is much

that we have not included, not least the extensive breadth and depth of

ideas that now constitute soliton theory. The exercises to some extent,

and the Further reading more so, enable the interested reader to take

many of these ideas much further.

Further reading

There are many texts now available that describe either general or very

specific aspects of soliton theory; some of

these

texts are listed below. The

derivation and properties of the various members of the KdV family of

equations that arise in water-wave theory are described mainly in

research papers; we provide a small selection for the interested reader.

3.2 Two texts that cover some of the ground that we have described here

are Infeld & Rowlands (1990) and Debnath (1994). Various deriva-

tions and discussions of

these

equations will be found in Korteweg &

de Vries (1895), Kadomtsev & Petviashvili (1970), Miles (1978, 1981)

and Johnson (1980).

3.3 A few of the texts that provide most of the essential features of

soliton theory are Lamb (1980), Ablowitz & Segur (1981), Dodd et

al (1982), Drazin & Johnson (1994) and Ablowitz & Clarkson

(1991).

The texts by Lamb and Drazin & Johnson, in particular,

present an elementary introduction to many of the ideas. More

advanced texts, generally touching on deeper issues, are Calogero

& Degasperis (1982) and Newell (1985). An excellent introduction

to the ideas, coupled with a description of some simple experiments,

is provided by Remoissenet

(1994).

In addition there are publications

that describe specific topics in soliton theory: Rogers & Shadwick

(1982) for Backlund transformations; Matsuno (1984) for the

bilinear transform; and Schuur (1986) for the asymptotic structure

of soliton solutions. Finally, the broader and deeper concepts that

relate soliton theory to Hamiltonian methods are described by

Faddeev & Takhtajan (1987) and Dickey (1991).

3.4 Nonlinear waves propagating over shear flows are described by

Benjamin (1962) and Freeman & Johnson (1970); the Burns condi-

tion is discussed by Thompson (1949), Burns (1953), Velthuizen &

van Wijngaarden (1969), Yih (1972), Brotherton-Ratcliffe & Smith

(1989) and Johnson (1991). The nature of the critical layer, and

Exercises 285

particularly the role of nonlinearity, is described in Benney &

Bergeron (1969), Davis (1969), Haberman (1972) and Varley &

Blythe (1983); and the connection between nonlinear wave propaga-

tion and the critical layer is examined by Redekopp (1977), Maslowe

& Redekopp (1980) and Johnson (1986). A theory for linear and

nonlinear ring waves over a shear flow is presented in Johnson

(1990).

The problems of waves moving over a variable depth have a long

history, starting with Green (1837) and Boussinesq (1871). Some of

the more recent papers, with the emphasis on nonlinear wave propa-

gation, are Peregrine (1967), Grimshaw (1970, 1971), Kakutani

(1971),

Tappert & Zabusky (1971), Johnson

(1973,

1994),

Leibovich & Randall (1973), Miles (1979) and, most importantly,

Knickerbocker & Newell (1980, 1985). The oblique interaction of

nonlinear plane waves is described in Miles (1977a,b); the case of a

large solitary wave interacting obliquely with a sech

wave is

discussed in Johnson (1982); see also Tanaka (1993).

Exercises

Q3.1 Standard KdV equation. Show that a scaling transformation,

u -> aw, x -> fix, t -> yt, for non-zero real constants a, /*, y,

enables the general KdV equation

+ Buu

+ Cu

xxx

= 0

(for real, non-zero, constants A,B,C) to be transformed into

- 6uu

+ u

xxx

= 0.

Q3.2 KdV for left-going waves. Repeat the calculation given in Section

3.2.1,

leading to the KdV equation (3.28), for waves that

propagate to the left (cf. Q1.47 and Q1.48).

Q3.3 KdV with surface tension. Repeat the calculation given in Section

3.2.1,

but retain the surface tension contribution (characterised

by the parameter W (or W

); see equation (1.64)) and derive the

corresponding KdV equation. Show that the inclusion of surface

tension alters only the coefficient of the third derivative term,

that is, the dispersive contribution.

Q3.4 Higher-order correction to the KdV equation. Continue the calcu-

lation described in Section 3.2.1 to find the equation that defines

rji(i-,

r). In the case of the travelling-wave solution, where both

286 3 Weakly nonlinear dispersive waves

and

rji

are functions only of

£ —

cz (cf. Q1.55), obtain an expres-

sion for

rjx

in terms of

by seeking a solution rj

{

TJQF(^

—

ex)

(where the prime denotes the derivative with respect to £

—

ex).

Q3.5 KdV similarity solution. Show that the KdV equation

- 6uu

+ u

xxx

= 0

possesses a similarity solution of the form

u(x, t) = -(3t)

F(rj),

x(3t)

for suitable values of the con-

stants m and n. (The inclusion of the factor 3, and the use of

the negative sign, are merely for convenience.) Hence obtain the

equation for F:

+ (6F -

rj)F

-2F = 0

and, by writing F = W'

—

with V = V(rj) and where

is a

constant to be determined, show that

-rjV-2V

after two integrations, provided that V decays sufficiently rapidly

as either

-> +oo or

-> —

oo.

[The equation for V(rj) is a Painleve equation of the second

kind; see Ince (1927). The use of soliton methods enables the

Painleve equations to be solved; see Ablowitz & Clarkson

(1991),

Drazin & Johnson (1993) and Airault (1979) as an intro-

duction to these ideas.]

Q3.6 KdV rational solution. Show that

w(x, 0 = 6x(x

- 24t)/(x

+ \2tf

is a solution of the KdV equation given in Q3.5.

[This solution is not particularly useful since it is singular on

+ 12/ = 0, although some other soliton equations do have

rational solutions that exist everywhere.]

Q3.7 A cKdV equation. Follow the calculation described in Section

3.2.3 for the concentric KdV equation, but now use a large

time variable x = e

t/8

; cf. equation (3.32). Hence obtain the

appropriate cKdV equation.

Q3.8 Solitary-wave solution of the Boussinesq equation. Obtain the

solitary-wave solution of the Boussinesq equation

- u

xxxx

= 0

Exercises 287

in the form u(x, t) = <zsech

{ft(x

—

ct) +

of}

for suitable relations

between the constants

b, c and a. Show that the wave may

propagate in either direction.

Q3.9

Boussinesq

-> KdV. By means of a suitable choice of far-field

variables, recover the KdV equation for right-going waves from

the Boussinesq equation, (3.41). Repeat this calculation for left-

going waves; cf. equation (3.28) and Q3.2.

Q3.10

Boussinesq

standard

Boussinesq.

Use the transformation

H = rj-

srj

X = x +

f rj(x\

s)dx'

—oo

and thereby obtain equation (3.42) from equation (3.41).

[This transformation is equivalent to writing the equation in a

Lagrangian rather than an Eulerian frame.]

Q3.ll

Solitary-wave solution

the

2D KdV

equation

I. Show that the

2D KdV equation

- 6uu

xxx

)

+ 3u

= 0

has the solitary-wave solution u(x, i) =

a sech

(A:x

+ ly

— cot

+ a)

for suitable relations between the constants

k, /,

and a.

Q3.12

Transformations

between ncKdV and 2D KdV

equations.

Show

that the nearly concentric KdV equation, (3.46), transforms

into the 2D KdV equation if

write

H =

ri(S,R,Y),

S =

%--R®\

Y = R®.

Conversely, show that the choice

= H((, r, 0), f =

+1 F

/r, 0 = F/r

transforms the 2D KdV equation, (3.20), into the ncKdV

equation.

Q3.13

Solution

the

ncKdV equation. Given that v(x,

y) is a solution

of the 2D KdV equation

- 6vv

xxx

)

-f- 3v

= 0,

show that u(x,t,y) = v(x

—

t/l2,t,yt) is a soluton of the

ncKdV equation

—

- 6uu

xrx

)

-rWw

= 0.

288 3 Weakly nonlinear dispersive waves

[This enables solutions for u, which decay sufficiently rapidly

as (x

+ y

-> 0, to be obtained from the solutions for v which

satisfy this same condition. However, solutions for u which have

a different behaviour at infinity cannot be obtained via this

transformation; see Dryuma (1983), Matveev & Salle (1991).]

Q3.14

Phase

shifts for a

2-soliton

KdV

solution.

The phase shifts exhib-

ited by the soliton behaviour in solution (3.59) can be examined

in this fashion: we consider the asymptotic form of the solitary

waves that appear as / -> ±oo. First, for £ = x

—

\6t = O(l) as

±oo, show that

and then for

= x -

= O(l) as / -> ±oo, show that

u ~ —

2sQch

(r]

± -log3);

all signs are vertically ordered. Hence deduce that the taller wave

moves forward by an amount x = ^log

and the shorter back by

x = log

relative to where they would have been if moving

throughout at constant speed.

Q3.15

Phase-shifts:

general.

Recast the calculation of Q3.14 in order to

find the phase shifts for the general 2-soliton solution, (3.58).

Q3.16

Character

the

2-soliton

KdV

solution.

Show that a special case

of the 2-soliton solution, (3.58), takes the form of

sech

pulse at

t = 0. Further, show that the pulse at t = 0 may have either one

or two local maxima.

[In this calculation you should first define x so that a sym-

metric profile occurs at t = 0; for further details see Lax (1968).]

Q3.17

Three-soliton solution

the

KdV

equation

I. Extend the calcula-

tion in Section 3.3.1 (Example 2) to obtain the general 3-soliton

solution of the KdV equation. Show that this can be written in

the form u(x, t) = -2(&/dj?)\ogA

where

3 3 3

A = i

J2Et+ L

i + F1

= 1

<;=i>

<i = i>

with E

= exp{2fc

(x - x

) -

%k]

t],

= {k

- k^f/fa + kj)

and < > denotes that j is to be chosen cyclically with respect

to i.

Exercises 289

Q3.18

Solitary-wave solution

the

2D KdV

equation

II. Use the choice

F = Qxp{-(kx + lz) + (k

- I

)y + 4(k

+ I

)t + a}

in the Marchenko equation, and hence recover the solitary-wave

solution obtained in Q3.ll; see Section 3.3.2. (Direct correspon-

dence with Q3.ll requires here that k + / -• -2k, k

- I

-»

21,

= -co/2.)

Q3.19

Two-soliton solution

the

2D KdV

equation

I. See Q3.18; now

write F as the sum of two appropriate exponentials and hence

derive the two-soliton solution in the form

u(x, i) = -2—}log(l + E

+ E

+ ^Ei£

)

where E

= exp{-(^ +

/,-)*

+ (k

- I

)y +

4(k

(

+ /?)/ + aj and

[This solution describes various configurations of two plane

waves that intersect obliquely and suffer a nonlinear interaction;

an excellent discussion of these solutions is to be found in

Freeman (1980).]

Q3.20 A 2D Boussinesq equation. Follow the derivation of the

Boussinesq equation (Section 3.2.5), but include the weak y-

dependence as required for the two-dimensional KdV equation

(Section 3.2.2). Hence show that, correct at O{e), the surface

wave satisfies the equation

where V

= — rj

. Finally, transform and rescale (exactly as in

Section 3.2.5) to obtain the 2D Boussinesq equation

Htt ~ Hxx + 3(// )xx - Hxxxx

—

HYY = 0.

Q3.21

Solitary-wave solution

the

Boussinesq

equation.

Seek a solu-

tion of the equation for H(X, t, Y) given in Q3.20 in the form

H =

a SQch

{hX

+ IY

— cot

for suitable relations between

the constants

k, /,

and a. Confirm that your solution is an

oblique wave that may propagate in one of two directions.

290 3 Weakly nonlinear dispersive waves

[This 2D Boussinesq equation is not a completely integrable

equation, although it still provides a description of the head-on

collision of oblique waves (cf. Q3.19) and it does possess some

interesting properties; see Johnson (1996).]

Q3.22 Solitary-wave solution of the cKdV equation. Show that a solution

for F of the pair of equations (3.65) is

F(x, z;t)= f f(st

l/3

)Ai(x + s)Ai

where/ is an arbitrary function and Ai is the Airy function. The

solitary-wave solution is usually regarded as that solution

obtained from the choice /(•) = £<$(•), where 8 is the Dirac delta

function and £ is a positive constant; construct the solitary-wave

solution of the cKdV equation, (3.64).

Q3.23 A similarity solution of the cKdV equation. Show that

u(x, t) = - — sech

{A(x +

)//

1/2

t > 0,

is a solution of the concentric KdV equation, (3.64), for any real

constant k.

[This solution is undefined on t = 0, is not real for t < 0 and

grows without bound as |x| -• oo at any fixed t.]

Q3.24 Bilinear operator. Prove these identities, where D™D"(a

•

b) is the

bilinear operator defined in equation (3.71):

(a) D?D

(a.b) = D

D?(a-b);

(b) D

•

b) =

(-l)

•

a) and hence that D

(a a) = 0forn

odd;

•

1) = D^D^l

•

a) = r

a/dx

for m + n even;

(d) ETOtexppO

•

exp(0

)} =

(co

- co.Tik, - k

f exp^ + 0

)

where 0

= k

— co

{

+ a

i = 1,2.

Q3.25 Three-soliton solution of the KdV equation II. Use Hirota's

bilinear method to find the expression for/(x, t) which generates

the 3-soliton solution of the KdV equation.

Q3.26 Two-dimensional KdV equation. Show that the bilinear form of

the equation

- 6uu

xxx

)

+ 3u

= 0

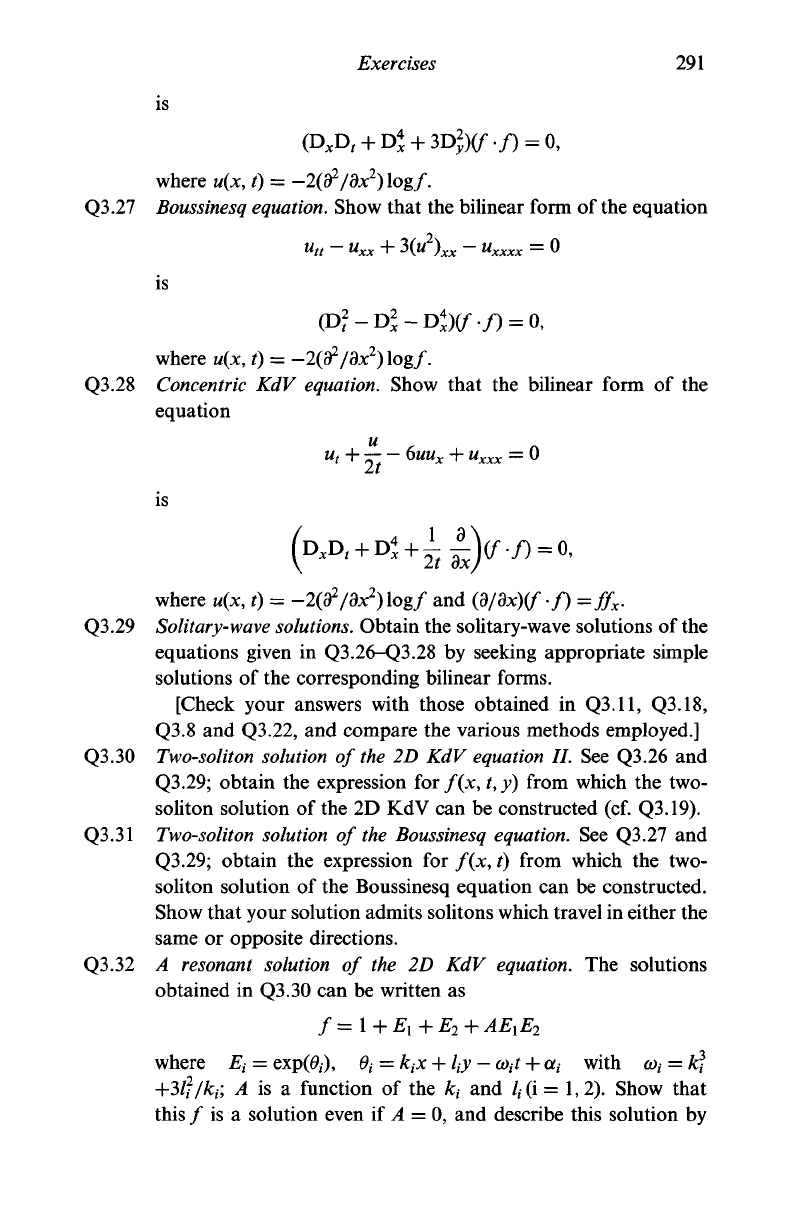
Exercises 291

where u(x, i) = -2(a

/3jc

) log/.

Q3.27

Boussinesq

equation.

Show that the bilinear form of the equation

~ u

+ 3(u\

xxxx

= 0

where w(x, i) = -l^/dx

) log/.

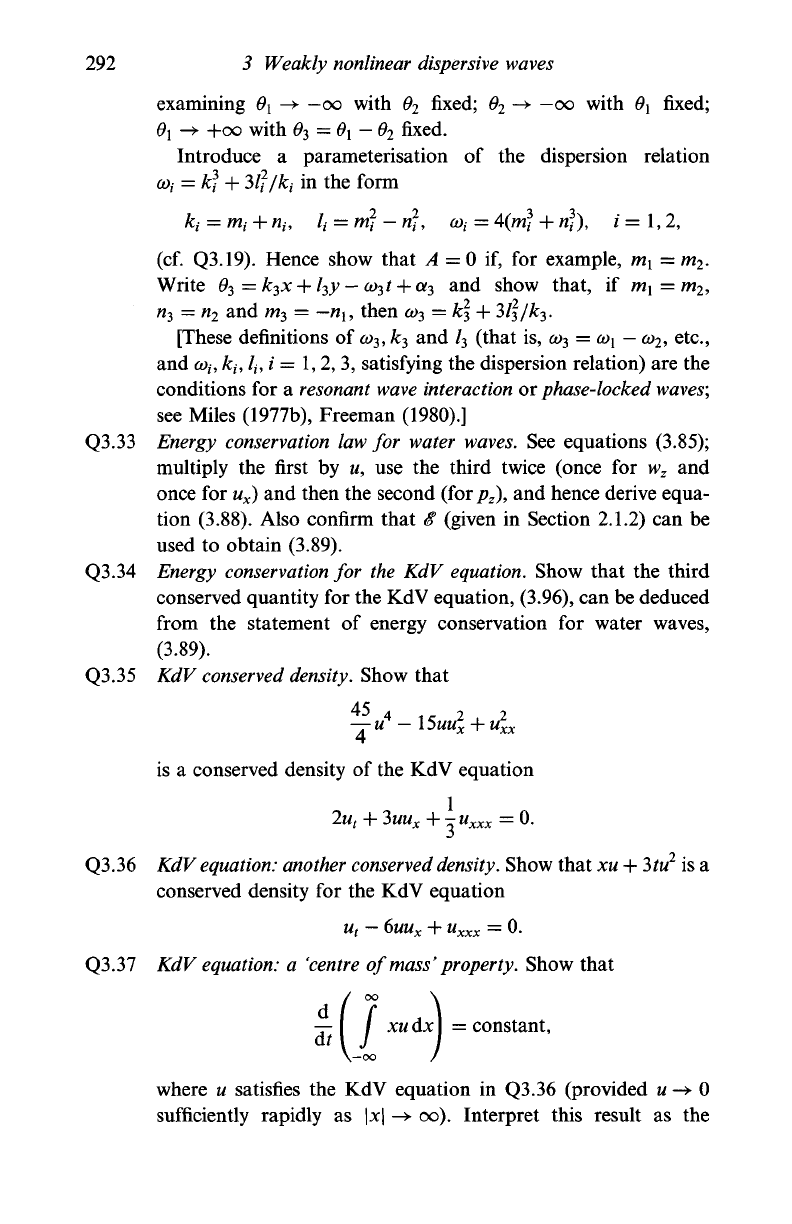
Q3.28 Concentric KdV equation. Show that the bilinear form of the

equation

xxx

= 0

where

K(JC,

0 =

-2(&/dJ)\ogf

and

(a/foXf

•/)

=ff

Q3.29 Solitary-wave

solutions.

Obtain the solitary-wave solutions of the

equations given in Q3.26-Q3.28 by seeking appropriate simple

solutions of the corresponding bilinear forms.

[Check your answers with those obtained in Q3.ll, Q3.18,

Q3.8 and Q3.22, and compare the various methods employed.]

Q3.30 Two-soliton solution of the 2D KdV equation II. See Q3.26 and

Q3.29;

obtain the expression for/(x, t,y) from which the two-

soliton solution of the 2D KdV can be constructed (cf. Q3.19).

Q3.31 Two-soliton solution of the Boussinesq equation. See Q3.27 and

Q3.29;

obtain the expression for /(x, i) from which the two-

soliton solution of the Boussinesq equation can be constructed.

Show that your solution admits solitons which travel in either the

same or opposite directions.

Q3.32 A resonant solution of the 2D KdV equation. The solutions

obtained in Q3.30 can be written as

f=l+E

+ AE

where E

= exp(fy), 6

= k

x + l

—

(Oft

+ a

{

with

COJ

= k]

+3J}/ki'

A is a function of the k

and /,(i= 1,2). Show that

this/

is a solution even if A = 0, and describe this solution by

292 3 Weakly nonlinear dispersive waves

examining 9\ -> — oo with 6

fixed; 6

-> — oo with 0! fixed;

0! -> +oo with

fixed.

Introduce a parameterisation of the dispersion relation

(Of —

h] + 3/f/A:/ in the form

+ n

-n

, co

A(rn] + »?), i = 1, 2,

(cf. Q3.19). Hence show that A = 0 if, for example, ra! = ra

Write 0

= k

x + l

—

t-\-a

and show that, if m

= n

and m

= —n

, then

= k\ +

[These definitions of

and l

(that is,

—

etc.,

and o)

kf, l

, i = 1, 2, 3, satisfying the dispersion relation) are the

conditions for a resonant wave interaction or phase-locked waves;

see Miles (1977b), Freeman (1980).]

Q3.33 Energy conservation law for water waves. See equations (3.85);

multiply the first by w, use the third twice (once for w

and

once for u

) and then the second (for p

), and hence derive equa-

tion (3.88). Also confirm that $ (given in Section 2.1.2) can be

used to obtain (3.89).

Q3.34 Energy conservation for the KdV equation. Show that the third

conserved quantity for the KdV equation, (3.96), can be deduced

from the statement of energy conservation for water waves,

(3.89).

Q3.35 KdV conserved density. Show that

is a conserved density of the KdV equation

+ 3uu

+-u

xxx

= 0.

Q3.36 KdV equation: another conserved density. Show that xu + 3tu

is a

conserved density for the KdV equation

—

6uu

+ u

xxx

= 0.

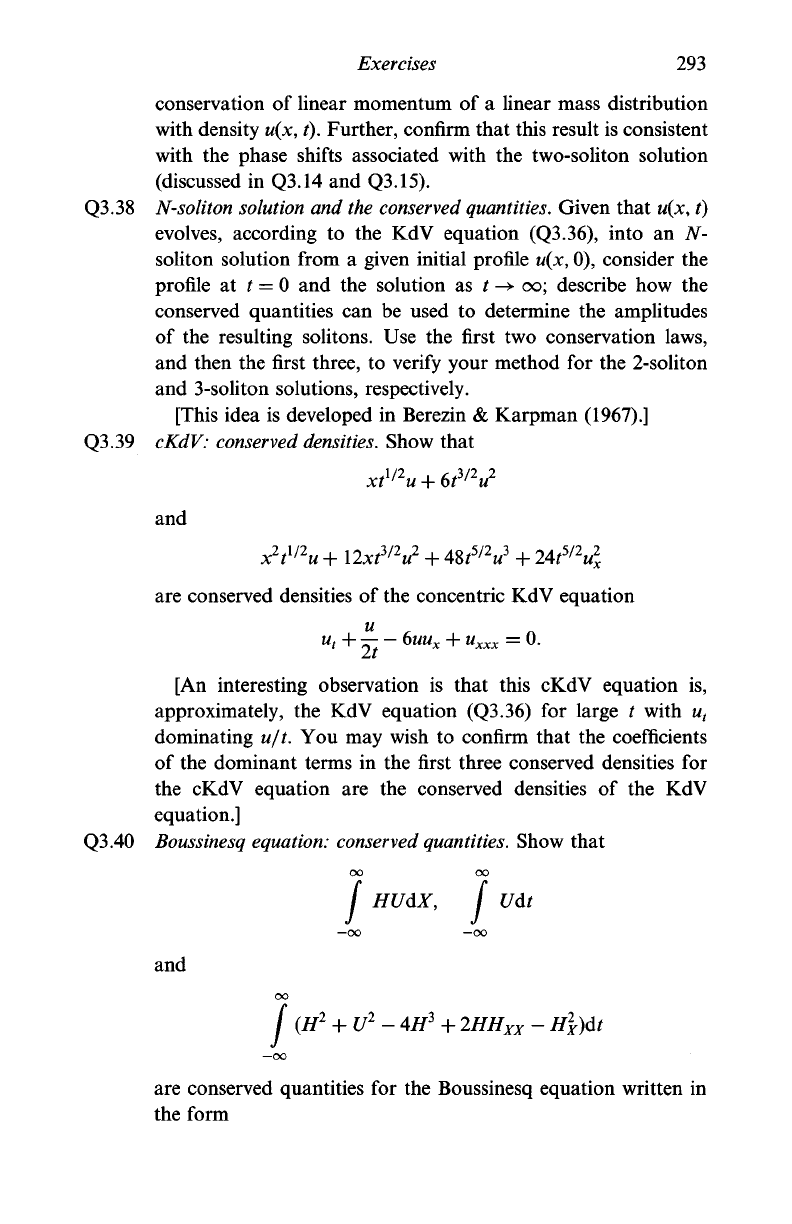
Q3.37 KdV equation: a 'centre of

mass*

property. Show that

oo \

/ xwdjcj = constant,

X) /

where u satisfies the KdV equation in Q3.36 (provided u -> 0

sufficiently rapidly as

|JC|

-> oo). Interpret this result as the

Exercises 293

conservation of linear momentum of a linear mass distribution

with density u(x, i). Further, confirm that this result is consistent

with the phase shifts associated with the two-soliton solution

(discussed in Q3.14 and Q3.15).

Q3.38

N-soliton

solution

and the

conserved

quantities.

Given that w(x, t)

evolves, according to the KdV equation (Q3.36), into an N-

soliton solution from a given initial profile u(x, 0), consider the

profile at t = 0 and the solution as t -* oo; describe how the

conserved quantities can be used to determine the amplitudes

of the resulting solitons. Use the first two conservation laws,

and then the first three, to verify your method for the 2-soliton

and 3-soliton solutions, respectively.

[This idea is developed in Berezin & Karpman (1967).]

Q3.39

cKdV:

conserved

densities.

Show that

and

xh^u + 12xW + 48 W +

24t

are conserved densities of the concentric KdV equation

[An interesting observation is that this cKdV equation is,

approximately, the KdV equation (Q3.36) for large t with u

dominating u/t. You may wish to confirm that the coefficients

of the dominant terms in the first three conserved densities for

the cKdV equation are the conserved densities of the KdV

equation.]

Q3.40

Boussinesq

equation:

conserved

quantities.

Show that

HUdX,

f Udt

and

+ U

- 4i/

2HH

)dt

—

are conserved quantities for the Boussinesq equation written in

the form