Hooke R.L. Principles of glacier mechanics
Подождите немного. Документ загружается.

Analysis of the effect of a small change 371
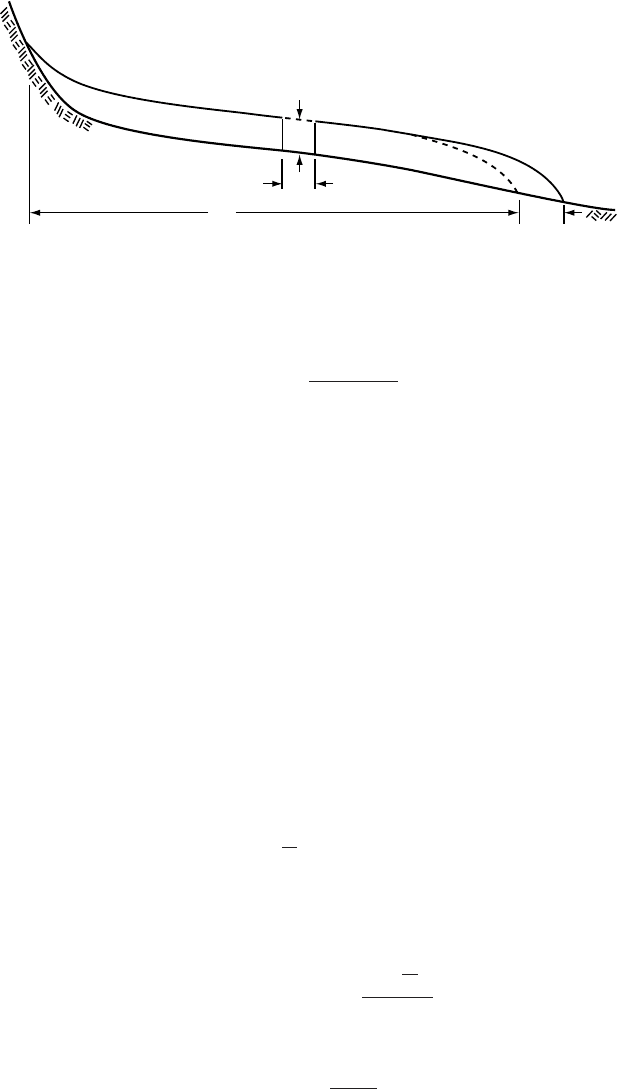
q
h
P
Based on
flow law
(
∂q
)
P
∂h
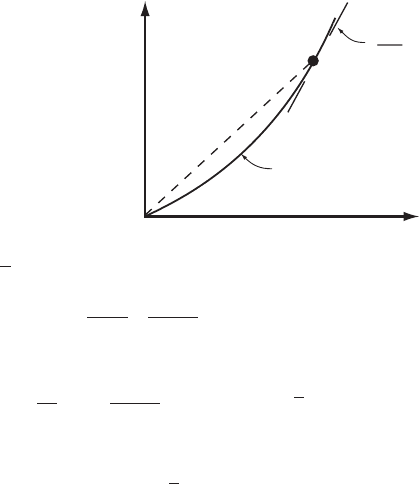
Figure 14.5. Relation
between mean speed of a
glacier and speed of a
kinematic wave.
Noting that q = uh,wehave:
q =
2
n + 2
S
f
ρgα
B
n
h
n+2
so:
c =
∂q
∂h
= 2
S
f
ρgα
B
n
h
n+1
= (n + 2)u (14.5)
or with n
∼
=
3:
c
∼
=
5
u (14.6)
In other words, the kinematic wave moves with a speed that is roughly
five times the depth-averaged velocity of the glacier. If there is basal
sliding and the sliding speed varies as τ
2
(Equation (7.10)), the ratio is
likely to be slightly less than 5. This relation applies, rigorously, only to
infinitesimal waves. Waves of finite amplitude may have higher speeds.
Analysis of the effect of a small change in mass
balance using a perturbation approach
Let us now, following Nye (1960, pp. 561–562), use perturbation tech-
niques to study the change in thickness with time after a small change in
mass balance. Consider the situation in which the specific mass balance
is shown by the solid line in Figure 14.6.Wewill refer to the situation
represented by this solid line as the “0” or datum or equilibrium state,
and analyze the effect of small perturbations from this state such as those
represented by the dashed lines in the figure. For example, during a cold
or unusually snowy year the mass balance may be increased everywhere
by an amount b
1
(x, t)sowehave:
b(x, t) = b
0
(x ) + b
1
(x , t)
Note that b
0
is a function only of x;itdoes not vary with time because
the datum state is a steady state. Other properties of the datum state are

372 Response of glaciers to changes in mass balance
Equilibrium
line
+b
−b
b
= 0
Distance from head of glacier
Cold year
Warm year
b
0
Figure 14.6. Perturbations in
mass balance from an
equilibrium state, b
0
.
q
0
(x, h, α), h
0
(x), and α
0
(x). In the perturbed state these become
q = q
0
+ q
1
, h = h
0
+ h
1
, and α = α
0
+ α
1
. Substituting these into
the continuity equation (Equation (14.2)) yields:
∂
∂x
(q
0
+ q
1
) +
∂
∂t
(h
0
+ h
1
) = b
0
+ b
1
(14.7)
We now write Equation (14.2)interms of the datum state, thus:
∂q
0
∂x
+
∂h
0
∂t
= b
0
and subtract this from Equation (14.7)toobtain:
∂q
1
∂x
+
∂h
1
∂t
= b
1
(14.8)
In passing, it is again worth noting that ∂h
0
/∂t = 0 because h
0
is a
property of the steady state.
At any position, x, q varies with h and α so we can write:
dq =
∂q
∂h
dh +
∂q
∂α
dα
or for small perturbations, dq = q
1
, dh = h
1
, and dα = α
1
so:
q
1
=
∂q
∂h
h
1
+
∂q
∂α
α
1
(14.9)
Previously we identified ∂q/∂h with the celerity of a kinematic wave,
c,orinthe datum state: (∂q/∂h)
0
= c
0
.Now we similarly define
D
0
= (∂q/∂α)
0
,sointhe datum state, Equation (14.9) becomes:
q
1
= c
0
h
1
+ D
0
α
1
(14.10)
This relation is valid only for small perturbations. Were we interested in
larger perturbations, terms involving h
1
2
, h
1
3
,...α
1
2
, α
1
3
,...would
have to be included. Thus, our approach is referred to as a linearized
theory.
Equations (14.8) and (14.10) are a pair of simultaneous differential
equations that can be solved for the change in ice flux and thickness

Analysis of the effect of a small change 373
resulting from a perturbation in net balance. Let us first eliminate q
1
from the two equations, thus:
∂c
0
∂x
h
1
+ c
0
∂h
1
∂x
+
∂ D
0
∂x
α
1
+ D
0
∂α
1
∂x
+
∂h
1
∂t
= b
1
(14.11)
Returning to Equation (14.1)wesee that in the datum and perturbed
states, respectively:
α
0
= β −
∂h
0
∂x
and α
0
+ α
1
= β −
∂h
0
∂x
−
∂h
1
∂x
(14.12)
Thus, subtracting the first of these expressions from the second:
α
1
=−∂h
1
/∂x. This result can be substituted into Equation (14.11)
to yield, after some rearranging:
∂h
1
∂t
= b
1
−
∂c
0
∂x
h
1
−
c
0
−
∂ D
0
∂x
∂h
1
∂x
+ D
0
∂
2
h
1
∂x
2
(14.13)
(i) (ii) (iii) (iv)
Equation (14.13)was first derived by Nye (1960,p.562). As he noted,
the terms in it have the following meanings.
(i) h
1
increases at a rate given by the perturbation in accumulation.
(ii) This term results in an exponential decrease or increase in the rate
of change of h
1
as we shall show below.
(iii) This represents a kinematic wave of constant h
1
. The speed of
propagation of the wave is c
0
− ∂D
0
/∂x in the +x direction. Note
that both c
0
and ∂D
0
/∂x have dimensions t
−1
.
(iv) This represents diffusive damping of the perturbation h
1
,inaccord
with the diffusion equation, with diffusivity D
0
.
Our objective now is to solve Equation (14.13) for a simple case, neglect-
ing diffusion. Then we will investigate the role of diffusion.
Solution for a small part of a glacier with uniform
longitudinal strain rate and without diffusion
Consider a situation in which a glacier is initially in a steady state with
an accumulation rate b
0
(Nye, 1960,p.563). Then the accumulation rate
increases abruptly by an amount b
1
to b = b
0
+ b
1
and remains at this
increased level indefinitely. Suppose ∂c
0
/∂x is independent of x on this
glacier. From Equations (14.5)wesee that:
∂c
0
∂x
∼
=
(n + 2)
∂
u
0
∂x
(14.14)
where ∂u
0
/∂x is the longitudinal strain rate, r
0
. This, thus, corresponds
to a situation in which the longitudinal strain rate is uniform in the
x-direction. We seek a solution to Equation (14.13) such that h
1
is
374 Response of glaciers to changes in mass balance
h
1
b
1
t
g
0
g
0
1
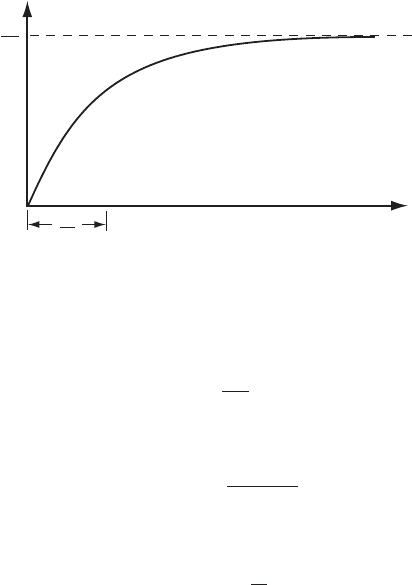
Figure 14.7. Asymptotic
adjustment of a glacier toward
a new steady state, b
1
/γ
0
,
following a perturbation in
accumulation rate, b
1
,inan
area of extending flow. 1/γ
0
is the response time.
independent of x so ∂h
1
/∂x = 0. Thus, the increase in thickness is uni-
form over the glacier. We will let γ
0
= ∂c
0
/∂x.
With these simplifications, Equation (14.13) becomes:
dh
1
dt
= b
1
− γ
0
h
1
(14.15)
Separating variables we obtain:
h
1
0
dh
1
b
1
− γ
0
h
1
=
t
0
dt
Integration yields:
h
1
=
b
1
γ
0
1 −e
−γ
0
t
(14.16)
If γ
0
is positive, corresponding to a positive longitudinal strain rate
(Equation (14.14)) such as we expect in an accumulation area, h
1
asymp-
totically approaches the value b
1
/γ
0
(Figure 14.7). In other words,
after a very long time, the glacier will have increased in thickness by
this amount. This is the situation described earlier and illustrated in
Figure 14.1a.
The quantity 1/γ
0
,which has the dimensions of time, is known as
the time constant for this change. This is sometimes associated with the
“response time” of a glacier, or the length of time required for a glacier
to respond to a change in climate. When t = 1/γ
0
,(1− e
−γ
0
t
)
∼
=
2/3
so h
1
is ∼2/3ofthe way to the new equilibrium state. Mathematically
(Equation (14.16)), it is clear that the new equilibrium state is never
reached. Thus, it would be meaningless to try, instead, to define the
response time as the total time required to attain a new steady state.
From Equation (14.14)wesee that 1/γ
0
∼
=
1/5r
0
.Inother words,
in this simple model the response time is inversely proportional to the
longitudinal strain rate. For example, typical longitudinal strain rates for
Storglaci¨aren, Barnes Ice Cap, and the Antarctic Ice Sheet are 0.015 a
−1
,
0.005 a
−1
, and 0.000 05 a
−1
respectively. Thus, the response time of
Effect of diffusion 375
Barnes Ice Cap might be expected to be three times as long as that of
Storglaci¨aren, and that of the Antarctic Ice Sheet, 100 times as long as
Barnes Ice Cap. While these multiples are not unrealistic, it turns out
that 1/5r
0
seriously underestimates the actual response time. As we will
see below, this is because diffusion has been neglected.
If γ
0
is negative, corresponding to longitudinal compression as
would be typical in an ablation zone, there is an obvious problem. Equa-
tion (14.16) then predicts that h
1
will increase exponentially with time.
Thus, a new steady state is never even approached. This is the situation
which we discussed in connection with Figure 14.1b.
Clearly, it is not possible to have the upper part of a glacier increasing
in thickness slowly and stably while the lower part is increasing rapidly
and unstably. In the absence of diffusion, Nye (1960) suggests that the
initial response in the ablation area would, indeed, be unstable. At any
location, however, stability would be restored when a kinematic wave,
initiated in the vicinity of the equilibrium line and propagating down
glacier, reached that location. With diffusion, however, such an unstable
response may never develop.
Effect of diffusion
Diffusion occurs whenever fluxes are proportional to gradients. In the
present case, the flux, q,isproportional to the slope (or gradient), α.
Where α is largest, on the downslope side of a wave, q is highest. Con-
versely, q is lowest on the upslope side of the wave. Thus, the flux into the
wave is diminished and that out of it is enhanced. This tends to decrease
the amplitude and increase the wavelength of a wave.
As in the case of c (or c
0
) (Equation (14.5)), an analytical expression
for D
0
can be obtained by differentiating q with respect to α, thus:
D
0
=
∂q
∂α
0
= n
2
n + 2
S
f
ρg
B
n
h
n+2
α
n−1
=
nq
α
or with n
∼
=
3:
D
0
∼
=
3
uh
α
(14.17)
In other words, diffusion will be most significant where the glacier is
thick, the speed high, and the slope low.
Unfortunately, it is difficult to probe this dependence more thor-
oughly at the level of the treatment herein. However, Nye (1963a,
pp. 442–445) has shown that diffusion decreases the rate of thickening,
a result that is intuitively logical. As a result, the response time increases
quite markedly. In one example, the response time increases by more
than an order of magnitude (Nye, 1963a,Figure 4a). In addition, the

376 Response of glaciers to changes in mass balance
∆
h
1
(
0
)
0
q
0
Figure 14.8. Geometry of
the terminus region.
increase in thickness of the glacier near the equilibrium line is substan-
tially greater when diffusion is taken into consideration.
The problem at the terminus
It is difficult to use Nye’s kinematic wave theory to study the details
of the advance and retreat of real glaciers. This is, in part, because the
mass flux, q, cannot go to zero at the terminus if the glacier is to respond
to an increase in accumulation by advancing. However, Equation (14.4)
suggests that u →0ash → 0. To avoid this, Nye (1963b,p.92) assumes
that the glacier is sliding at the terminus so q = u
b0
(
0
)h where u
b0
(
0
)
is the sliding speed, u
b
,atthe terminus,
0
,inthe datum state. Here
0
is the length of the glacier, measured from the bergschrund. Then
c
0
= ∂q/∂h = u
b0
(
0
).
In addition, the amount of advance, ,issensitive to the assumed
geometry of the terminus. As shown in Figure 14.8:
=
h
1
(
0
)
tan θ
0
(14.18)
where h
1
(
0
)isthe perturbation in ice thickness at the terminus. Thus
depends on θ
0
.
Further study of the response time
J´ohannesson et al. (1989)have studied the question of response times
and of conditions at the terminus in greater detail. They identify three
possible natural time scales that might be used in the analysis of glacier
responses:
t
C
=
0
c
0
m
ma
−1
(14.19a)
t
D
=
2
0
π
2
D
0
m
2
m
2
a
−1
(14.19b)
t
V
=
V
1
b
1
0
m
3
m
−1
ma
−1
m
(14.19c)
Further study of the response time 377
Here, t
C
and t
D
are time constants for propagation or diffusion
*
of a
disturbance over the length of a glacier. In effect, they are measures of
the time required to establish the general shape of the new thickness
profile, h
1
(x, t). As the size of such a disturbance decreases with time,
the rate of propagation or diffusion also decreases. Therefore, as with
1/γ
0
, t
C
and t
D
are measures of the time required for the processes to
proceed about 2/3ofthe way to completion. Similarly, as we shall show
below, t
V
is the time required for accumulation (or loss) of about 2/3of
the volume (per unit width), V
1
, required to re-establish an equilibrium
geometry after a perturbation in mass balance that adds a volume of ice
b
1
0
to the glacier every year.
J´ohannesson et al. find that t
V
is usually appreciably longer than t
C
or t
D
. This means that perturbations in ice thickness are spread out over
the glacier by propagation and diffusion rather quickly in comparison
with the time needed for accumulation of the additional mass. Clearly, a
glacier cannot be considered to have returned some specified fraction of
the way to a new equilibrium state until the necessary additional mass
has accumulated (or been lost).
In an extension of the Nye theory, t
V
would be viewed approximately
as follows: at any given time after a perturbation in mass balance, the
mean perturbation in thickness, averaged over the length of the glacier,
would equal the perturbation at the terminus, h
1
(
0
,t), multiplied by some
function of the conditions in the datum state, of the magnitude of the
perturbation, b
1
, and of time, thus:
h
1
(x , t) = f (c
0
, D
0
, b
1
, t) · h
1
(
0
, t) (14.20)
Remember that
0
is the position of the terminus in the datum state. Once
anew equilibrium geometry has been attained, at t =∞, the increase in
volume of the glacier would be obtained by multiplying Equation (14.20)
by the length of the glacier, thus:
V
1
= h
1
0
= f (t =∞) ·h
1
(
0
, ∞)
0
(14.21)
However, once a new steady state has been attained, the annual mass
gain resulting from the perturbation,
b
1
0
, must equal the flux past the
old terminus position, u
b0
(
0
)·h
1
, thus:
b
1
0
= u
b0
(
0
)h
1
(
0
, ∞) (14.22)
Eliminating h
1
from these two equations yields:
V
1
=
f (t =∞)
b
1
2
0
u
b0
(
0
)
(14.23)
*
The π
2
term in Equation (14.19b) comes from the Fourier solution of the diffusion
equation (T. J´ohannesson, written communications dated November 7 and 14, 1996).
378 Response of glaciers to changes in mass balance
∆
0
∆
h
0 max
Figure 14.9. Geometrical argument for evaluating t
V
. During an advance, ,
the mass that must be added to a glacier is approximately ·h
0max
.
Whence, from Equation (14.19c):
t
V
=
f (t =∞)
0
u
b0
(
0
)
(14.24)
Thus, by this approach, t
V
turns out to be sensitive to the unknown sliding
speed at the terminus. In addition, the function, f,ishighly sensitive to
the details of the variations in c
0
and D
0
, especially near the terminus
(J´ohannesson et al., 1989,p.364 and appendices).
J´ohannesson et al.havedeveloped a much simpler geometrical argu-
ment to estimate t
V
. Consider the situation in Figure 14.9 in which an
advance of a glacier by an amount is illustrated graphically by cutting
the glacier at its point of maximum thickness and sliding the lower part
forward by . Then, the increase in volume of the glacier is approx-
imately ·h
0max
. Detailed numerical modeling suggests that this is a
good approximation to the response of a real glacier when the dynamical
properties of the glacier are the same in the initial and final state, and
thus influence the initial and final profiles in the same way. Now, rather
than equate the annual mass gain resulting from the perturbation with
the flux past the old terminus position, as in Equation (14.22), we equate
it with the mass loss over the new part of the glacier, , thus:
b
1
0
=
|
b
t
|
(14.25)
where b
t
is the mean net balance rate over which may be approximated
by b(
0
), the net balance rate at the terminus (a negative quantity) if
is small. Therefore:
V
1
= h
0 max
=
h
0 max
b
1
0
|
b(
0
)
|
(14.26)
Whence, from Equation (14.19c):
t
V
=
h
0 max
|
b(
0
)
|
(14.27)
Further study of the response time 379
Thus, t
V
can be estimated, quite easily, from knowledge of the thickness
of the glacier and the net balance rate at the terminus.
We noted above that t
V
is a time constant in the same sense that 1/γ
0
is. Let us now demonstrate this. Immediately after a permanent change
in balance rate, b
1
, the rate at which additional mass, V
1
,isacquired
by the glacier, dV
1
/dt,isB
1
= b
1
0
.However, as the glacier becomes
longer (by an amount (t)), some of the additional annual input is lost
through ablation in the new part of the terminus region. Thus:
dV
1
dt
= B
1
−
|
b
0
(
0
)
|
Now from Equation (14.26)orFigure 14.9,
∼
=
V
1
/h
0max
, so:
dV
1
dt
= B
1
−
|
b
0
(
0
)
|
h
0 max
V
1
Comparing this with Equation (14.15), it is clear that h
0 max
/
|
b(
0
)
|
is
the analog of 1/γ
0
.
When calculating t
V
in practice, the three-dimensional geometry
of the glacier must be taken into consideration. Thus, in the case
of a glacier like Storglaci¨aren that has a number of overdeepened
basins in its longitudinal profile, h
0max
needs to be replaced by an
appropriate longitudinally averaged thickness. In addition, the termi-
nus of Storglaci¨aren is constrained between bedrock and morainal highs
so that its width is about half the average width of the glacier (Figure
12.9). Accordingly, Equations (14.25)to(14.27) need to be generalized
to three dimensions. For example, if we write Equation (14.25) as:
b
1
A
0
=
|
b(
0
)
|
W (
0
) (14.28)
where A
0
is the initial area of the glacier and W(
0
)isthe width of the
terminus, Equation (14.19c) becomes:
t
V
=
V
1
b
1
A
0
(14.29)
V
1
must now be estimated based on the glacier geometry. For instance,
by analogy with Figure 14.9, one might consider that the new geometry
could be approximated by (mentally) sliding forward the central part of
the glacier of width W(
0
). V
1
is then h
0max
W(
0
) ,where h
0
max
is a
mean thickness over this central part. Inserting this in Equation (14.29)
and using Equation (14.28) then yields:
t
V
=
h
0 max
|
b(
0
)
|
(14.30)
Harrison et al.(2001)have pointed out that the formulation of t
V
in Equation (14.19c) ignores the normal increase in b
n
with elevation.
Owing to this increase, perturbations that result in thickening or thinning

380 Response of glaciers to changes in mass balance
of a glacier are effectively amplified. This positive feedback, known
as the B¨o
ð
varsson effect (B¨oðvarsson, 1955), can lead to significant
underestimates of the response time. In extreme cases, it may lead to
unstable (or runaway) growth or shrinkage of a glacier.
To include this effect, Harrison et al. suggest adding a term to Equa-
tion (14.27), thus:
t
VH
=
H
|
b
e
|
− G
e
H
(14.31)
H is now a thickness scale, not necessarily equal to h
0 max
, b
e
is an effective
balance rate at or just below the terminus, and G
e
is the effective budget
gradient. The subscript H is added to t
V
to distinguish this time scale from
that of J´ohannesson et al. (Readers should refer to Elsberg et al.(2001)
and Harrison et al.(2001) for a rigorous derivation of Equation (14.31)
and definitions of b
e
, G
e
, and H. Suffice it to say here that the theory is
grounded in the reference-surface approach to mass balance discussed
briefly in Chapter 3 (p. 25).) It will be readily seen that the added term
has the desired effect. Both G
e
and H are positive, so subtracting G
e
H
from |b
e
| in the denominator increases t
VH
and in extreme cases may
make t
VH
negative – the unstable response.
Unfortunately, b
e
, G
e
, and H are not easy to determine. To evaluate
b
e
one needs detailed balance rate data from the terminus that can be
extrapolated into the area below the terminus and also a map showing
bed elevations in the terminus area. In an application of their theory to
South Cascade Glacier in the state of Washington (USA), Elsberg et al.
(2001) found that setting b
e
≈ 0.75 b(
0
) yielded a good approximation
to the true value. Likewise, rigorously H = dV/dA and neither V nor
A are measured routinely. Finally, an average value of G
e
may not be
appropriate; again Elsberg et al.(2001) found that setting G
e
equal to 0.9
times the specific balance gradient half way between the terminus and the
equilibrium line worked well on South Cascade Glacier, but they caution
that these approximations may not be appropriate for other glaciers.
An important characteristic of Equation (14.31) appears if one notes
that since b
e
is a balance rate near the terminus, and b
n
= 0atthe
equilibrium line, then b
e
/z
t→e
≈ G
e
,where z
t→e
is the elevation
difference between the terminus and the equilibrium line. Then the ratio
of the two terms in the denominator of Equation (14.31) is:
G
e
H
|
b
e
|
≈
H
z
t→e
Clearly, t
VH
becomes large as this ratio approaches unity, and the system
is unstable if it exceeds unity. z
t→e
will be smaller on glaciers that are
relatively flat, and may approach H on such glaciers. Thus glaciers with
