Henini M. Handbook of Self Assembled Semiconductor Nanostructures for Novel devices in Photonics and Electronics

Подождите немного. Документ загружается.

Quantum Dot Superluminescent Diodes 579

at high injection currents due to the higher degeneracy of ES energy levels and shows 22 nm

FWHM. The ES level can contribute to gain with twice as many carriers as the corresponding GS

level due to higher angular momentum degeneracy [38] . The multistate emission, peculiar in QD

systems, is promising for the achievement of very broad spectral characteristics, provided that

the emission from each line can be broadened enough to achieve a smooth spectral superposition

at the injection level when the intensity of the two lines is comparable.

In the previous paragraph we discussed the inﬂ uence of p-doping on the modal gain, reporting

an increase of

mod

max

for increasing doping level. Figure 19.12b reports the L – I characteristics of

three identical 4 mm long SLEDs realized from the same samples whose gain curves are reported

in Fig. 19.10 . The effect of p-doping is conﬁ rmed by the analysis of the L – I characteristics shown

in Fig. 19.12b . The output powers in the regime of GS  ES emissions (circles in ﬁ gure) go up to

60–70 mW for the highly p-doped device while stay limited to less than 10 mW for the undoped

one. These measurements demonstrate that the use of p-doping in QD superluminescent diodes

results in increased output powers and conﬁ rm the estimation of a modal gain increase larger

than the internal loss increase for the considered doping levels.

These samples, as the ﬁ ve QD layers sample previously presented, were not optimized for

achieving large bandwidths and the spectral characteristics were very similar to the ones

reported in the inset of Fig. 19.12a , with well-separated GS and ES emissions. In the next para-

graph we report the characteristics of QD-SLEDs with improved spectral characteristics.

19.3.3 Wide-gain devices

As discussed in sections 19.2.1 and 19.2.2 the spectral characteristics of QD-SLEDs can be

improved through the use of chirped multilayers and/or increasing the inhomogeneous broad-

ening of each QD layer by optimizing the growth conditions. Some feedback about the optical

properties of such structures can be obtained through the analysis of the spectral and L – I char-

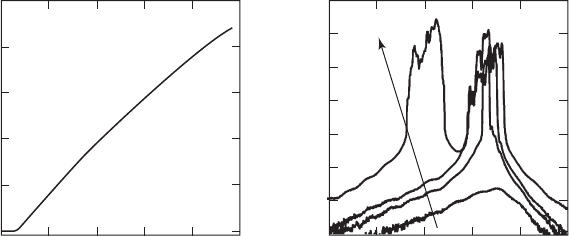
acteristics of ridge-waveguide lasers. As showed in Fig. 19.13a , 2 mm long lasers realized from

a ﬁ ve-QD layer chirped structure (PL emission reported in Fig. 19.5 ) show low threshold cur-

rent densities of about 200 A/cm

and high slope efﬁ ciencies of 0.22 W/A per facet, signatures

of high recombination efﬁ ciency and therefore good crystal quality. Figure 19.13b displays the

corresponding spectra. The device starts lasing through GS recombination around 1265 nm.

The emission line progressively broadens with increasing current up to a linewidth of 35 nm, dem-

onstrating that chirping is effective in broadening the gain spectrum. At very high injection lasing

takes place also through ES recombinations and a second lasing line appears also on the high-

energy side of the spectrum, as already observed on standard 1.3 μ m lasers [39, 40] . The very

large lasing line, stemming from a uniformly broadened spectral gain, is very promising for the

2

3

4

5

6

7

8

0 50 100 150 200 250

600 mA

100 mA

25 mA

8 mA

Output power (mW)

Intensity (a.u.)

1100 1150 1200 1250 1300 1350

Wavelength (nm)Current (mA)

Slope: 0.22 W/A

(a) (b)

1 μs pulsewidth,

5% duty cycle

Figure 19.13 (a) L – I characteristics of a 2 mm long and 5 μ m wide Fabry–Perot ridge-waveguide laser realized

using ﬁ ve chirped QD layers. (b) Corresponding spectra.

CH019-I046325.indd 579CH019-I046325.indd 579 6/27/2008 5:21:33 PM6/27/2008 5:21:33 PM

580 Handbook of Self Assembled Semiconductor Nanostructures for Novel Devices in Photonics and Electronics

realization of tunable lasers with large tunability ranges. In the framework of the project EU FP6

integrated project “ ZODIAC ” , tunable distributed feedback lasers were realized from this sample

with a tunability range of about 70 nm.

1.5

4

5

6

7

8

Output power (mV)

Intensity (a.u.)

(a) (b)

0.5

Current (A)

Wavelength (nm)

Room temperature

1 s pulsewidth 5% duty cycle

Current (mA)

800

500

300

200

100

0.2

0.4 0.6

GS  ES

120 nm

0.8

1000 1100

1200

1300 1400

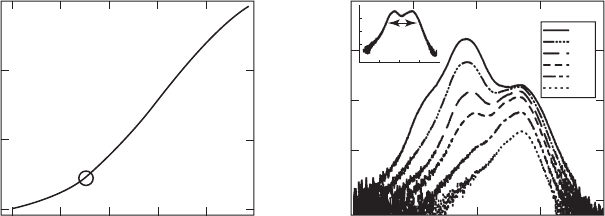
Figure 19.14 (a) L – I curve of a 4 mm long SLED realized using ﬁ ve chirped QD layers, measured at room

temperature in pulsed regime. The circle identiﬁ es the regime of comparable GS and ES emissions. (b) Corresponding

spectra. The 300 mA spectrum is reported in the inset, showing a bandwidth of 120 nm.

Tilted ridge-waveguide SLEDs fabricated from the same wafer show a behaviour similar to the

one reported for the narrow gain sample but with smaller saturated output powers, which results

from the decreased maximum modal gain produced by the bandwidth increase [14, 17] . In Fig.

19.14a the room-temperature L – I characteristics of a 4 mm long and 5 μ m wide SLED are reported.

The saturated output power in this case goes up to a few hundred μ W for the GS emission and up to

a few mW when the SLED operates in the ES emission regime. Figure 19.14b displays the spectral

characteristics of the same device. At low injection current the emission from the GS dominates,

with a spectral width of 55 nm (FWHM) centred around 1265 nm. The ground state then saturates

with increasing current and the carriers begin to ﬁ ll the excited states, resulting in a broadening of

the spectrum on the short wavelength side as already observed on a narrow gain sample. At cur-

rents  400 mA the ES emission eventually dominates the spectrum, due to the higher maximum

gain on the ES. At 300 mA, due to simultaneous contribution of the two states, a combined spectral

width of 120 nm is obtained, with a 3 dB dip between GS and ES peaks (see spectrum in inset). This

bandwidth is very promising for the application in high-resolution OCT systems; however, the out-

put power in this regime is limited to a few hundred μ W and does not meet the mW-range output

powers required by the application. A way to achieve higher output powers, as detailed in section

19.3.1, is the use of a higher number of QD layers in the active region, p-doping the active region

and using bent waveguides. Tilted-ridge SLEDs, 4 mm long, with similar linewidth characteristics

but a higher number of QD layers in the active region result in improved output power as shown

in the inset of Fig. 19.15a . The power in the regime of large bandwidth (  100 nm) increases from

the 300 μ W of the ﬁ ve QD layer SLED up to 2–3 mW for a ten QD layer SLED and up to 10–15 mW

for an 18 QD layer SLED. Both the ten and the 18 QD layer samples maintain a spectral charac-

teristic similar to the one of the ﬁ ve QD layer sample, with a 3 dB peak to valley ratio between GS

and ES. Best SLED performance in a chirped structure can be achieved using 18 QD layers with p -

doped GaAs spacers (10 nm thick regions, 10 nm above each QD layer, doping level 5e17 cm

 3

) and

0.35

0.65

As claddings. Three repetitions of six chirped QD layers were used, where chirping was

performed using different In content in the capping layers of ﬁ ve QD layers and also adjusting the

amount of InAs deposed to grow the QDs of the sixth layer.

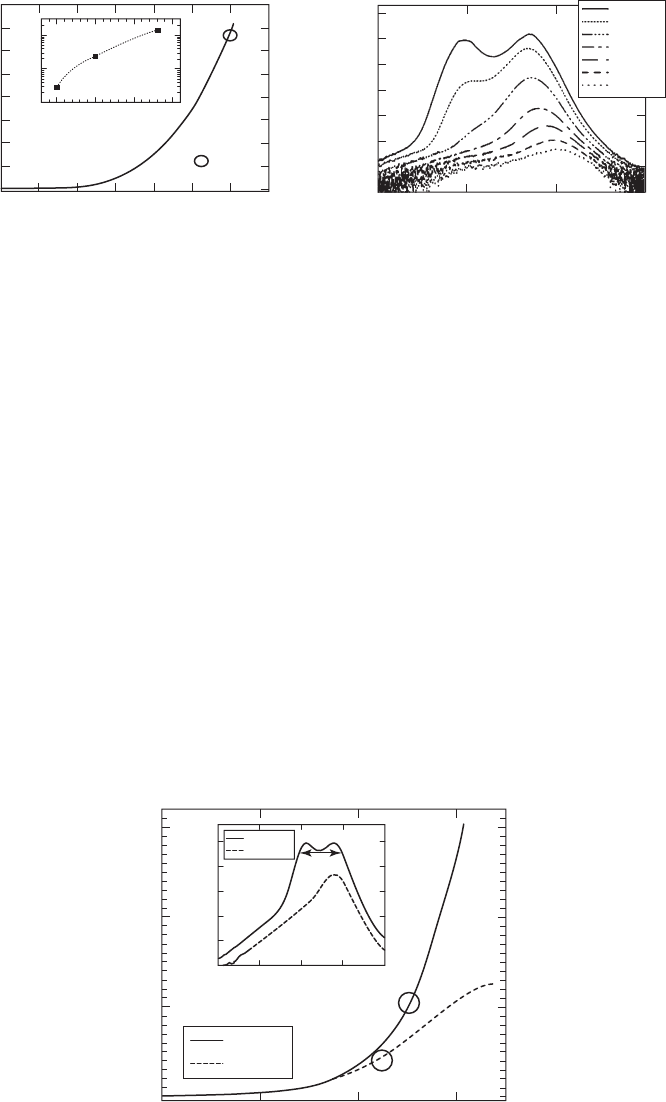
In Fig. 19.15a we report the L – I characteristics of a 4 mm long tilted SLED realized from this

structure. The measurements were taken in pulsed regime (50 kHz, 4% duty cycle, at a heat-sink

temperature of 20°C. The output power reaches several tens of mW at the highest current levels.

A similar contrbution from GS and ES (broad spectral regime indicated by a circle) is achieved at

a current of 1200 mA, corresponding to a high output power of  30 mW. This could be further

increased by applying anti-reﬂ ection (AR) coatings.

CH019-I046325.indd 580CH019-I046325.indd 580 6/27/2008 5:21:33 PM6/27/2008 5:21:33 PM

Quantum Dot Superluminescent Diodes 581

Figure 19.15b shows the corresponding spectral characteristics. The device shows the usual

GS–ES spectral behaviour, with a large GS  ES combined bandwidth at high injection. A band-

width of 100 nm (FWHM) centred at 1235 nm, with a relatively small 5 dB valley between GS

and ES emissions is reached around 1200 mA. The pulsed operation provides an estimate of the

best performance that could be achieved with these devices; however, a CW operation is neces-

sary for the applications, which could be limited by heating at the high currents where the best

bandwidth/power performance is achieved. In this regard the use of bent-stripe devices may be

helpful, because it allows the achievement of higher output powers at lower currents. The appli-

cation of bent structures also opens the way to the use of shorter cavities which may be desir-

able for mass production, and which could be further pursued by increasing the reﬂ ectivity of the

straight facet through high-reﬂ ection (HR) coatings. This kind of structure can compensate for

the low gain showed by QDs emitting in the 1.3 μ m region as compared to quantum wells (QWs).

A comparison between the pulsed and CW L–I characteristics measured on a 2 mm bent SLED are

reported in Fig. 19.16 . CW and pulsed characteristics are superposed up to 300–400 mA (corre-

sponding to 5–7 kA/cm

). At higher bias the CW characteristics become dominated by heating

effects and show a roll-over around 700 mA. The CW output power in the regime of large band-

width is about 2–3 mW corresponding to 100 nm spectral bandwidth and a 3 dB dip between GS

and ES peaks. The spectral characteristics are shown in the inset. Higher output power could be

achieved by applying HR coatings on the straight facet and AR coatings on the tilted facet.

2

3

4

5

6

7

8

9

0 200

(a) (b)

400 600

Power at GS  ES (mW)

0.1

5101520

Number of QD layers

GS  ES

Current (mA) Wavelength (nm)

Intensity (a.u.)

Output power (mW)

800

1000

1200

1400

1100 1200

1200 mA

750 mA

450 mA

300 mA

220 mA

150 mA

100 mA

1300 1400

Figure 19.15 (a) L – I characteristics of a 4 mm long and 3 μ m wide tilted SLED containing 18 chirped QD layers

and p -doping. In the inset are reported the output powers of SLEDs containing ﬁ ve, ten and 18 undoped QD layers

(squares) emitting in the GS  ES regime. The dashed line is a guide to the eye. (b) Spectra corresponding to the L – I

characteristics shown in (a).

1000 1100 1200

430 mA

200 mA

100 nm

1300 1400

Wavelength (nm)

Intensity (a.u.)

Power (mW)

0 200 400

3

4

5

6

7

8

Current (mA)

600

Pulsed

Figure 19.16 Pulsed (continuous line) and CW (dashed line) L – I characteristics of a 2 mm long bent QD-SLED

containing 18 p -doped QD layers. The corresponding CW spectra are reported in the inset.

CH019-I046325.indd 581CH019-I046325.indd 581 6/27/2008 5:21:34 PM6/27/2008 5:21:34 PM

582 Handbook of Self Assembled Semiconductor Nanostructures for Novel Devices in Photonics and Electronics

The modulation between GS and ES emissions affects the coherence properties of the emission

resulting in a coherence function with non-negligible side lobes, which can reduce the effective

resolution achieved once the SLEDs are applied to OCT. A detailed description of the coherence

properties of QD-SLEDs is reported in the next section.

19.3.4 Coherence properties

The coherence length l

is a quantity frequently used to characterize the coherence properties

of the light source, as it is directly related to the resolution achievable in the OCT technique. l

related to the maximum path difference between the two arms of an optical interferometer for

which the light wave is still capable of generating an interference pattern and it is often deﬁ ned

as the FWHM of the ﬁ rst order degree of coherence. To a ﬁ rst approximation it can also be

expressed in terms of the spectral width as:



(19.5)

where λ is the central wavelength, Δ λ the spectral broadening and k is a spectrum form factor

corresponding to 0.32 for Lorentzian-, to 0.66 for Gaussian- and to 1 for rectangular-shaped

spectra (values derived using the power-equivalent width of the corresponding coherence func-

tions [41] ).

The ﬁ rst-order degree of coherence is easily measured with an optical interferometer. Besides

the FWHM, its shape is also of interest for OCT. The spectral modulations (as the valley between

GS and ES emissions) result in short-range side lobes of the coherence function which can reduce

the effective OCT resolution, while the presence of a short-range spectral modulation (as the

residual FP modulation) results in long-range secondary subpeaks. The residual Fabry–Perot

modulation (commonly called spectral ripple) results in parasitic subpeaks of the coherence func-

tion at an optical path difference equal to 2 n

eff

L were L is the SLED length and n

eff

the refractive

index for the guided mode. A high intensity of such secondary subpeaks, which is determined

by the spectral modulation depth, is undesirable as it may produce distortion effects when using

the SLED for imaging. As QD-SLEDs usually present low gain values, ripple is not expected to

be a concern and its analysis will be omitted here. Also, the devices were not optimized to

minimize the optical feedback through the application of AR coatings, which would be desirable

for the analysis of the secondary subpeaks of the coherence function.

Figure 19.17b displays the short-range fringe visibility measured with a Michelson

interferometer-based optical spectrum analyser on two different 2 mm long tilted SLEDs, one

1.2

0.8

0.6

0.4

0.2

0.1

0.01

1000 1100 40 20 0 20 40

Chirped

Narrow gain

Calculated

Mirror displacement (μm)

1200

(a) (b)

Chirped

Narrow gain

1300 1400

Wavelength (nm)

Intensity (a.u.)

Degree of coherence

Figure 19.17 (a) CW spectra measured over a narrow gain and a chirped 2 mm long SLED. The spectra have been

shifted vertically for clarity. (b) Corresponding degree of coherence measured observing the fringes ’ visibility in a

Michelson interferometer (dots and rhombs). The degree of coherence calculated through inverse Fourier transform

of the chirped spectrum is also reported (continuous line).

CH019-I046325.indd 582CH019-I046325.indd 582 6/27/2008 5:21:34 PM6/27/2008 5:21:34 PM

Quantum Dot Superluminescent Diodes 583

with a narrow gain spectrum and the other chirped. The measurements are reported for the nar-

row gain device emitting in a pure GS regime (rhombs) and for the chirped device emitting in a

GS  ES regime (dots). The corresponding spectra are displayed in Fig. 19.17a in linear scale,

and show an FWHM of 45 and 180 nm, corresponding to an FWHM of the measured degree

of coherence of 28 and 9 μ m, respectively. The chirped spectrum has been shifted vertically for

clarity. While the single peak spectra correspond to a single peak coherence function (in the

ideal case of a Gaussian spectrum the coherence function is also Gaussian), the chirped GS  E S

emission generates side lobes that could result in a reduced OCT resolution. The side lobes in

the degree of coherence of the chirped spectrum show an 8 dB suppression ratio. During these

measurements the chirped sample operated on a very low gain regime and low output power of a

few hundred μ W (ﬁ ve QD layers, 2 mm tilted cavity) which resulted in the extremely broad band-

width of 180 nm and l

≈ 1 0 μ m. On a high-gain SLED the bandwidth is rather expected to be

about 100 nm, which would result in l

≈ 1 5 μ m.

Spectral emission and coherence function are connected through a Fourier transform. It can

be shown that the spectral density S ( v ) and coherence function G ( τ ) of the radiation are related

through the Wiener–Khinchin theorem [41] . The theorem is a useful tool as it allows the calcu-

lation of the degree of coherence starting from any arbitrary spectral shape of the emission, thus

avoiding repetitive and time-consuming experiments. We checked the validity of calculations

through a comparison with the coherence measurements. Together with measurements, the

degree of coherence calculated through inverse Fourier transform of the chirped spectrum is

reported in Fig. 19.17b (continuous line). We ﬁ nd a very good agreement, with almost negligible

errors over more than two orders of magnitude. The calculations can then be applied to evaluate

the coherence function for SLEDs emitting in the regime of high power and GS  ES emission, as

for the case of the 18 QD layer, p-doped SLEDs described in the previous paragraph. The degree of

coherence calculated from a spectrum with 100 nm bandwidth and a 3 dB dip between GS and

ES emissions (spectrum in inset of Fig. 19.16 ) shows 15 μ m FWHM and a 5 dB suppression ratio

of side lobes. As we will see in section 19.4, a much better side lobe suppression can be obtained

with a ﬂ at-top spectral emission.

19.3.5 Temperature characteristics

Since their theoretical proposal QD devices have been predicted to show better temperature sta-

bility when compared to their QW or bulk counterpart [42, 43] . Due to the discrete nature of QD

energy levels, carriers are supposed to suffer a smaller thermal dispersion resulting in a lower

thermal sensitivity of devices. In spite of these predictions most of the devices realized so far

showed a temperature dependence similar to the one obtained on InP-based devices for 1.3 μ m

emission, with laser characteristic temperature T

smaller than 100 K in the 20–80°C interval

[44–47] . To explain the temperature sensitivity of the QD lasers, several different mechanisms

have been proposed in the literature. Re-emission of carriers towards the barrier and wetting

layer activated by increasing temperature and the subsequent radiative and/or non-radiative

recombinations outside the dots is one of these [48, 49] . Indeed Matthews et al. showed that the

temperature sensitivity of short wavelength (1 μ m) QD lasers is caused by gain saturation due to

the presence of a high density of states in the WL [36] . Other authors, in contrast, have attributed

the temperature characteristics of QD lasers to the thermal sensitivity of non-radiative recombi-

nations [50] or to the spreading of holes over the closely spaced energy levels of the valence band

[37] . The situation is different in p -doped QD devices, for which lasers with improved temperature

characteristics have been recently demonstrated [56–58] . The idea of using p -doping to reduce

the temperature dependence, which was ﬁ rst proposed in QDs by Shchekin and Deppe [37] ,

derives from the hypothesis of a thermal dependence governed by the spreading of holes over the

closely spaced energy levels in the valence band. Forcing a high number of holes to be conﬁ ned

in the WL and QD valence band through p -doping should therefore reduce the inﬂ uence of ther-

mal spreading. In contrast to this picture, two independent authors have recently reported that

the temperature dependence of the current threshold in p -doped QD lasers at room temperature

stems from a redistribution of carriers over the QD ensemble [51, 52] . This is likely to happen

around room temperature (in undoped devices a similar phenomenon takes place around 200 K)

CH019-I046325.indd 583CH019-I046325.indd 583 6/27/2008 5:21:35 PM6/27/2008 5:21:35 PM

584 Handbook of Self Assembled Semiconductor Nanostructures for Novel Devices in Photonics and Electronics

due to the strong Coulomb attraction in p -doped devices, which results in an increased potential

barrier. As we will see in the following paragraph, p -doping is not only beneﬁ cial to improve the

gain characteristics of QD-SLEDs, but also results in a higher temperature stability of the SLED

L – I curves. While the temperature dependence in undoped devices can be explained in terms of

a temperature increasing non-radiative recombination, a more complex dynamics is at the origin

of the higher stability in p -doped structures.

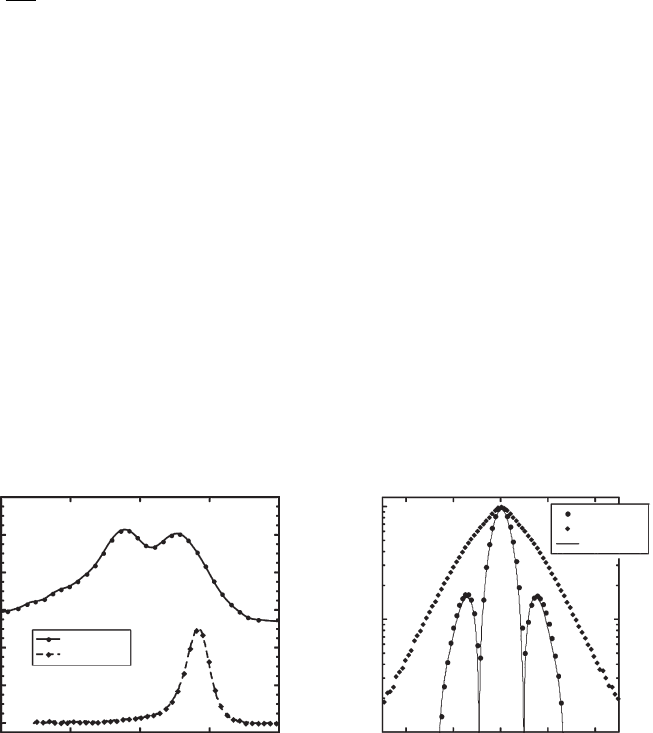
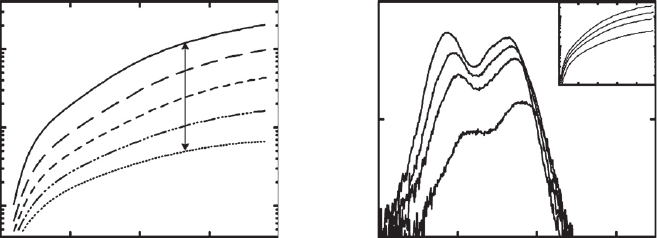
Figure 19.18 shows the typical temperature characteristics of undoped QD-SLEDs. Temperature

controlled measurements were performed mounting the devices p -side up on a copper sub-mount

with the double function of n -electrode and heat-sink. The temperature of the heat-sink was

controlled with a Peltier thermoelectric cooler. In the left panel the L – I characteristics of 4 mm

long and 3 μ m wide narrow gain device (ten QD layers) are reported for increasing temperatures

in the interval 20–85°C. At high injection the output power exhibits a thermal degradation of

15 dB, which is similar to the decrease observed in SLEDs realized with the competing InP-based

technology. The reduction in output power is associated with a reshaping of the emission spectra,

which vary their bandwidth and GS/ES intensity ratio. The typical spectral behaviour is shown in

the right panel of Fig. 19.18 where the spectra of the chirped ﬁ ve QD layer SLED described in sec-

tion 19.3.3 are displayed for increasing temperature (20–60°C), at the ﬁ xed current of 300 mA.

The corresponding L – I caracteristics are reported in the inset, with a decrease similar to the nar-

row gain sample. The current was ﬁ xed at 300 mA so as to have a broad spectral emission (GS 

ES) at 20°C. When the temperature is increased the spectral intensity is decreased with a higher

suppression for the ES emission than for the GS, which must be related to a smaller steady-state

population of carriers in the dots. One possible explanation for the reduced carrier density ver-

sus temperature might be found in an increase in non-radiative recombinations. The overall

spectrum moves to the red linearly with a rate of about 0.5 nm/K due to the usual T -dependence

of the band gap in InAs and GaAs.

8

300 mA pulsed

7

8

Pulsed

20°C

30°C

40°C

60°C

40°C

55°C

70°C

85°C

0.1

(a)

200

1100

(b)

1200 1300

1400

Wavelength (nm)

400

Current (mA)

Power (mW)

Intensity (a.u.)

600

15 dB

800

0400

Current (mA)

Power (mW)

800

20°C

30°C

40°C

60°C

0.1

0.01

Figure 19.18 (a) L – I characteristics for varying temperatures of a 4 mm long, 3 μ m wide narrow-gain SLED

with tilted ridge-waveguides. (b) Spectral characteristics of a 4 mm long, 5 μ m wide chirped SLED with tilted ridge-

waveguides for varying temperatures. The corresponding L – I curves are shown in the inset.

As we have described in section 19.3.1 the L – I characteristics of a superluminescent diode are

determined by modal gain and internal losses. Both these quantities were therefore analysed ver-

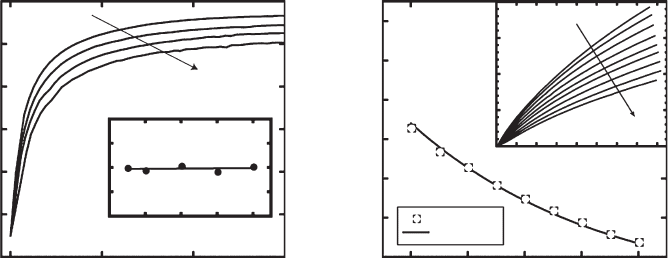
sus temperature to identify the origin of the temperature degradation. Figure 19.19a shows the

modal gain curves measured on a tilted ridge-waveguide at 20, 40, 60 and 80°C at the GS peak

position (narrow gain, same sample as Fig. 19.18a ). The gain was measured with the technique

described in section 19.3.1, studying the ampliﬁ cation of a tunable laser injected and detected

from the opposite facets of the waveguide. The laser wavelength was adjusted to the GS peak posi-

tion for each temperature. The curves show a stronger saturation of gain for increasing tempera-

ture. This behaviour was conﬁ rmed over a series of different samples with undoped active regions

and is therefore a general ﬁ nding. The internal losses, in contrast, show a completely temperature

CH019-I046325.indd 584CH019-I046325.indd 584 6/27/2008 5:21:35 PM6/27/2008 5:21:35 PM

Quantum Dot Superluminescent Diodes 585

independent characteristic. They were measured through the analysis of the transmission of the

tunable laser tuned out of resonance with the QD spectrum. A plot of the experimental points

together with a linear ﬁ t are displayed in the inset of Fig. 19.19a . SLED characteristics are there-

fore completely determined by the temperature variation in modal gain.

1.4

1.2

0.8

0.6

0.4

0.2

20°C

80°C

10

20

30

40

(a) (b)

500

Modal gain (cm

1

)

1000

1.1

1.05

0.95

0.9

Normalized

internal losses (a.u.)

Power (mW)

020

0.01

0.02

20°C

100°C

0.03

Current (mA)

12 16

60 80

Experiment

Exp. fit

Temperature (°C)

1500

40 60

20 80

100

Temperature (°C)

External efficiency (a.u.)

Current density (A/cm

)

Figure 19.19 (a) Modal gain curves for different temperatures, measured on a narrow-gain 2 mm long tilted ridge-

waveguide (realized from the same wafer of the SLEDs whose L – I characteristics are reported in Fig. 19.18 ). The

normalized internal loss versus temperature is reported in the inset (circles) together with a linear ﬁ t. (b) Single facet

external efﬁ ciencies of short tilted waveguides (500 μ m) realized from the same wafer. The points are ﬁ tted assuming

an exponential decrease of the ratio τ

/ τ

. The corresponding L – I characteristics are displayed in inset.

Two physical processes are seen as possibly responsible for the temperature dependence of

gain in these QDs. The ﬁ rst is the dispersion of holes over the closely spaced energy levels in the

valence band. This would result in an increase in the spontaneous emission decay time τ

due

to the decreased probability of electron–hole recombination. The second is the increase in non-

radiative recombination which can derive from Auger [53] and/or defect assisted processes [54] .

This would result in a decrease in the non-radiative lifetime τ

, thus subtracting carriers to the

radiative recombinations. Additional insight about the different processes is obtained from the

L – I characteristics of LEDs versus temperature. Indeed, in an LED the effects related to stimu-

lated emission and absorption can be neglected resulting in a simple dependence of the emitted

output power on the carrier populations. This can be done measuring the low-injection, edge-

emitted electroluminescence from short tilted waveguides L  5 0 0 μ m (where gain and losses

can be neglected), fabricated on the same wafer as the SLED. The assumption of low gain and

losses is experimentally conﬁ rmed by the absence of any evident spectral narrowing of the edge-

emitted electroluminescence for increasing current injections. The inset of Fig. 19.19b shows

the L – I characteristics of such a structure in the 20–100°C temperature interval. The efﬁ ciency

decrease versus temperature is exponential at all the current levels, and a characteristic tempera-

ture T

 70 K is found by exponential ﬁ tting of the low injection efﬁ ciency. The experimental

points together with the ﬁ t are displayed in Fig. 19.19b . A similar T

is found from ﬁ tting of the

threshold characteristics of Fabry–Perot lasers realized from the same sample, suggesting that

the same processes are at the origin of the temperature characteristics of lasers around room

temperature. However, as the internal efﬁ ciency at ﬁ xed injection in an LED structure is expected

to vary proportionally to the ratio τ

/ τ

, the intensity decrease of the curves versus temperature

does not allow one to distinguish between an increase in the spontaneous emission decay time

and a decrease in the non-radiative lifetime τ

Further information about the temperature behaviour is provided by the analysis of the

temperature-dependent decay time of the photoluminescence signal. Measurements were per-

formed on a test sample containing one QD layer grown under the same conditions used for the laser

structure. At low temperature (0–200 K) the decay time slightly increases which can be attributed

CH019-I046325.indd 585CH019-I046325.indd 585 6/27/2008 5:21:35 PM6/27/2008 5:21:35 PM

586 Handbook of Self Assembled Semiconductor Nanostructures for Novel Devices in Photonics and Electronics

to an increase of τ

determined by the hole spreading over the closely spaced energy levels in

the valence band. In contrast, around room temperature the decay time undergoes an important

decrease (associated with the efﬁ ciency decrease) which can be associated with a reduction of τ

(increased non- radiative recombinations). We attribute to this effect the temperature character-

istics of undoped QD devices around room temperature. A similar temperature dependence has

been recently reported in [55] , where the authors have measured the decay time studying the

time-resolved differential photoluminescence induced by pump and probe laser pulses. Increasing

non-radiative recombinations could be due to a temperature-activated non-radiative chan-

nel in the dots, or to thermal evaporation and subsequent non-radiative recombination in the

WL. Detailed calculations show that the T -dependence of gain, LED efﬁ ciency, laser thresholds

and lifetime can all be explained by hole evaporation to the WL and defect-related non-radiative

recombination in the WL.

The situation is different in p -doped QD devices, for which lasers with improved temperature

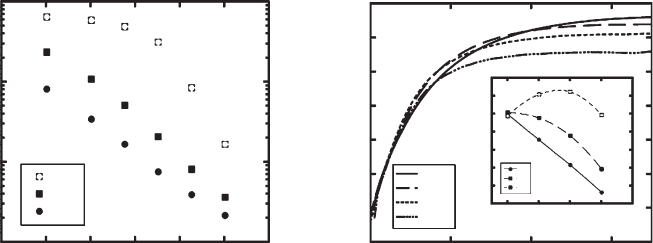
characteristics have been recently demonstrated [56–58] . Figure 19.20a shows a comparison

between the temperature degradation of output power at ﬁ xed current for SLEDs with and with-

out p -doped actve regions (same devices as described in section 19.3.2). The output power shows

good temperature stability in the temperature interval 20–60°C and an important decrease

at higher temperatures for the highly doped device (C). In contrast, the power decrease in the

undoped SLED (A) is exponential over the full temparature range 20–85°C, while an intermediate

behaviour is observed for the slightly doped device (B). Similar characteristics have been reported

in edge emitting lasers, with temperature insensitive threshold characteristics in the interval 20–

70°C for p -doped structures [57, 58] . As in the case of the intrinsic sample we measured gain

characteristics and internal losses over 2 mm long tilted waveguides. Also the p -doped samples

show temperature independent internal losses and strongly T -dependent gain curves, conﬁ rming

that gain is the main driving force for the temperature dependence of device characteristics. The

gain curves measured at the GS peak position of sample C are displayed in Fig. 19.20b . At high

injection the curves show a gain saturation versus temperature similar to the undoped device. In

this regime the carrier concentration is very high and the dots are expected to be strongly ﬁ lled

(GS, ES and part of the higher excited states) so that the gain decrease is likely produced by a sim-

ilar decrease in the non-radiative lifetime for p -doped and undoped devices. On the other hand,

the behaviour is different at smaller injection, where the doped sample inverts the trend show-

ing higher gain values at higher temperatures. The inset of Fig. 19.20b reports the modal gain

extracted at a ﬁ xed current corresponding to a gain of about 8 cm

 1

at 20°C versus temperature,

for the undoped and p-doped samples. The gain decrease for the intrinsic sample is linear while

100

10

20

30

40

0.1

20°C

40°C

60°C

80°C

Modal gain (cm

1

)

Temperature (°C)

100

2

1000

Modal gain (cm

1

)

2000 3000

Current density (A/cm

)

20 40 60 80

(a) (b)

Power (mV)

Temperature (°C)

100 120

Figure 19.20 (a) Output power versus temperature measured at ﬁ xed current for QD-SLEDs with identical epitaxial

structure but different doping levels of the active region. A  undoped, B  slightly p -doped, C  highly p -doped. The

currents are chosen so that GS  ES emission at room temperature. (b) Modal gain curves for different temperatures,

measured on a narrow-gain 2 mm long tilted ridge-waveguide with p -doped active region (sample C). Inset : Modal gain

versus temperature measured at ﬁ xed injection for samples A, B and C. The lines are guides to the eye.

CH019-I046325.indd 586CH019-I046325.indd 586 6/27/2008 5:21:36 PM6/27/2008 5:21:36 PM

Quantum Dot Superluminescent Diodes 587

the highly p -doped sample (C) shows a gain increase up to 60°C, and a 80°C value as high as

20°C (the situation is again intermediate for the slightly p -doped sample). This effect might be

produced by thermal escape of carriers from the dots and transfer into the deeper levels (larger

dots) where they can take part more effectively to the gain at the peak position. The decrease in

current threshold exhibited by lasers with undoped QDs at lower temperatures (around 200 K)

has also been attributed to this effect [50] . The likely reason why thermalization does not start

to become signiﬁ cant until higher temperatures in p -doped devices is the more difﬁ cult carrier

evaporation due to the electrostatic attraction and band bending. The presence of a more efﬁ cient

capture in p -doped QDs has been demonstrated through the analysis of time-resolved PL decay

times [59] , while the thermal redistribution of carriers has been reported observing the narrow-

ing of the spontaneously emitted electroluminescence spectra versus temperature [51, 52] .

To summarize, an important decrease (15 dB) in the output powers of undoped SLEDs is

observed in the 20–85°C temperature interval, which is comparable to the typical thermal deg-

radation observed in InP-based SLEDs. This is produced by thermally activated non-radiative

recombinations, which can be deduced through the analysis of the L – I curves and extraction efﬁ -

ciencies of short tilted cavity devices and through the analysis of the temperature-dependent PL

lifetime measured in time-resolved experiments. Detailed calculations show that the main proc-

ess at the origin of such degradation is thermal evaporation of holes towards the wetting layer

and subsequent non-radiative recombinations. In contrast, a better temperature stability can

be achieved through the use of p -doping in the 20–60°C temperature interval. The temperature

characteristics of devices with p -doped active regions present a more complex behaviour. Better

stability can be achieved for the SLED emission and for the laser thresholds but usually at the

expense of a higher threshold current. Here the temperature dependence is driven by a different

interplay versus temperature of non-radiative recombinations, thermalization of carriers over

the QD ensemble and variation of the radiative decay times.

19.4 Modelling QD SLEDs

Rate-equation models (REMs) are widely used in the literature to model a QD system [60–64] ,

inspired by the description of average carrier densities in bulk materials, quantum wells or quan-

tum wires. In this approach the temporal evolution of carrier populations and photon numbers

inside an optical cavity may be modelled through a system of coupled differential equations. For

the simulation of laser diodes a mean ﬁ eld model considering all the quantities averaged over the

cavity position may be fully satisfactory as the ﬁ nite reﬂ ectivities of the cavity facets make the

photon distribution rather uniform across the device length. In contrast, in devices operating

on a single pass like superluminescent diodes, photon numbers and carrier populations can be

strongly position dependent and a more sophisticated model developed with a travelling-wave

(TW) approach is necessary. A TW-REM model for the calculation of QD-SLED characteristics is

introduced in the next two sections, ﬁ rst in a single-mode approximation that allows the calcula-

tion of the L – I curves of narrow gain devices, and then introducing homogeneous and inhomo-

geneous broadenings to reproduce the spectral characteristics.

19.4.1 Travelling-wave rate-equation models

The fact that the spatial dependence of gain saturation cannot be neglected in low Q cavities was

pointed out in some early papers at the beginning of the 1970s [65, 66] . This has led to a revi-

sion of the rate equations which incorporates a spatial dependence to study the ampliﬁ cation of

light pulses in ampliﬁ ers [67] , to analyse mode locking in lasers [68] , or to model the L – I charac-

teristics of superluminescent diodes [69] . From the model presented in [69] , here we develop a

travelling-wave rate-equation model (TW-REM) that can be used to model the L – I characteristics

of QD-SLEDs. The TW-REM will be further developed in the next section, to account for the spec-

tral broadening of the QD emission, which will allow the calculation of SLED spectra.

A starting point is to express the photon numbers into the guided mode as position dependent.

This can be done introducing the linear photon densities n

ϕ 

(number of photons in the optical

CH019-I046325.indd 587CH019-I046325.indd 587 6/27/2008 5:21:36 PM6/27/2008 5:21:36 PM

588 Handbook of Self Assembled Semiconductor Nanostructures for Novel Devices in Photonics and Electronics

mode per unit length) at the spatial point x , which are directly related to the ﬁ eld amplitudes E



of a forward (  ) and backward (  ) travelling wave:

nn n



ϕϕ

(19.6)

with the photon densities

∝



in a slowly varying envelope approximation. As for pho-

tons, we consider linear densities for carriers rather than carrier numbers, dependent on time

and x position along the cavity.

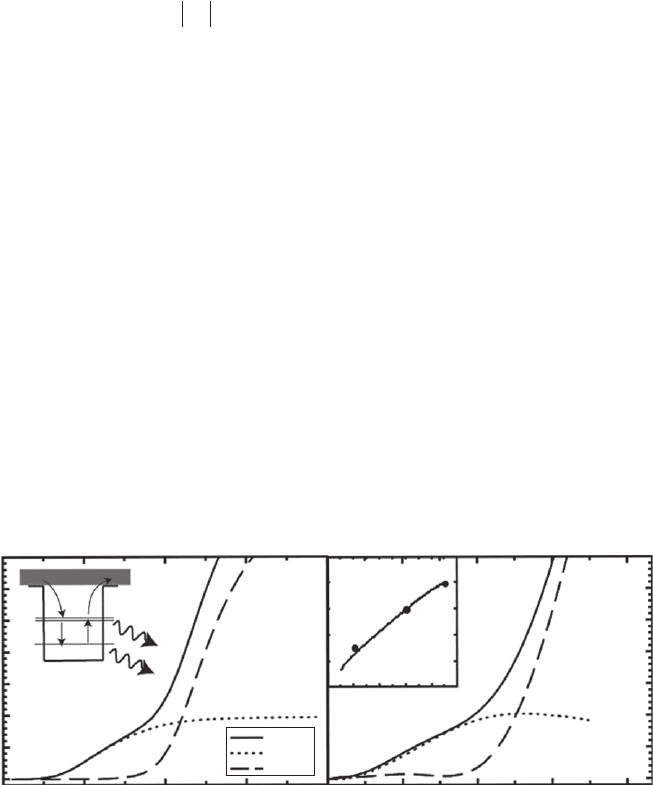
The QD system is modelled (see sketch in inset of Fig. 19.21a ) considering two bound states

(GS and ES) and a reservoir level with much higher degeneracy where the current is directly

injected (corresponding to the wetting layer (WL) in the real system). We neglect the presence

of the highest excited states (QDs emitting at 1.3 μ m usually show 4–5 distinct emissions stem-

ming from recombinations between different bound states [70] ) and consider a direct capture

from the wetting layer to the ﬁ rst excited state, thus reducing the number of equations to be

solved. Electrons and holes are considered as bound excitons, and are described through a single

equation for each energy level (GS, ES and WL). Carrier transitions between different energy lev-

els are described in terms of a constant relaxation time from the ES to the GS, and capture time

from the WL to the ES. Capture and relaxations are the ones observed in time-resolved PL experi-

ments, and the energy separation between GS, ES and WL corresponds to the separation between

the energies associated with the transitions. A drawback of this model is the absence of closely

spaced energy levels for the holes. This results in almost temperature-independent calculations

in the range 0–350 K, as the spacing between energy levels is larger than k

T . This does not rep-

resent a limitation if the model is applied for calculations at a ﬁ xed temperature. In general, the

dynamics of electron–hole populations in QD ensembles is still the object of discussions and it is

not completely clear whether they can be considered excitons or free carriers. This is because of

the complex correlation existing between electron and hole capture processes and likely mediated

by Auger mechanisms.

3.5

2.5

1.5

0.5

(a) (b)

GSES

Output power (mW)

0 0.3 0.6

0.9

Current (A)

1.2

0.1

0.01

SLD length (mm)

Figure 19.21 (a) Calculated L – I characteristics of a 3 mm long SLED containing ﬁ ve QD layers (continuous

line). The GS (dotted) and ES (dashed) contributions to the full L – I characteristics are also reported. A sketch of

the rate equation model used for the L – I characteristics calculation of QD-SLEDs is displayed in the inset. (b) L – I

characteristics measured on a 3 mm long narrow-gain SLED containing ﬁ ve QD layers. GS and ES contributions to

the L – I were separated by spectral ﬁ ltering. Inset : Measured (dots) and calculated (line) saturated GS output power

versus cavity length for tilted SLEDs.

Figure 19.21 displays a schematic representation of the rate-equation model. Carriers directly

injected in the WL are then captured by the QD excited state where they can relax towards the

ground state. The thermal escape towards higher energy levels is also considered, and radiative

recombination can take place from both GS and ES. The equations for the time-and

CH019-I046325.indd 588CH019-I046325.indd 588 6/27/2008 5:21:36 PM6/27/2008 5:21:36 PM