Helms L.L. Potential Theory

Подождите немного. Документ загружается.

11.4 Superfunctions for Elliptic Operators 417

Since

lim sup

y→x,y∈Ω

−u(y)=− lim inf

y→x,y∈Ω

u(y) ≤−h(x),

−u ∈ L(−f,−g, −h) whenever u ∈ U(f,g,h).

Recall the standing assumption that |β(x)| =1forallx ∈ Σ.Partof

the hypotheses of the following two lemmas is an exception to the standard

requirement that f and g be ﬁxed functions. The following theorem was

proved for ﬁxed f and g by Lieberman [39] assuming that γ ≤−γ

< 0.

Theorem 11.4.10 If Ω is a bounded open subset of R

,Σ is a relatively

open subset of ∂Ω, ν has a continuous extension to Σ

−

,β · ν ≥ >0 on

Σ,c ≤ 0 on Ω,γ ≤ 0 on Σ,F⊂C

(Ω) with sup

f∈F

f

0,Ω

≤ M<+∞,

and G⊂C

(Σ) with sup

g∈G

g

0,Σ

≤ N<+∞,then

f∈F,g∈G



U(f,g) ∩ C

(Ω

−

) ∩ C

(Ω ∪ Σ)



= ∅

and

f∈F,g∈G



L(f,g) ∩ C

(Ω

−

) ∩ C

(Ω ∪ Σ)



= ∅;

moreover, if Σ = ∂Ω and h ∈ C

(∂Ω ∼ Σ), then the same conclusions are

valid for U(f,g,h) and L(f,g,h).

Proof: For each x ∈ Σ

−

,letV (x) be a neighborhood of x such that

Osc

V (x)∩Σ

ν ≤ (1 − ρ)/2where0<ρ<1, Since Σ

−

is compact, there

are points x

,...,x

∈ Σ

−

such that Σ

−

⊂∪

i=1

V (x

). Let {ψ

}

i=1

be a

partition of unity; that is, ψ

∈ C

∞

(V (x

)), 0 ≤ ψ

≤ 1, and



i=1

(x)=1

for all x ∈ Σ

−

.Fori =1,...,p,letM

u(x)=ψ

(x)Mu(x),x ∈ Σ,not-

ing that M

u(x)=0ifx ∈ supp ψ

, the support of ψ

.Notealsothat

Mu(x)=



i=1

u(x),x ∈ Σ.Foreachi =1,...,p,letx

(i)

be a ﬁxed

point V (x

) ∩Σ

−

and let a

(i)

= ν(x

(i)

). Note that |a

(i)

| =1sinceν is a unit

vector. For x ∈ supp ψ

∩ Σ,



<≤ β(x) · ν(x

(i)

)+β(x) · (ν(x) − ν(x

(i)

) ≤ β(x) · a

(i)

+(1− ρ)/2

from which it follows that β(x) · a

(i)

≥ ρ/2. Suppose now that supp ψ

∩

supp ψ

∩ Σ = ∅ for j = i. For any x ∈ supp ψ

∩ Σ and z

∈ supp ψ

∩

supp ψ

∩ Σ

 ≤ β(x) · ν(x

(j)

)+β(x) ·



ν(x) − ν(z

)+ν(z

) − ν(x

)



≤ β(x) · a

(j)

+(1− ρ)

from which it follows that β(x) · a

(j)

≥ ρ.Fori =1,...,p and λ>0, let

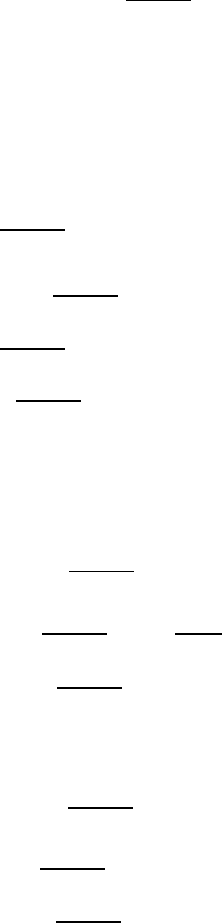
418 11 Oblique Derivative Problem

(x)=

M + N



− e

λa

(i)

·x



,x∈ Ω,

and let v(x)=



i=1

(x),x ∈ Ω.Letλ>0 be any real number satisfying

the conditions |x|≤λ for all x ∈ Ω, −(λρ/2) + |γ|

< −1, and

−mλ

+ λ|b|

+ |c|

< −1,i=1,...,p.

Then

(x)=

M + N

⎛

⎝

−λ



j,k=1

(x)a

(i)

− λ



j=1

(x)a

(i)

− c(x)

⎞

⎠

λa

(i)

·x

+ c(x)

M + N

≤

M + N



−λ

m + λ|b|

+ |c|



λa

(i)

·x

≤−

M + N

−λ

Therefore, L





≤−(M + N)onΩ. Simultaneously for all f ∈F,





≤−M ≤−f 

≤ f on Ω.Forx ∈ supp ψ

,bychoiceofλ

(x)=−λ

M + N





k=1

(x)β

(x)a

(i)



λa

(i)

·x

+ ψ

(x)γ(x)v

(x)

≤

M + N

(x)



−λρ

+ |γ|



λa

(i)

·x

≤−

M + N

(x)e

−λ

For j = i and x ∈ supp ψ

(x)=−λ

M + N





k=1

(x)β

(x)a

(j)



λa

(j)

·x

+ ψ

(x)γ(x)v

(x)

≤

M + N

(x)(−λρ + |γ|

λa

(j)

·x

≤−

M + N

(x)e

−λ

Summing over j =1,...,p,M

v(x) ≤−(M + N)ψ

(x)e

−λ

and by summing

over i =1,...,p,Mv(x) ≤−(M + N)e

−λ

.Thus,M(ve

) ≤−(M + N ) ≤

−N ≤−g

≤ g simultaneously for all g ∈G.Since|x|≤λ,eachv

≥ 0

11.4 Superfunctions for Elliptic Operators 419

on Ω and so w = ve

≥ 0onΩ.Thusw is a superfunction simultaneously

for all f ∈F,g ∈G.IfΣ = ∂Ω and h ∈ C

(∂Ω ∼ Σ), then w + h

a superfunction simultaneously for all f ∈F,g ∈Gand w + h

≥ h on

∂Ω ∼ Σ.

Lemma 11.4.11 Under the conditions of the preceding theorem, if u ∈

(Ω

−

) ∩ C

(Ω ∪ Σ) satisﬁes the equations Lu = f on Ω for f ∈ C

(Ω) ∩

(2+b)

(Ω), Mu = g for g ∈ C

(Σ) ∩H

(1+b)

1+α

(Σ) and u = h on ∂Ω ∼ Σ,when

nonempty, for h ∈ C

(∂Ω ∼ Σ),then

u

0,Ω

≤



C(f 

+ g

) if Σ = ∂Ω

C(f 

+ g

+ h

) if Σ = ∂Ω

where C = C(β

,d(Ω).

Proof: Consider the case ∂Ω ∼ Σ = ∅ and the function v = w + h

≥ 0of

the preceding proof for which Lv ≤ f on Ω, Mv ≤ g on Σ,andv ≥ h

on ∂Ω ∼ Σ. Letting u

∗

= u − v, Lu

∗

≥ 0onΩ,Mu

∗

≥ 0onΣ,and

∗

≤ 0on∂Ω ∼ Σ. By the Strong Maximum Principle, Corollary 11.2.2,

∗

≤ 0onΩ;thatis,u ≤ v = w + h

.Sincew ≤ C(f 

+ g

)on

Ω,u ≤ C(f

+ g

+ h

). As the same result applies to −u, −f, −g and

−h, the inequality holds for −u and for |u|. Taking the supremum over Ω,

u

≤ C(f 

+ g

+ h

The following theorem was proved by Lieberman [39] assuming that γ ≤

−γ

< 0forsomeconstantγ

Theorem 11.4.12 If Σ = ∂Ω,β·ν ≥ >0 for some >0, and either c<0

on Ω or γ<0 on Σ,thenu =inf{v; v ∈ U(f,g)}∈C

(Ω)∩C

(Ω∪Σ), Lu =

f on Ω,andMu = g on Σ.

Proof: Consider any v

∈ U(f,g)andv

−

∈ L(f,g), the two sets being

nonempty by Theorem 11.4.10. Since v

∈ C

(Ω

−

)andu ≤ v

,uis bounded

above. Since v

−

≤ v for all v ∈ U(f,g)by(iii) of Lemma 11.4.5, u is bounded

below and therefore bounded on Ω.Consideranyy ∈ Ω ∪ Σ and any U ∈

A(y). By Choquet’s lemma, Lemma 2.2.8, there is a sequence { v

} in U(f,g)

with the property that if w is a l.s.c. function on U ∩ Ω satisfying w ≤

inf {v

; j ≥ 1} on U ∩ Ω,thenw ≤ u on U ∩ Ω. Since the minimum of two

functions in U(f,g) is again in U(f,g) by Lemma 11.4.5, it can be assumed

that the sequence {v

} is decreasing by replacing v

by min (v

,...,v

), if

necessary. For each j ≥ 1, let w

=(v

)

U∩Ω

, the lowering of v

over U ∩ Ω.

The sequence {w

} is also decreasing by Lemma 11.4.5. Note that w

LS(y, U,v

)onU ∩ Ω implies that Lw

= f on U ∩ Ω,Mw

= g on U ∩ Σ,

and that w

∈ C

(cl(U ∩Ω) ∩C

(U ∩(Ω ∪Σ)) according to Theorem 11.3.7.

If v

−

is any element of L(f,g), then v

≥ w

≥ v

−

on Ω for all j ≥ 1

and therefore w



0,∂(U∩Ω)∼(U∩Σ)

≤ max (v



0,Ω

, v

−



0,Ω

) < +∞ for all

j ≥ 1. It follows from Lemma 11.4.8 that there is a subsequence {w

} of

420 11 Oblique Derivative Problem

the {w

} sequence that converges uniformly on U ∩ (Ω ∪ Σ) to a function

w ∈ C

(cl(U ∩ Ω)) ∩C

(U ∩ (Ω ∪ Σ)) with Lw = f on U ∩ Ω and Mw = g

on U ∩Σ.Sincew ≤ inf {v

; j ≥ 1} on U ∩ Ω, w ≤ u on U ∩Ω and therefore

w = u on U ∩Ω.Thus,u ∈ C

(cl(U ∩Ω))∩C

(U ∩(Ω∪Σ)), Lu = f on U ∩Ω,

and Mu = g on U ∩Σ.Sincey and U are arbitrary, u ∈ C

(Ω ∪Σ), Lu = f

on Ω,andMu = g on Σ.

The following theorem is proved in the same way by using Lemma 11.4.7

in place of Lemma 11.4.5.

Theorem 11.4.13 If Σ = ∂Ω,β · ν ≥ >0 for some >0,c ≤ 0

on Ω, γ ≤ 0 on Σ,andν has a continuous extension to Σ

−

,thenu =

inf {v; v ∈ U(f,g,h)}∈C

(Ω)∩C

(Ω∪Σ), Lu = f on Ω,andMu = g on Σ.

As in Section 9.7, the function u =sup{w; w ∈ L(f,g,h)} will be called

the Perron subsolution and the corresponding v =inf{w : w ∈ U(f,g,h)}

the Perron supersolution. These two functions will be denoted by H

−

f,g,h

and H

f,g,h

, respectively. If H

f,g,h

= H

−

f,g,h

,thenh is said to be (L, M)-

resolutive and the common value is denoted by H

f,g,h

.Ifitcanbeshown

that lim

y→x,y∈Ω

f,g,h

(y)=h(x) for all x ∈ ∂Ω ∼ Σ, then it would follow

from Theorems 11.4.12, 11.4.13, and the strong maximum principle, Corol-

lary 11.3.6, that H

f,g,h

= H

−

f,g,h

on Ω and that h is (L, M)-resolutive. As in

the classical case, it is a question of ﬁnding conditions under which points

of ∂Ω ∼ Σ are regular boundary points. As was the case in Section 9.7, ap-

proximate barriers will be used to answer this question. As before, a region

Ω ⊂ R

will be called (L, M)-regular if lim

y→x,y∈Ω

f,g,h

(y)=h(x) for all

x ∈ ∂Ω ∼ Σ and all h ∈ C

(∂Ω ∼ Σ).

11.5 Regularity of Boundary Points

It was shown in Theorem 2.6.29 that the existence of a cone C ⊂∼ Ω with

vertex at x ∈ ∂Ω implies that x is a regular boundary point for the Dirichlet

problem u =0onΩ and u = g on ∂Ω. More generally, it was shown in

Theorem 9.8.8 that the existence of such a cone implies that x is a regular

boundary point for the elliptic boundary value problem Lu = f on Ω and

u = g on ∂Ω. The boundary behavior of a solution to the oblique derivative

problem Lu = f on Ω, Mu = g on Σ,andu = h on ∂Ω ∼ Σ is more

complicated. The behavior of u at points x ∈ Σ is determined by the existence

theorems, Theorems 11.4.12 and 11.4.13, which assert that u ∈ C

(Ω ∪ Σ)

and Mu = g on Σ.Forpointsx ∈ ∂Ω ∼ Σ, it is necessary to distinguish two

cases:

(i) x ∈ ∂Ω ∼ Σ,d(x, Σ) > 0

(ii) x ∈ ∂Ω ∼ Σ,d(x, Σ)=0.

11.5 Regularity of Boundary Points 421

The existence of a cone C as described above suﬃces to show in the ﬁrst case

that x is a regular boundary point. Only a minor modiﬁcation of the proof of

Lemma 9.8.8 is needed to prove this assertion, namely, by replacing M therein

by M + N. To show that x ∈ ∂Ω ∼ Σ is a regular boundary point when

d(x, Σ) = 0 requires the assumption of a wedge in ∼ Ω rather than a cone

and the construction of an approximate barrier as deﬁned in Deﬁnition 9.8.1.

The imposition of a wedge condition at such boundary points was introduced

by Lieberman in [40].

In addition to the assumption of the preceding section that |β(x)| =1for

all x ∈ Σ, it will be assumed that ν and the β

,i=1,...,n, have continuous

extensions to Σ

−

.Let(y

,...,y

)=(y



) be a local coordinate system

at x

chosen so that ν(x

) is in the direction of the y

-axis. By means of a

translation followed by a rotation of axes, the x and y coordinate systems

are related by an equation y =(x − x

)O where O is an orthogonal matrix.

Letting

ν(y)=ν(x)and

β(y)=β(x), the condition ν(x) · β(x) ≥ δ>0

on Σ carries over to the condition ˜ν(y) ·

β(y) ≥ δ>0 since an orthogonal

transformation preserves inner products. In particular,

0 <δ≤ ν(x

) · β(x

)=˜ν(0) ·

β(0) =

(11.23)

since

ν(0) = (0, 1, 0,...,0) in the y-coordinate system. Under the transforma-

tion from the x-coordinate system to the y-coordinate system, the operator

L is transformed as in Equation (9.33). It will be assumed that the operator

L is deﬁned on a neighborhood of Ω

−

in the remainder of this chapter.

Let (r, θ) denote polar coordinates in the y

-plane so that

= r cos θ, y

= r sin θ.

Also let W(θ

,θ

)denoteawedgeinR

that is cylindrical in the y



-direction

and deﬁned by the equation

W (θ

,θ

)={y : θ

<θ<θ

};

if θ

> 0,W(−θ

,θ

) will be denoted by W (θ

). The function f(θ)inthe

following lemma was taken from [40].

Lemma 11.5.1 If (i) x

∈ ∂Ω ∼ Σ with d(x

,Σ)=0, (ii) there is a neigh-

borhood V of x

and a wedge W (θ

,θ

) ⊂∼ Ω,withθ

−θ

< 2π, (iii) there

is a δ>0 such that ν(x) · β(x) ≥ δ>0 for all x ∈ Σ, and (iv) there is a

wedge W (ψ

) such that Σ ∩ V ⊂ W (ψ

) where 0 <ψ

< arccos (1 − δ

/2),

then there are constants λ

> 0,r

> 0,andafunctionf ∈ C

([0, 2π]) such

that

L(r

f(θ))

λ−2

≤−1, 0 < |λ| <λ

,r < r

,θ

<θ<θ

(11.24)

422 11 Oblique Derivative Problem

and

M(r

f(θ))

λ−1

≤−1, 0 < |λ| <λ

,r < r

, |θ| <ψ

. (11.25)

Proof: Itcanbeassumedthatr ≤ 1andthatλ

< 1. Consider the function

f(θ)=2e

4πq

− pe

qθ

, 0 ≤ θ ≤ 2π,

where

p = e

−qθ

,q=4|˜a

+1,

and ˜a

is the coeﬃcient in Equation (9.33). Note that f (θ) >e

4πq

for 0 <

θ<2π. Assuming for the time being that m = 1 and using the Miller

representation, Theorem 9.8.5, with n =2

L(r

f(θ)) ≤ r

λ−2



˜a

λ(λ − 1)f +2˜a

(λ − 1)f

+˜a

θθ

+ λf )



+ r

λ−1



λf +

+˜crf



Since ˜a

≥ m =1,

lim sup

λ→0

λ−2



L(r

f(θ)) − r

λ−1

(

+˜crf)



≤−2˜a

+ f

θθ

≤ q(−2|˜a

− 1)

uniformly for r<1andθ

<θ<θ

. Thus, there are constants c



> 0and



> 0 such that

L(r

f(θ)) ≤−c



λ−2

+ r

λ−1

(

+˜crf)

for |λ| <λ



uniformly for r<1,θ

<θ<θ

. Letting c

= |

+|˜c|

|f|

L(r

f(θ)) ≤−c



λ−2

+ c

λ−1

, |λ| <λ



uniformly for r<1,θ

<θ<θ

. There is therefore a constant r



< 1such

that

L(r

f(θ))

λ−2

≤−c

, |λ| <λ



,r < r



,θ

<θ<θ

, (11.26)

where c

= c



/2. Now consider M(r

f(θ)). By Equations (9.29) and (9.30),

M(r

f(θ)) = r

λ−1



(

(y)cosθ +

(y)sinθ)λf

+(−

(y)sinθ +

(y)cosθ)f

+˜γrf



(11.27)

Let ω(θ)=(cosθ, sin θ, 0,...,0) be a point of W(ψ

). Since

(cos (θ + π/2), sin (θ + π/2)) = (−sin θ, cos θ),

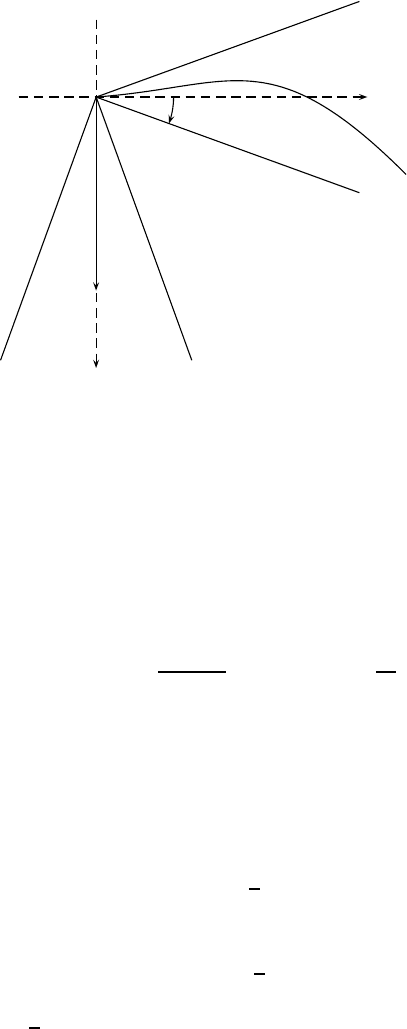
11.5 Regularity of Boundary Points 423

ν(0)

• !(µ)

•



(µ)

W(Ã

)

Fig. 11.1 y

-section of Σ

the point ω



(θ)=(−sin θ, cos θ, 0,...,0) is in the wedge obtained by rotating

W (ψ

) about its edge through π/2 radians as depicted in Figure 11.1. The

distance from ˜ν(0) to ω



(θ)isamaximumwhenθ = ψ

so that

|˜ν(0) −ω



(θ)|

≤ 2(1 − cos ψ

Choose 0 <<δsuch that

< arccos



1 −

(δ − )



< arccos



1 −



Using the fact that ˜ν is continuous at 0, there is a constant 0 <r



< 1such

that

|˜ν(y) − ˜ν(0)| <, r<r



Since

0 <δ≤

β(y) · ˜ν(y)=

β(y) ·(˜ν(y) − ˜ν(0)) +

β(y) ·(˜ν(0) −ω



(θ)) +

β(y) ·ω



(θ),

β(y) · ω



(θ) ≥ (δ − ) −

√

2(1 − cos ψ

)

1/2

Returning to Equation (11.27), if |θ| <ψ

and r<r



,then

M(r

f(θ)) ≤ r

λ−1



λf +((δ − ) −

√

2(1 − cos ψ

)

1/2

+˜γrf



Since (δ − ) −

√

2(1 − cos ψ

)

1/2

> 0andf

≤−pq exp (−qψ

)for|θ| <ψ

there are positive numbers c

,andc

such that

424 11 Oblique Derivative Problem

M(r

f(θ)) ≤ r

λ−1

λ − c

+ c

r).

By further reducing r



,thereisaλ



> 0 and a positive constant c

such that

M(r

f(θ)) ≤−r

λ−1

,r<r



,λ< λ



. (11.28)

The Inequalities (11.24) and (11.25) follow from the Inequalities (11.26) and

(11.28) by replacing f/min (c

)byf, assuming that m = 1. Apply this

result to the operators (1/m)L and (1/m)M and then replace f/m by f to

get the general result.

Deﬁnition 11.5.2 If Ω and Σ satisfy the hypotheses of Theorem 11.5.1 at

∈ ∂Ω ∼ Σ = ∅,thenΩ and Σ are said to satisfy wedge conditions at x

Theorem 11.5.3 If (i) Ω is a bounded open subset of R

, (ii) Σ is a rela-

tively open subset of ∂Ω of class C

2+α

with Σ = ∂Ω, (iii) β · ν ≥ >0

on Σ for some 0 <<1, (iv) there is an exterior cone at each point

x ∈ ∂Ω ∼ Σ with d(x, Σ) > 0, (v) wedge conditions are satisﬁed at each

point x ∈ ∂Ω ∼ Σ with d(x, Σ)=0,(vi)F⊂C

(Ω) ∩ H

(2+b)

(Ω) with

sup

f∈F

f

0,Ω

≤ M<+∞,g ∈ C

(Σ) ∩ H

(1+b)

1+α

(Σ),andh ∈ C

(∂Ω ∼ Σ),

then for each x

∈ ∂Ω ∼ Σ

lim

y→x

,y∈Ω

f,g,h

(y)=h(x

) uniformly for f ∈F.

Proof: The proof assuming (iv) is the same as the proof of Lemma 9.8.8.

Consider any x

∈ ∂Ω ∼ Σ satisfying (v). As in Lemma 9.8.2, it suﬃces to

prove there is an approximate barrier {w

} at x

.Considerany

φ ∈

f∈F



A(f,g,h) ∩ C

(Ω

−

) ∩ C

(Ω ∪ Σ)



which is nonempty by Theorem 11.4.10. Note that φ is a supersolution simul-

taneously for all f ∈F. It can be assumed that h(x

)+φ is a supersolution,

that h(x

)+φ ≥ h on ∂Ω ∼ Σ,andthatφ(x

) ≥ 0, for if not, replace φ by

2|h|

0,∂Ω∼Σ

+|φ|

0,Ω

+φ since h(x

)+2|h|

0,∂Ω∼Σ

+|φ|

0,Ω

+φ is a superfunction

by Lemma 11.4.4. For each j ≥ 1, let U

be a neighborhood of x

such that

(i) h(x) <h(x

,x∈ U

∩ (∂Ω ∼ Σ),

(ii) L(r

f(θ)) < −1onU

∩ Ω,

(iii) M(r

f(θ)) < −1onU

∩ Σ

where w = r

f(θ) is the function of the preceding lemma for some ﬁxed λ

satisfying |λ| <λ

< 1. For each j ≥ 1, choose k(j) ≥ 1sothat

11.5 Regularity of Boundary Points 425

(iv) k(j) > max



M + |c|



h(x



g −M(h(x

)



0,Σ

≥ max





f − L(h(x

)



0,Ω



g −M(h(x

)



0,Σ

for all f ∈F.

(v)

+ k(j)w>φon ∂U

∩Ω.

The latter condition is possible, since f(θ) ≥ e

4πq

on [0, 2π],asnotedinthe

proof of the preceding lemma. On U

∩ Ω,



h(x

+ k(j)w



≤ L



h(x



− k(j)

≤ L



h(x



−



f − L



h(x





≤ L



h(x



+ f − L



h(x



= f.

Similarly, on U

∩ Σ,



h(x

+ k(j)w



≤ g.

It follows that the function h(x

)+1/j +k(j)w is a superfunction for f and g

on U

∩ Ω by Lemma 11.4.4. Now let



min {h(x

+ k(j)w, h(x

)+φ} on U

∩ Ω

h(x

)+φ on Ω ∼ U

By (i) of Lemma 11.4.5, w

is a superfunction on U

∩ Ω and on Ω ∼ cl U

since h(x

)+φ is a superfunction on Ω.Consideranypointz ∈ ∂U

∩Ω.Since

h(x

)+1/j+k(j)w(z) >h(x

)+φ(z), there is a neighborhood V ∈A(z)such

that h(x

)+1/j + k(j)w>h(x

)+φ on cl V .ConsideranyU ⊂ V, U ∈A(z).

Then

(z)=h(x

)+φ(z) ≥ L(z, U, h(x

)+φ)=L(z,U, w

)

which shows that w

is a superfunction on Ω.On(∂Ω ∼ Σ) ∩ U

,h(x

1/j + k(j)w ≥ h(x

)+1/j > h and h(x

)+φ ≥ h so that w

≥ h on

(∂Ω ∼ Σ) ∩ U

.On(∂Ω ∼ Σ) ∼ U

= h(x

)+φ ≥ h. Then, w

≥ h on

∂Ω ∼ Σ and w

is a supersolution on Ω.Atx

)=min{h(x

,h(x

)+φ(x

)}

426 11 Oblique Derivative Problem

and

lim

j→∞

)=min{h(x

),h(x

)+φ(x

)} = h(x

The convergence is uniform for f ∈Fsince w

depends only on M.This

completes the proof that {w

} is an upper approximate barrier at x

.Only

minor modiﬁcations of the above arguments are needed to construct a lower

approximate barrier {w

−

} at x

Theorem 11.5.4 Under the conditions of the preceding theorem, the oblique

derivative problem

Lu = f on Ω, Mu = g on Σ, and u = h on ∂Ω ∼ Σ

has a unique solution u ∈ C

(Ω

−

) ∩ C

(Ω ∪ Σ).

Proof: It follows from Theorem 11.4.13 that H

f,g,h

∈ C

(Ω ∪ Σ)and

that lim

y→x

,y∈Ω

f,g,h

= h(x

) for all x

∈ ∂Ω ∼ Σ as in Lemma 9.8.2.

By the remarks at the end of Section 11.4, H

f,g,h

= H

−

f,g,h

so that h is

(L, M)-resolutive. Letting u = H

f,g,h

, Lu = f on Ω,Mu = g on Σ,and

u = h on ∂Ω ∼ Σ. Uniqueness follows from the strong maximum principle,

Corollary 11.3.6.

Example 11.5.5 If Ω is a convex polytope in R

and Σ is a union of non-

contiguous faces and β is nontangential, then it is easily seen that Ω satisﬁes

the wedge conditions at all points x of ∂Ω ∼ Σ with d(x, Σ)=0andthath

is (L, M)-resolutive for each f,g as described above.

In the Σ = ∂Ω case, the theorem corresponding to this theorem is

Theorem 11.4.12 which asserts the existence of a solution to the equation

Lu = f subject to the boundary condition Mu = g assuming c<0orγ<0

with uniqueness following in this case from Corollary 11.3.6. In the c =0and

γ = 0 case, some additional criteria is required for uniqueness.

Both Theorems 11.4.11 and 11.4.12 provide for the existence of a solution

to the oblique derivative boundary value problem in C

(Ω) ∪ C

(Ω ∪ Σ).

In order to show that the solution has additional properties, a global ver-

sion of Inequality (11.4) is needed. Moreover, the hypotheses that f ∈

(Ω) ∩ H

(2+b)

(Ω)andg ∈ C

(Σ) ∩ H

(1+b)

1+α

(Σ) call for modiﬁcations of

the corresponding norms.

Deﬁnition 11.5.6 If f ∈ C

(Ω) ∩ H

(b)

k+α

(Ω),k+ b + α ≥ 0, let

|f|

∗(b)

k+α,Ω

=max(f

0,Ω

, |f |

(b)

k+α,Ω

)

and for g ∈ C

(Σ) ∩ H

(b)

k+α

(Σ), let

|g|

∗(b)

k+α,Σ

=max(g

0,Σ

, |g|

(b)

k+α,Σ