Hahn Th. (ed.) International tables for crystallography. Vol. A. Space-group symmetry
Подождите немного. Документ загружается.

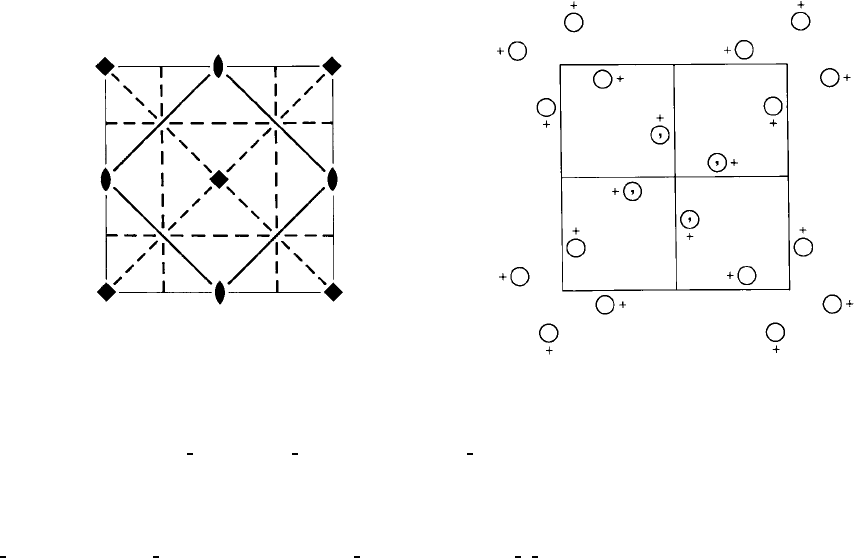
P4bm C
2
4v
4mm Tetragonal
No. 100 P4bm
Patterson symmetry P4/mmm
Origin on 41g
Asymmetric unit 0 ≤ x ≤
1
2
;0≤ y ≤
1
2
;0≤ z ≤ 1; y ≤
1
2
− x
Symmetry operations
(1) 1 (2) 2 0,0,z (3) 4
+
0,0,z (4) 4
−
0,0,z
(5) ax,
1
4
,z (6) b
1
4
,y,z (7) mx+
1
2
, ¯x, z (8) g(
1
2
,
1
2
,0) x,x,z
384
International Tables for Crystallography (2006). Vol. A, Space group 100, pp. 384–385.
Copyright © 2006 International Union of Crystallography

CONTINUED No. 100 P4bm
Generators selected (1); t(1, 0,0); t(0,1,0); t(0,0,1); (2); (3); (5)
Positions
Multiplicity,
Wyckoff letter,
Site symmetry
Coordinates Reflection conditions
General:
8 d 1(1)x,y,z (2) ¯x, ¯y,z (3) ¯y,x,z (4) y, ¯x,z
(5) x +
1
2
, ¯y +
1
2
,z (6) ¯x +
1
2
,y +
1
2
,z (7) ¯y +
1
2
, ¯x +
1
2
,z (8) y +
1
2
,x +
1
2
,z
0kl : k = 2n
h00 : h = 2n
Special: as above, plus
4 c ..mx,x +
1
2
,z ¯x, ¯x +
1
2
,z ¯x +
1
2
,x,zx+
1
2
, ¯x, z no extra conditions
2 b 2 . mm
1
2
,0, z 0,
1
2
,zhkl: h + k = 2n
2 a 4 .. 0,0,z
1
2
,
1
2
,zhkl: h + k = 2n
Symmetry of special projections
Along [001] p4gm
a
= ab
= b
Origin at 0,0,z
Along [100] p1m1
a
=
1
2
bb
= c
Origin at x, 0,0
Along [110] p1m1
a
=
1
2
(−a + b) b
= c
Origin at x,x , 0
Maximal non-isomorphic subgroups
I
[2] P411 (P4, 75) 1; 2; 3; 4
[2] P21m (Cmm2, 35) 1; 2; 7; 8
[2] P2b1(Pba2, 32) 1; 2; 5; 6
IIa none
IIb [2] P4
2
bc (c
= 2c) (106); [2] P4nc (c
= 2c) (104); [2] P4
2
nm (c
= 2c) (102)
Maximal isomorphic subgroups of lowest index
IIc
[2] P4bm (c
= 2c) (100); [9] P4bm (a
= 3a,b
= 3b) (100)
Minimal non-isomorphic supergroups
I
[2] P4/nbm(125); [2] P4/mbm(127)
II [2] C 4mm (P4mm, 99); [2] I 4cm (108)
385
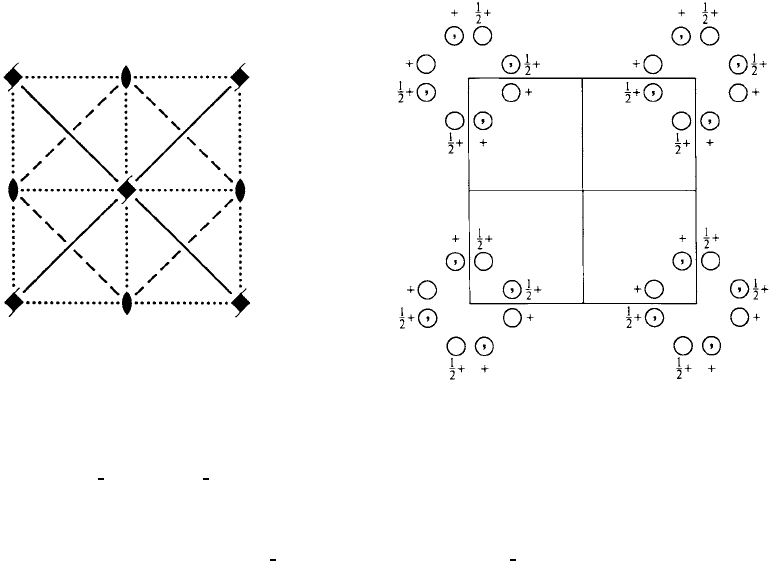
P4
2
cm C
3
4v
4mm Tetragonal
No. 101 P4
2
cm Patterson symmetry P4/mmm
Origin on 2mm on 4
2
cm
Asymmetric unit 0 ≤ x ≤
1
2
;0≤ y ≤
1
2
;0≤ z ≤ 1; x ≤ y
Symmetry operations
(1) 1 (2) 2 0, 0,z (3) 4
+
(0,0,
1
2
) 0,0,z (4) 4
−
(0,0,
1
2
) 0,0, z
(5) cx,0,z (6) c 0,y,z (7) mx, ¯x,z (8) mx,x, z
386
International Tables for Crystallography (2006). Vol. A, Space group 101, pp. 386–387.
Copyright © 2006 International Union of Crystallography
CONTINUED No. 101 P4
2
cm
Generators selected (1); t(1, 0,0); t(0,1,0); t(0,0,1); (2); (3); (5)
Positions
Multiplicity,
Wyckoff letter,
Site symmetry
Coordinates Reflection conditions
General:
8 e 1(1)x,y,z (2) ¯x, ¯y,z (3) ¯y,x,z +
1
2
(4) y, ¯x,z +
1
2
(5) x, ¯y,z +
1
2
(6) ¯x,y, z +
1
2
(7) ¯y, ¯x,z (8) y,x,z
0kl : l = 2n
00l : l = 2n
Special: as above, plus
4 d ..mx,x,z ¯x, ¯x,z ¯x,x,z +
1
2
x, ¯x,z +
1
2
no extra conditions
4 c 2 .. 0,
1
2
,z
1
2
,0, z +
1
2
0,
1
2
,z +
1
2
1
2
,0, zhkl: h + k,l = 2n
2 b 2 . mm
1
2
,
1
2
,z
1
2
,
1
2
,z +
1
2
hkl : l = 2n
2 a 2 . mm 0,0,z 0,0,z +
1
2
hkl : l = 2n
Symmetry of special projections
Along [001] p4mm
a
= ab
= b
Origin at 0,0,z
Along [100] p1m1
a
= bb
=
1
2
c
Origin at x,0,0
Along [110] p1m1
a
=
1
2
(−a + b) b
= c
Origin at x,x , 0
Maximal non-isomorphic subgroups
I
[2] P4
2
11(P4
2
, 77) 1; 2; 3; 4
[2] P21m (Cmm2, 35) 1; 2; 7; 8
[2] P2c1(Pcc2, 27) 1; 2; 5; 6
IIa none
IIb [2] C 4
2
cd(a
= 2a,b
= 2b)(P4
2
bc, 106); [2] C4
2
cm (a
= 2a,b
= 2b)(P4
2
mc, 105)
Maximal isomorphic subgroups of lowest index
IIc
[3] P4
2
cm (c
= 3c) (101); [9] P4
2
cm(a
= 3a,b
= 3b) (101)
Minimal non-isomorphic supergroups
I
[2] P4
2
/mcm (132); [2] P4
2
/ncm(138)
II [2] C 4
2
cm(P4
2
mc, 105); [2] I 4cm(108); [2] P4mm(c
=
1
2
c) (99)
387
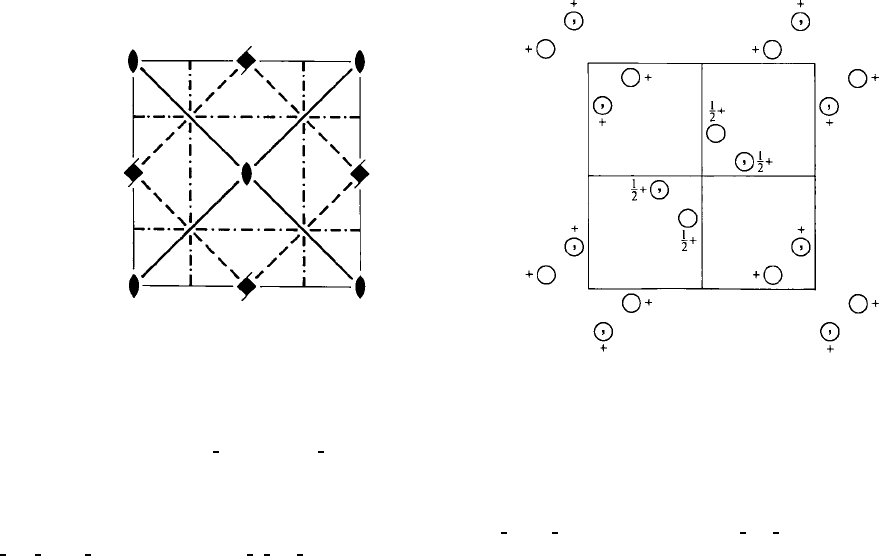
P4
2
nm C
4
4v
4mm Tetragonal
No. 102 P4
2
nm Patterson symmetry P4/mmm
Origin on 2mm on 21m
Asymmetric unit 0 ≤ x ≤
1
2
;0≤ y ≤
1
2
;0≤ z ≤ 1; x ≤ y
Symmetry operations
(1) 1 (2) 2 0, 0,z (3) 4
+
(0,0,
1
2
) 0,
1
2
,z (4) 4
−
(0,0,
1
2
)
1
2
,0, z
(5) n(
1
2
,0,
1
2
) x,
1
4
,z (6) n(0,
1
2
,
1
2
)
1
4
,y,z (7) mx, ¯x,z (8) mx,x,z
388
International Tables for Crystallography (2006). Vol. A, Space group 102, pp. 388–389.
Copyright © 2006 International Union of Crystallography

CONTINUED No. 102 P4
2
nm
Generators selected (1); t(1, 0,0); t(0,1,0); t(0,0,1); (2); (3); (5)
Positions
Multiplicity,
Wyckoff letter,
Site symmetry
Coordinates Reflection conditions
General:
8 d 1(1)x,y,z (2) ¯x, ¯y,z (3) ¯y +
1
2
,x +
1
2
,z +
1
2
(4) y +
1
2
, ¯x +
1
2
,z +
1
2
(5) x +
1
2
, ¯y +
1
2
,z +
1
2
(6) ¯x+
1
2
,y +
1
2
,z +
1
2
(7) ¯y, ¯x, z (8) y,x,z
0kl : k + l = 2 n
00l : l = 2n
h00 : h = 2n
Special: as above, plus
4 c ..mx,x,z ¯x, ¯x,z ¯x +
1
2
,x +
1
2
,z +
1
2
x +
1
2
, ¯x +
1
2
,z +
1
2
no extra conditions
4 b 2 .. 0,
1
2
,z 0,
1
2
,z +
1
2
1
2
,0, z +
1
2
1
2
,0, zhkl: h + k,l = 2n
2 a 2 . mm 0,0,z
1
2
,
1
2
,z +
1
2
hkl : h + k + l = 2n
Symmetry of special projections
Along [001] p4gm
a
= ab
= b
Origin at 0,
1
2
,z
Along [100] c1m1
a
= bb
= c
Origin at x,0,0
Along [110] p1m1
a
=
1
2
(−a + b) b
= c
Origin at x,x , 0
Maximal non-isomorphic subgroups
I
[2] P4
2
11(P4
2
, 77) 1; 2; 3; 4
[2] P21m (Cmm2, 35) 1; 2; 7; 8
[2] P2n1(Pnn2, 34) 1; 2; 5; 6
IIa none
IIb [2] F 4
1
dc(a
= 2a,b
= 2b,c
= 2c)(I 4
1
cd, 110); [2] F 4
1
dm(a
= 2a,b
= 2b,c
= 2c)(I 4
1
md, 109)
Maximal isomorphic subgroups of lowest index
IIc
[3] P4
2
nm (c
= 3c) (102); [9] P4
2
nm (a
= 3a,b
= 3b) (102)
Minimal non-isomorphic supergroups
I
[2] P4
2
/nnm(134); [2] P4
2
/mnm (136)
II [2] C 4
2
cm(P4
2
mc, 105); [2] I 4mm (107); [2] P4bm (c
=
1
2
c) (100)
389
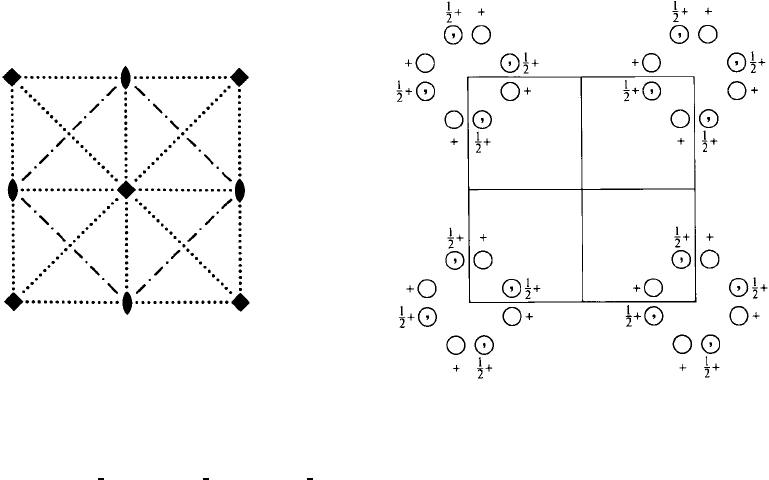
P4cc C
5
4v
4mm Tetragonal
No. 103 P4cc
Patterson symmetry P4/mmm
Origin on 4cc
Asymmetric unit 0 ≤ x ≤
1
2
;0≤ y ≤
1
2
;0≤ z ≤
1
2
Symmetry operations
(1) 1 (2) 2 0, 0,z (3) 4
+
0,0,z (4) 4
−
0,0,z
(5) cx,0,z (6) c 0,y,z (7) cx, ¯x,z (8) cx, x,z
390
International Tables for Crystallography (2006). Vol. A, Space group 103, pp. 390–391.
Copyright © 2006 International Union of Crystallography

CONTINUED No. 103 P4cc
Generators selected (1); t(1, 0,0); t(0,1,0); t(0,0,1); (2); (3); (5)
Positions
Multiplicity,
Wyckoff letter,
Site symmetry
Coordinates Reflection conditions
General:
8 d 1(1)x,y,z (2) ¯x, ¯y,z (3) ¯y,x, z (4) y, ¯x,z
(5) x, ¯y,z +
1
2
(6) ¯x,y, z +
1
2
(7) ¯y, ¯x,z +
1
2
(8) y,x,z +
1
2
0kl : l = 2n
hhl : l = 2n
00l : l = 2n
Special: as above, plus
4 c 2 .. 0,
1
2
,z
1
2
,0, z 0,
1
2
,z +
1
2
1
2
,0, z +
1
2
hkl : h + k,l = 2n
2 b 4 ..
1
2
,
1
2
,z
1
2
,
1
2
,z +
1
2
hkl : l = 2n
2 a 4 .. 0,0,z 0,0,z +
1
2
hkl : l = 2n
Symmetry of special projections
Along [001] p4mm
a
= ab
= b
Origin at 0,0,z
Along [100] p1m1
a
= bb
=
1
2
c
Origin at x,0,0
Along [110] p1m1
a
=
1
2
(−a + b) b
=
1
2
c
Origin at x,x , 0
Maximal non-isomorphic subgroups
I
[2] P411 (P4, 75) 1; 2; 3; 4
[2] P21c (Ccc2, 37) 1; 2; 7; 8
[2] P2c1(Pcc2, 27) 1; 2; 5; 6
IIa none
IIb [2] C 4cd (a
= 2a,b
= 2b)(P4nc, 104)
Maximal isomorphic subgroups of lowest index
IIc
[2] C 4cc (a
= 2a,b
= 2b)(P4cc, 103); [3] P4cc (c
= 3c) (103)
Minimal non-isomorphic supergroups
I
[2] P4/mcc(124); [2] P4/ncc(130)
II [2] I 4cm (108); [2] P4mm (c
=
1
2
c) (99)
391
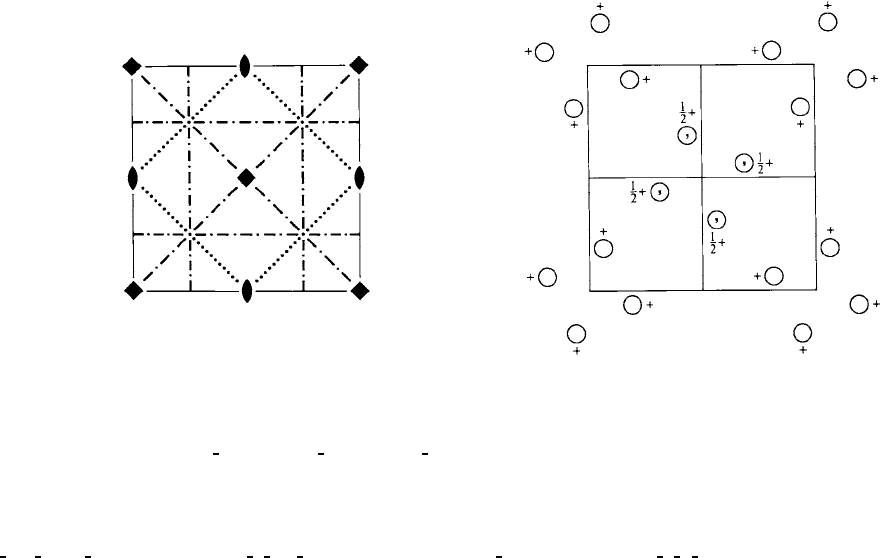
P4nc C
6
4v
4mm Tetragonal
No. 104 P4nc
Patterson symmetry P4/mmm
Origin on 41n
Asymmetric unit 0 ≤ x ≤
1
2
;0≤ y ≤
1
2
;0≤ z ≤
1
2
Symmetry operations
(1) 1 (2) 2 0, 0,z (3) 4
+
0,0,z (4) 4
−
0,0,z
(5) n(
1
2
,0,
1
2
) x,
1
4
,z (6) n(0,
1
2
,
1
2
)
1
4
,y,z (7) cx+
1
2
, ¯x, z (8) n(
1
2
,
1
2
,
1
2
) x,x,z
392
International Tables for Crystallography (2006). Vol. A, Space group 104, pp. 392–393.
Copyright © 2006 International Union of Crystallography

CONTINUED No. 104 P4nc
Generators selected (1); t(1, 0,0); t(0,1,0); t(0,0,1); (2); (3); (5)
Positions
Multiplicity,
Wyckoff letter,
Site symmetry
Coordinates Reflection conditions
General:
8 c 1(1)x,y,z (2) ¯x, ¯y,z (3) ¯y,x,z (4) y, ¯x,z
(5) x +
1
2
, ¯y +
1
2
,z +
1
2
(6) ¯x+
1
2
,y +
1
2
,z +
1
2
(7) ¯y +
1
2
, ¯x +
1
2
,z +
1
2
(8) y +
1
2
,x +
1
2
,z +
1
2
0kl : k + l = 2 n
hhl : l = 2n
00l : l = 2n
h00 : h = 2n
Special: as above, plus
4 b 2 .. 0,
1
2
,z
1
2
,0, z
1
2
,0, z +
1
2
0,
1
2
,z +
1
2
hkl : h + k,l = 2n
2 a 4 .. 0,0,z
1
2
,
1
2
,z +
1
2
hkl : h + k + l = 2n
Symmetry of special projections
Along [001] p4gm
a
= ab
= b
Origin at 0,0,z
Along [100] c1m1
a
= bb
= c
Origin at x, 0,0
Along [110] p1m1
a
=
1
2
(−a + b) b
=
1
2
c
Origin at x,x , 0
Maximal non-isomorphic subgroups
I
[2] P411 (P4, 75) 1; 2; 3; 4
[2] P21c (Ccc2, 37) 1; 2; 7; 8
[2] P2n1(Pnn2, 34) 1; 2; 5; 6
IIa none
IIb none
Maximal isomorphic subgroups of lowest index
IIc
[3] P4nc (c
= 3c) (104); [9] P4nc (a
= 3a,b
= 3b) (104)
Minimal non-isomorphic supergroups
I
[2] P4/nnc(126); [2] P4/mnc(128)
II [2] C 4cc (P4cc, 103); [2] I 4mm (107); [2] P4bm(c
=
1
2
c) (100)
393
