Goldfarb D. Biophysics DeMYSTiFied

Подождите немного. Документ загружается.

92 Biophysics DemystifieD

0.00

0612 18 24 30 36 42 48 54 60 66 72 78 84 90 96

0.10

0.20

0.30

0.40

0.50

0.60

0.70

0.80

0.90

1.00

Percent of Distributions

Cumulative Probability

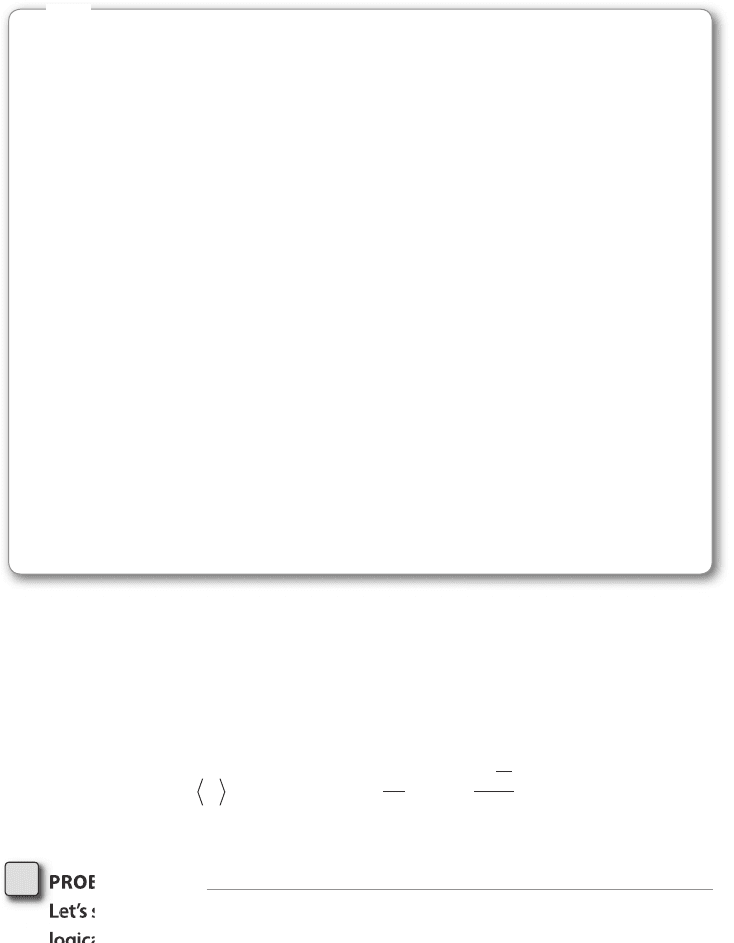
FIgure 5-9 • Cumulative probability versus percent of distributions contributing to that probability for a

system of 40 molecules.

Once we have enough molecules so to safely ignore 99.9% of the distribu-

tions, there is another fact that allows us to ignore even more. The less than

0.1% of distributions that we do not ignore are all very similar to each other.

For example, for this small set of significant distributions, the number of

molecules on any given energy level differs by less than 1% from one distribu-

tion to another. Therefore any one of these distributions can be used as an

approximation for the others with less than 1% error.

As we increase the number of molecules even further, the percentage of

significant distributions becomes so small and the differences among them

so slight that the whole problem becomes simply a matter of finding the

most probable distribution. The most probable distribution, in such cases,

is an extremely close approximation for any distribution of any signifi-

cance. The most probable distribution is called the Boltzmann distribution,

named for Ludwig Boltzmann who first derived a formula for it in the

1860s.

chapter 5 statistical Mechanics 93

Boltzmann Distribution

Finding the most probable distribution is just a matter of finding the distribu-

tion with the largest number of ways of arranging the molecules.

As before, we define a distribution (of molecules among energy levels) by

specifying the number of molecules at each available energy level. If we write

the number of molecules on energy level i as n

, then a distribution is defined

by specifying all n

from n

through

MAX

, where L

MAX

is the highest energy level

available to the system.

If we can come up with a formula for n

for the most probable distribution,

we can use that formula in all of our statistical mechanical calculations, and this

is exactly what Boltzmann did.

One more thing to note about L

MAX

: Unless otherwise stated, we assume that

all energy levels between 1 and L

MAX

are available to the molecules and L

MAX

not just the highest available energy level but is also equal to the total number

of available energy levels.

Once we have any distribution, that is, once we know all of the n

, then

regardless of what that distribution is, the following two relationships apply:

The total number of molecules is equal to the sum, over all of the energy levels,

of the number of molecules on each energy level, as we saw in Eq. (5-2).

∑

MAX

still struggling

Many texts, when discussing statistical mechanics, will speak of the Boltz-

mann distribution as if it is the only distribution found in nature, that is, the

only way that nature distributes energy within a system of particles. you

should keep in mind that other distributions can and do occur. however,

their probability is typically very low and their existence so transitory that

for any realistic and significant number of molecules, the Boltzmann distri-

bution is all that matters. Only in the extreme case of small numbers of mol-

ecules and/or relatively little energy, do we need to consider other

distributions in our calculations.

94 Biophysics DemystifieD

The number of different ways to arrange the molecules and still have

the same distribution is given by Eq. (5-1a). We can rewrite Eq. (5-1a)

using the product symbol (p) meaning take the product of all n

! from

i = 1 to L

MAX

∏

()!

MAX

(5-1b)

Mathematically, finding the most probable distribution amounts to finding

the set of n

that maximizes Eq. (5-1b) for a given value of N and of L

MAX

. We

will skip the somewhat involved mathematics for maximizing Eq. (5-1b). The

interested reader can look up the derivation in most libraries or on the Web.

The end result is that the set of n

that maximizes W is given by

−

(5-3)

where n

is the number of molecules with energy E

(i.e., on energy level i), N

is the total number of molecules, b is a constant that can be determined by

comparison with experimental data, and Z is the sum of all

−

for all energy

levels; that is,

−

∑

MAX

(5-4)

Z is called the partition function. It contains all of the information as to how the

total energy is partitioned among the molecules. Comparison with experimental

thermodynamic data shows the b = 1/kT where T is the absolute temperature

and k is Boltzmann’s constant, equal to 1.3806 × 10

−23

J/K. Thus the classic for-

mula for the Boltzmann distribution of particles among energy levels is

−

(5-5)

Things to remember about the Boltzmann distribution are

1. It is simply the most probable distribution of N molecules across L

MAX

energy levels. We call it the Boltzmann distribution (instead of the most

probable distribution) to honor Ludwig Boltzmann who first derived a

formula for it in the 1860s.

2. For any reasonable number of molecules and amount of energy, the prob-

ability of the Boltzmann distribution overshadows all other distributions.

chapter 5 statistical Mechanics 95

This does not mean that the other distributions do not occur, only that

they occur with such low probability and typically so transiently that for

nearly all intents and purposes calculations based on the Boltzmann dis-

tribution are accurate and precise. We need not worry about other distri-

butions except in the extreme case of a small number of molecules or a

very small amount of energy to distribute.

3. Equation (5-5) assumes that N is at least 60 or so. This is because it

uses Stirling’s approximation for n factorial. However, there are times

when even for N < 60, Boltzmann’s distribution gives a close enough

approximation.

Statistical Mechanical Calculations

Now that we have only a single distribution to worry about, we can use that

distribution to make statistical mechanical calculations for

The fraction of molecules in a particular energy state

•

The average and total energy of the system•

The fraction, or proportion, of molecules on a particular energy level i is the num-

ber of molecules on that energy level divided by the total number of molecules.

−

(5-6)

This is also the probability of finding that any particular molecule has energy

. For example, if 1/5 of the molecules have energy E

and if we could some-

how sample and measure the energy of a single molecule, there would be a

probability of 1/5, or .2 of finding that the molecule we sampled had an energy

of E

. Therefore we sometimes write P

instead of F

−

(5-7)

The point is that the fraction of molecules in a particular state is equivalent

to the probability of finding a molecule in that particular state. This is an impor-

tant point because sometimes we want to know the probability of a particular

biophysical process occurring. The probability of a process occurring is often

related to the probability of a particular energy state occurring.

96 Biophysics DemystifieD

The average energy (per molecule) in the system is the amount of energy at

each energy level times the fraction of molecules at that particular energy level,

summed over all possible energy levels.

EEFE

ii i

===

∑∑∑

−

MAX

(5-8)

PROBLEM 5-4

Let’s say we have a sample containing a very large number of a particular bio-

logical molecule and we would like to model the various conformational states

of this molecule as having three possible energy levels. In our model, the three

energy levels available to our molecule are 4.0 × 10

–20

J, 4.4 × 10

–20

J, and 4.8 ×

–20

J. Assuming the Boltzmann distribution, calculate the fraction of mole-

cules expected to be at each energy level at room temperature (295 K).

PROBLEM

Let’s say we have a sample containing a very large number of a particular bio-

logical molecule and we would like to model the various conformational states

PROBLEM

Let’s say we have a sample containing a very large number of a particular bio-

still struggling?

how can the probability of a biophysical process be related to the probability of a

particular energy state? Let’s take an example. Suppose the body needs more in-

sulin. In order for our cells to make more insulin, the gene that contains instruc-

tions for making insulin needs to be expressed. this means that a certain group of

proteins needs to bind to the Dna at a point on the Dna very near to where the

beginning of that gene is. this in turn may require the Dna to be in a particular

conformation, or to be supercoiled to a certain extent, thus defining a specific

energy level that the Dna must be in for insulin to be made. the probability of

finding the Dna in that state affects the probability of insulin being produced.

Similarly the proteins involved may also each need to be in a particular conforma-

tion in order to bind to the Dna, thus defining a particular energy state for each

protein. the probabilities of each of these states, for the Dna and for the proteins,

affect the probability of insulin being produced. the body adjusts conditions as

needed in order to alter these probabilities: increasing the probability when insu-

lin is needed, and decreasing the probability when not. statistical mechanics can

be used to calculate these probabilities under various conditions and thus help us

to understand the mechanisms involved at a molecular level.

chapter 5 statistical Mechanics 97

SOLUTION

You’ll probably want your calculator for this one. Typically the first thing we

do in statistical mechanics is calculate the partition function. Everything

else we might want to calculate depends on the partition function. The

partition function in our case is given by

 



40 10 44 10

MAX

∑

222



20 20

48 10JJ

kT kT

Since the energy levels are expressed in joules, we express Boltzmann’s constant

in joules: k = 1.3806 × 10

–23

J/K. Then the first term of the partition function is



40 10 40 10

20 20

..JJ

(1.3806 10 J/K

))K()

295

9 8213

5 4282 10

3

Similarly the second term is



44 10

295

10 80

()

(1.3806 10 J/K) K

2 0329 10

3

And the third term is



48 10

295

()

(1.3806 10 J/K) K

11 786

0 76135 10

3

So the total value of the partition function at 295 K is

5.4242 × 10

– 5

+ 2.0329 × 10

– 5

+ 0.76135 × 10

– 5

= 8.2224 × 10

– 5

Notice that all of the units cancel out. The partition function is a dimension-

less quantity.

Now that we have calculated the partition function, the fraction of mol-

ecules at each energy level is given by Eq. (5-6).

40 10



(1.3806 10 J/K

))K

5.4282 10

8.2224 10

()

295

66 0



Similarly,

24 7



2.0329 10

8.2224 10

and

93



0.76135 10

8.2224 10

SOLUTION

You’ll probably want your calculator for this one. Typically the first thing we

do in statistical mechanics is calculate the partition function. Everything

✔

98 Biophysics DemystifieD

PROBLEM 5-5

Given the same model as in Prob. 5-4, (three energy levels available: 4.0 ×

–20

J, 4.4 × 10

–20

J, and 4.8 × 10

–20

J, and the Boltzmann distribution) cal-

culate the fraction of molecules expected to be at each energy level at

body temperature (310 K).

SOLUTION

Everything is the same as in Prob. 5-4, except now the temperature is 310 K

instead of 295 K. The three terms of the partition function are



40 10

310

9 346

()

(1.3806 10 J/K) K

8 7305 10

3



44 10

310

10 28

()

(1.3806 10 J/K) K

3 4288 10

3



48 10

310

11 21

()

(1.3806 10 J/K) K

1 3466 10

3

The fraction of molecules at each energy level is

40 10

310



()

(1.3806 10 J/K) K

77305 10

13.506 10



64 6.%

25 4



3.4288 10

13.506 10

10 0



1.3466 10

13.506 10

Compare this with the results from Prob. 5-4. The increase in temperature

from 295 K to 310 K causes a decrease in the fraction of molecules at the low-

est energy level, from 66% to 64.6%. At the same time it causes an increase

in the fraction of molecules in the higher energy levels. F

went from 24.7%

to 25.4%, and F

went from 9.3% to 10.0%. These results are summarized in

Table 5-5. Increasing the temperature increases the internal energy of the

system which, according to statistical mechanics, is equivalent to increasing

the average energy of the molecules in the system. Statistical mechanics also

tells us that this increase in average energy comes about by moving some of

the molecules in the lowest energy level to higher energy levels.

PROBLEM

Given the same model as in Prob. 5-4, (three energy levels available: 4.0 ×

–20

J, 4.4 × 10

PROBLEM

Given the same model as in Prob. 5-4, (three energy levels available: 4.0 ×

SOLUTION

Everything is the same as in Prob. 5-4, except now the temperature is 310 K

✔

chapter 5 statistical Mechanics 99

Degeneracy

In statistical mechanics we speak of atoms or molecules existing in different

states, where each state corresponds to some change in the atom or molecule.

A state could be a particular confirmation, a particular mode of vibration, or a

particular configuration of the electrons. In many cases, each state is on its own

a unique energy level.

Sometimes, however, two or more states have the same energy. The molecule

can be in either of these two states and still be on the same energy level. When

this happens, we say that the energy level is degenerate, meaning that there is

more than one state with that amount of energy. The degeneracy of the energy

level is the number of different states with that energy.

For example, suppose a molecule has seven different conformations (shapes)

that it can be in. Now suppose of the seven different conformational states, one

shape has energy E

, one has energy E

, four have energy E

, and one has energy

. We say that energy level 3 is degenerate because there is more than one con-

firmation with energy E

. The degeneracy of energy level E

is 4. There are four

states all with energy E

There are two ways of dealing with degeneracy in statistical mechanics. One

way is to sum over all possible states of the system. So, for example, we write

the partition function

−

∑

STATES

(5-9)

STATES

is the number of states available to each molecule in the system.

Each j corresponds to a different state of the system, but the E

are not neces-

sarily all different; two or more of the E

can be the same. If there is no degen-

eracy, then all of the E

are different, and N

STATES

is equal to L

MAX

. But if there

is degeneracy, then at least some of the E

will be the same and N

STATES

will be

larger than L

MAX

TABLE 5-5 Fraction of molecules at

each energy level for 295

K and 310 K.

= 295 K

= 310 K

66.0% 64.6%

24.7% 25.4%

9.3% 10.0%

100 Biophysics DemystifieD

The second way to deal with degeneracy is to sum over energy levels (as we

did previously) but to multiply each term of the partition function by the

degeneracy of the energy level corresponding to that term. Thus the partition

function would be written

−

∑

MAX

(5-10)

where 

is the degeneracy of the ith energy level.

The two ways of dealing with degeneracy are equivalent. The method we

choose will depend on which is easier to use or more beneficial for the particu-

lar situation. Summing over energy levels is mathematically simpler, so we will

use that method most commonly unless there is a specific benefit to summing

over states. In the case of the example of a molecule with seven conformational

states, the difference between the two methods is the difference between the

following two equations:

Summing over states,

e eeeee

+++++

−−

−−−−

3333

−E

(5-11)

Summing over energy levels,

ee ee

++ +

−−

−

4⋅

(5-12)

One final note: Sometimes energy levels are referred to as energy states. They

are the same thing. The key is the use of the word energy to distinguish between

all possible states of the system, including those states with the same energy,

versus only the energy states (i.e., levels) of the system and using degeneracy to

account for other states of the system.

In an effort to keep the distinction clear, we will usually use the word levels

when talking about energy and the word states when including all configura-

tions of the system (not just energy states, but conformations, vibrational modes,

rotational modes, etc.). Sometimes, however, it will make sense for us to use the

term energy states. Just keep in mind that energy states and energy levels mean

the same thing.

The Reference State and Relative Energy

It is often convenient to define a reference state of the system and define all

energy levels relative to the energy of the reference state. For example, when

using statistical mechanics to examine the unwinding of the DNA double helix,

chapter 5 statistical Mechanics 101

we might define the fully twisted, closed double helix as the reference state. All

other relevant states of the system then involve partially or fully unwinding the

DNA double helix. Typically the reference state is chosen as the lowest energy

state of the system and is therefore sometimes also called the ground state. The

energy terms in our statistical mechanical calculations are then always the dif-

ference between the energy of a particular state and the ground state. Since

these involve energy differences, we write them as DE

, where

= E

– E

ref

(5-13)

and E

ref

is the energy of the reference state. One of the advantages of defining

a reference state is that we don’t have to know E

ref

. We only need to know or

measure the energy change in going from one state to the next, something that

experimentally is often much simpler to do than measuring the absolute energy

of a particular state.

With a reference state defined this way, we write the partition function as

follows:

−

∑

∆

MAX

(5-14)

Notice the introduction of 1 as the first term in the equation. This comes

from the fact that the energy difference between the reference state and itself

is zero: DE

ref

= E

ref

– E

ref

= 0, and any number raised to the zeroth power is equal

to 1. Notice that we are still counting from i = 1. We do this simply as a matter

of convenience by defining the reference energy level as level zero. Thus, we

can write the energy of the reference state as E

and DE

= E

– E

. We have also

defined the reference state as having no degeneracy (again a choice of conve-

nience), but if it did have degeneracy, we could write

−

∑

ωω

∆

MAX

(5-15)

where 

is the degeneracy of the reference energy state.

gibbs energy and the Biophysical Partition Function

When applying statistical mechanics in biophysics, we are typically interested in

conformational transitions in large biomolecules (such as DNA, proteins, and

lipids) or binding interactions of biological significance. In such cases the relevant

energy change is the Gibbs energy change. Other energy changes, for example,