Franc J-P. Fundamentals of Cavitation

Подождите немного. Документ загружается.

FUNDAMENTALS OF CAVITATION194

9.1

Typical shape of a truncated foil

with a ventilated base

Ventilation is operated artificially from

an air compressor on board. Air passes

through the legs of the boat or throug

the propeller hub and is injected at the

base of the foils or the propeller blades.

Thus, the base pressure is increased and

the drag is lowered.

When the angle of attack increases, air tends to invade the foil upper side, which

results in a decrease of the lift. Such a regime must be avoided, so that the operating

range of incidences is usually rather small [R

OWE 1979].

This kind of foil is suited to boat speeds in the range 40-80 knots, for which classical

non-cavitating foils are inoperative. For larger speeds, only supercavitating foils

with a non-wetted upper side can be used.

From an experimental viewpoint, it is essential that the hydrodynamic tunnel in

which ventilation tests are conducted is able to eliminate the injected air, so that

the water entering the test section is free of any traces of air after recirculation in

the tunnel loop. If the flow is unsteady, as is the case for pulsating cavities, the test

facility must ensure a good decoupling between the test section and the rest of the

loop in order to avoid any influence of the facility on the results.

In the case of three-dimensional foils of finite span, additional air cavities develop

in the tip vortices. They coexist with the two-dimensional air cavities whose

behavior is not significantly modified by them, except for very small values of

the wing aspect ratio [V

ERRON & MICHEL 1984].

9.1.2. THE MAIN PARAMETERS

The global features of ventilated flows such as cavity length, lift or drag, depend

on the relative cavity underpressure s

(see § 1.4.3):

(9.1)

is a reference pressure and p

the cavity pressure.

The pressure inside the cavity is the sum of the vapor pressure (as the cavity is

usually saturated with water vapor) and the pressure of the injected air:

ppp

c v air

(9.2)

In the case of substantial cavity pressure fluctuations, p

air

is the mean value of

the air pressure inside the cavity. For ventilated flows, p

is generally negligible

compared to p

air

9 - VENTILATED SUPERCAVITIES 195

The air flowrate is normally imposed and the resulting air pressure inside the cavity

is a priori unknown. It depends upon the mode of evacuation of air at the rear of

the cavity, which, in turn, depends upon the global flow geometry, particularly the

cavity length and the circulation, so that all parameters are strongly coupled.

Analysis of the experimental results [M

ICHEL 1971, 1984] suggests that the relative

underpressure be split into two terms:

— the classical cavitation parameter s

, which characterizes the ambient reference

pressure p

(9.3)

— and the relative mean pressure of the air inside the cavity, which takes into

account its elastic behavior due to compressibility:

air

(9.4)

Thus, the relative cavity underpressure s

is given by:

sss

cva

(9.5)

As s

is usually small, the two parameters s

and s

are close in value.

In the case of pulsating cavities, the regimes of pulsation are fairly well identified

via the ratio

, or equivalently

(see SILBERMAN &SONG, 1961, and

ONG, 1962, who first described the pulsation regimes).

As for the pulsation frequency f, the experimental results are satisfactorily correlated

by introducing the following non-dimensional frequency:

air

(9.6)

where l is the mean cavity length and d a fixed reference length, typically the chord

of the forebody.

As for the mass flowrate of air Q

, two non-dimensional flowrate coefficients can

be considered. These are:

— the mass flowrate coefficient:

(9.7)

— and the volumetric flowrate coefficient:

air

(9.8)

FUNDAMENTALS OF CAVITATION196

Here, S is a reference surface area. The mean air density r

air

can be determined

from the measurement of the cavity pressure p

combined with the BOYLE-MARIOTTE

law. In the last equation, the quantity

m air

represents the volumetric flowrate

of air.

If we introduce the mean velocity of the air inside the cavity V

air

and take an area

close to the cavity cross-section for S, we have approximately:

QVS

m air air

(9.9)

so that the volumetric air flow coefficient C

gives the order of magnitude of the

ratio of the air velocity to the water velocity:

air

(9.10)

The values of C

typically lie in the range 0.05 to 0.5.

Among the other classical non-dimensional parameters to be considered, there is

the F

ROUDE number:

(9.11)

which is often determined using the cavity length l in place of the reference length d.

9.1.3. CAVITY LENGTH

Experimental tests conducted on various configurations have shown that the

relative mean cavity length l /d, as well as the global lift and drag coefficients C

and C

, depend primarily on the relative cavity underpressure s

and, to a smaller

extent, on the F

ROUDE number, i.e.:

(,)s

(9.12)

In the case of a ventilated foil, the incidence a should be included.

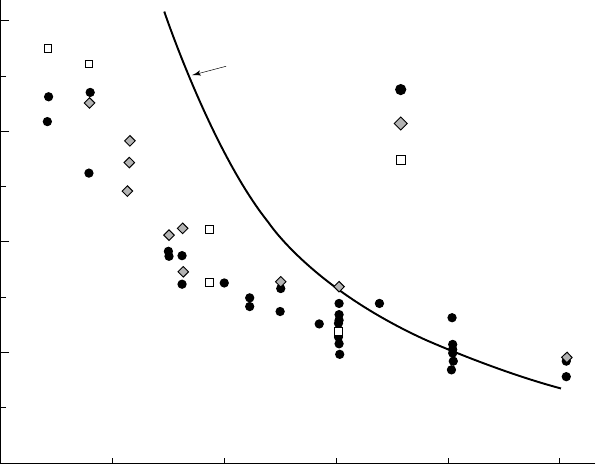
The influence of the relative cavity underpressure on cavity length is shown in

figure 9.2 in the case of ventilated cavities behind a wedge.

The experimental points correspond to various values of the cavitation number s

ranging from 0.4 to 10. Thus, the ambient pressure and consequently the different

air flow regimes (see § 9.1.5) do not significantly influence the mean cavity length.

The variation of l/c with s

suggests a power law (see chap. 6):

(9.13)

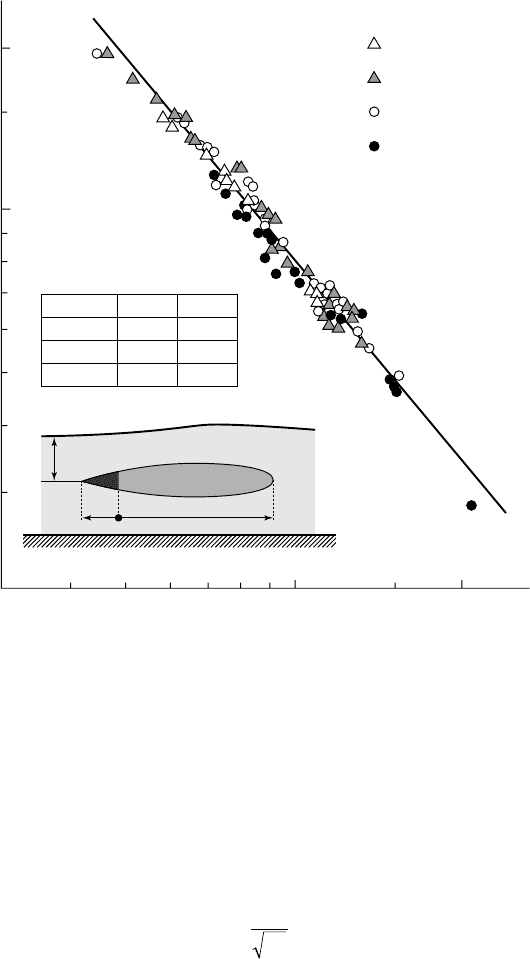
9 - VENTILATED SUPERCAVITIES 197

The table in figure 9.2 gives the values of A and n for three different submersion

depths. These values are close to those for vapor supercavities. The theoretical

asymptotic value of n for an infinite medium is 2 (see chap. 6), while n approaches

unity for small values of the submersion depth.

h [mm] A n

70 0.38 1.16

140 0.45 1.26

210 0.34 1.47

10 m/s

8 m/s

6 m/s

4 m/s

0.04 0.06 0.08 0.1 0.2

9.2 - Variation of the non-dimensional mean cavity length versus the relative

underpressure ss

for ventilated cavities behind a two-dimensional wedge

(chord 60.5 mm, base 17 mm) for four different values of the water velocity

The wedge is at mid-height of a free surface channel of 280 mm in depth. Values of

A and n for two other immersion depths are given in the table [from M

ICHEL, 1971].

In the range of velocities considered here, the F

ROUDE number has only negligible

influence on the cavity length, except for the smallest values of s

where a slight

deviation with respect to the power law becomes visible on figure 9.2. If the

ROUDE number is calculated using the cavity length, as suggested in section 6.2.2:

(9.14)

FUNDAMENTALS OF CAVITATION198

gravitational effects are expected to be negligible if Fr

is much larger than one.

For the smallest values of s

considered above, this condition is not fulfilled as the

ROUDE number is relatively small and close to one (typically

= 24.

for

l /c =18

l =109.m

and

Vms= 8/

), so that gravitational effects become more significant.

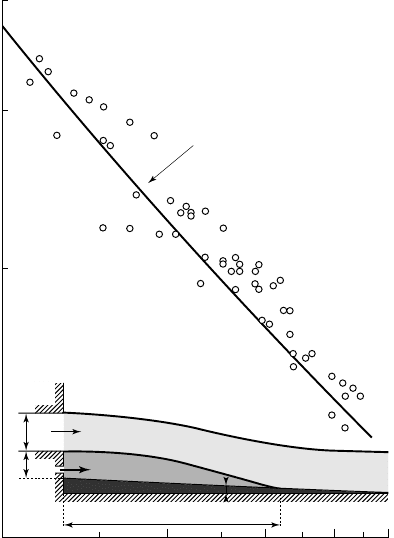
The dependence of the cavity length on the cavity underpressure s

in the half-

cavity configuration presented in figure 9.3 is qualitatively very similar to the case

of a wedge [L

AALI & MICHEL 1984]. This configuration is representative of the flow

in spillways aerators. It is simpler than the hydrofoil since the effects of incidence

and circulation are ruled out.

0.02 0.04 0.06 0.10.08

Relative cavity length l/h

Relative cavity underpressure s

air

Cavity



= –––– (1 + – sin b)

2Hh

9.3 - Cavity length versus ss

in the case of the half-cavity

The cavity is formed between an initially horizontal plane water jet of height

Hmm= 138 9.

and a wall with a small slope

=∞31.

. The step height is

hmm= 30

The reference pressure p

used in the definition of

and

is the pressure p

the upper free surface of the jet. The different points correspond to various values

and of the velocity (between 4 and 12 m/s) [from LAALI & MICHEL, 1984].

9 - VENTILATED SUPERCAVITIES 199

9.1.4. AIR FLOWRATE AND CAVITY PRESSURE

The cavity pressure p

depends mainly upon the air flowrate and to a smaller extent

upon the ambient pressure. This dependency can be written non-dimensionally as

follows:

cQvv

C(,)

(9.15)

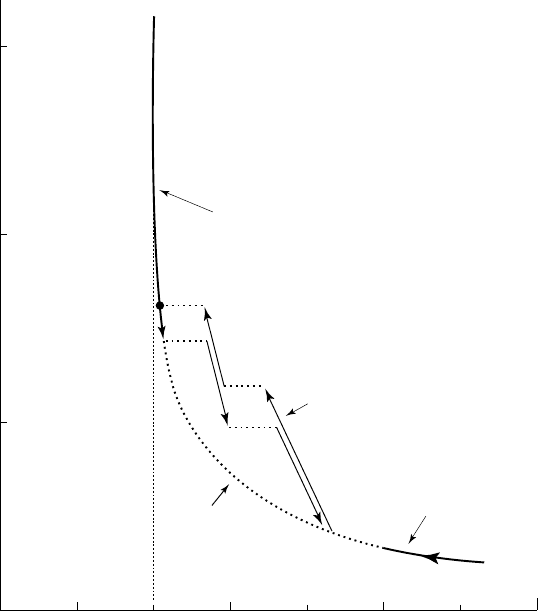
A schematic representation of the previous relationship is given on figure 9.4.

It shows the complex behavior of ventilated cavities with hysteresis effects. The

experimental procedure used to obtain the graph in figure 9.4 involves gradually

increasing the air flowrate up to high values before decreasing it.

Without pulsation

k+1

2D flow

3D flow

k+1

= pulsating regimes

with k, k + 1 ... wavelengths

0.3

0.2

0.1

0 0.01 0.03 0.05

Relative cavity underpressure σ

Volume airflow coefficient C

9.4 - Typical evolution of the relative cavity underpressure

for an increasing, then decreasing, air flowrate (␴

is constant)

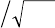
FUNDAMENTALS OF CAVITATION200

The ambient pressure, and therefore the cavitation parameter s

, is large enough

so that no vapor cavity preexists before air injection. In fact, if such a vapor cavity

were present, the phenomena would practically be unchanged except for small

-values (lower than 0.2 typically) for which a very small rate of air injection

would result in a large increase in cavity length, disclosing a kind of global flow

instability.

Roughly speaking, the curve of figure 9.4 is L-shaped. For very small injection

rates, the air forms separate bubbles which are entrapped in the alternate B

ÉNARD-

ÁRMÁN vortices of the body wake. For larger air flowrates, a continuous cavity

appears and it becomes possible to measure the cavity pressure and to determine

the corresponding s

parameter. This condition corresponds to the starting point

on the right-hand side of the curve.

A subsequent small increase in the air flowrate produces a large increase in the air

pressure and in the cavity length. This is due to the difficulty for air to escape from

the cavity. On the contrary, on the vertical branch of the curve, air is evacuated via

big bubbles, which break from the cavity on its upper interface upstream of its

closure point due to gravity. This mode of air evacuation is very efficient, so that

the cavity pressure generally reaches a maximum. Hence, the parameter s

tends to

a minimum (s

) and the cavity length to a maximum.

The connecting region between the horizontal and the vertical branch of the curve

is characterized by periodic pulsations of the air cavity, which will be considered

more extensively in next section.

Examples of experimental curves

Qv c

()s

, corresponding to three water velocities

in the half-cavity configuration are shown in figure 9.5.

The minimum value s

of the relative cavity underpressure s

depends mainly

upon the F

ROUDE number

Fr V gH=

, as shown on figure 9.6. It decreases with the

ROUDE number, i.e. with the flow velocity for a given jet height. The parameter s

is also an increasing function of s

at constant FROUDE number, as seen in figure 9.6,

where several experimental points are indexed with the corresponding s

-values.

For reference purposes, figure 9.6 shows the curve

Fr= 2

corresponding to the

case of a jet with equal values of pressure on both sides. The pressure on the free

surface is assumed equal to the pressure inside the cavity and the jet is subject

only to gravity. When s

is smaller than 2/Fr

, which occurs for small values of

the F

ROUDE number, the pressure in the cavity is greater than the ambient pressure.

The water jet lies on a cushion of pressurized air and the transverse pressure

gradient promotes the rise of air bubbles. An increase in the air flow compels the

air to escape the cavity from its upper interface by separate bubbles, without any

increase in cavity length.

9 - VENTILATED SUPERCAVITIES 201

However, for larger values of the FROUDE number, the transverse pressure gradient

diminishes and tends to be reversed, which makes the rise of air bubbles more

difficult, so that a greater value of the cavity length, or a smaller value of s

, is

required for the appropriate air evacuation.

Pulsating

regime R

Non pulsating

regime

8 m/s

10 m/s

12 m/s

0.4

0.5

0.3

0.2

0.1

0.01 0.02 0.03 0.04 0.05

Relative cavity underpressure σ

Volume airflow coefficient C

9.5 - Evolution of the cavity pressure ␴

with the air flowrate C

at constant ambient pressure

␴

= 044.

in the case

of the half-cavity configuration

(

Hmm= 100 3.

hmm= 49 2.

)

The area S in the air flow coefficient is based on the height h and the width of the

test section (see fig. 9.3) [from L

AALI & MICHEL, 1984].

FUNDAMENTALS OF CAVITATION202

Regime R1

Regime R2

Non-pulsating regime

1.44

0.294

1.50

0.60

0.29

0.32

0.30

0.29

1.02

1.11

3.02

8.06

= –––

0.08

0.06

0.04

0.02

42681012

Minimum relative underpressure s

ROUDE

number Fr

9.6 - Variation of the minimum relative underpressure ␴

with the FROUDE number for the half-cavity configuration

Several experimental points are indexed with the corresponding

-values.

[from L

AALI & MICHEL, 1984]

9.1.5. PULSATION REGIMES

The intermediate region in figure 9.4 is characterized by periodic pulsations of the

air cavity. In this case, undulations of increasing amplitude are convected on both

free frontiers of the ventilated cavity at a speed equal to the liquid velocity on the

interfaces. They join at the rear part of the cavity, allowing air to escape periodically

under the form of separate air pockets, as shown on figure 9.7.

The air pressure undergoes periodic and almost sinusoidal fluctuations in time.

The minimum pressure occurs when an air pocket leaves the cavity. At the same

time, undulations of the free surface arise at the two trailing edges of the wedge.

The cavity, as it reaches its minimum length, has the shape of one or several

spindles. The corresponding flow regimes are named R

, R

, ..., R

according to

the number k of spindles. Regime R

is presented in figure 9.12. In general, high

order regimes are obtained for large values of the ambient pressure or, more

precisely, for small values of the parameter

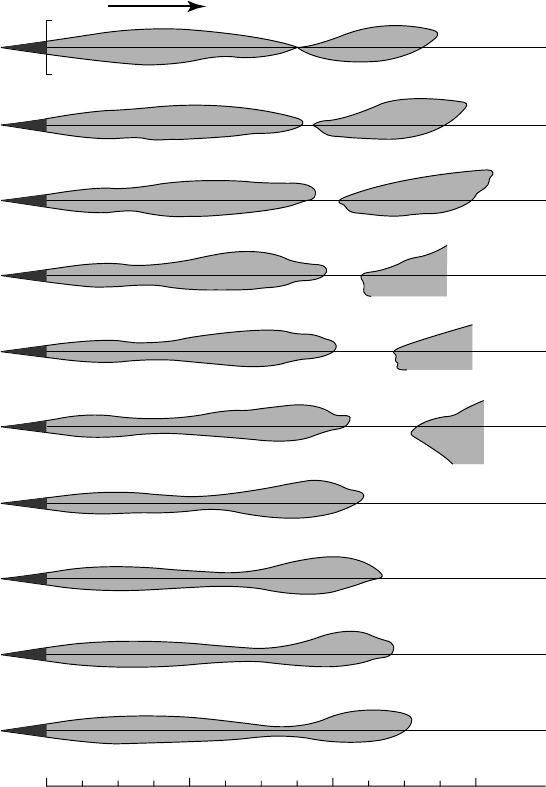
9 - VENTILATED SUPERCAVITIES 203

40 mm

– 40 mm

600

4002000

0.1 T

0.2 T

0.3 T

0.4 T

0.5 T

0.6 T

0.7 T

0.8 T

0.9 T

9.7 - Evolution of a one-wave cavity during one period

of pulsation for V ==

4 m/s, ␴

= 0.111, ␴

/␴

= 0.137

The pulsation frequency is

fHz= 11 1.

for this R

regime. A slight gravitational effect

is visible [from M

ICHEL, 1971].

The passage from one regime R

to the next R

k+1

occurs for critical values of the

volumetric air flow coefficient. It is accompanied by a jump in both the cavity

pressure and the cavity length. The critical values of C

are different for increasing

or decreasing injection rates (see fig. 9.4), so that the curve

Qv c

()s

presents

hysteresis loops.