Everitt B.S. The Cambridge Dictionary of Statistics

Подождите немного. Документ загружается.

Instrumental var iab l e esti mato r: An estimator for linear regression models with endogenous

covariates. Consider a regression model y ¼ α þβx þ " where x is an endogenous variable

since it is correlated with the error term ". Such endogeneity, which may be due to for

instance covariate measurement error or unobserved heterogeneity, renders the ordinary

least squares estimator of β inconsistent. However, a consistent estimator can be obtained if

an instrumental variable z is found which is correlated with x but uncorrelated with ". The

instrumental variable estimator of β is given by:

¼ðz

xÞ

1

where z, x and y are n x 1 vectors and n is the sample size. [Microeconometrics, 2005, A. C.

Cameron and P. K. Trivedi, Cambridge University Press, Cambridge.]

Integrated hazard functi on: Synonym for cumulative hazard function.

Intenti on-to-treat analysis: A procedure in which all patients randomly allocated to a treatment

in a

clinical trial

are analysed together as representing that treatment, whether or not they

completed, or even received it. Here the initial random allocation not only decides the

allocated treatment, it decides there and then how the patient’s data will be analysed, whether

or not the patient actually receives the prescribed treatment. This method is adopted to

prevent disturbances to the prognostic balance achieved by randomization and to prevent

possible bias from allowing compliance, a factor often related to outcome, to determine the

groups for comparison. [

Controlled Clinical Trials

, 2000, 21, 167–189.]

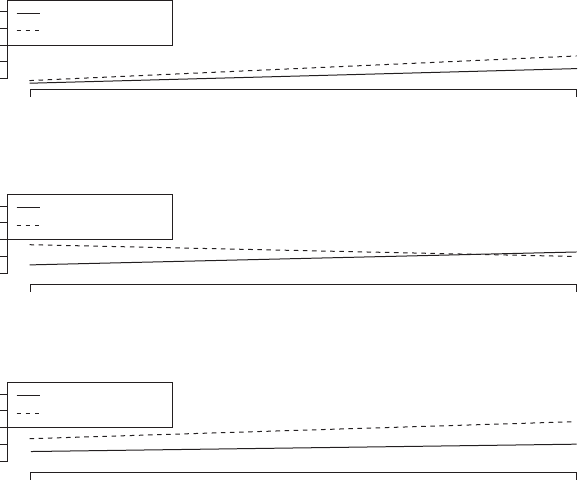
Interaction: A term applied when two (or more) explanatory variables do not act independently on a

response variable. Figure 72 shows an example from a 2  2 factorial design. See also

additive effect. [SMR Chapter 12.]

Intercept: The parameter in an equation derived from a regression analysis corresponding to the

expected value of the response variable when all the explanatory variables are zero.

Intercro ppi ng experi ments: Experiments involving growing two or more crops at the same

time on the same piece of land. The crops need not be planted nor harvested at exactly the

same time, but they are usually grown together for a signiﬁcant part of the growing

season. Used extensively in the tropics and subtropics, particularly in developing coun-

tries where people are rapidly depleting scarce resources but not producing enough food.

[Statistical Design and Analysis for Intercropping Experiments, 1999, T. Walter, Springer,

New York.].

Interim analyses: Analyses made prior to the planned end of a

clinical trial

, usually with the aim of

detecting treatment differences at an early stage and thus preventing as many patients as

possible receiving an ‘inferior’ treatment. Such analyses are often problematical particularly

if carried out in a haphazard and unplanned fashion. See also alpha spending function.

[Statistics in Medicine, 1994, 13, 1401–10.]

Interior analysis: A term sometimes applied to analysis carried out on the ﬁtted model in a

regression problem. The basic aim of such analyses is the identiﬁcation of problem areas

with respect to the original

least squares

ﬁt of the ﬁtted model. Of particular interest is the

disposition of individual data points and their relative

inﬂuence

on global measures used as

guides in subsequent stages of analysis or as estimates of parameters in subsequent models.

Outliers

from the ﬁtted or predicted response, multivariate outliers among the explanatory

variables, and points in the space of explanatory variables with great

leverage

on the ﬁtted

model should be identiﬁed, and their inﬂuence evaluated before further analysis is under-

taken. [Quality and Quantity, 1994, 28,21–53.]

219

Interpolation: The process of determining a value of a function between two known values without

using the equation of the function itself. [SMR Chapter 3.]

Interquarti le range: A measure of spread given by the difference between the ﬁrst and third

quartiles of a sample. [SMR Chapter 3.]

Interrupted time series design: A study in which a single group of subjects is measured several

times before and after some event or manipulation. Often also used to describe investigations

of a single subject. See also longitudinal data and N of 1 clinical trial.[Experimental

and Quasi-Experimental Designs for Generalized Casual Inference, 2002, W. R. Shadish,

T. D. Cook and D. T. Campbell, Houghton Mifﬂin, Boston.]

Interruptib le designs: Experimental designs that attempt to limit the information lost if an experi-

ment is prematurely ended. [Technometrics, 1982, 24,55–8.]

Interval-censoredobservations: Observations that often arise in the context of studies of time

elapsed to a particular event when subjects are not monitored continuously. Instead the prior

occurrence of the event of interest is detectable only at speciﬁc times of observation, for

example, at the time of medical examination. [Statistics in Medicine, 1988, 7, 1139–46.]

Interval esti mate: See estimate.

Interval esti mation: See estimation.

Interval variable: Synonym for continuous variable.

Intervened Poisson distribution: A probability distribution that can be used as a model for a

disease in situations where the incidence is altered in the middle of a data collection period

Present

Drug X, diet absent

Drug X, diet present

160 200 240

Mean BP

Absent

Biofeed

160 200 240

Mean BP

160 200 240

Mean BP

Drug Y, diet absent

Drug Y, diet present

Drug Z, diet absent

Drug Z, diet present

Present Absent

Biofeed

Present Absent

Biofeed

Fig. 72 Interaction in a 2 2  2 design. (Reproduced from Experiment Design and Statistical

Methods, D. R. Boniface, by permission of the publishers Chapman and Hall, London.)

220

due to preventative treatments taken by health service agencies. The mathematical form of

the distribution is

Pðnumber of cases ¼ xÞ¼½e



ðe



 1Þ

1

½ð1 þÞ

 



=x!

where x ¼ 1; 2; .... The parameters ð

0Þ and ð0   1Þmeasure incidence and

intervention, respectively. A zero value of  is indicative of completely successful preven-

tive treatments, whereas  ¼ 1 is interpreted as a status quo in the incidence rate even after

the preventive treatments are applied. [Communications in Statistics – Theory and Methods,

1995, 24, 735–54.]

Interventionanalysisintime series: An extension of

autoregressive integrated moving average

models

applied to

time series

allowing for the study of the magnitude and structure of

changes in the series produced by some form of intervention. An example is assessing how

efﬁcient is a preventive programme to decrease monthly number of accidents. [Journal of

the American Statistical Association, 1975, 70,70–9.]

Intervention study: Synonym for clinical trial.

Interviewer bias: The

bias

that occurs in surveys of human populations because of the direct result

of the action of the interviewer. This bias can arise for a variety of reasons including failure

to contact the right persons and systematic errors in recording the answers received from the

respondent. [Journal of Occupational Medicine, 1992, 34, 265–71.]

Intraclass contingency table: A table obtained from a

square contingency table

by pooling the

frequencies of cells corresponding to the same pair of categories. Such tables arise fre-

quently in genetics when the genotypic distribution at a single locus with r alleles,

; A

; ...; A

, is observed. Since A

is indistinguishable from A

, i 6¼ j, only the total

frequency of the unordered pair A

is observed. Thus the data consist of the frequencies of

homozygotes and the combined frequencies of heterozygotes. [Statistics in Medicine, 1988,

7, 591–600.]

Intraclasscorrelation: Although originally introduced in genetics to judge sibling correlations, the

term is now most often used for the proportion of variance of an observation due to between-

subject variability in the ‘true’ scores of a measuring instrument. Speciﬁcally if an observed

value, x, is considered to be true score (t) plus measurement error (e), i.e.

x ¼ t þ e

the intraclass correlation is



ð

þ 

where 

is the variance of t and 

the variance of e. The correlation can be estimated from a

study involving a number of raters giving scores to a number of patients. Also used to

quantity the dependence of responses for units nested in clusters according to a random

intercept model. [KA2 Chapter 26.]

Intrinsic aliasing: See aliasing.

I n t rinsi ca lly no n-linea r mode l s: See non-linear models.

Intrinsic error: A term most often used in a clinical laboratory to describe the variability in results

caused by the inate imprecision of each analytical step.

Int r i nsic rate of natu ral inc rease: Synonym for Malthusian parameter.

221

In vari ance: A property of a set of variables or a statistic that is left unchanged by a transformation. The

variance of a set of observations is, for example, invariant under addition or subtraction of a

constant from the data.

Inverse Bernoulli sampling: A series of

Bernoulli trials

that is continued until a preassigned

number, r, of successes have been obtained; the total number of trials necessary to achieve

this, n, is the observed value of a random variable, n, having a

negative binomial distribu-

tion

.[Statistica Neerlandica, 2002, 56, 387–399.]

Inversedistributionfunction: A function GðαÞsuch that the probability that the random variable

X takes a value less than or equal to it is α, i.e.

Pr½X  GðαÞ ¼ α

Inverse Gaussian distribution: Synonym for inverse normal distribution.

Inverse normal distribution: The probability distribution, f (x), given by

f ðxÞ¼



2px



exp 

lðx Þ

2

()

; x

where  and l are both positive. The mean, variance,

skewness

and

kurtosis

of the

distribution are as follows:

means ¼ 

variance ¼ 

skewness ¼ 3ð=lÞ

kurtosis ¼ 3 þ

15

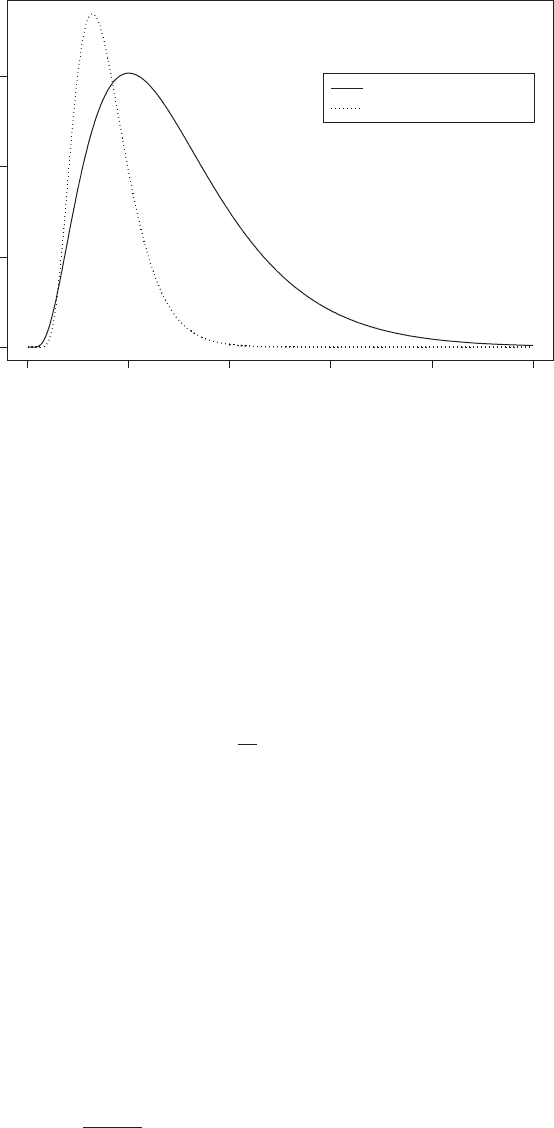
A member of the

exponential family

which is skewed to the right. Examples of the

disitribution are shown in Fig. 73. [STD Chapter 21.]

Inverse polynomial functions: Functions useful for modelling many

dose–response

relation-

ships in biology. For a particular dose or stimulus x, the expected value of the response

variable, y,isdeﬁned by

E ð yÞ¼

x þα

i¼0

ðx þαÞ

x  0

The parameters, β

; β

; ...; β

,deﬁne the shape of the dose–response curve and α deﬁnes its

position on the x axis. A particularly useful form of the function is obtained by setting α ¼ 0

and d ¼ 1. The resulting curve is

E ð yÞ¼

þ β

x  0

which can be rewritten as

EðyÞ¼

þ x

where k

¼ 1=β

and k

¼ β

=β

. This ﬁnal equation is equivalent to the

Michaelis–Menten

equation. [Biometrics, 1966, 22, 128–41.]

222

In ve rse sin e transfo rmati o n: Synonymous with arc sine transformation.

Inverse survival functi on: The quantile, ZðαÞ that is exceeded by the random variable X with

probability α, i.e.

P½X

ZðαÞ ¼ α

ZðαÞ¼Gð1  αÞ where G is the

inverse distribution function

Inversion theorem: A theorem that proves that a probability distribution, f(x), is uniquely deter-

mined by its

characteristic function

, ðtÞ. The theorem states that

f ðxÞ¼

1

itx

ðtÞdt

[KA1 Chapter 4.]

Inverted Wishart distribution: The distribution of the inverse of a positive deﬁnite matrix, A,if

and only if A

1

has a Wishart distribution. [Aspects of Multivariate Statistical Theory, 1982,

R. J. Muirhead, Wiley, New York.]

IRLS: Abbreviation for iteratively reweighted least squares.

Irreducible chain: A

Markov chain

in which all states intercommunicate. [Introduction to

Probability Theory and its Applications, Volume 1, 2nd edition, 1967, W. Feller, Wiley,

New York.]

Irwin ^ Hall distribution: The probability distribution of the sum, S,ofn independent random

variables each with a

uniform distribution

in (0,1). The distribution is given by

PrðS ¼ sÞ¼

ðn 1Þ!

j¼0

ð1Þ



ðs jÞ

n1

; k  s  k þ 1; 0  k  n  1

¼ 0; elsewhere

f(x)

0246810

0123

mu = 1.0, lambda = 2.0

mu = 1.0, lambda = 6.0

Fig. 73 Examples of inverse normal distributions:  ¼ 1:0; l ¼ 2:0, 6.0.

223

[Continuous Univariate Distributions, Volume 2, 2nd edition, 1995, N. L. Johnson, S. Kotz

and N. Balakrishnan, Wiley, New York.]

Irwin, Joseph Oscar (1898^1982): Born in London, Irwin was awarded a mathematics scholar-

ship to Christ’s College, Cambridge in December 1917, but because of the war did not

graduate until 1921, when he immediately joined

Karl Pearson

’s staff at University College,

London. In 1928 he joined

Fisher

at Rothamsted Experimental Station, working there until

1931, when he joined the Medical Research Council, where he stayed until 1965. He worked

on a variety of statistical problems arising from areas such as animal carcinogenicity,

accident proneness, vaccines and hot environments for soldiers in the tropics. Received

the Royal Statistical Society’s Guy Medal in silver in 1953 and acted as President of the

British Region of the Biometric Society in 1958 and 1959. Irwin died on 27 July 1982 in

Schaffhausen, Switzerland.

Ising^ Stev ens d i stri b ut io n: The probability distribution of the number of runs, X, of either of

two types of objects (n

of one kind and n

of the other) arranged at random in n ¼ n

þ n

positions around a circle. Given by

PrðX ¼ xÞ¼



 1

x 1



þ n

 1



[Annals of Eugenics , 1939, 9,10–17.]

Isobo le: See isobologram.

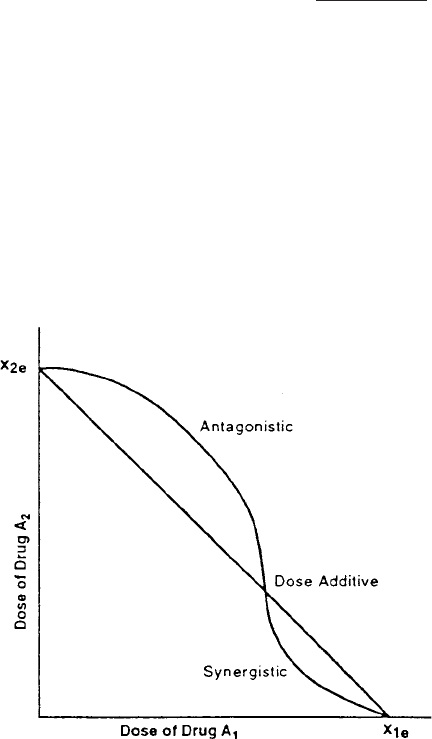
Isobologram: A diagram used to characterize the interactions among jointly administered drugs or

chemicals. The contour of constant response (i.e. the isobole), is compared to the ‘line of

additivity’, i.e. the line connecting the single drug doses that yield the level of response

associated with that contour. The interaction is described as

synergistic

, additive, or

antag-

onistic

according to whether the isobole is below, coincident with, or above the line of

additivity. See Fig. 74 for an example. [Statistics in Medicine, 1994, 13, 2289–310.]

Fig. 74 An example of an

isobologram.

224

Isoton i c reg ressio n: A form of regression analysis that minimizes a weighted sum of squares

subject to the condition that the regression function is order preserving. [Statistical Inference

under Order Restrictions, 1972, R. E. Barlow, D. J. Bartholomew, J.M Bremmer and H. D.

Brunk, Wiley, New York.]

Item difficulty: See Rasch model.

Item non-response: A term used about data collected in a survey to indicate that particular

questions in the survey attract refusals, or responses that cannot be coded. Often this type

of missing data makes reporting of the overall response rate for the survey less relevant. See

also non-response.[Journal of the American Statistical Association, 1994, 89, 693–6.]

Ite m -r esponse th eo ry: A set of statistical models and methods designed to measure abilities from

items answered in ability tests. See also Rasch model.[Psychometrika, 1981, 443–59.]

Ite m- t ota lcor r elat ion: A widely used method for checking the homogeneity of a scale made up of

several items. It is simply the

Pearson’s product moment correlation coefﬁcient

of an

individual item with the scale total calculated from the remaining items. The usual rule of

thumb is that an item should correlate with the total above 0.20. Items with lower correlation

should be discarded. [Health Measurement Scales, 3rd edition, 2003, D. L. Streiner and

G. R. Norman, Oxford Medical Publications, Oxford.]

Iterated bootstrap: A two-stage procedure in which the samples from the original

bootstrap

population are themselves bootstrapped. The technique can give

conﬁdence intervals

more accurate coverage than simple bootstrapping. [Biometrika, 1994, 81, 331–40.]

Iterated conditional modes algorithm (ICM): A procedure analogous to

Gibbs sampling

with the exception that the mode of each conditional

posterior distribution

is determined at

each update, rather than sampling a value from these conditional distributions. [Ordinal

Data Modelling, 1999, V. E. Johnson and J. H. Abbers, Springer, New York.]

Ite r ati on: The successive repetition of a mathematical process, using the result of one stage as the input

for the next. Examples of procedures which involve iteration are

iterative proportional

ﬁtting

, the

Newton–Raphson method

and the

EM algorithm

Iteratively reweighted least squares (IRLS): A

weighted least squares

procedure in which

the weights are revised or re-estimated at each iteration. In many cases the result is

equivalent to

maximum likelihood estimation

. Widely used when ﬁtting

generalized linear

models

. [GLM Chapter 2.]

Ite r a t i v e proportio n a l fi tting: An algorithm that allows the construction of two-way contin-

gency tables with speciﬁed

marginal totals

and a speciﬁed degree of association. The

algorithm can be used to ﬁnd

maximum likelihood estimates

of the frequencies to be

expected assuming a particular

log-linear model

when such estimates cannot be found

directly from simple calculations using relevant marginal totals. [The Analysis of

Contingency Tables, 2nd edition, 1992, B. S. Everitt, Chapman and Hall/CRC Press,

London.]

225

Jaccard coefficient: A

similarity coefﬁcient

for use with data consisting of a series of

binary

variables

that is often used in

cluster analysis

. The coefﬁcient is given by

a þb þ c

where a, b and c are three of the frequencies in the 2 × 2 cross-classiﬁcation of the variable

values for subjects i and j. The critical feature of this coefﬁcient is that ‘negative matches’ are

excluded. See also matching coefﬁcient. [MV1 Chapter 3.]

J ackkn i fe: A procedure for reducing bias in estimation and providing approximate

conﬁdence

intervals

in cases where these are difﬁcult to obtain in the usual way. The principle behind

the method is to omit each sample member in turn from the data thereby generating n

separate samples each of size n − 1. The parameter of interest, , can now be estimated from

each of these subsamples giving a series of estimates,



;



; ...;



. The jackknife estimator

of the parameter is now

 ¼ n

 ðn  1Þ

i¼1



where

 is the usual estimator using the complete set of n observations. The jackknife

estimator of the standard error of

 is

^

ðn 1Þ

i¼1





Þ

where



 ¼

i¼1



A frequently used application is in

discriminant analysis

, for the estimation of

misclassiﬁ-

cation rates

. Calculated on the sample from which the

classiﬁcation rule

is derived, these are

known to be optimistic. A jackknifed estimate obtained from calculating the discriminant

function n times on the original observations, each time with one of the values removed, is

usually a far more realistic measure of the performance of the derived classiﬁcation rule.

[KA1 Chapter 10.]

Jacobian leverage: An attempt to extend the concept of

leverage

in regression to

non-linear models

of the form

¼ f

ðqÞþ

ði ¼ 1; ...; nÞ

where f

is a known function of the vector of parameters q. Formally the Jacobian leverage,

is the limit as b ! 0of

226

qÞf

qðk; bÞg=b

where

q is the

least squares

estimate of q and

qðk; bÞis the corresponding estimate when y

replaced by y

þ b. Collecting the

sinann × n matrix J give

J ¼

Vð

V ½

’

½

WÞ

1

where

’

is the n × 1 vector of residuals with elements

’

¼ y

 f

qÞ and

’

½

W¼

i¼1

’

: W

denotes the

Hessian matrix

of f

ðqÞ and

V is the matrix whose

ith row is @f

ðqÞ=@q

evaluated at q ¼

q. The elements of J can exhibit

superleverage

[Biometrika, 1993, 80,99–106.]

James^Stein estimators: A class of estimators that arise from discovery of the remarkable fact

that in a

multivariate normal distribution

with dimension at least three, the vector of sample

means,



x, may be an inadmissable estimator of the vector of population means, i.e. there are

other estimators whose risk functions are everywhere smaller than the risk of



x, where the

risk function of an estimator is deﬁned in terms of variance plus bias squared. If

¼½X

; X

; ...; X

 then the estimators in this class are given by



a;b

ðXÞ¼ 1 

b þX



If a is sufﬁciently small and b is sufﬁciently large, then 

a;b

has everywhere smaller risk than

X.[Journal of Multivariate Analysis, 2006, 97, 1984–1986.]

Jeffreys’sdistance: See Kullback –Leibler information.

Jeffreys, SirHarold(1891^1989): Born in Fatﬁeld, Tyne and Wear, Jeffreys studied at St John’s

College, Cambridge where he was elected to a Fellowship in 1914, an appointment he held

without a break until his death. From 1946 to 1958 he was Plumian Professor of Astronomy.

Made signiﬁcant contributions to both geophysics and statistics. His major work Theory

of Probability is still in print nearly 70 years after its ﬁrst publication. Jeffreys was made

a Fellow of the Royal Society in 1925 and knighted in 1953. He died in Cambridge on

18 March 1989.

Jeffreys’sprior: A probability distribution proportional to the square root of the

Fisher information

[Kendall’s Advanced Theory of Statistics, Volume 2B, 2nd edition, 2004, A. O’Hagan and

J. Forster, Arnold, London.]

Jelinski ^ Moranda model: A model of software reliability that assumes that failures occur

according to a Poisson process with a rate decreasing as more faults are discovered. In

particular if  is the true failure rate per fault and N is the number of faults initially present,

then the failure rate for the ith fault (after i − 1 faults have already been detected, not

introducing new faults in doing so) is

¼ðN  i þ 1Þ

[Statistical Computer Performance Evaluation, 1972, ed. P. Freberger, Academic Press,

New York.]

Jenkins, Gwilym Meirion (1933^1982): Jenkins obtained a ﬁrst class honours degree in

mathematics from University College, London in 1953 and a Ph.D. in 1956. He worked

for two years as a junior fellow at the Royal Aircraft Establishment gaining invaluable

227

experience of the spectral analysis of time series. Later he become lecturer and then reader at

Imperial College London where he continued to make important contributions to the

analysis of

time series

. Jenkins died on 10 July 1982.

Jensen’sinequality: For a random variable y, the inequality

gðÞE½gðyÞ

where  ¼ EðyÞ and g is a concave function. Used in information theory to ﬁnd bounds or

performance indicators. [Bulletin of the Australian Mathematical Society, 1997, 55, 185–9.]

Jewell’s estimator: An estimator of the

odds ratio

given by

ψ ¼

ðb þ1Þðc þ 1Þ

where a, b, c and d are the cell frequencies in the

two-by-two contingency table

of interest.

See also Haldane’s estimator.[Statistics in Medicine, 1989, 8, 987–96.]

Jitteredsampling: A term sometimes used in the analysis of

time series

to denote the sampling of a

continuous series where the intervals between points of observation are the values of a

random variable. [Annals of the Institute of Statistical Mathematics , 1996, 48,29–48.]

Jit tering: A procedure for clarifying

scatterplots

when there is a multiplicity of points at many of the

plotting locations, by adding a small amount of random variation to the data before graphing.

An example is shown in Fig. 75.

Johnson-Neyman technique: A technique that can be used in situations where

analysis of

covariance

is not valid because of heterogeneity of slopes. With this technique the expected

value of the response variable is assumed to be a linear function of the one or two covariates

of interest, but not the same linear function in the different subject groups. [Psychometrika,

1964, 29, 241 –256.]

Johnson’s system of distributions: A ﬂexible family of probability distributions that can be

used to summarize a set of data by means of a mathematical function which will ﬁt the data

and allow the estimation of percentiles. Based on the following transformation

z ¼ γ þk

ðx; l;Þ

where γ is a standard normal variable and the k

ðx; l;Þ are chosen to cover a wide range of

possible shapes. The following are most commonly used:

ðx; l;Þ¼sin h

1

x  



the S

distribution;

ðx; l;Þ¼ln

x  

l þ  x



the S

distribution;

ðx; l;Þ¼ln

x  



the S

distribution. The S

is in essence a three-parameter

lognormal distribution

since the

parameter l can be eliminated by setting γ



¼ γ   ln l so that z ¼ γ



þ  lnðx  Þ. The

is a distribution bounded on ð;  þ lÞ and the S

is an unbounded distribution. [KA1

Chapter 6.]

228