Edelstein-Keshet L. Mathematical Models in Biology
Подождите немного. Документ загружается.


Discrete Processes
in
Biology
sively
on
what
causes these geometric designs
and
patterns
in
plants, although
the
subject
has
been pursued
for
over
three centuries.
2
Fibonacci stumbled unknowingly onto
the
esoteric realm
of r
through
a
ques-
tion
related
to the
growth
of
rabbits (see problem 14). Equation
(1) is
arguably
the
first
mathematical idealization
of a
biological
phenomenon phrased
in
terms
of a re-
cursion relation,
or in
more common terminology,
a
difference
equation.
Leaving aside
the
mystique
of
golden rectangles, parastichies,
and
rabbits,
we
find
that
in
more mundane realms, numerous biological events
can be
idealized
by
models
in
which similar discrete equations
are
involved. Typically, populations
for
which difference equations
are
suitable
are
those
in
which adults
die and are
totally
replaced
by
their progeny
at fixed
intervals
(i.e.,
generations
do not
overlap).
In
such
cases,
a
difference equation might summarize
the
relationship between popula-
tion density
at a
given generation
and
that
of
preceding generations. Organisms that
undergo abrupt changes
or go
through
a
sequence
of
stages
as
they
mature
(i.e.,
have discrete life-cycle stages)
are
also commonly described
by
difference
equa-
tions.
The
goals
of
this chapter
are to
demonstrate
how
equations
such
as (1)
arise
in
modeling
biological
phenomena
and to
develop
the
mathematical techniques
to
solve
the
following problem: given particular starting population levels
and a
recursion
re-
lation, predict
the
population level
after
an
arbitrary number
of
generations have
elapsed.
(It
will soon
be
evident that
for a
linear equation such
as
(1),
the
mathemat-
ical sophistication required
is
minimal.)
To
acquire
a
familiarity with difference equations,
we
will begin with
two
rather
elementary examples:
cell
division
and
insect growth.
A
somewhat more elab-
orate problem
we
then investigate
is the
propagation
of
annual
plants. This topic will
furnish
the
opportunity
to
discuss
how a
slightly more complex model
is
derived.
Sections
1.3 and 1.4
will
outline
the
method
of
solving certain linear
difference
equations.
As a
corollary,
the
solution
of
equation
(1) and its
connection
to the
golden
mean will
emerge.
1.1
BIOLOGICAL
MODELS
USING
DIFFERENCE
EQUATIONS
Cell
Division
Suppose
a
population
of
cells
divides synchronously,
with
each member producing
a
daughter
cells.
3
Let us
define
the
number
of
cells
in
each generation with
a
subscript,
that
is, M1, M
2
, . . . , M
n
are
respectively
the
number
of
cells
in the first,
second,
. . . , nth
generations.
A
simple equation relating successive generations
is
2. An
excellent
summary
of the
phenomena
of
phyllotaxis
and the
numerous
theories
that
have
arisen
to
explain
the
observed
patterns
is
given
by R. V.
Jean
(1984).
His
book contains
nu-
merous suggestions
for
independent
research
activities
and
problems related
to
phyllotaxis.
See
also
Thompson (1942).
3.
Note that
for
real
populations only
a > 0
would make
sense;
a < 0 is
unrealistic,
and
a
= 0
would
be
uninteresting.
6
The
Theory
of
Linear
Difference
Equations
Applied
to
Population
Growth
1
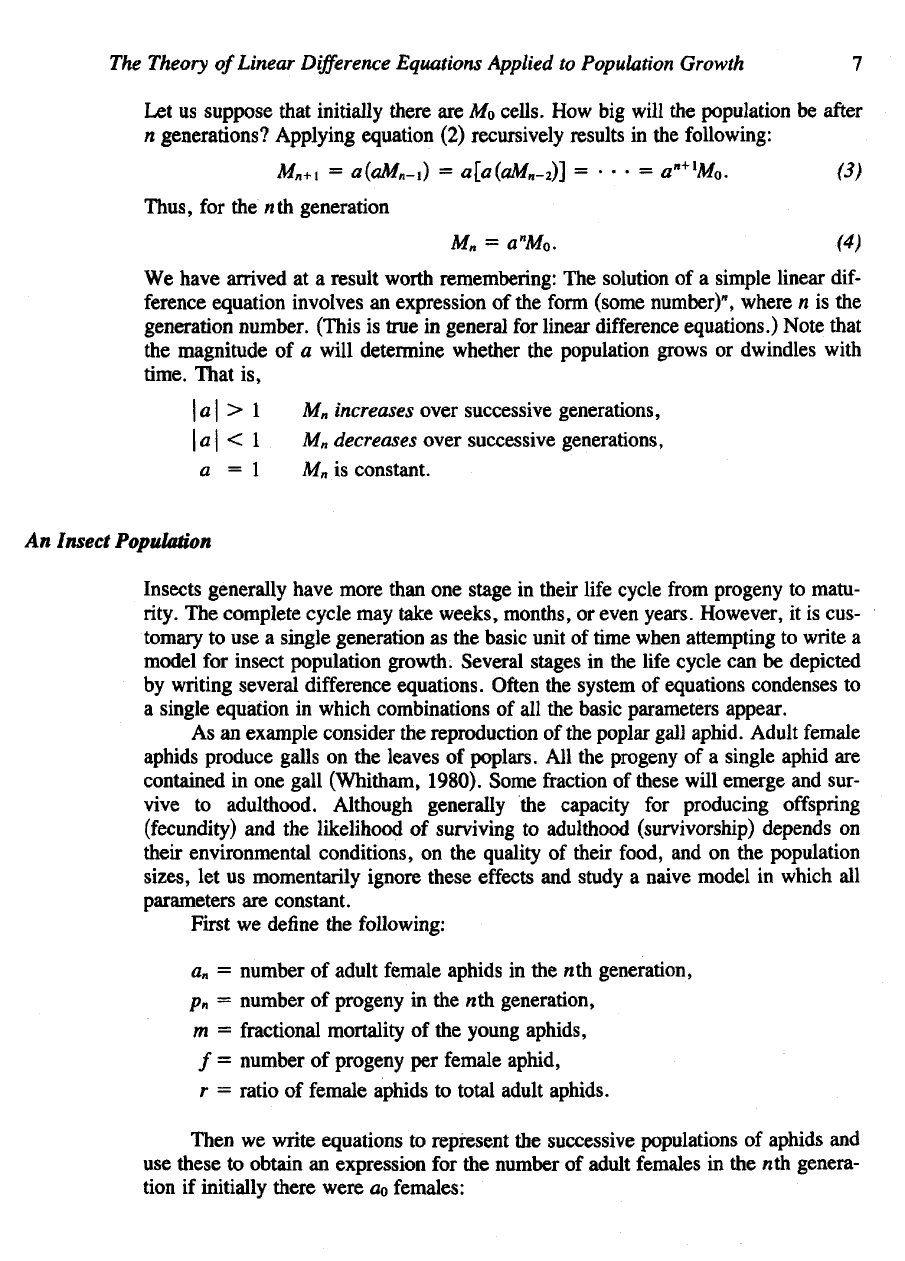
Let us
suppose
that initially there
are Mo
cells.
How big
will
the
population
be
after
n
generations?
Applying equation
(2)
recursively results
in the
following:
Thus,
for the nth
generation
We
have arrived
at a
result worth remembering:
The
solution
of a
simple linear dif-
ference
equation involves
an
expression
of the
form
(some number)", where
n is the
generation number. (This
is
true
in
general
for
linear difference equations.) Note that
the
magnitude
of a
will determine whether
the
population grows
or
dwindles
with
time.
That
is,
a
a
> 1 M
n
increases over successive generations,
< 1 M
n
decreases over successive generations,
a
= 1 M
n
is
constant.
An
Insect Population
Insects generally have more than
one
stage
in
their
life
cycle
from
progeny
to
matu-
rity. The
complete
cycle
may
take weeks, months,
or
even years. However,
it is
cus-
tomary
to use a
single
generation
as the
basic unit
of
time when attempting
to
write
a
model
for
insect population growth. Several stages
in the
life
cycle
can be
depicted
by
writing several difference equations.
Often
the
system
of
equations condenses
to
a
single
equation
in
which combinations
of all the
basic
parameters appear.
As
an
example
consider
the
reproduction
of the
poplar gall aphid. Adult female
aphids produce galls
on the
leaves
of
poplars.
All the
progeny
of a
single aphid
are
contained
in one
gall (Whitham, 1980). Some
fraction
of
these will emerge
and
sur-
vive
to
adulthood. Although generally
the
capacity
for
producing
offspring
(fecundity)
and the
likelihood
of
surviving
to
adulthood (survivorship) depends
on
their environmental conditions,
on the
quality
of
their
food,
and on the
population
sizes,
let us
momentarily ignore these
effects
and
study
a
naive model
in
which
all
parameters
are
constant.
First
we
define
the
following:
a
n
=
number
of
adult female aphids
in the nth
generation,
p
n
=
number
of
progeny
in the nth
generation,
m =
fractional mortality
of the
young
aphids,
/ =
number
of
progeny
per
female aphid,
r =
ratio
of
female aphids
to
total adult aphids.
Then
we
write equations
to
represent
the
successive
populations
of
aphids
and
use
these
to
obtain
an
expression
for the
number
of
adult females
in the nth
genera-
tion
if
initially there were
a
0
females:
8
Discrete Processes
in
Biology
Equation
(7) is
again
a first-order
linear difference equation,
so
that solution
(8)
fol-
lows
from
previous remarks.
The
expression
fr(1
– m) is the per
capita number
of
adult
females that each mother aphid produces.
1.2
PROPAGATION
OF
ANNUAL PLANTS
Annual
plants produce seeds
at the end of a
summer.
The flowering
plants wilt
and
die, leaving their progeny
in the
dormant
form
of
seeds that must survive
a
winter
to
give rise
to a new
generation.
The
following spring
a
certain fraction
of
these seeds
germinate. Some seeds might remain dormant
for a
year
or
more before reviving.
Others
might
be
lost
due to
predation, disease,
or
weather.
But in
order
for the
plants
to
survive
as a
species,
a
sufficiently
large population must
be
renewed
from
year
to
year.
In
this section
we
formulate
a
model
to
describe
the
propagation
of
annual
plants.
Complicating
the
problem somewhat
is the
fact
that annual plants produce
seeds that
may
stay dormant
for
several years before germinating.
The
problem thus
requires
that
we
systematically
keep
track
of
both
the
plant population
and the re-
serves
of
seeds
of
various ages
in the
seed bank.
Stage
1:
Statement
of the
Problem
Plants produce seeds
at the end of
their growth season (say August),
after
which they
die.
A
fraction
of
these
seeds
survive
the
winter,
and
some
of
these germinate
at the
beginning
of the
season (say May), giving
rise to the new
generation
of
plants.
The
fraction
that germinates depends
on the age of the
seeds.
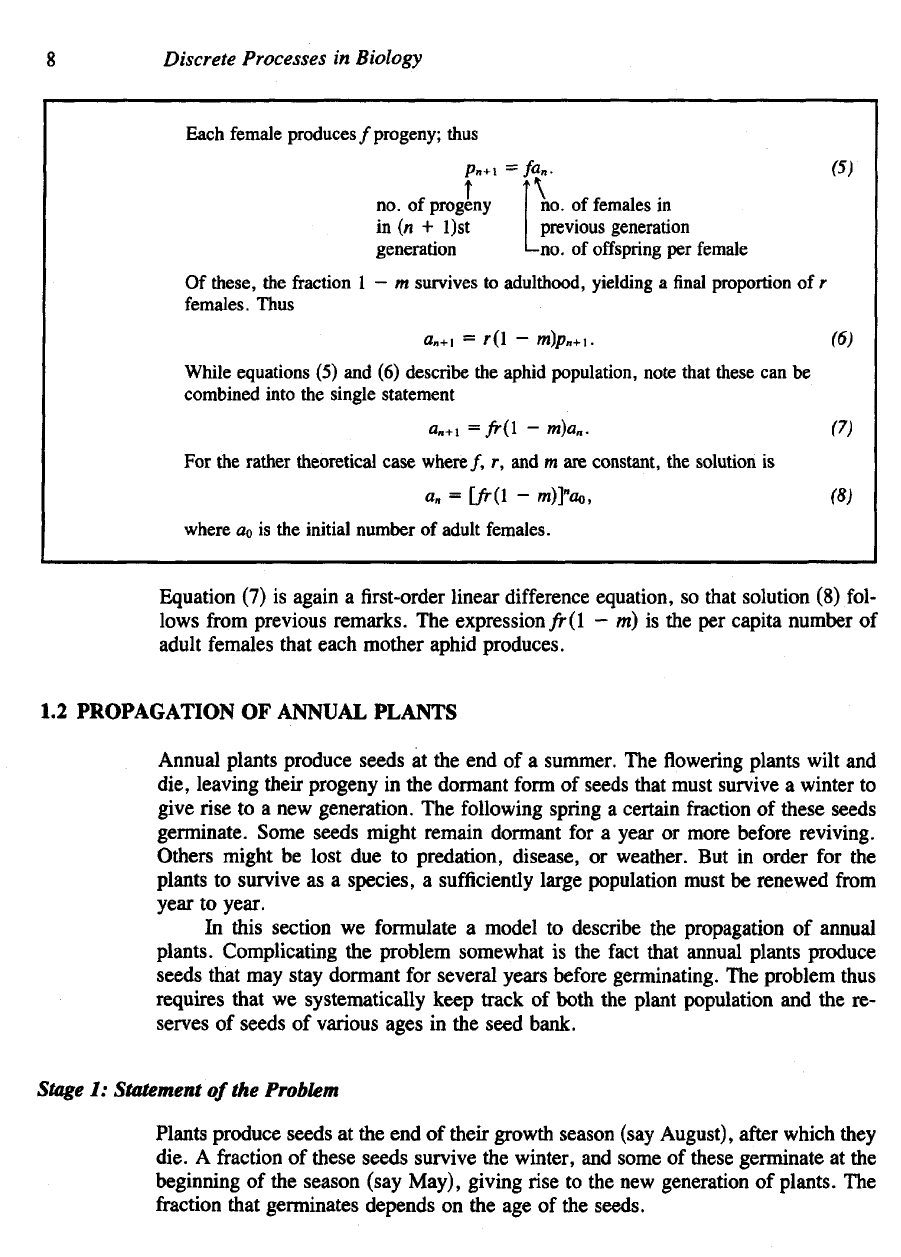
Each female produces
f
progeny; thus
Of
these,
the fraction 1 — m
survives
to
adulthood, yielding
a final
proportion
of r
females.
Thus
While equations
(5) and (6)
describe
the
aphid population, note that these
can be
combined into
the
single statement
For the
rather
theoretical
case
where
f, r, and m are
constant,
the
solution
is
where
a
0
is the
initial number
of
adult females.
The
Theory
of
Linear
Difference
Equations
Applied
to
Population
Growth
9
Stage
2:
Definitions
and
Assumptions
We first
collect
all the
parameters
and
constants specified
in the
problem. Next
we
define
the
variables.
At
that
stage
it
will prove
useful
to
consult
a
rough sketch such
as
Figure 1.2.
Parameters:
r =
number
of
seeds
produced
per
plant
in
August,
a =
fraction
of
one-year-old
seeds that germinate
in
May,
ß = fraction of
two-year-old seeds that germinate
in
May,
a =
fraction
of
seeds that survive
a
given winter.
In
defining
the
variables,
we
note that
the
seed bank changes
several
times
during
the
year
as a
result
of (1)
germination
of
some seeds,
(2)
production
of new
seeds,
and
(3)
aging
of
seeds
and
partial mortality.
To
simplify
the
problem
we
make
the
following assumption:
Seeds
older
than
two
years
are no
longer viable
and can be
neglected.
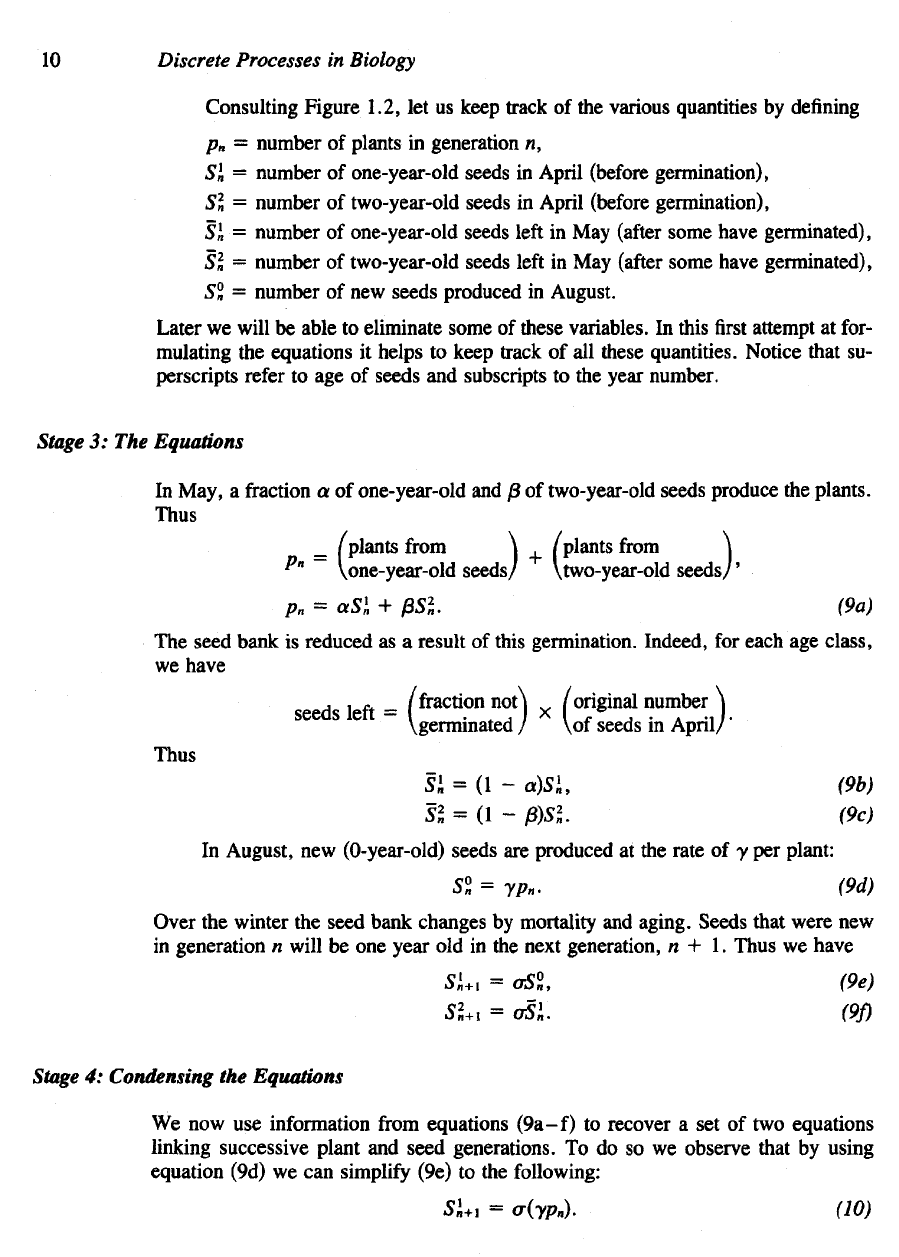
Figure
1.2
Annual plants produce
y
seeds
per
plant
each
summer.
The
seeds
can
remain
in the
ground
for
up to two
years
before
they germinate
in the
springtime.
Fractions
a
of
the
one-year-old
and ß
of
the
two-year-old seeds give rise
to a new
plant
generation. Over
the
winter seeds
age,
and a
certain
proportion
of
them
die.
The
model
for
this
system
is
discussed
in
Section
1.2.
10
Discrete Processes
in
Biology
Consulting Figure 1.2,
let us
keep track
of the
various quantities
by
defining
p
n
=
number
of
plants
in
generation
n,
Sn
=
number
of
one-year-old seeds
in
April (before germination),
S2n
=
number
of
two-year-old seeds
in
April (before germination),
S1n =
number
of
one-year-old seeds
left
in May
(after
some have germinated),
S2n
=
number
of
two-year-old seeds
left
in May
(after
some have germinated),
S0n =
number
of new
seeds produced
in
August.
Later
we
will
be
able
to
eliminate some
of
these variables.
In
this
first
attempt
at
for-
mulating
the
equations
it
helps
to
keep track
of all
these quantities. Notice that
su-
perscripts refer
to age of
seeds
and
subscripts
to the
year number.
Stage
3: The
Equations
In
May,
a
fraction
a of
one-year-old
and ß of
two-year-old seeds produce
the
plants.
Thus
The
seed
bank
is
reduced
as a
result
of
this germination.
Indeed,
for
each
age
class,
we
have
Thus
In
August,
new
(0-year-old) seeds
are
produced
at the
rate
of y per
plant:
Over
the
winter
the
seed
bank changes
by
mortality
and
aging.
Seeds
that were
ne
in
generation
n
will
be one
year
old in the
next generation,
n + 1.
Thus
we
have
Stage
4:
Condensing
the
Equations
We
now use
information
from
equations (9a–f)
to
recover
a set of two
equations
linking
successive plant
and
seed
generations.
To do so we
observe that
by
using
equation (9d)
we can
simplify (9e)
to the
following:
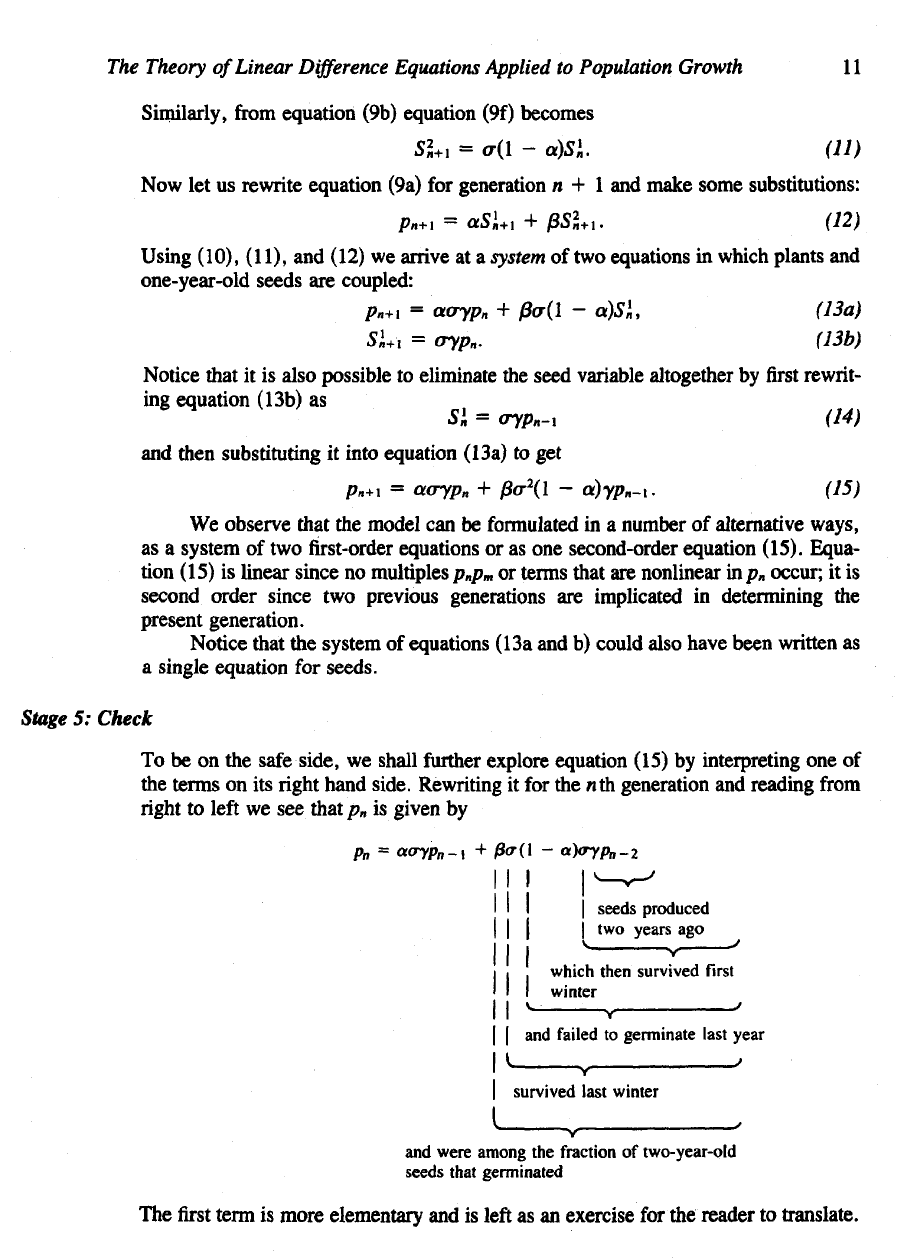
The
Theory
of
Linear
Difference
Equations
Applied
to
Population
Growth
11
Sim rly,
from
equation (9b) equation (9f) becomes
Now
let us
rewrite equation (9a)
for
generation
n + 1 and
make some substitutions:
Using
(10), (11),
and
(12)
we
arrive
at a
system
of two
equations
in
which plants
and
one-year-old seeds
are
coupled:
Notice that
it is
also
possible
to
eliminate
the
seed
variable altogether
by first
rewrit-
ing
equation (13b)
as
and
then substituting
it
into equation (13a)
to get
We
observe that
the
model
can be
formulated
in a
number
of
alternative ways,
as
a
system
of two
first-order equations
or as one
second-order equation (15). Equa-
tion
(15)
is
linear since
no
multiples
p
n
p
m
or
terms that
are
nonlinear
in p
n
occur;
it is
second order since
two
previous generations
are
implicated
in
determining
the
present generation.
Notice that
the
system
of
equations (13a
and b)
could also have been written
as
a
single equation
for
seeds.
Stage
5:
Check
To be on the
safe
side,
we
shall
further
explore equation (15)
by
interpreting
one of
the
terms
on its right
hand side. Rewriting
it for the nth
generation
and
reading
from
right to
left
we see
that
p
n
is
given
by
The first
term
is
more elementary
and is
left
as an
exercise
for the
reader
to
translate.

12
Discrete Processes
in
Biology
1.3
SYSTEMS
OF
LINEAR DIFFERENCE EQUATIONS
The
problem
of
annual plant reproduction leads
to a
system
of two first-order
differ-
ence equations (10,13),
or
equivalently
a
single second-order equation (15).
To un-
derstand
such equations,
let us
momentarily turn
our
attention
to a
general
system
of
the
form
As
before, this
can be
converted
to a
single higher-order equation. Starting
with
(16a)
and
using (16b)
to
eliminate y
n+1
,
we
have
From equation (16a),
Now
eliminating
y
n
we
conclude that
or
more simply that
In
a
later chapter, readers
may
remark
on the
similarity
to
situations encountered
in
reducing
a
system
of
ordinary
differential
equations (ODEs)
to
single ODEs (see
Chapter
4). We
proceed
to
discover properties
of
solutions
to
equation (17)
or
equiv-
alently,
to
(16a,
b).
Looking
back
at the
simple
first-order
linear difference equation (2),
recall
that
solutions
to it
were
of the
form
While
the
notation
has
been changed slightly,
the
form
is
still
the
same: constant
de-
pending
on
initial conditions times some number raised
to the
power
n.
Could this
type
of
solution work
for
higher-order linear equations such
as
(17)?
We
proceed
to
test this idea
by
substituting
the
expression
x
n
=
Chn"
(in the
form
of
x
n+1
=
Ch
n+1
and
x
n+2
=
CA
n+2
)
into equation (17), with
the
result that
Now
we
cancel
out a
common factor
of
CA".
(It may be
assumed that
CA" = 0
since
x
n
= 0 is a
trivial solution.)
We
obtain
Thus
a
solution
of the
form (18) would
in
fact
work, provided that
A
satisfies
the
quadratic equation (19), which
is
generally called
the
characteristic equation
of
(17).

The
Theory
of
Linear
Difference
Equations
Applied
to
Population Growth
13
To
simplify notation
we
label
the
coefficients appearing
in
equation (19)
as
follows:
The
solutions
to the
characteristic
equation (there
are two of
them)
are
then:
These numbers
are
called eigenvalues,
and
their properties will uniquely determine
the
behavior
of
solutions
to
equation (17).
(Note:
much
of the
terminology
in
this
section
is
common
to
linear algebra;
in the
next section
we
will arrive
at
identical
re-
sults
using matrix notation.)
Equation (17)
is
linear; like
all
examples
in
this chapter
it
contains only scalar
multiples
of the
variables—no quadratic, exponential,
or
other nonlinear expres-
sions.
For
such equations,
the
principle
of
linear
superposition
holds:
if
several
dif-
ferent
solutions
are
known, then
any
linear combination
of
these
is
again
a
solution.
Since
we
have just determined that
A" and A3 are two
solutions
to
(17),
we can
con-
clude that a general solution is
provided
h1 = h
2
.
(See problem
3 for a
discussion
of the
case
h1 =
h
2
.) This
ex-
pression
involves
two
arbitrary
scalars,
A1 and A
2
,
whose values
are not
specified
by
the
difference equation (17) itself. They depend
on
separate constraints, such
as
par-
ticular known values attained
by x.
Note that specifying
any two x
values uniquely
determines
A1 and A
2
.
Most commonly,
xo and x1, the
levels
of a
population
in the
first two
successive generations,
are
given
(initial
conditions)',
A1 and A
2
are
deter-
mined
by
solving
the two
resulting linear algebraic equations (for
an
example
see
Section 1.7).
Had we
eliminated
x
instead
of y
from
the
system
of
equations (16),
we
would have obtained
a
similar result.
In the
next section
we
show that general
so-
lutions
to the
system
of first-order
linear equations (16) indeed take
the
form
The
connection between
the
four
constants
A1, A
2
, B1, and B
2
will then
be
made
clear.
1.4 A
LINEAR ALGEBRA REVIEW
4
Results
of the
preceding
section
can be
obtained
more directly
from
equations (16a,
b)
using linear algebra techniques. Since these
are
useful
in
many situations,
we
will
briefly
review
the
basic
ideas.
Readers
not
familiar with matrix notation
are
encour-
4. To the
instructor: Students unfamiliar with linear algebra and/or complex numbers
can
omit
Sections
1.4 and 1.8
without
loss
of
continuity.
An
excellent
supplement
for
this chapter
is
Sherbert
(1980).
14
Discrete Processes
in
Biology
aged
to
consult Johnson
and
Riess (1981), Bradley (1975),
or any
other elementary
linear
algebra
text.
Recall
that
a
shorthand
way of
writing
the
system
of
algebraic linear equations,
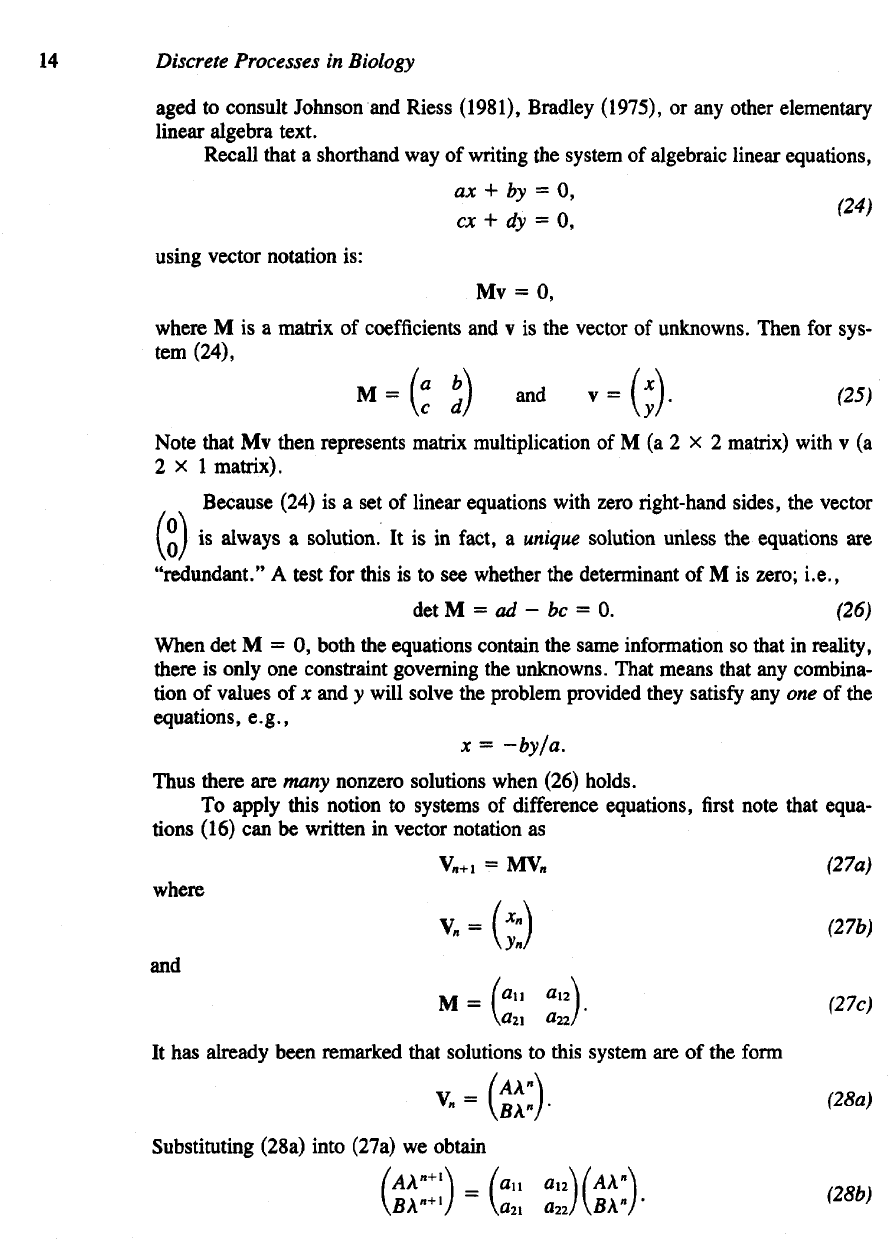
using vector notation
is:
where
M is a
matrix
of
coefficients
and v is the
vector
of
unknowns. Then
for
sys-
tem (24)
Note that
Mv
then represents matrix multiplication
of M (a 2 x 2
matrix)
with
v (a
2
x 1
matrix).
Because (24)
is a set of
linear equations with zero
right-hand
sides,
the
vector
(00
1 is
always
a
solution.
It is in
fact,
a
unique solution unless
the
equations
are
"redundant."
A
test
for
this
is to see
whether
the
determinant
of M is
zero; i.e.,
When
det M = 0,
both
the
equations contain
the
same information
so
that
in
reality,
there
is
only
one
constraint governing
the
unknowns. That means that
any
combina-
tion
of
values
of x and y
will solve
the
problem provided they
satisfy
any one of the
equations, e.g.,
Thus there
are
many nonzero solutions when (26) holds.
To
apply this notion
to
systems
of
difference equations,
first
note that equa-
tions (16)
can be
written
in
vector notation
as
where
and
It has
already been remarked that solutions
to
this system
are of the
form
Substituting (28a) into (27a)
we
obtain
The
Theory
of
Linear
Difference
Equations
Applied
to
Population Growth
15
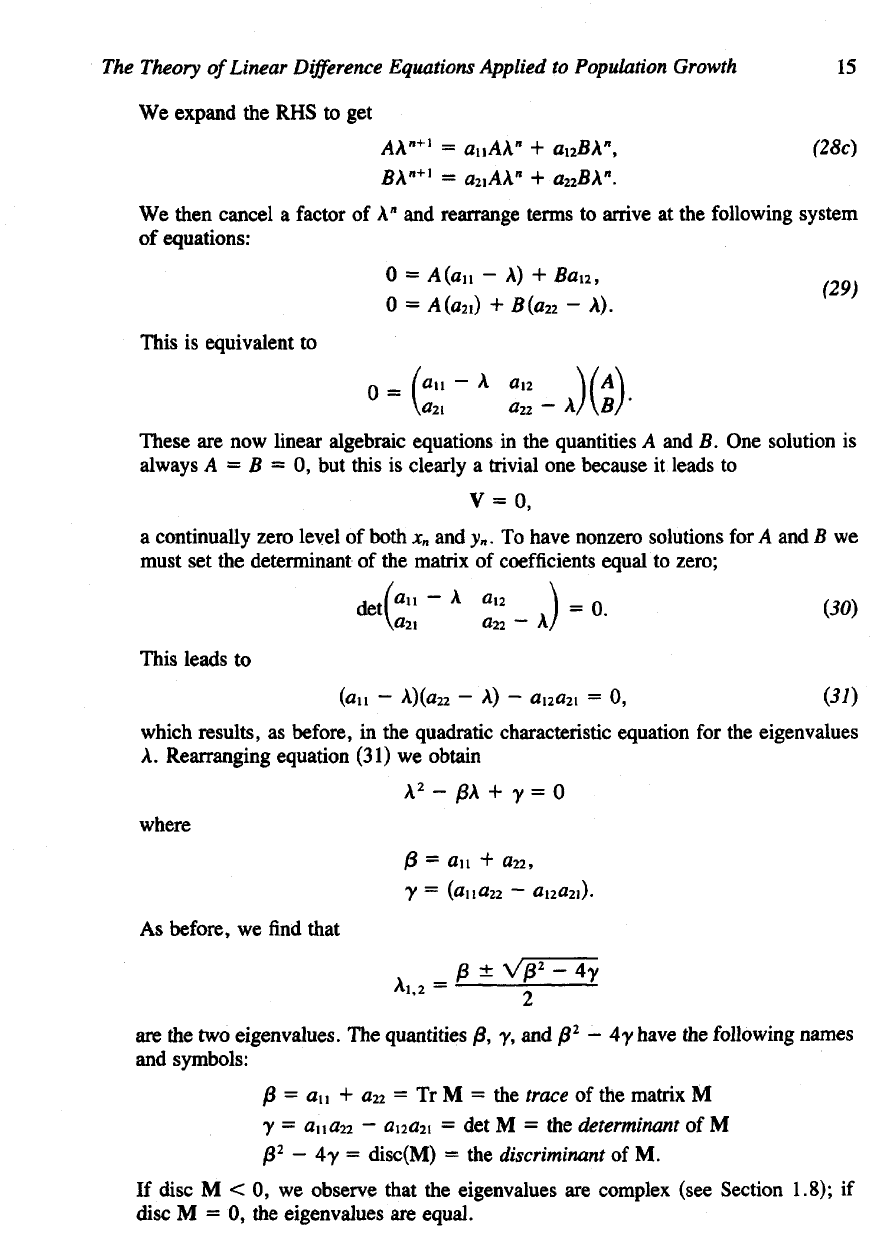
We
expand
the RHS to get
We
then ca l
a
factor
of A" and
rearrange terms
to
arrive
at the
following system
of
equations:
This
is
equivalent
to
These
are now
linear algebraic equations
in the
quantities
A and B. One
solution
is
always
A = B = 0, but
this
is
clearly
a
trivial
one
because
it
leads
to
a
continually zero level
of
both
x
n
and V
n
. To
have nonzero solutions
for A and B we
must
set the
determinant
of the
matrix
of
coefficients equal
to
zero;
This leads
to
which
results,
as
before,
in the
quadratic characteristic equation
for the
eigenvalues
A.
Rearranging equation (31)
we
obtain
re
As
before,
we find
that
are
the two
eigenvalues.
The
quantities
ß, r and ß
2
- 4y
have
the
following names
and
symbols:
ß = a11 + a22 = Tr M = the
trace
of the
matrix
M
y —
a11a22
–
a12a21
= det M = the
determinant
of M
j8
2
— 4y =
disc(M)
= the
discriminant
of M.
If
disc
M < 0, we
observe that
the
eigenvalues
are
complex (see Section 1.8);
if
disc
M = 0, the
eigenvalues
are
equal.
