Dong S.-H. Wave Equations in Higher Dimensions

Подождите немного. Документ загружается.

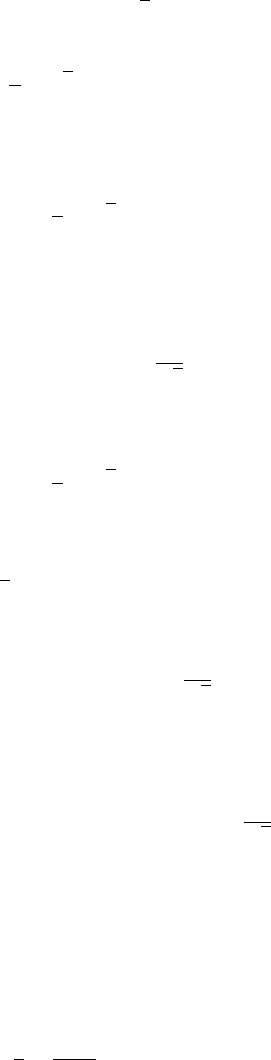
104 8 Wavefunction Ansatz Method

With the same reason, we choose α =−

√

2. Likewise, if a

= 0, but a

p+1

p+2

=···=0, we can obtain A

=0, which leads to the following relation

√

2(κ +1 +2p). (8.59)

To expound this method, we will give the exact solutions for p =0, 1 as follows.

(1): when p =0, it is easy to obtain

√

2(κ +1). (8.60)

On the other hand, it is shown from Eq. (8.15) that B

= 0, which means

λ =0. This refers to harmonic oscillator case. The eigenfunction for p =0 can

be simply written as

(0)

(r) =a

exp



−

√



, (8.61)

where a

is the expansion constant.

(2): when p =1, the eigenvalue can be obtained as

√

2(κ +3). (8.62)

In this case, it is shown from B

−A

=0 that a constraint on the potential

parameters and κ is given by

√

2g +2g

(1 +κ) +λ = 0. (8.63)

The eigenfunction for p =1 can be read as

(1)

(r) =(a

exp



−

√



, (8.64)

where the a

and a

are the expansion constants. Generally, if a

= 0, but

p+1

p+2

=···=0, we can obtain A

=0. The eigenfunction can be writ-

ten as

(p)

(r) =(a

+···+a

exp



−

√



, (8.65)

where a

(i =0, 1, 2,...,p) are the expansion constants.

6 Screened Coulomb Potential

For this potential

V(r)=

r +λ

,a<−b, (8.66)

6 Screened Coulomb Potential 105

take the following ansatz

U(r)=e

βr



n=0

(r +λ)r

n+κ+1/2

. (8.67)

Substituting this into Eq. (8.4) and setting the coefﬁcient of r

n+κ+1/2

to zero, we

have

n+1

n+2

=0, (8.68)

where

=−2a −2b +(3 +2n +2κ)β,

=2(1 +βλ)(n +κ +1/2) −2aλ +n(n +2κ),

=λn(n +2κ),

(8.69)

and

+2E = 0. (8.70)

BasedonthisEq.(8.70)wetake

β =−

√

−2E. (8.71)

This is required by the physically acceptable solution. On the other hand, if a

=0,

but a

p+1

p+2

p+3

=···=0, it is easy to obtain A

=0, i.e.,

=−

(a +b)

2(3/2 +κ +p)

. (8.72)

To show this method, we present exact solutions for p =0 and 1 below.

(1): when p =0, it is found from Eq. (8.72) that

=−

(a +b)

2(3/2 +κ)

. (8.73)

The restriction on the parameters of the potential and κ can be obtained from

=0as

(κ +1/2)(κ +3/2) =λ(2aκ +bκ +2a +b/2). (8.74)

The eigenfunction for p =0 now becomes

(0)

(r) =a

exp



−



−2E



(r +λ), (8.75)

where a

is the expansion constant.

(2): when p =1, one can obtain the eigenvalue as follows:

=−

(a +b)

2(5/2 +κ)

. (8.76)

The restriction on the parameters of the potential and κ can be obtained from

. The eigenfunction for p =1 becomes

(1)

(r) =(a

r)r

exp



−



−2E



(r +λ), (8.77)

106 8 Wavefunction Ansatz Method

where the a

and a

are expansion constants. For a given p,ifa

= 0, but

p+1

p+2

=···=0, we have A

=0. The eigenfunction can be written as

(p)

=(a

r +···+a

exp



−



−2E



(r +λ), (8.78)

where a

(i =0, 1, 2,...,p) are the expansion constants.

7MorsePotential

Due to its mathematical advantages, the harmonic oscillator model has been widely

used to describe the interaction force of the diatomic molecule. Nevertheless, it is

well known that the real molecular vibrations are anharmonic. Among many molec-

ular potentials, the Morse potential as an ideal and typical anharmonic potential

permits an exactly mathematical treatment and has been the subject of interest since

1929 [244]. In particular, the Morse potential will reduce to the harmonic oscillator

in the harmonic limit.

Choose the separated atoms limit as the zero-point energy. The Morse potential

has the following form

V(r)=V

−2βr

−2e

−βr

), (8.79)

where V

> 0 corresponds to its depth, β is related to the range of the potential, and

r gives the relative distance from the equilibrium position of the atoms.

Following approach [245] and our recent work [246], we can take the ansatz for

the wavefunction

R(r) =r

−br

G(r), b =

√

−2E, E < 0. (8.80)

Substitution of this into (8.4) leads to

G(r)

+(2τr

τ −1

−2br

)

dG(r)



τ(τ −1)r

τ −2

−2bτr

τ −1

+(b

+2E −2V (r))r

−



−



τ −2



G(r) =0, (8.81)

which is re-arranged as

[τ(τ −1) −(κ

−1/4)]G(r) +



2τ

dG(r)

−2bτG(r)





G(r)

−2b

dG(r)

−2V(r)G(r)



=0. (8.82)

From the behavior of the wavefunction at the origin, it is shown from Eqs. (8.81)

and (8.82) that

τ =κ +

=l +

D −1

, (8.83)

where another solution τ =1/2 −κ is ignored in physics.

7 Morse Potential 107

Asshownin[246], we expand the Morse potential about the origin as follows:

V(r)=2V

∞



l=0

, (8.84)

where we have used an important formula

−2βr

−2e

−βr

=(e

−βr

−1)

−1

∞



l=0

(−1)

l−1

−1)

∞



l=0

. (8.85)

We take the standard series for G(r) with the form

G(r) =

∞



k=0

,γ

=0. (8.86)

Substituting of this, together with Eq. (8.85), into Eq. (8.82) and setting the coefﬁ-

cients of the powers of r

(n =l +k +2) to zero, one can obtain

∞



k=0

[k(2κ +k)]γ

−

∞



k=0

2b(k +κ +1/2)γ

k+1

−4V

∞



k=0

∞



l=0

l+k+2

=0,

(8.87)

from which we have

=bγ

b(2κ +3)γ

+4V

4 +4κ

b(2κ +5)γ

+4V

(γ

+γ

)

9 +6κ

(8.88)

Similarly continuing to use Eq. (8.87), we can ﬁnally obtain the expansion coefﬁ-

cients γ

b(2κ +2n −1)γ

n−1

+4V

n,k

n(2κ +n)

, (8.89)

with

n,k

n−2=N



l=0,l≥2

N−l

. (8.90)

Accordingly, we can ﬁnally obtain the analytical solutions of the Schrödinger

equation with the Morse potential in arbitrary dimensions as

R(r) =r

1/2+κ

−br

∞



n=0

,γ

=0. (8.91)

108 8 Wavefunction Ansatz Method

Thus, for a given Morse potential, we can always ﬁnd the suitable eigenvalue to

make the wavefunction R(r) convergent when r tends to inﬁnity.

8 Conclusions

In this Chapter we have carried out the solutions of the D-dimensional radial

Schrödinger equation with some anharmonic potentials applying an ansatz to the

wavefunction and obtained the restrictions on the parameters of the potential and κ.

These potentials include the sextic potential, singular one fraction power potential,

mixture potential, non-polynomial potential, screened Coulomb potential and Morse

potential. Before ending this Chapter, we give a useful remark on this method. As

far as all insoluble physical quantum systems, we have to treat them by different

approximate methods. By using present wavefunction ansatz method, one is able to

only obtain some so-called eigenfunctions with some constraints on the potential

parameters and quantum numbers.

Chapter 9

The Levinson Theorem for Schrödinger

Equation

1 Introduction

The Levinson theorem [109] as an important theorem in quantum scattering theory

establishes the relation between the total number n

of bound states and the phase

shift δ

(0) of the scattering states at zero momentum. For the Schrödinger equation

with a spherically symmetric potential V(r), the short-range central potential V(r)

satisﬁes the boundary conditions



r|V(r)|dr < ∞,



∞

|V(r)|dr < ∞.

(9.1)

They are required for the nice behavior of the wavefunction at the origin and the

analytic property of the Jost function, respectively.

Since 1949 the Levinson theorem has been proved by different methods and gen-

eralized to different equations and potentials [184, 247–296]. With the recent inter-

est in higher-dimensional ﬁeld theory, we attempt to establish the Levinson theorem

for the D-dimensional Schrödinger equation by the Sturm-Liouville theorem.

This Chapter is organized as follows. We study the scattering states and bound

states in Sects. 2 and 3, respectively. The Sturm-Liouville theorem is carried out

in Sect. 4. The non-relativistic Levinson theorem in D dimensions is established

in Sect. 5. The general case will be discussed in Sect. 6. In Sect. 7 we present the

comparison theorem. We summarize our conclusions in Sect. 8.

2 Scattering States and Phase Shifts

Let us consider the D-dimensional Schrödinger equation



−





+V(r)



(r) =E(r), (9.2)

S.-H. Dong, Wave Equations in Higher Dimensions,

DOI 10.1007/978-94-007-1917-0_9, © Springer Science+Business Media B.V. 2011

109

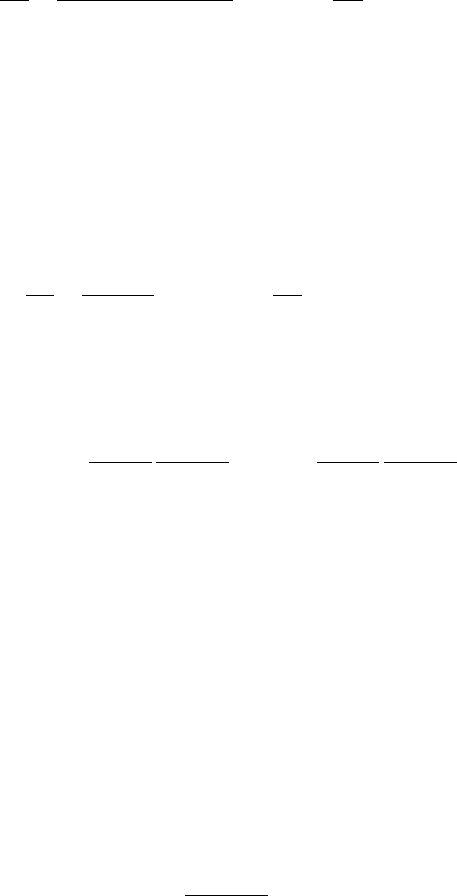
110 9 The Levinson Theorem for Schrödinger Equation

which keeps invariant in spatial rotation. Therefore, choose the wavefunction as

(r) =r

−(D−1)/2

(r)Y

D−1

...l

(

x). (9.3)

By separating the angular variables from the wavefunction we obtain the radial

Schrödinger equation as



−

(l −1 +D/2)

−1/4



(r) =−



{E −V(r)}R

(r), (9.4)

which is a real equation so that we only discuss its real solutions.

For simplicity, we ﬁrst study Eq. (9.4) with a cutoff potential

V(r)=0, when r ≥r

, (9.5)

where r

is a sufﬁciently large radius. The general case will be studied in Sect. 6.

Similar to previous works [290, 291], we introduce a parameter λ for V(r)

V(r,λ)=λV (r), V (r, 1) =V(r). (9.6)

Thus, Eq. (9.4) can be modiﬁed as



∂

∂r

−

−1/4



(r, λ) =−



[E −V(r,λ)]R

(r, λ), (9.7)

where η =|l −1 +D/2| is the same as κ given in Eq. (5.4) for difference. As you

see below, we use κ to denote the energy.

We now solve this equation in two regions and match the logarithmic derivative

of the radial function at r

(E, λ) ≡



(r, λ)

∂R

(r, λ)

∂r



r=r

−



(r, λ)

∂R

(r, λ)

∂r



r=r

. (9.8)

From (9.1) there exists a convergent solution to Eq. (9.7) at the region [0,r

]. When

V(r,0) =0 this solution is given by

(r, 0) =(πkr/2)

1/2

(kr), (9.9)

when E>0 and k =(2ME)

1/2

/,

(r, 0) =e

−iηπ/2

(πκr/2)

1/2

(iκr), (9.10)

when E ≤ 0 and κ = (−2ME)

1/2

/. J

(x) is the Bessel function and R

(r, 0) in

Eqs. (9.9) and (9.10) is a real function. A multiplied factor on R

(r, 0) is unimpor-

tant.

In the region (r

, ∞),wehaveV(r,λ)=0. When E>0 the combination of two

oscillatory solutions to Eq. (9.7) can always satisfy Eq. (9.8) so that there exists a

continuous spectrum

(r, λ) =(π kr/2)

1/2

[cosδ

(k, λ)J

(kr) −sinδ

(k, λ)N

(kr)]

∼cos



kr −

(2η −1)π

+δ

(k, λ)



, when r →∞, (9.11)

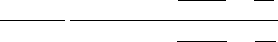
3 Bound States 111

where N

(kr) is the Neumann function. Although V(r,λ)does not depend on λ in

the region (r

, ∞), through Eq. (9.8), R

(r, λ) and the phase shift δ

(k, λ) depend

on λ. In fact, from Eq. (9.8) we can obtain

tanδ

(k, λ) =

(kr

)

(kr

)

(E, λ) −k



(kr

)

(kr

)

−

(E, λ) −k



(kr

)

(kr

)

−

, (9.12)

(k) ≡δ

(k, 1), (9.13)

where the prime denotes the derivative of the Bessel function, the Neumann func-

tion, and later the Hankel function with respect to their arguments. It is found from

Eq. (9.12) that the δ

(k, λ) is determined up to a multiple of π due to the period of

the tangent function. As usual, we may use the convention that

(k) =0, when V(r)=0, (9.14)

which implies that δ

(∞) =0 as deﬁned in [1].

3 Bound States

Because when E ≤0 there is only one convergent solution to Eq. (9.7) in the region

r>r

,Eq.(9.7) is not always satisﬁed

(r, λ) =e

i(η+1)π/2

(πκr/2)

1/2

(1)

(iκr) ∼e

−κr

, when r →∞, (9.15)

where H

(1)

(x) is the Hankel function of the ﬁrst kind. Actually, R

(r, λ) in

Eq. (9.15) does not depend on λ. The matching condition (9.8) may be satisﬁed

only for some discrete energy E, where a bound state appears. Therefore, there ex-

ists a discrete spectrum for E ≤0.

It is worth paying attention to the solutions with E =0. If A

(0, 1) (zero momen-

tum and λ =1) is equal to (1 −2η)/(2r

), it matches a solution of zero energy

(r, 1) =r

(1−2η)/2

<r<∞, (9.16)

from which we know the solution describes a bound state for l>2 −D/2 and a half

bound state for l ≤2 −D/2.

4 The Sturm-Liouville Theorem

Since Eq. (9.7) is a Sturm-Liouville type equation, then it must satisfy the Sturm-

Liouville theorem. For this problem, it is known that the logarithmic derivative of

wavefunction is monotonic with respect to the energy [297]. Due to this property,

the Sturm-Liouville theorem has become a powerful tool in proving the Levinson

theorem.

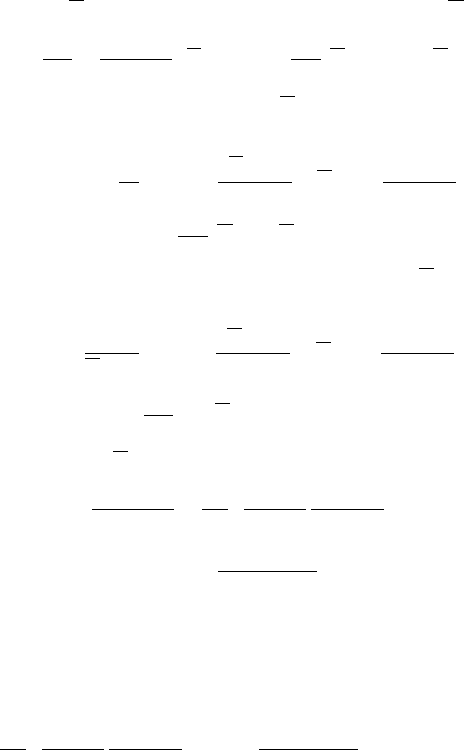
112 9 The Levinson Theorem for Schrödinger Equation

Denote by R

(r, λ) the solution to Eq. (9.7) for the energy E



∂

∂r

−

−1/4



(r, λ) =−



[E −V(r,λ)]R

(r, λ). (9.17)

Multiplying Eq. (9.7) and Eq. (9.17)by

(r, λ) and R

(r, λ), respectively and cal-

culating their difference, we have

∂

∂r



(r, λ)

∂

(r, λ)

∂r

−

(r, λ)

∂R

(r, λ)

∂r



=−



(E −E)R

(r, λ)R

(r, λ). (9.18)

From the boundary condition, both solutions R

(r, λ) and R

(r, λ) vanish at the ori-

gin. Integrating Eq. (9.18) from 0 to r

, we obtain

E −E



(r, λ)

∂

(r, λ)

∂r

−

(r, λ)

∂R

(r, λ)

∂r



r=r

−

=−



(r, λ)R

(r, λ)dr. (9.19)

By taking the limit

E →E,wehave

∂A

(E, λ)

∂E

∂

∂E



(r, λ)

∂R

(r, λ)

∂r



r=r

−

=−



,λ)



(r, λ)

dr < 0. (9.20)

When E = 

/(2M) is larger than zero and tends to zero, we have

(E, λ) =A

(0,λ)−c

+···,c

≤0. (9.21)

Similarly, from the boundary condition that R

(r, λ) tends to zero at inﬁnity, we

have

∂

∂E



(r, λ)

∂R

(r, λ)

∂r



r=r



,λ)



∞

(r, λ)

dr > 0. (9.22)

This is another form of the Sturm-Liouville theorem [297]. As E increases, the

logarithmic derivative of the radial function at r

−

decreases monotonically, but

that at r

for E ≤ 0 increases monotonically. When E ≤ 0 because both sides of

Eq. (9.8) are monotonic as energy changes, the variety of the A

(0,λ) determines

the number of bound states as λ changes.

5 The Levinson Theorem

In this section we apply the Sturm-Liouville theorem to show that both phase shifts

and the number of bound states are related with the variety of the logarithmic deriva-

tive A

(0,λ).

5 The Levinson Theorem 113

We ﬁrst study relation between the n

and the A

(0,λ) when the potential

changes. From the Sturm-Liouville theorem, when E ≤ 0 the logarithmic deriva-

tive of the radial function at r

is monotonic with respect to E.FromEq.(9.15)we

obtain the logarithmic derivative



(r, λ)

∂R

(r, λ)

∂r



r=r

iκH

(1)

(iκr

)



(1)

(iκr

)

−



1−2η

, when E ∼0,

−∞, when E →−∞,

(9.23)

which does not depend on λ. On the other hand, when λ = 0 we obtain from

Eq. (9.10)

(E, 0) =



(r, 0)

∂R

(r, 0)

∂r



r=r

−

iκJ



(iκr

)

(iκr

)

−



2η+1

, when E ∼0,

∞, when E →−∞.

(9.24)

It is evident from Eqs. (9.23) and (9.24) that there is no overlap between two variant

ranges of two logarithmic derivatives when λ = 0 and as the energy increases from

−∞ to 0. Thus, there is no bound state except for the case of l = 0 and D = 2,

where a half bound state occurs at E =0.

Second, we study the relation between the δ

(0,λ) and the A

(0,λ) when the po-

tential changes. The δ

(0,λ) is the limit of the δ

(k, λ) as k tends to zero. Therefore,

we are interested in the δ

(k, λ) at a sufﬁciently small momentum k, i.e., k 1/r

In this case we obtain from Eq. (9.12)

tanδ

(k, λ) =

−π(kr

)

2η

(η +1)(η)

(l, λ) −

2η+1

(0,λ)−c

−

1−2η

[1 −

(kr

)

(2η−1)(η−1)

]

, (9.25)

for l>2 −D/2,

tanδ

(k, λ) =

−π(kr

)

(0,λ)−

(0,λ)−c

[1 +2(kr

)

ln(kr

)]

(9.26)

for l = 2 −D/2(D =4 and l =0orD =2 and l =1),

tanδ

(k, λ) =−(kr

)

(0,λ)−

(0,λ)−c

, (9.27)

for l = (3 −D)/2(D =3 and l =0), and

tanδ

(k, λ) =

2ln(kr

)

(0,λ)−c

−

[1 −(kr

)

]

(0,λ)−c

−

[1 +

ln(kr

)

]

, (9.28)

for l = 1 −D/2(D =2 and l =0).