Damodaran A. Applied corporate finance
Подождите немного. Документ загружается.


11
11
IV. Annuity Given Future Value
Individuals or businesses who have a fixed obligation to meet or a target to meet (in
terms of savings) some time in the future need to know how much they should set aside
each period to reach this target. If you are given the future value and are looking for an
annuity - A(FV,r,n) in terms of notation:
!
Annuity given Future Value = A(FV,r, n) = FV
r
(1 + r)
n
- 1
"
#
$
%
&
'
In any balloon payment loan, only interest payments are made during the life of
the loan, while the principal is paid at the end of the period. Companies that borrow
money using balloon payment loans or conventional bonds (which share the same
features) often set aside money in sinking funds during the life of the loan to ensure that
they have enough at maturity to pay the principal on the loan or the face value of the
bonds. Thus, a company with bonds with a face value of $100 million coming due in 10
years would need to set aside the following amount each year (assuming an interest rate
of 8%):
!
Sinking Fund Provision each year = $100,000,000
.08
(1.08)
10
- 1
"
#
$
%
&
'
= $6,902,950
The company would need to set aside $6.9 million at the end of each year to ensure that
there are enough funds ($10 million) to retire the bonds at maturity.
V. Effect Of Annuities At The Beginning Of Each Year
The annuities considered thus far in this chapter are end-of-the-period cash flows.
Both the present and future values will be affected if the cash flows occur at the
beginning of each period instead of the end. To illustrate this effect, consider an annuity
of $ 100 at the end of each year for the next 4 years, with a discount rate of 10%.
0 1 2 3
..
..
.
4
$ 100 $ 100 $ 100$ 100
10% 10% 10%10%
Contrast this with an annuity of $100 at the beginning of each year for the next four
years, with the same discount rate.

12
12
0 1 2 3 4
$ 100 $ 100 $ 100$ 100
10% 10% 10%10%
Since the first of these annuities occurs right now, and the remaining cash flows take the
form of an end-of-the-period annuity over 3 years, the present value of this annuity can
be written as follows:
!
PV of $100 at beginning of each of next 4 years = $100 + $100
1 -
1
(1.10)
3
.10
"
#
$
$
$
$
%
&
'
'
'
'
In general, the present value of a beginning-of-the-period annuity over n years can be
written as follows:
!
PV of Beginning of Period Annuities over n years = A + A
1 -
1
(1 + r)
n -1
r
"
#
$
$
$
$
%
&
'
'
'
'
This present value will be higher than the present value of an equivalent annuity at the
end of each period.
The future value of a beginning-of-the-period annuity typically can be estimated
by allowing for one additional period of compounding for each cash flow:
!
FV of a Beginning - of - the - Period Annuity = A (1 + r)
(1 + r)
n
- 1
r
"
#
$
%
&
'
This future value will be higher than the future value of an equivalent annuity at the end
of each period.
Consider again the example of an individual who sets aside $2,000 at the end of
each year for the next 40 years in an IRA account at 8%. The future value of these
deposits amounted to $ 518,113 at the end of year 40. If the deposits had been made at
the beginning of each year instead of the end, the future value would have been higher:
!
Expected Value of IRA (beginning of year) = $2,000 (1.08)
(1.08)
40
- 1
.08
"
#
$
%
&
'
= $559,562
13
13
As you can see, the gains from making payments at the beginning of each period can be
substantial.
Growing Annuities
A growing annuity is a cash flow that grows at a constant rate for a specified
period of time. If A is the current cash flow, and g is the expected growth rate, the time
line for a growing annuity appears as follows –
0 1 2 3
A(1+g)
2
A(1+g)
3
A(1+g)
nA(1+g)
n
...........
Note that, to qualify as a growing annuity, the growth rate in each period has to be the
same as the growth rate in the prior period.
In most cases, the present value of a growing annuity can be estimated by using
the following formula –
!
PV of a Growing Annuity = A(1 + g)
1 -
(1 + g)
n
(1 + r)
n
r - g
"
#
$
$
$
$
%
&
'
'
'
'
The present value of a growing annuity can be estimated in all cases, but one - where the
growth rate is equal to the discount rate. In that case, the present value is equal to the
nominal sums of the annuities over the period, without the growth effect.
PV of a Growing Annuity for n years (when r=g) = n A
Note also that this formulation works even when the growth rate is greater than the
discount rate.
1
To illustrate a growing annuity, suppose you have the rights to a gold mine for the
next 20 years, over which period you plan to extract 5,000 ounces of gold every year. The
current price per ounce is $300, but it is expected to increase 3% a year. The appropriate
discount rate is 10%. The present value of the gold that will be extracted from this mine
can be estimated as follows:
1
Both the denominator and the numerator in the formula will be negative, yielding a positive present value.

14
14
!
PV of extracted gold = $300 * 5000 * (1.03)
1 -
(1.03)
20
(1.10)
20
.10 - .03
"
#
$
$
$
$
%
&
'
'
'
'
= $16,145,980
The present value of the gold expected to be extracted from this mine is $16.146 million;
it is an increasing function of the expected growth rate in gold prices. Figure 6 illustrates
the present value as a function of the expected growth rate.
Perpetuities
A perpetuity is a constant cash flow at regular intervals forever. The present value
of a perpetuity can be written as
!
PV of Perpetuity =
A
r
where A is the perpetuity. The most common example offered for a perpetuity is a
console bon. A console bond is a bond that has no maturity and pays a fixed coupon.
Assume that you have a 6% coupon console bond. The value of this bond, if the interest
rate is 9%, is as follows:
Value of Console Bond = $60 / .09 = $667

15
15
The value of a console bond will be equal to its face value (which is usually $1000) only
if the coupon rate is equal to the interest rate.
Growing Perpetuities
A growing perpetuity is a cash flow that is expected to grow at a constant rate
forever. The present value of a growing perpetuity can be written as:
!
PV of Growing Perpetuity =
CF
1
(r - g)
where CF
1
is the expected cash flow next year, g is the constant growth rate and r is the
discount rate. While a growing perpetuity and a growing annuity share several features,
the fact that a growing perpetuity lasts forever puts constraints on the growth rate. It has
to be less than the discount rate for this formula to work.
Growing perpetuities are especially useful when valuing equity in publicly traded
firms, since they could potentially have perpetual lives. Consider a simple example. In
1992, Southwestern Bell paid dividends per share of $2.73. Its earnings and dividends
had grown at 6% a year between 1988 and 1992 and were expected to grow at the same
rate in the long term. The rate of return required by investors on stocks of equivalent risk
was 12.23%.
Current Dividends per share = $2.73
Expected Growth Rate in Earnings and Dividends = 6%
Discount Rate = 12.23%
With these inputs, we can value the stock using a perpetual growth model:
Value of Stock = $2.73 *1.06 / (.1223 -.06) = $46.45
As an aside, the stock was actually trading at $70 per share. This price could be justified
by using a higher growth rate. The value of the stock is graphed in figure 7 as a function
of the expected growth rate.
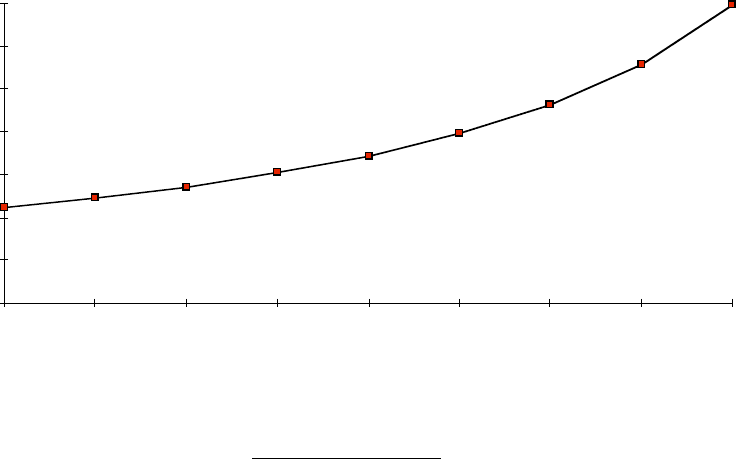
16
16
Figure 3.7: SW Bell -Value versus Expected Growth
$0.00
$10.00
$20.00
$30.00
$40.00
$50.00
$60.00
$70.00
0% 1% 2% 3% 4% 5% 6% 7% 8%
Expected Growth Rate
Value of Stock
The growth rate would have to be approximately 8% to justify a price of $70. This
growth rate is often referred to as an implied growth rate.
Conclusion
Present value remains one of the simplest and most powerful techniques in
finance, providing a wide range of applications in both personal and business decisions.
Cash flow can be moved back to present value terms by discounting and moved forward
by compounding. The discount rate at which the discounting and compounding are done
reflect three factors: (1) the preference for current consumption, (2) expected inflation
and (3) the uncertainty associated with the cash flows being discounted.
In this chapter, we explored approaches to estimating the present value of five
types of cash flows: simple cash flows, annuities, growing annuities, perpetuities, and
growing perpetuities.

1
1
APPENDIX 4
OPTION PRICING
In general, the value of any asset is the present value of the expected cash flows
on that asset. In this section, we will consider an exception to that rule when we will look
at assets with two specific characteristics:
• They derive their value from the values of other assets.
• The cash flows on the assets are contingent on the occurrence of specific events.
These assets are called options, and the present value of the expected cash flows on these
assets will understate their true value. In this section, we will describe the cash flow
characteristics of options, consider the factors that determine their value and examine
how best to value them.
Cash Flows on Options
There are two types of options. A call option gives the buyer of the option the right
to buy the underlying asset at a fixed price, whereas a put option gives the buyer the right
to sell the underlying asset at a fixed price. In both cases, the fixed price at which the
underlying asset can be bought or sold is called the strike or exercise price.
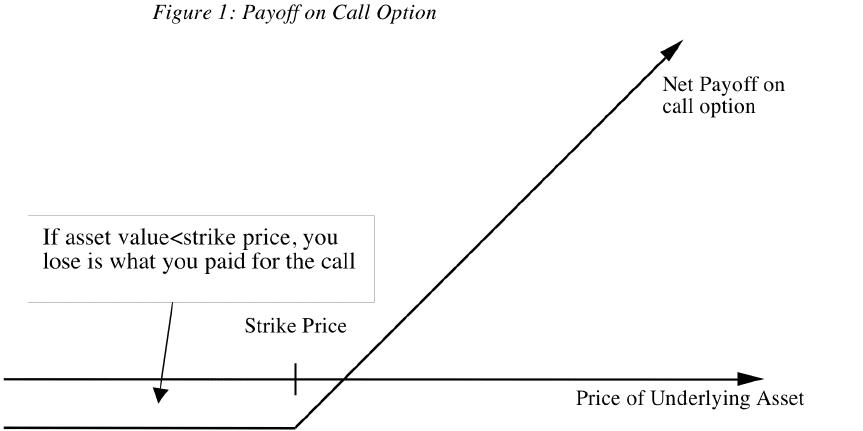
To look at the payoffs on an option, consider first the case of a call option. When
you buy the right to sell an asset at a fixed price, you want the price of the asset to
increase above that fixed price. If it does, you make a profit, since you can buy at the
fixed price and then sell at the much higher price; this profit has to be netted against the
cost initially paid for the option. However, if the price of the asset decreases below the
strike price, it does not make sense to exercise your right to buy the asset at a higher
price. In this scenario, you lose what you originally paid for the option. Figure 1
summarizes the cash payoff at expiration to the buyer of a call option.
2
2
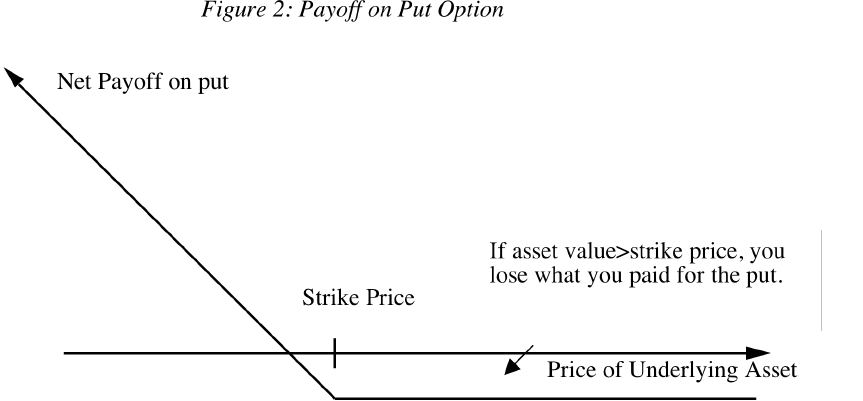
With a put option, you get the right to sell at a fixed price, and you want the price
of the asset to decrease below the exercise price. If it does, you buy the asset at the
exercise price and then sell it back at the current price, claiming the difference as a gross
profit. When the initial cost of buying the option is netted against the gross profit, you
arrive at an estimate of the net profit. If the value of the asset rises above the exercise
price, you will not exercise the right to sell at a lower price. Instead, the option will be
allowed to expire without being exercised, resulting in a net loss of the original price paid
for the put option. Figure 2 summarizes the net payoff on buying a put option.
3
3
With both call and put options, the potential for profit to the buyer is significant, but the
potential for loss is limited to the price paid for the option.
Determinants of Option Value
What is it that determines the value of an option? At one level, options have
expected cash flows just like all other assets, and that may seem like good candidates for
discounted cash flow valuation. The two key characteristics of options -- that they derive
their value from some other traded asset, and the fact that their cash flows are contingent
on the occurrence of a specific event -- does suggest an easier alternative. We can create
a portfolio that has the same cash flows as the option being valued, by combining a
position in the underlying asset with borrowing or lending. This portfolio is called a
replicating portfolio and should cost the same amount as the option. The principle that
two assets (the option and the replicating portfolio) with identical cash flows cannot sell
at different prices is called the arbitrage principle.
Options are assets that derive value from an underlying asset; increases in the
value of the underlying asset will increase the value of the right to buy at a fixed price
and reduce the value to sell that asset at a fixed price. On the other hand, increasing the
strike price will reduce the value of calls and increase the value of puts.
While calls and puts move in opposite directions when stock prices and strike
prices are varied, they both increase in value as the life of the option and the variance in
the underlying asset’s value increases. The reason for this is the fact that options have
4
4
limited losses. Unlike traditional assets that tend to get less valuable as risk is increased,
options become more valuable as the underlying asset becomes more volatile. This is so
because the added variance cannot worsen the downside risk (you still cannot lose more
than what you paid for the option) while making potential profits much higher. In
addition, a longer life for the options just allows more time for both call and put options
to appreciate in value. Since calls provide the right to buy the underlying asset at a fixed
price, an increase in the value of the asset will increase the value of the calls. Puts, on the
other hand, become less valuable as the value of the asset increase.
The final two inputs that affect the value of the call and put options are the
riskless interest rate and the expected dividends on the underlying asset. The buyers of
call and put options usually pay the price of the option up front, and wait for the
expiration day to exercise. There is a present value effect associated with the fact that the
promise to buy an asset for $ 1 million in 10 years is less onerous than paying it now.
Thus, higher interest rates will generally increase the value of call options (by reducing
the present value of the price on exercise) and decrease the value of put options (by
decreasing the present value of the price received on exercise). The expected dividends
paid by assets make them less valuable; thus, the call option on a stock that does not pay
a dividend should be worth more than a call option on a stock that does pay a dividend.
The reverse should be true for put options.
A Simple Model for Valuing Options
Almost all models developed to value options in the last three decades are based
upon the notion of a replicating portfolio. The earliest derivation, by Black and Scholes,
is mathematically complex, and we will return to it in chapter 27. In this chapter, we
consider the simplest replication model for valuing options – the binomial model.
The Binomial Model
The binomial option pricing model is based upon a simple formulation for the
asset price process in which the asset, in any time period, can move to one of two
possible prices. The general formulation of a stock price process that follows the
binomial is shown in Figure 3.
Figure 3: General Formulation for Binomial Price Path
