Cottingham W.N., Greenwood D.A. An Introduction to the Standard Model of Particle Physics
Подождите немного. Документ загружается.


Problems 99
eν
e
→ eν
e
and e
¯
ν
e
→ e
¯
ν
e
). Thus another weak interaction must exist. The experi-
mental investigation of this is difficult because of the smallness of the cross-sections
at the available energies. We shall see from the Standard Model that the effective
interaction Lagrangian required is again of current–current form,
L
int
=
−G
F
√
2
( j
neutral
)
µ
( j
neutral
)
µ
, (9.15)
where, in terms of Dirac spinors,
( j
neutral
)
µ
=
¯
ν
e
γ
µ
1
2
(1 − γ
5
)ν
e
+
¯
ψ
e
γ
µ
(c
V
− c
A
γ
5
)ψ
e
(9.16)
+similar terms for the µ and τ lepton families,
and c
V
and c
A
are parameters. The current is called a neutral current because it
does not induce a change of charge as do the currents (9.2). (Note that it will also
contribute to the scattering eν
e
→ eν
e
.)
Rewriting (9.16) with two-component spinors,
( j
neutral
)
µ
= (ν
eL
)
†
˜σ
µ
ν
eL
+ (c
V
+ c
A
)e
†
L
˜σ
µ
e
L
+(c
V
+ c
A
)e
†
R
σ
µ
e
R
+ similar µ and τ terms. (9.17)
In this form it is evident that right-handed lepton fields as well as left-handed
are involved in the neutral currents. The parameters c
V
and c
A
are related to the
Weinberg angle θ
w
, which appears in the Standard Model, as we shall see in Chapter
12 (equation (12.24)). The subscripts V and A refer, respectively, to the vector and
axial vector nature of the terms in (9.16). (See Section 5.5.)
One might anticipate that neutral currents are also present in atomic physics,
and indeed they are. However, their effects are hard to discern experimentally.
Forexample, they induce parity violation in atoms, but at atomic energies the
weak interaction gives a very small effect. Indeed the decay of an unstable nuclear
or atomic system through the neutral current must always compete with faster
electromagnetic decays, and for this reason neutral current decays in these systems
have never been observed.
Problems
9.1 In the decay of the π
−
at rest, π
−
→ e
−
+
¯
ν
e
, show that
1
2
#
1 −
υ
e
c
$
=
m
2
e
m
2
π
+ m
2
e
.

100 The weak interaction: low energy phenomenology
9.2 Show that the density of final states for the decay of Problem 9.1 is
ρ(E) =
V
(2π)
3
4π p
2
e
d p
e
dE
where V is the normalisation volume and
d p
e
dE
=
E
e
m
π
.
9.3 Obtain the ratio of decay rates given by equation (9.4).
9.4 The term in L
int
describing the decay π
−
→ e
−
+
¯
ν
e
is
L
int
= α
π
e
†
L
˜σ
µ
ν
eL
∂
µ
π
.
Assume that this gives a corresponding term V(0) in the effective Hamiltonian,
V (0) =−α
π
e
†
L
˜σ
µ
ν
eL
∂
µ
π
d
3
x.
(This assumption will be justified in Chapter 12.)
The transition probability per unit time for the decay is to lowest order
2π|e
p
,
¯
ν
p
|V (0)|π
−
(rest)|
2
ρ(E)
where ρ(E)isgiven by Problem 9.2.
Use the free field expansions given in equations (3.35) and (6.24), and Problem
6.5,toevaluate the matrix element above and hence verify equation (9.3).
9.5 Verify the equivalence of the expressions (9.2) and (9.6) for the current j
µ
.
9.6 Taking the effective Lagrangian of Section 9.3, show that the conserved current asso-
ciated with the U(1) symmetry ψ
e
→ e
iα
ψ
e
,ν
e
→ e
iα
ν
e
,isthe electron electron–
neutrino current
j
µ
=
¯
ψ
e
γ
µ
ψ
e
+ ¯ν
e
γ
µ
ν
e
.
Show that the conserved current associated with e
iβ
in the transformations (9.7)
is
¯
ψ
e
γ
µ
ψ
e
+
¯
ψ
µ
γ
µ
ψ
µ
+
¯
ψ
τ
γ
µ
ψ
τ
+ i[(
†
∂
µ
− ∂
µ
†
)
+α
π
( j
µ†
†
− j
µ
)].
Construct the Lagrangian density that results, when the electromagnetic field is
introduced by elevating the global U(1) symmetry of the phase factor e
iβ
into a local
gauge symmetry.
9.7 Estimate G
F
from the expression (9.11) and the experimental lifetime τ
µ
.
9.8 Using a suitable Lorentz invariant, obtain equation (9.13).
Problems 101
9.9 Pick out the term in the effective Lagrangian density (9.8) that contributes to the
scattering
e
−
+ ν
e
→ e
−
+ ν
e
,
and the term in (9.15) that contributes to the scattering
e
−
+ ν
µ
→ e
−
+ ν
µ
.
9.10 The K
−
is like the π
−
,but with an s quark replacing the d. An effective inter-
action with leptons is similar in form to equation (9.1), with
K
replacing
π
and α
K
replacing α
π
. Use the analogue of equation (9.4) to estimate the ratio
τ (K → µ
¯
ν
µ
)/τ (K → e
¯
ν
e
), and compare with the observed value (2.44 ± 0.1) ×
10
−5
(m
K
= 493.68 MeV).
The mean life τ (K
−
→ µ
−
¯
ν
µ
)ismeasured to be 1.948 × 10
−8
s. Estimate α
K
/α
π
.
9.11 Obtain the decay rate (9.5).

10
Symmetry breaking in model theories
In Chapter 9, ‘effective’ weak interaction Lagrangian densities were constructed.
When used in low orders of perturbation theory, these account well for the observed
phenomena at low energies. Difficulties arise in higher order perturbation theory, as
they do in quantum electrodynamics. There is, however, an important difference: it
has been proved that these effective Lagrangian theories cannot be renormalised and
they are therefore unsatisfactory. Furthermore, at higher energies new phenomena
appear, and it is now well established experimentally that the weak interaction is
mediated by the W
+
,W
−
and Z bosons. How are these particles to be incorporated in
a theory of the weak interaction that can be renormalised, and which has the same
seeming inevitability as QED? The answer lies in the Weinberg–Salam unified
theory of the electromagnetic and weak interactions. As an introduction to the
Weinberg–Salam theory we shall in this chapter consider ‘model’ theories, the
mathematics of which is fairly simple, but which contain the basic ideas we shall
need.
10.1 Global symmetry breaking and Goldstone bosons
A possible Lagrangian density for a complex scalar field = (φ
1
+ iφ
2
)/
√
2is
L = ∂
µ
†
∂
µ
− m
2
†
(10.1)
(cf. equation (3.32)).
In this expression (∂
†
/∂t)(∂/∂t) can be regarded as the kinetic energy density
and ∇
†
·∇ + m
2
†
as the potential energy density (see Section 3.3). If is
constant, independent of space and time, the only contribution to the energy is
m
2
†
. Since m
2
is positive this will be a minimum when φ
1
= φ
2
= 0. Thus
= 0 corresponds to the ‘vacuum’ state. Consider now the Lagrangian density
obtained by changing the sign in front of m
2
. This would be unstable: the potential
102
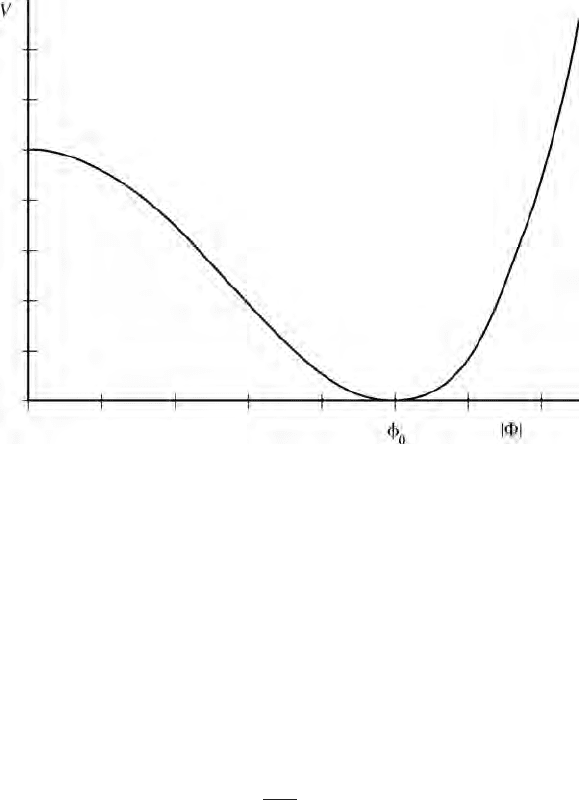
10.1 Global symmetry breaking: Goldstone bosons 103
Figure 10.1 Plot of V = (m
2
/2φ
2
0
)[
∗
− φ
2
0
]
2
as a function of ||; is here a
classical field.
energy density is then unbounded below. Stability can be restored by introducing
a term (m
2
/2φ
2
0
)(
†
)
2
where φ
2
0
is another (real) parameter. For convenience we
add a constant term m
2
φ
2
0
/2, and then
L = ∂
µ
†
∂
µ
− V (
†
)
where
V (
†
) =
m
2
2φ
2
0
†
− φ
2
0
2
. (10.2)
The form of V is shown in Fig. (10.1). The minimum field energy is now obtained
with constant independent of space and time, but such that
†
=||
2
= φ
2
0
.
Such a field is not unique but is defined by a point on the circle ||=φ
0
in the
state space (φ
1
,φ
2
), so that the number of possible vacuum states is infinite.
An analogy with magnetism is helpful. The Hamiltonian describing a
Heisenberg ferromagnet has rotational symmetry: all directions in space are equiv-
alent. However, in its ground state a ferromagnet is magnetised in some particular
direction, which is not determined within the theory, and the rotational symmetry
is lost. This is an example of spontaneous symmetry breaking.

104 Symmetry breaking in model theories
The Lagrangian density (10.2) has a ‘global’ U(1) symmetry: →
=
e
−iα
, L → L
= L, for any real α. Equivalently,
φ
1
= φ
1
cos α + φ
2
sin α,
φ
2
=−φ
1
sin α + φ
2
cos α.
The transformation rotates the state round a circle ||
2
=constant in the state space
(φ
1
,φ
2
). If we pick out the particular direction in (φ
1
,φ
2
) space for which is real,
and take the vacuum state to be (φ
0
, 0), we break the U(1) symmetry.
Expanding about this ground state (φ
0
, 0), we put = φ
0
+ (1/
√
2)(χ + iψ).
The Lagrangian density becomes
L =
1
2
∂
µ
χ∂
µ
χ +
1
2
∂
µ
ψ∂
µ
ψ −
m
2
2φ
2
0
√
2φ
0
χ +
χ
2
2
+
ψ
2
2
2
. (10.3)
After breaking the U(1) symmetry we must interpret the new fields. (In much the
same way, the excited states of a ferromagnet cannot be discussed until the spatial
symmetry has been broken.) In place of the complex field ,wehavetwo coupled
scalar real fields χ and ψ.Wewrite
L = L
free
+ L
int
where
L
free
=
1
2
∂
µ
χ∂
µ
χ − m
2
χ
2
+
1
2
∂
µ
ψ∂
µ
ψ. (10.4)
L
free
represents free particle fields, and contains all the terms in L that are quadratic
in the fields. For classical fields and small oscillations, these terms dominate. The
rest of the Lagrangian density, L
int
, corresponds to interactions between the free
particles and higher order corrections to their motion.
There is a quadratic term −m
2
χ
2
in (10.4), so that the χ field corresponds to
a scalar spin-zero particle of mass
√
2m (by comparison with (3.18)). In the case
of the ψ field there is no such quadratic term: the corresponding scalar spin-zero
particle is therefore massless. The massless particles that always arise as a result of
global symmetry breaking are called Goldstone bosons.
10.2 Local symmetry breaking and the Higgs boson
We now generalise further, and construct a Lagrangian density that is invariant
under a local U(1) gauge transformation,
→
= e
−iqθ
,
10.2 Local symmetry breaking and the Higgs boson 105
where θ = θ(x) may be space and time dependent. This requires the introduction
of a (massless) gauge field A
µ
,asinSection 7.5, and we take
L = [(∂
µ
− iqA
µ
)
†
][(∂
µ
+ iqA
µ
)] −
1
4
F
µν
F
µν
− V (
†
), (10.5)
where F
µν
= ∂
µ
A
ν
− ∂
ν
A
µ
, and again
V (
†
) =
m
2
2φ
2
0
†
− φ
2
0
2
.
L is invariant under the local gauge transformation
(x) →
(x) = e
−iqθ
(x), A
µ
(x) → A
µ
(x) = A
µ
(x) + ∂
µ
θ(x).
A minimum field energy is obtained when the fields A
µ
vanish, and is constant,
defined by a point on the circle ||=φ
0
.Any gauge transformation on this field
configuration is also a minimum. Again we have an infinity of vacuum states.
Given (x), we can always choose θ (x)sothat the field
(x) = e
−iqθ
(x)is
real. This breaks the symmetry, since we are no longer free to make further gauge
transformations.
Putting
(x) = φ
0
+ h(x)/
√
2, where h(x)isreal, gives
L = [(∂
µ
− iqA
µ
)(φ
0
+ h/
√
2)][(∂
µ
+ iqA
µ
)(φ
0
+ h/
√
2)]
−
1
4
F
µν
F
µν
−
m
2
2φ
2
0
√
2φ
0
h +
1
2
h
2
2
. (10.6)
For clarity, we again separate this into
L = L
free
+ L
int
where, dropping the primes on the gauge field,
L
free
=
1
2
∂
µ
h∂
µ
h − m
2
h
2
−
1
4
F
µν
F
µν
+ q
2
φ
2
0
A
µ
A
µ
,
L
int
= q
2
A
µ
A
µ
√
2φ
0
h +
1
2
h
2
−
m
2
h
2
2φ
2
0
√
2φ
0
h +
1
4
h
2
.
(10.7)
Before symmetry breaking, we had a complex scalar field = (φ
1
+ iφ
2
)/
√
2,
and a massless vector field with two polarisation states (Section 4.4). In L
free
we
have a single scalar field h(x) corresponding to a spinless boson of mass
√
2m,
and a vector field A
µ
, corresponding to a vector boson of mass
√
2qφ
0
, with three
independent components (Section 4.9).
This mechanism for introducing mass into a theory was invented by Higgs (1964)
and others (for example Anderson, 1963), and the particle corresponding to the field
h(x) is called a Higgs boson. As a consequence of local symmetry breaking the gauge

106 Symmetry breaking in model theories
field acquires a mass, and the massless spin-zero Goldstone boson that appeared
in our example of global symmetry breaking in Section 10.1 is replaced by the
longitudinal polarised state of this massive spin one boson.
In the Weinberg and Salam ‘electroweak’ theory, the masses of the W
±
and
Z particles arise as a result of symmetry breaking. The resulting theory can be
renormalised, whereas the phenomenological theory of Chapter 9 cannot be renor-
malised. The form of V (
†
) that has been introduced in this chapter appears also
in the electroweak theory. It may seem a somewhat arbitrary feature. However, it
can be shown to be the most general form that can be renormalised.
Problems
10.1 What interaction term in the model Lagrangian density (10.3) allows the massive
boson to decay into two Goldstone bosons? Show that the decay rate in lowest order
perturbation theory is
1
τ (χ → ψψ)
=
m
χ
128π
m
χ
φ
0
2
.
10.2 Show that with the model Lagrangian density (10.7), the vector boson would be
stable, but if the coupling constant q < m/(2φ
0
) the scalar boson would decay into
two vector bosons.
11
Massive gauge fields
In the preceding chapter (Section 10.2), we set up a simple Lorentz invariant
Lagrangian density, which we required to be also invariant under a local U(1)
transformation. This requirement leads to the introduction of a ‘gauge field’ A
µ
.
The system has a degenerate ground state. Breaking the local symmetry results in
the appearance of a vector field carrying mass, together with a scalar Higgs field
also carrying mass. The motivation for introducing mass in this way is that the
subsequent quantum theory can be renormalised. In this chapter we apply the same
idea to a more complicated Lagrangian, which will turn out to have remarkable
physical significance.
11.1 SU(2) symmetry
As a further generalisation, which is basic to the Standard Model, we shall construct
a Lagrangian density that is invariant under a local SU(2) transformation as well as
a local U(1) transformation. The idea was first explored by Yang and Mills (1954).
We introduce a two-component field
=
A
B
, (11.1)
where now
A
and
B
are both complex scalar fields,
A
= φ
1
+ iφ
2
,
B
= φ
3
+ iφ
4
,
giving, in total, four real fields.
If e
−iθ
is any element of the group U(1) and U is any element of the group SU(2)
(discussed in Appendix B), so that U
†
U = UU
†
= 1,werequire the Lagrangian
density to be invariant under the U(1) × SU(2) transformation
→
= e
−iθ
U. (11.2)
107
108 Massive gauge fields
A simple Lagrangian density that has a global U(1) × SU(2) symmetry is
L
= ∂
µ
†
∂
µ
− V (
†
). (11.3)
In terms of the real fields,
†
=
∗
A
A
+
∗
B
B
= φ
2
1
+ φ
2
2
+ φ
2
3
+ φ
2
4
,
∂
µ
†
∂
µ
= ∂
µ
φ
1
∂
µ
φ
1
+ ∂
µ
φ
2
∂
µ
φ
2
+ ∂
µ
φ
3
∂
µ
φ
3
+ ∂
µ
φ
4
∂
µ
φ
4
.
If V (
†
) = m
2
†
, this Lagrangian density corresponds to four independent
free scalar fields, all with the same mass m (cf. (3.18)).
In the Standard Model, the U(1) and SU(2) global symmetries are promoted to
local symmetries. The U(1) transformation may be written
→
= e
−iθ
= exp(−iθτ
0
), (11.4a)
where in this context we write τ
0
for the unit matrix
τ
0
=
10
01
.
For this to become a local symmetry, we must introduce a vector gauge field B
µ
(x)τ
0
with the transformation law
B
µ
(x) → B
µ
(x) = B
µ
(x) + (2/g
1
)∂
µ
θ, (11.4b)
and make the replacement
i∂
µ
→ i∂
µ
− (g
1
/2)B
µ
,
as in Chapter 7. Here the constant g
1
is a dimensionless parameter of the theory,
and the factor 2 follows convention.
Any element of SU(2) can be written in the form
U = exp(−iα
k
τ
k
) (11.5)
where the α
k
are three real numbers and the τ
k
are the three generators of the group
SU(2). The τ
k
are identical to the Pauli spin matrices:
τ
1
=
01
10
,τ
2
=
0 −i
i0
,τ
3
=
10
0 −1
.
For the global SU(2) symmetry to be made into a local SU(2) symmetry, with U =
U(x) dependent on space and time coordinates, we must introduce a vector gauge
field W
µ
k
(x) for each generator τ
k
. The transformation law for the matrices
W
µ
(x) = W
µ
k
(x)τ
k
