Coburn J.W. Algebra and Trigonometry

Подождите немного. Документ загружается.

Increased Student Engagement . . .

▶

Chapter Openers highlight Chapter Connections, an interesting application

exercise from the chapter, and provide a list of other real-world connections

to give context for students who wonder how math relates to them.

▶

Examples throughout the text feature word problems,

providing students with a starting point for how to solve

these types of problems in their exercise sets.

▶

Chapter Openers hi

hli

ht Chapter Connections, an interestin

application

exercise from the chapter and provide a list of other real world connections

Making mathematics meaningful requires that students experience the connection between mathematics

and its impact on the world they live in. This text is also the result of a powerful commitment to

provide applications of the highest quality, having close ties to the examples, and with carefully monitored

levels of difficulty.

Many of these examples were born of my own diverse life experiences, others came from a curious,

lucid, and even visionary folly that allows one to seize upon the every day events of life, and see the

significant or meaningful mathematics in the background. My ever-present notebook was used a

thousand times to capture that casual observation, or that sudden burst of inspiration that is the genesis

for outstanding applications. These were supported at home by a substantial library of reference and

research books, an eye toward both history and current events, and of course our modern marvel of

a research tool—the Internet. After a (sometimes long) period of thought, reflection, and research,

followed by a wording and a rewording of the exercise so that it would resonate with students while

filling the need, a significant and meaningful application was born. —JC

Relations,

Functions, and

Graphs

CHAPTER OUTLINE

2.1 Rectangular Coordinates; Graphing Circles

and Other Relations 152

2.2 Graphs of Linear Equations 165

2.3 Linear Graphs and Rates of Change 178

2.4 Functions, Function Notation, and the Graph

of a Function 190

2.5 Analyzing the Graph of a Function 206

2.6 The Toolbox Functions and Transformations 225

2.7 Piecewise-Deﬁned Functions 240

2.8 The Algebra and Composition of Functions 254

CHAPTER CONNECTIONS

Viewing a function in terms of an equation, a

table of values, and the related graph, often

brings a clearer understanding of the rela-

tionships involved. For example, the power

generated by a wind turbine is often modeled

by the function , where P is

the power in watts and v is the wind velocity

in miles per hour. While the formula enables

us to predict the power generated for a given

wind speed, the graph offers a visual repre-

sentation of this relationship, where we note

a rapid growth in power output as the wind

speed increases. This application appears as

Exercise 107 in Section 2.6.

Check out these other real-world connections:

Earthquake Area (Section 2.1, Exercise 84)

Height of an Arrow (Section 2.5, Exercise 61)

Garbage Collected per Number of Garbage

Trucks (Section 2.2, Exercise 42)

Number of People Connected to the Internet

(Section 2.3, Exercise 109)

P1v2

125

151

EXAMPLE 10 Determining the Domain and Range from the Context

Paul’s 1993 Voyager has a 20-gal tank and gets 18 mpg. The number of miles he

can drive (his range) depends on how much gas is in the tank. As a function we

have where M(g) represents the total distance in miles and g

represents the gallons of gas in the tank. Find the domain and range.

Solution Begin evaluating at since the tank cannot hold less than zero gallons. On a

full tank the maximum range of the van is or .

Because of the tank’s size, the domain is

Now try Exercises 94 through 101

g 30, 204.

M1g 2

30, 360420

18 360 miles

M1g 2

18g,

C. You’ve just learned how

to use function notation and

evaluate functions

▶

Application Exercises at the end of each section are the hallmark of

the Coburn series. Never contrived, always creative, and born out of the

author’s life and experiences, each application tells a story and appeals

to a variety of teaching styles, disciplines, backgrounds, and interests.

▶

Math in Action Applets, located online, enable students to work

collaboratively as they manipulate applets that apply mathematical

concepts in real-world contexts.

▶

Exam

les throu

hout the text feature word

roblems,

“

I especially like the depth and variety of applications in this textbook.

Other College Algebra texts the department considered did not share

this strength. In particular, there is a clear effort on the part of the author

to include realistic examples showing how such math can be utilized in

the real world.

”

—George Alexander, Madison Area Technical College

▶

Mthi Ati A lt

l d li bl d k

“

[The application problems] answered the question, ‘When are we

ever going to use this?’

”

—Student class tester at Metropolitan Community College–Longview

Application Exercises

at the end of each section are the hallmark of

“

One of this text’s strongest features is the wide range of applications

exercises. As an instructor, I can choose which exercises fit my

teaching style as well as the student interest level.

”

—Stephen Toner, Victor Valley College

tween mathematics

Through Meaningful Applications

Through

Meaningful

Applications

cob19529_fm_i-xl.indd Page x 1/2/09 5:54:30 PM usercob19529_fm_i-xl.indd Page x 1/2/09 5:54:30 PM user /Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10/Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10

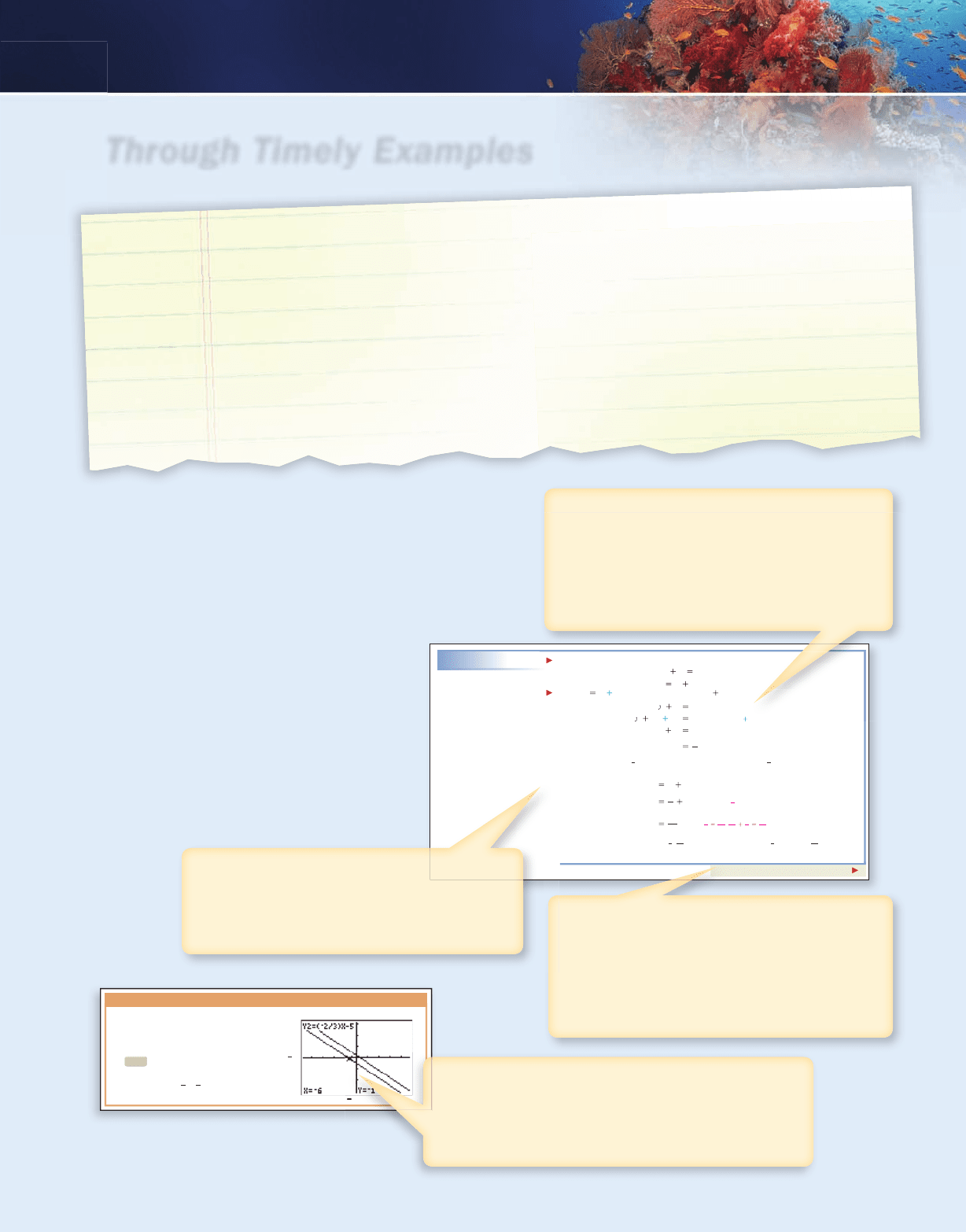
In mathematics, it would be difficult to overstate the importance of examples that set the stage

for learning. Not a few educational experiences have faltered due to an example that was too

difficult, a poor fit, out of sequence, or had a distracting result. In this series, a careful and

deliberate effort was made to select examples that were timely and clear, with a direct focus on the

concept or skill at hand. Everywhere possible, they were further designed to link previous concepts

to current ideas, and to lay the groundwork for concepts to come. As a trained educator knows,

the best time to answer a question is often before it’s ever asked, and a timely sequence of carefully

constructed examples can go a long way in this regard, making each new idea simply the next logical,

even anticipated step. When successful, the mathematical maturity of a student grows in unnoticed

increments, as though it was just supposed to be that way. —JC

Through Timely Examples

GRAPHICAL SUPPORT

Graphing the lines from Example 8 as Y1 and

Y2 on a graphing calculator, we note the lines

do appear to be parallel (they actually must

be since they have identical slopes). Using

the 8:ZInteger feature of the TI-84

Plus we can quickly verify that Y2 indeed

contains the point ( ).

6, 1

ZOOM

▶

Titles have been added to Examples in this edition to

highlight relevant learning objectives and reinforce the

importance of speaking mathematically using vocabulary.

▶

Annotations located to the right of the solution sequence

help the student recognize which property or procedure is

being applied.

“

The incorporation of technology and graphing calculator

usage . . . is excellent. For the faculty that do not use the

technology it is easily skipped. It is very detailed for the

students or faculty that [do] use technology.

”

—Rita Marie O’Brien, Navarro College

EXAMPLE 3 Solving a System Using Substitution

Solve using substitution:

Solution Since we can replace y with in the ﬁrst equation.

ﬁrst equation

substitute for y

simplify

result

The x-coordinate is To ﬁnd the y-coordinate, substitute for x into either of the

original equations. Substituting in the second equation gives

second equation

substitute for x

The solution to the system is Verify by substituting for x and for y into

both equations.

Now try Exercises 23 through 32

x 2

2 4

x 2 4x 1x 22 4

y 4

2y x 2,

y 4

y x 2

Exercises 23 throu

h 3

“

I particularly like the ‘Now Try exercises . . .’ after

each group of examples. I have not seen this in

other texts and it is a really nice addition. I usually

tell my students which examples correspond to

which exercises, so this will save time and effort

on my part.

”

—Scott Berthiaume, Edison State College

Solv

ing

a S

stem Usin

Substitution

ng su

tut

nce we can repla

with

in the ﬁrst equation

ﬁ

rst e

uatio

subst

itute

for

rst

“

The author does a great job in describing the

examples and how they are to be written. In the

examples, the author shows step by step ways

to do just one problem . . . this makes for a

better understanding of what is being done.

”

—Michael Gordon, student class

tester at Navarro College

▶

“Now Try” boxes immediately following

Examples guide students to specific matched

exercises at the end of the section, helping

them identify exactly which homework

problems coincide with each discussed

concept.

▶

Graphical Support Boxes, located after

selected examples, visually reinforce

algebraic concepts with a corresponding

graphing calculator example.

examples

visually

reinforce

concepts with a correspondin

calculator example.

“

I thought the author did a good job of explaining

the content by using examples, because there

was an example of every kind of problem.

”

—Brittney Pruitt, student class tester at

Metropolitan Community College–Longview

cob19529_fm_i-xl.indd Page xi 1/2/09 5:54:39 PM usercob19529_fm_i-xl.indd Page xi 1/2/09 5:54:39 PM user /Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10/Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10

xii

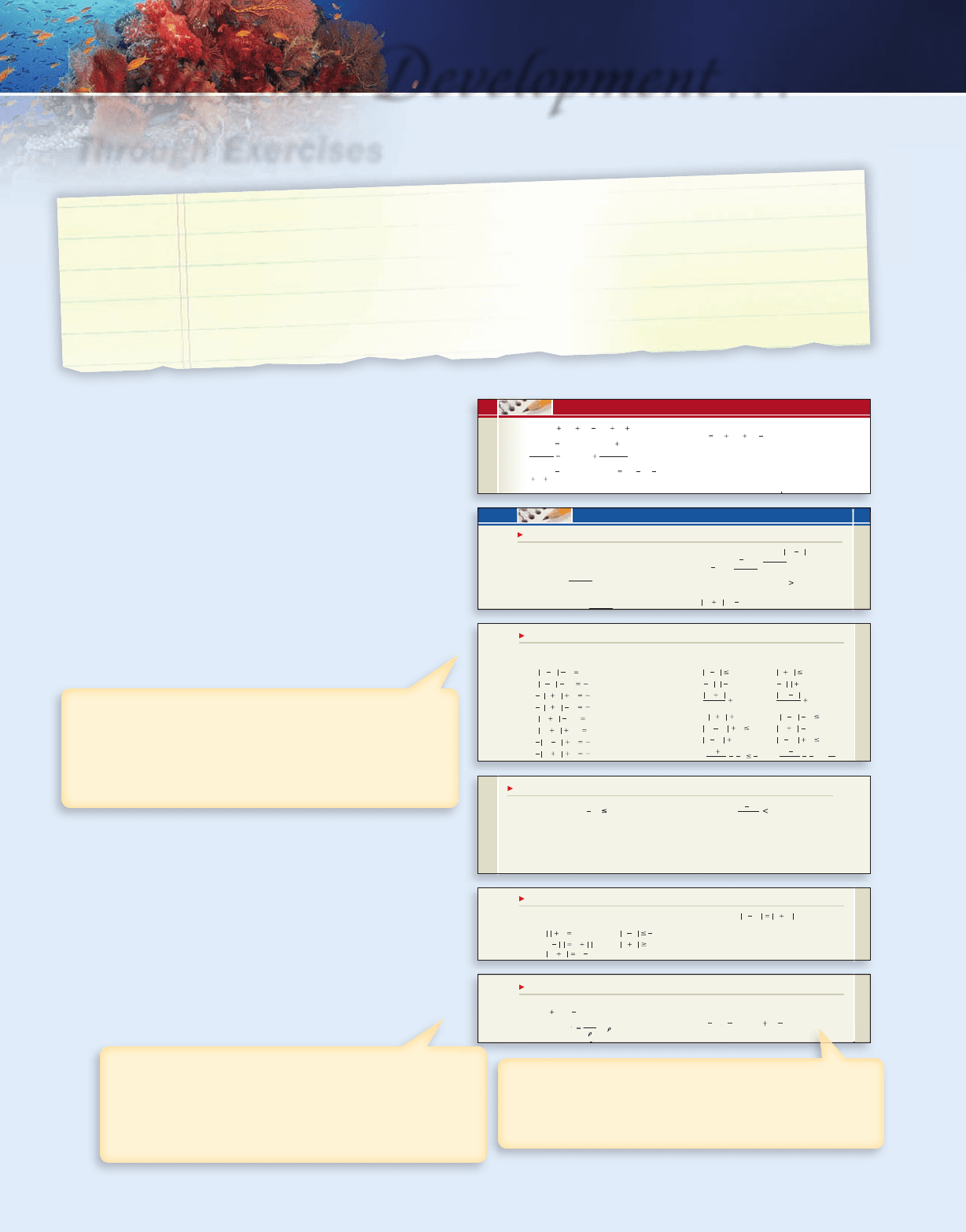
Mid-Chapter Checks

Mid-Chapter Checks provide students with a good stopping

place to assess their knowledge before moving on to the

second half of the chapter.

Solid Skill Development . . .

Through Exercises

apter

ecks

Throu

Exercises

I have included a wealth of exercises in support of each section’s main ideas. I constructed

each set with great care, in an effort to provide strong support for weaker students, while

challenging advanced students to reach even further. I also designed the various exercises to

support instructors in their teaching endeavors—the quantity and quality of the exercises allow

for numerous opportunities to guide students through difficult calculations, and to illustrate

important problem-solving techniques.—JC

End-of-Section Exercise Sets

▶

Concepts and Vocabulary exercises to help students

recall and retain important terms.

▶

Extending the Concept exercises that require

communication of topics, synthesis of related

concepts, and the use of higher-order thinking

skills.

1.3 EXERCISES

CONCEPTS AND VOCABULARY

Fill in the blank with the appropriate word or phrase.

Carefully reread the section if needed.

1. When multiplying or dividing by a negative

quantity, we the inequality to maintain a

true statement.

2. To write an absolute value equation or inequality in

sim

liﬁed form, we the absolute value

4. The absolute value inequality is

true when and

Describe each solution set (assume ). Justify your

answer.

5. ax b 6 k

k 0

3x 6 6

3x 6 7

3x 6 6 12

DEVELOPING YOUR SKILLS

Solve each absolute value equation. Write the solution in

set notation.

10.

11.

12.

13.

14.

3q 4 3 5

7p 3 6 5

2w 5 6.3 11.2

4v 5 6.5 10.3

2y 3 4 14

3x 5 6 15

n 5 14 2

m 1 7 3

Solve each absolute value inequality. Write solutions in

interval notation.

25. 26.

27. 28.

29. 30.

31. 32.

33. 34.

35. 36.

37. 38.

2y 3

4x 5

2 7u 7 44 3z 12 6 7

2c 3 5 6 13b 11 6 9

q 2 7 83 p 4 5 6 8

3w 2

6 6 8

5v 1

8 6 9

2 n 3 7 73 m 2 7 4

y 1 3x 2 7

WORKING WITH FORMULAS

55. Spring Oscillation

A weight attached to a spring hangs at rest a distance

of x in. off the ground. If the weight is pulled down

(stretched) a distance of L inches and released, the

weight begins to bounce and its distance d off the

ground must satisfy the indicated formula. If x

equals 4 ft and the spring is stretched 3 in. and

released, solve the inequality to ﬁnd what distances

56. A “Fair” Coin

If we ﬂipped a coin 100 times, we expect “heads”

to come up about 50 times if the coin is “fair.” In a

study of probability, it can be shown that the

number of heads h that appears in such an

experiment must satisfy the given inequality to be

considered “fair.” (a) Solve this inequality for h.

1.645

EXTENDING THE CONCEPT

67. Determine the value or values (if any) that will

make the equation or inequality true.

.b.a

.d.c

2x 1 x 3

x 3 6xx

x x x

x 2

x x 8

68. The equation has only one

solution. Find it and explain why there is only one.

5 2x 3 2x

MAINTAINING YOUR SKILLS

69. (R.4) Factor the expression completely:

70. (1.1) Solve for

(physics).

C A

18x

21x

60x.

72. (1.2) Solve the inequality, then write the solution

set in interval notation:

312x 52 7 21x 12 7.

EXERCISES

MID-CHAPTER CHECK

1. gnisu etupmoC

long division and write the result in two ways:

dna )a(

. )b(

2. Given that is a factor of

use the rational zeroes theorem to

write f(x) in completely factored form.

x 6,

f 1x2

x 2

dividend

divisor

1quotient2

remainder

divisor

dividend

1quotient21divisor2 remainder

7x 142 1x 22

9. Use the Guidelines for Graphing to draw the graph

10. When ﬁghter pilots train for dogﬁghting, a “hard-

deck” is usually established below which no

competitive activity can take place. The polynomial

graph given shows Maverick’s altitude above and

below this hard-deck during a 5-sec interval.

a. What is the minimum

ibl d

q1x2 x

2x 8.

Altitude

(100s of feet)

▶

Developing Your Skills exercises to provide

practice of relevant concepts just learned with

increasing levels of difficulty.

▶

Working with Formulas exercises to demonstrate

contextual applications of well-known formulas.

▶

Maintaining Your Skills exercises that address

skills from previous sections to help students

retain previously learning knowledge.

(

)

for (

phy

cs).

“

The strongest feature seems to be the wide variety of

exercises included at the end of each section. There are

plenty of drill problems along with good applications.

”

—Jason Pallett, Metropolitan Community College–Longview

reta

n prev

ous

earn

now

“

He not only has exercises for skill development, but also

problems for ‘extending the concept’ and ‘maintaining

your skills,’ which our current text does not have. I also

like the mid-chapter checks provided. All these give

Coburn an advantage in my view.

”

—Randy Ross, Morehead State University

practice

relevant

concepts

just

learned

with

increasin

levels of difficult

▶

“

Some of our instructors would mainly assign the developing

your skills and working with formula problems, however, I

would focus on the writing, research and decision making

[in] extending the concept. The flexibility is one of the

things I like about the Coburn text.

”

—Sherry Meier, Illinois State University

cob19529_fm_i-xl.indd Page xii 1/2/09 5:54:46 PM usercob19529_fm_i-xl.indd Page xii 1/2/09 5:54:46 PM user /Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10/Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10

xiii

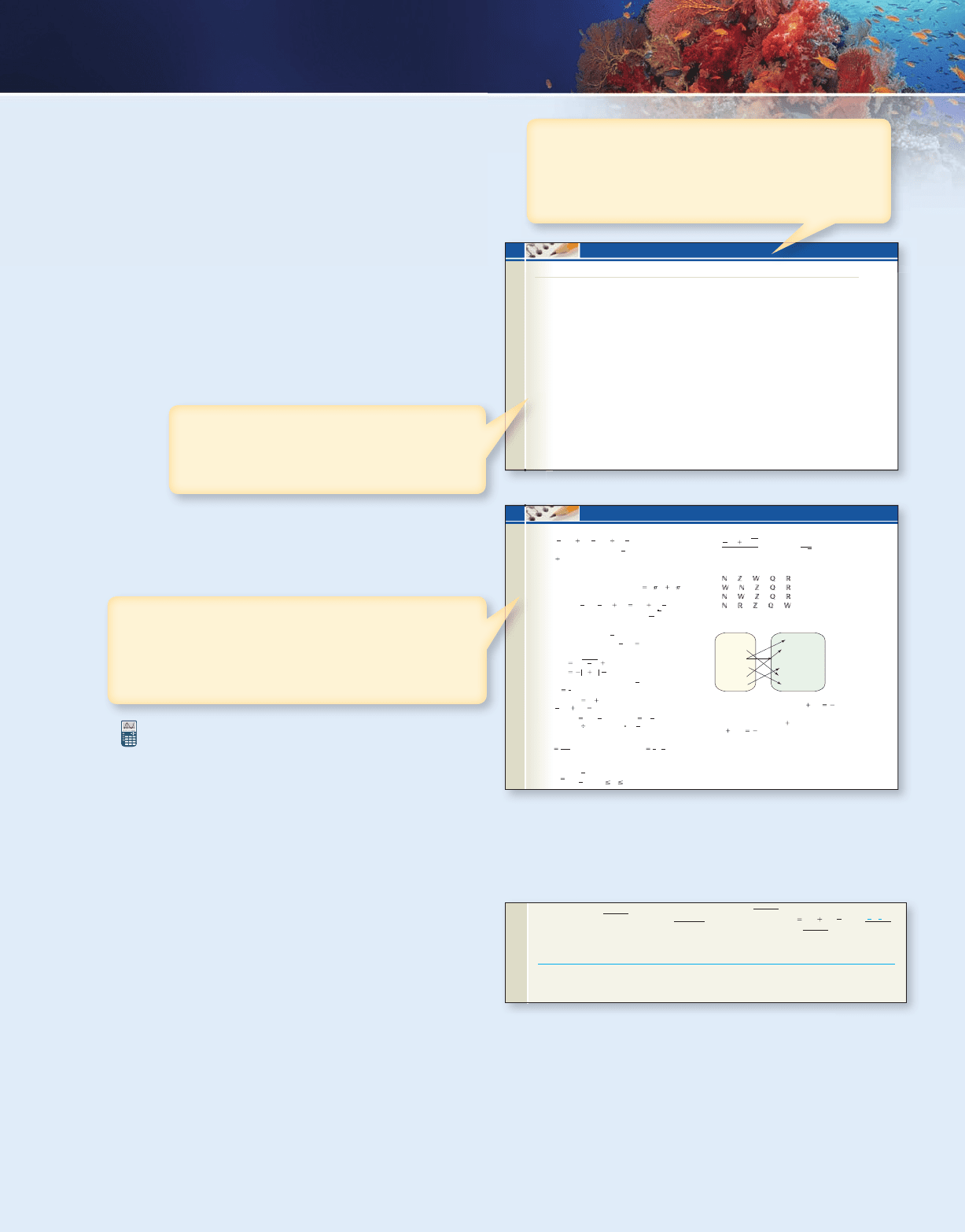
SECTION 1.1 Linear Equations, Formulas, and Problem Solving

KEY CONCEPTS

•

An equation is a statement that two expressions are equal.

•

Replacement values that make an equation true are called solutions or roots.

•

Equivalent equations are those that have the same solution set.

•

To solve an equation we use the distributive property and the properties of equality to write a sequence of simpler,

equivalent equations until the solution is obvious. A guide for solving linear equations appears on page 75.

•

If an equation contains fractions, multiply both sides by the LCD of all denominators, then solve.

•

Solutions to an equation can be checked using back-substitution, by replacing the variable with the proposed

solution and verifying the left-hand expression is equal to the right.

•

An equation can be:

1. an identity, one that is always true, with a solution set of all real numbers.

2. a contradiction, one that is never true, with the empty set as the solution set.

3. conditional, or one that is true/false depending on the value(s) input.

•

To solve formulas for a speciﬁed variable, focus on the object variable and apply properties of equality to write

this variable in terms of all others.

•

The basic elements of good problem solving include:

1. Gathering and organizing information

2. Making the problem visual

3. Developing an equation model

4. Using the model to solve the application

For a complete review, see the problem-solving guide on page 78.

SUMMARY AND CONCEPT REVIEW

End-of-Chapter Review Material

Exercises located at the end of the chapter provide students with

the tools they need to prepare for a quiz or test. Each chapter

features the following:

CUMULATIVE REVIEW CHAPTERS 1–2

1. Perform the division by factoring the numerator:

2. Find the solution set for: and

3. The area of a circle is 69 cm

. Find the

circumference of the same circle.

4. The surface area of a cylinder is

Write r in terms of A and h (solve for r).

5. . rof evloS

6. Evaluate without using a calculator: .

7. Find the slope of each line:

a. through the points: and (2, 5).

b. a line with equation .

8. Graph using transformations of a parent function.

a. .

b. .

9. Graph the line passing through with a slope

of then state its equation.

10. Show that is a solution to

11. Given and ﬁnd:

and

12. Graph by plotting the y-intercept, then counting

to ﬁnd additional points:

13. Graph the piecewise deﬁned function

and determine

the following:

f 1x2 e

4 x 6 2

12x 8

x 2m

¢y

¢x

f 21 22.1f

g21x2,1f

g21x2,

g1x2

x 2f 1x2

2x 26 0

1 5i

3, 22

f 1x2

x 2 3

f 1x2

1x 2 3

5y 20

4, 72

x: 213 x2 5x 41x 12 7

2 r

2 rh.

2 6 8.

x 6 5

2x 102 1x 52.

16. Simplify the radical expressions:

.b.a

17. Determine which of the following statements

are false, and state why.

18. Determine if the following relation is a function.

If not, how is the deﬁnition of a function violated?

19. Solve by completing the square. Answer in both

exact and approximate form:

20. Solve using the quadratic formula. If solutions are

complex, write them in form.

21. The National Geographic Atlas of the World is a very

large, rectangular book with an almost inexhaustible

panoply of information about the world we live in.

The length of the front cover is 16 cm more than its

width, and the area of the cover is 1457 cm

. Use this

information to write an equation model, then use the

uadratic formula to determine the len

th and width

20x 51

49 20x

Michelangelo

Titian

Raphael

Giorgione

da Vinci

Correggio

Parnassus

La Giocanda

The School of Athens

Jupiter and Io

Venus of Urbino

The Tempest

( ( ( (

10 172

▶

Chapter Summary and Concept Reviews that present

key concepts with corresponding exercises by section in

a format easily used by students.

▶

Mixed Reviews that offer more practice on topics from

the entire chapter, arranged in random order requiring

students to identify problem types and solution strategies

on their own.

▶

Practice Tests that give students the opportunity to check

their knowledge and prepare for classroom quizzes, tests,

and other assessments.

▶

Cumulative Reviews that are presented at the end of

each chapter help students retain previously learned

skills and concepts by revisiting important ideas from

earlier chapters (starting with Chapter 2).

•

For

“

We always did reviews and a quiz before the

actual test; it helped a lot.

”

—Melissa Cowan, student class tester

Metropolitan Community College–Longview

▶

Graphing Calculator icons appear next to exercises

where important concepts can be supported by the

use of graphing technology.

ION 1.1

SECT

Linear E

quat

ions

,Fo

rmul

as,

and Pr

oblem Solvin

MMA

Y AND

EPT REVI

“

The summary and concept review was very helpful

because it breaks down each section. That is what

helps me the most.

”

— Brittany Pratt, student class tester at Baton Rouge

Community College

“

The cumulative review is very good and is considerably

better than some of the books I have reviewed/used. I

have found these to be wonderful practice for the final

exam.

”

—Sarah Clifton, Southeastern Louisiana University

Homework Selection Guide

A list of suggested homework exercises has been provided for each section of the text (Annotated Instructor’s Edition only).

This feature may prove especially useful for departments that encourage consistency among many sections, or those having a

large adjunct population. The feature was also designed as a convenience to instructors, enabling them to develop an inventory

of exercises that is more in tune with the course as they like to teach it. The Guide provides prescreened and preselected

assignments at four different levels: Core, Standard, Extended, and In Depth.

• Core: These assignments go right to the heart of the

material, offering a minimal selection of exercises that cover

the primary concepts and solution strategies of the section,

along with a small selection of the best applications.

• Standard: The assignments at this level include the Core

exercises, while providing for additional practice without

excessive drill. A wider assortment of the possible variations on a theme are included, as well as a greater variety of

applications.

• Extended: Assignments from the Extended category expand on the Standard exercises to include more applications, as well

as some conceptual or theory-based questions. Exercises may include selected items from the Concepts and Vocabulary,

Working with Formulas, and the Extending the Thought categories of the exercise sets.

• In Depth: The In Depth assignments represent a more comprehensive look at the material from each section, while

attempting to keep the assignment manageable for students. These include a selection of the most popular and highest-quality

exercises from each category of the exercise set, with an additional emphasis on Maintaining Your Skills.

on a

eme are

as w

asagreatervar

ty o

1. After a vertical , points on the graph are

farther from the x-axis. After a vertical ,

points on the graph are closer to the x-axis.

and .

3. The vertex of is at

and the graph opens .

h1x2 31x 52

HOMEWORK SELECTION GUIDE

Core: 7–59 every other odd, 61–73 odd, 75–91 every other odd, 93–101

odd, 105, 107 (33 Exercises)

Standard: 1–4 all, 7–59 every other odd, 61, 63–73 odd, 75–91 every other

odd, 93–101 odd, 105, 107, 109 (38 Exercises)

stretch

compression

reﬂections

(

5, 9)

upward

Extended: 1–4 all, 7–59 every other odd, 61, 63–73 odd, 75–91 every other

odd, 93–101 odd, 103, 105, 106, 107, 109, 111, 112, 114, 117 (44 Exercises)

In Depth: 1–6 all, 7–59 every other odd, 61, 63–73 odd, 75–91 every other odd,

93–101 odd, 103, 104, 105–110 all, 111–117 all (54 Exercises)

cob19529_fm_i-xl.indd Page xiii 1/16/09 8:57:22 PM usercob19529_fm_i-xl.indd Page xiii 1/16/09 8:57:22 PM user /Don't del/DDOOON'T_DEL_YASH/6-12-08/HARRIS_CH-16/Don't del/DDOOON'T_DEL_YASH/6-12-08/HARRIS_CH-16

While examples and applications are arguably the most prominent features of a mathematics text,

it’s the writing style and readability that binds them together. It may be true that some students

don’t read the text, and that others open the text only when looking for an example similar to the

exercise they’re currently working. But when they do and for those students who do (read the text),

it’s important they have a text that “speaks to them,” relating concepts in a form and at a level

they understand and can relate to. Ideally this text will draw students in and keep their interest,

becoming a positive experience and bringing them back a second and third time, until it becomes

habitual. At this point, students might begin to see the true value of their text (as more that just

a source of problems—pun intended), and it becomes a resource for learning on equal footing with

any other form of supplemental instruction. —JC

Strong Connections . . .

xiv

WhWh

ilil

exex

amam

plpl

ndnd

caca

titi

onon

s s

arar

e e

lyly

t t

hehe

ost

ominent features of a mathematics text,

t prominent features of a math

atics

xt,

’’

’

titi

ddd

bibi

lili

tyty

thth

ogether It may be truethatsome

udents

Through a Conversational Writing Style

Conversational Writing Style

John Coburn’s experience in the classroom and his strong connections

to how students comprehend the material are evident in his writing

style. He uses a conversational and supportive writing style, providing

the students with a tool they can depend on when the teacher is not

available, when they miss a day of class, or simply when working on

their own. The effort John has put into the writing is representative

of his unofficial mantra: “If you want more students to reach the top,

you gotta put a few more rungs on the ladder.”

Through Student Involvement

How do you design a student-friendly textbook? We decided to get students involved by

hosting two separate focus groups. During these sessions we asked students to advise us on how they use their books,

what pedagogical elements are useful, which elements are distracting and not

useful, as well as general feedback on page layout. During this process there

were times when we thought, “Now why hasn’t anyone ever thought of that

before?” Clearly these student focus groups were invaluable. Taking direct

student feedback and incorporating what is feasible and doesn’t detract from

instructor use of the text is the best way to design a truly student-friendly

text. The next two pages will highlight what we learned from students so

you can see for yourself how their feedback played an important role in the

development of the Coburn series.

“

The author does a fine job with his narrative.

His explanations are very clear and concise.

I really like his explanations better than in my

current text.

”

—Tammy Potter, Gadsden State College

“

The author does an excellent job of

engagement and it is easily seen that he is

conscious of student learning styles.

”

—Conrad Krueger, San Antonio College

cob19529_fm_i-xl.indd Page xiv 1/2/09 5:54:55 PM usercob19529_fm_i-xl.indd Page xiv 1/2/09 5:54:55 PM user /Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10/Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10

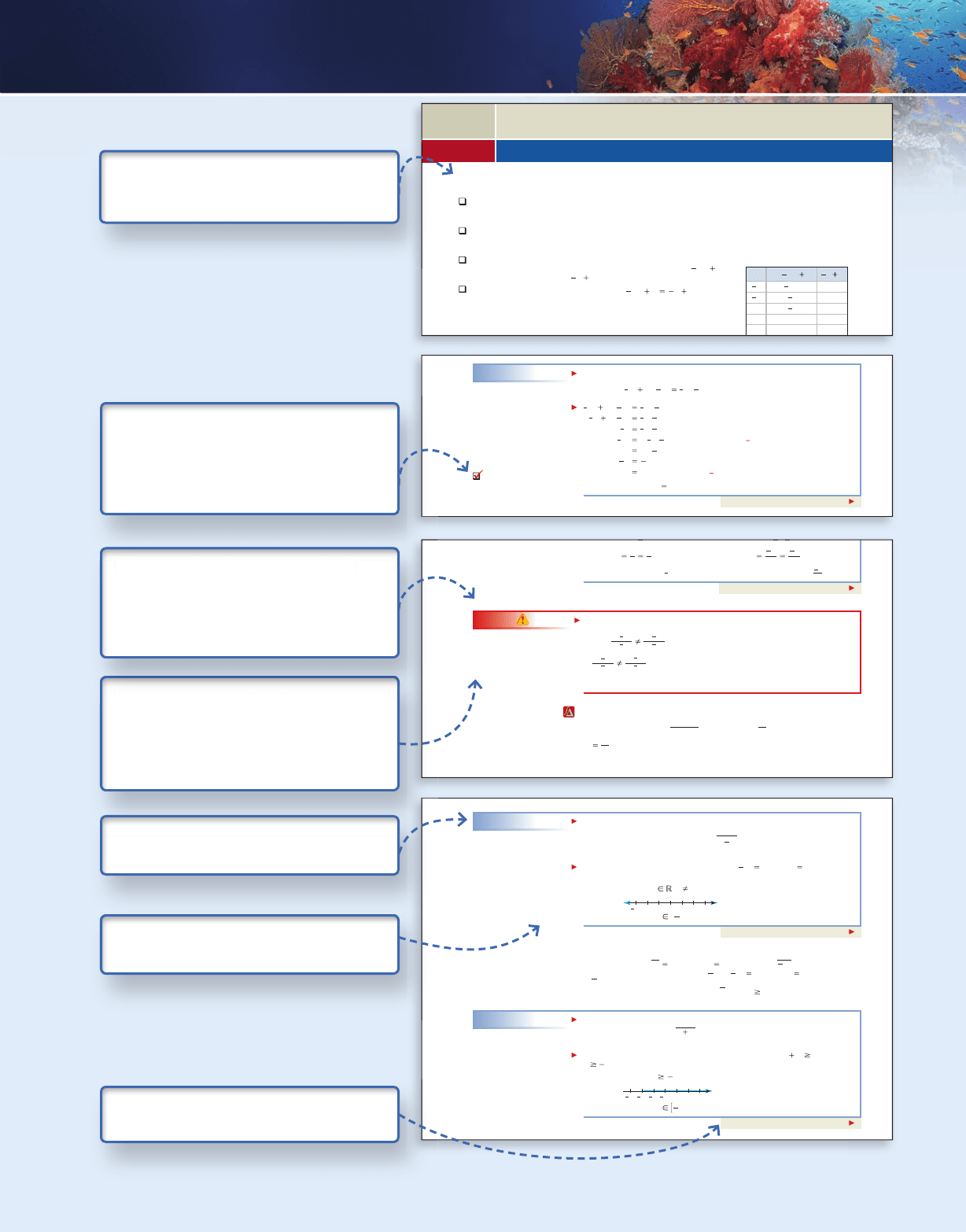
EXAMPLE 2 Solving a Linear Equation with Fractional Coefﬁcients

Solve for n:

Solution original equation

distributive property

combine like terms

multiply both sides by

distributive property

subtract 2n

multiply by

Verify the solution is using back-substitution.

Now try Exercises 13 through 30

n 12

1 n 12

n 12

2n 12

LCD 4 41

n2 41

n 32

n 3

n 2 2

n 3

1n 82 2

1n 62

1n 82 2

1n 62.

A. You’ve just learned how

to solve linear equations

using properties of equality

In a study of algebra, you will encounter many families of equations, or groups of

equations that share common characteristics. Of interest to us here is the family of

linear equations in one variable, a study that lays the foundation for understanding

more advanced families. In addition to solving linear equations, we’ll use the skills we

develop to solve for a speciﬁed variable in a formula, a practice widely used in science,

business, industry, and research.

A. Solving Linear Equations Using Properties of Equality

An equation is a statement that two expressions are

equal. From the expressions and

we can form the equation

which is a linear equation in one variable. To solve

an equation, we attempt to ﬁnd a speciﬁc input or x-

value that will make the equation true, meaning the

left

hand expression will be equal to the right. Using

31x 12 x x 7.

x 7,

31x

12 x

Learning Objectives

In Section 1.1 you will learn how to:

A. Solve linear equations

using properties of

equality

B. Recognize equations

that are identities or

contradictions

C. Solve for a specified

variable in a formula or

literal equation

D. Use the problem-solving

guide to solve various

problem types

1.1 Linear Equations, Formulas, and Problem Solving

11 6

25 5

112

x 731x 12 x

Table 1.1

EXAMPLE 8 Determining the Domain of an Expression

Determine the domain of the expression . State the result in set notation,

graphically, and using interval notation.

Solution Set the denominator equal to zero and solve: yields This means

2 is outside the domain and must be excluded.

• Set notation:

• Graph:

• Interval notation:

Now try Exercises 61 through 68

A second area where allowable values are a concern involves the square root oper-

ation. Recall that since However, cannot be written as

the product of two real numbers since and

49. In other words,

represents a real number only if the radicand is positive or zero. If X represents

an algebraic expression, the domain of is

EXAMPLE 9 Determining the Domain of an Expression

Determine the domain of State the domain in set notation, graphically,

and in interval notation.

Solution The radicand must represent a nonnegative number. Solving gives

• Set notation:

• Graph:

• Interval notation:

Now try Exercises 69 through 76

x 3, q 2

[

1123 24 0

x 36

3 0

061X

71 72

1 72 49

497

7 49.149 7

1 q , 22 ´ 12, q 2

)

1 123450

x , x 26

2.x 2 0

x 2

The slope of this line is . The slope of this line is .

Now try Exercises 33 through 40

CAUTION When using the slope formula, try to avoid these common errors.

1. The order that the x- and y-coordinates are subtracted must be consistent,

since .

2. The vertical change (involving the y-values) always occurs in the numerator:

3. When x

or y

is negative, use parentheses when substituting into the formula to

prevent confusing the negative sign with the subtraction operation.

Actually, the slope value does much more than quantify the slope of a line, it

expresses a rate of change between the quantities measured along each axis. In appli-

cations of slope, the ratio is symbolized as . The symbol is the Greek

letter delta and has come to represent a change in some quantity, and the notation

is read, “slope is equal to the change in y over the change in x.” Interpreting

slope as a rate of change has many signiﬁcant applications in college algebra and

beyond.

¢y

¢x

¢y

¢x

change in y

change in x

4 1 228 2

Students told us they liked when the

examples were linked to the exercises.

Students said that Learning Objectives

should clearly define the goals of each

section.

Examples are “boxed” so students can

clearly see where they begin and end

Students told us that the color red should

only be used for things that are really

important. Also, anything significant

should be included in the body of the

text; marginal readings imply optional.

tudents told us that the color red should

Described by students as one of the

most useful features in a math text,

Caution Boxes signal a student to stop

and take note in order to avoid mistakes

in problem solving.

Students asked for Check Points

throughout each section to alert them

when a specific learning objective has

been covered and to reinforce the use

of correct mathematical terms.

Examples are called out in the margins

so they are easy for students to spot.

cob19529_fm_i-xl.indd Page xv 1/2/09 5:55:01 PM usercob19529_fm_i-xl.indd Page xv 1/2/09 5:55:01 PM user /Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10/Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10

xvi

Solve using the zero product property. Be sure each

equation is in standard form and factor out any

common factors before attempting to solve. Check all

answers in the original equation.

.8.7

9. 10.

11. 12.

13. 14.

15. 16.

17. 18.

19.

20.

21.

22.

23.

24.

25.

26.

27.

28.

29.

30.

31.

32.

Solve each equation. Identify any extraneous roots.

33.

34.

35.

36.

2y 3

3y 5

a 2

a 1

m 3

x 1

64 0

1 0

9 0

8 0

16x

48 0

2 0

625 0

256 0

1 0

81 0

27x

28x

81 27x

12x

10x 60

7 7x

4x 12 3x

18 9x 2x

4x 5x

64x 02x

16x 0

15x 2x

13x

42x22x x

39.

40.

41.

42.

43.

44.

Solve for the variable indicated.

45. for f 46. for z

47. for r 48. for p

49. for h 50. for g

51. for 52. for

Solve each equation and check your solutions by

substitution. Identify any extraneous roots.

53. a. b.

54. a. b.

55. a. b.

c. d.

56. a. b.

57. a.

c. 1x

2 12x 2

3 223 x

1x 9 1x 9

x 3 21

2x 17

6x 7

5 6

3 1

3 4x 7 43 1

5p 2

2x 9 1

3x 7

2m 3

2 3

7 3x 3 72 1

3m 1

5 15x 1 x214x 1 10

13x 1 3313x 5 9

h;r

;

p f

R r

;

n 1

2n 1

3n 1

2a 1

5a 3

a 3

p 2

5p 6

p 3

n 3

n 6

n 2

x 5

x 1

x 5

x 7

WORKING WITH FORMULAS

79. Lateral surface area of a cone:

The lateral surface area (surface

area excluding the base) S of a

cone is given by the formula

shown, where r is the radius of

the base and h is the height of

the cone. (a) Solve the equation

for h. (b) Find the surface area

of a cone that has a radius of 6 m

and a height of 10 m. Answer in

simplest form.

S r2r

80. Painted area on a canvas:

A rectangular canvas is to contain a small painting

with an area of and requires 2-in. margins

on the left and right, with 1-in. margins on the top

and bottom for framing. The total area of such a

canvas is given by the formula shown, where x is

the height of the painted area.

a. What is the area A of the canvas if the height of

the painting is in.?

b. If the area of the canvas is what

are the dimensions of the painted area?

120 in

52 in

60x 104

88. Composite ﬁgures—gelatin capsules: The gelatin

capsules manufactured for cold and ﬂu medications

are shaped like a cylinder with a hemisphere on

each end. The interior volume V of each capsule

can be modeled by where h is

the height of the cylindrical portion and r is its

radius. If the cylindrical portion of the capsule is

8 mm long what radius would give

the capsule a volume that is numerically equal to

times this radius?

89. Running shoes: When a popular running shoe is

priced at $70, The Shoe House will sell 15 pairs

each week. Using a survey, they have determined

that for each decrease of $2 in price, 3 additional

pairs will be sold each week. What selling price will

give a weekly revenue of $2250?

90. Cell phone charges: A cell phone service sells 48

subscriptions each month if their monthly fee is

$30. Using a survey, they ﬁnd that for each

decrease of $1, 6 additional subscribers will join.

What charge(s) will result in a monthly revenue of

$2160?

Projectile height: In the absence of resistance, the height

of an object that is projected upward can be modeled by the

equation where h represents the

height of the object (in feet) t sec after it has been thrown, v

represents the initial velocity (in feet per second), and k

represents the height of the object when (before it hast

h 16t

vt k,

1h 8 mm2,

velocity

160

ft/sec

and

height

240

runs

out of fuel and becomes a projectile.

a. How high is the rocket three seconds later?

Four seconds later?

b. How long will it take the rocket to attain a

height of 640 ft?

c. How many times is a height of 384 ft attained?

When do these occur?

d. How many seconds until the rocket returns to

the ground?

93. Printing newspapers: The editor of the school

newspaper notes the college’s new copier can

complete the required print run in 20 min, while

the back-up copier took 30 min to do the same

amount of work. How long would it take if both

copiers are used?

94. Filling a sink: The cold water faucet can ﬁll a sink

in 2 min. The drain can empty a full sink in 3 min.

If the faucet were left on and the drain was left

open, how long would it take to ﬁll the sink?

95. Triathalon competition: As one part of a

Mountain-Man triathalon, participants must row a

canoe 5 mi down river (with the current), circle a

buoy and row 5 mi back up river (against the

current) to the starting point. If the current is

ﬂowing at a steady rate of 4 mph and Tom Chaney

made the round-trip in 3 hr, how fast can he row

in still water? (Hint: The time rowing down

river and the time rowing up river must add up

to 3 hr.)

96. Flight time: The ﬂight distance from Cincinnati,

Ohio, to Chicago, Illinois, is approximately

300 mi. On a recent round-trip between these cities

in my private plane, I encountered a steady 25 mph

headwind on the way to Chicago, with a 25 mph

tailwind on the return trip. If my total ﬂying time

Because students spend a lot of time in

the exercise section of a text, they said

that a white background is hard on their

eyes . . . so we used a soft, off-white color

for the background

Students said having a lot of icons was

confusing. The graphing calculator is the

only icon used in the exercise sets; no

unnecessary icons are used

Students told us that directions should be

in bold so they are easily distinguishable

from the problems.

cob19529_fm_i-xl.indd Page xvi 1/2/09 5:55:06 PM usercob19529_fm_i-xl.indd Page xvi 1/2/09 5:55:06 PM user /Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10/Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10

College Algebra, Second Edition

Review

䉬

Equations and Inequalities

䉬

Relations, Functions, and Graphs

䉬

Polynomial

and Rational Functions

䉬

Exponential and Logarithmic Functions

䉬

Systems of

Equations and Inequalities

䉬

Matrices

䉬

Geometry and Conic Sections

䉬

Additional

Topics in Algebra

ISBN 0-07-351941-3, ISBN 978-0-07351941-8

College Algebra Essentials, Second Edition

Review

䉬

Equations and Inequalities

䉬

Relations, Functions, and Graphs

䉬

Polynomial

and Rational Functions

䉬

Exponential and Logarithmic Functions

䉬

Systems of

Equations and Inequalities

ISBN 0-07-351968-5, ISBN 978-0-07351968-5

Algebra and Trigonometry, Second Edition

Review

䉬

Equations and Inequalities

䉬

Relations, Functions, and Graphs

䉬

Polynomial

and Rational Functions

䉬

Exponential and Logarithmic Functions

䉬

Trigonometric

Functions

䉬

Trigonometric Identities, Inverses and Equations

䉬

Applications of

Trigonometry

䉬

Systems of Equations and Inequalities

䉬

Matrices

䉬

Geometry and

Conic Sections

䉬

Additional Topics in Algebra

ISBN 0-07-351952-9, ISBN 978-0-07-351952-4

Precalculus, Second Edition

Equations and Inequalities

䉬

Relations, Functions, and Graphs

䉬

Polynomial and

Rational Functions

䉬

Exponential and Logarithmic Functions

䉬

Trigonometric

Functions

䉬

Trigonometric Identities, Inverses and Equations

䉬

Applications of

Trigonometry

䉬

Systems of Equations and Inequalities, and Matrices

䉬

Geometry

and Conic Sections

䉬

Additional Topics in Algebra

䉬

Limits

ISBN 0-07-351942-1, ISBN 978-0-07351942-5

Trigonometry, Second Edition—Coming in 2010!

Introduction to Trigonometry

䉬

Trigonometric Functions

䉬

Trigonometric Identities

䉬

Trigonometric Inverses and Equations

䉬

Applications of Trigonometry

䉬

Conic

Sections and Polar Coordinates

ISBN 0-07-351948-0, ISBN 978-0-07351948-7

Coburn’s Precalculus Series

xvii

cob19529_fm_i-xl.indd Page xvii 1/2/09 5:55:14 PM usercob19529_fm_i-xl.indd Page xvii 1/2/09 5:55:14 PM user /Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10/Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10

Making Connections . . .

xviii

Through New and Updated Content

New to the Second Edition

▶

An extensive reworking of the narrative and reduction of advanced concepts enhances the clarity of the exposition,

improves the student’s experience in the text, and decreases the overall length of the text.

▶

A modified interior design based on student and instructor feedback from focus groups features increased font size,

improved exercise and example layout, more white space on the page, and the careful use of color to enhance the

presentation of pedagogy.

▶

Chapter Openers based on applications bring awareness to students of the relevance of concepts presented in each

chapter.

▶

The removal of algebraic proofs from the main body of the text to an appendix provides better focus in the chapter

and presents mathematics in a less technical manner.

▶

Checkpoints throughout each section alert students when a specific learning objective has been covered and

reinforce the use of correct mathematical terms.

▶

The Homework Selection Guide, appearing in each exercise section in the Annotated Instructor’s Edition, provides

instructors with suggestions for developing core, standard, extended, and in-depth homework assignments without

much prep work.

▶

The Modeling with Technology feature between chapters presents standalone coverage of regression, with

pedagogy, exercises, and applications for those instructors who choose to cover this material

Chapter-by-Chapter Changes

CHAPTER R A Review of Basic Concepts and Skills

• Square and cube roots are now covered together.

• Section R.2 features more opportunities for mathematical modeling as well as a summary of exponential properties.

• Examples using radicals have been added to Section R.3 to provide more practice solving, factoring, and

simplifying.

• The discussion of factoring in Section R.4 now includes x

− k, when k is not a perfect square, and higher-degree

expressions.

• A Chapter Overview has been added to the end of the chapter, offering students a study tool for the review of

prerequisite topics.

CHAPTER 1 Equations and Inequalities

• Chapter 1 now includes coverage of absolute value equations and inequalities in Section 1.3.

• Information on solving quadratics has been consolidated to a single section (1.5) and summary boxes are now used

for solving linear equations, solving quadratic equations, and solution methods for quadratic equations.

• Examples and exercises employing the use of a graphing calculator have been added throughout the chapter.

CHAPTER 2 Relations, Functions, and Graphs

• The organization of Chapter 2 has changed from the first edition in an effort to concentrate the introduction of

graphs and general functions.

• Coverage of the midpoint formula, the distance formula, and circles has been improved and reorganized (Section 2.1).

• Linear graphs are established early in the chapter (Sections 2.2 and 2.3) before functions are introduced.

• The section on the toolbox (basic parent) functions (2.6) now appears after analyzing graphs (Section 2.5) to improve

connections among the material.

• Coverage of rates of change has been consolidated while coverage of the implied domain, distance quotient, end

behavior, and even/odd functions has been expanded and improved.

• Additional applications of the floor and ceiling functions and the algebra of functions have been added.

• Regression material in this chapter has been removed and concentrated in the between-chapter Modeling with

Technology feature.

cob19529_fm_i-xl.indd Page xviii 1/2/09 5:55:21 PM usercob19529_fm_i-xl.indd Page xviii 1/2/09 5:55:21 PM user /Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10/Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10

xix

CHAPTER 3 Polynomial and Rational Functions

• Chapter 3 has been significantly reorganized to bring focus to and provide a better bridge between general functions

and polynomial functions.

• More coverage of completing the square and increased emphasis on graphing using the vertex formula is found in

Section 3.1.

• Complex conjugates, zeroes of multiplicity, and number of zeroes have been realigned together in Section 3.2.

• Section 3.3 features an improved description of Descartes’ rule of signs, as well as stronger connections between

the fundamental theorem of algebra and the linear factorization theorem and its corollaries.

• A better introduction regarding polynomials versus nonpolynomials is found in Section 3.4, in addition to an

improved discussion of end behavior.

• Section 3.6 provides better treatment of removable discontinuities and a clearer discussion of pointwise versus

asymptotic continuities.

• Section 3.8 presents a clearer, stronger connection between previously covered topics and applications of variation

and the toolbox functions.

• Regression material in this chapter has been removed and concentrated in the between-chapter Modeling with

Technology feature.

CHAPTER 4 Exponential and Logarithmic Functions

• Chapter 4 now begins with coverage of one-to-one and inverse functions given their applications for exponents and

logarithms.

• This section (4.1) includes examples of finding inverses of rational functions, as well as better coverage of restricting

the domain to find the inverse.

• Coverage of base e as an alternative to base 10 or b is addressed in one section (4.2) as opposed to two sections as

in the first edition.

• Likewise, coverage of properties of logs and log equations is found in the same section (4.4).

• A clear introduction to fundamental logarithmic properties has also been added to Section 4.4.

• Applications have been added and improved throughout the chapter.

• Regression material in this chapter has been removed and concentrated in the between-chapter Modeling with

Technology feature.

CHAPTER 5 Introduction to Trigonometric Functions

• Section 5.1 includes improved DMS to decimal degrees conversion coverage, improved introduction to standard

45-45-90 and 30-60-90 triangles, better illustrations of longitude and latitude applications, and streamlined/clarified

coverage of angular and linear velocity

• Section 5.2 has improved coverage of co-functions, and better illustrations for angles of elevation and depression

• Section 5.3 has improved applications, and the connection between f and f-1 is introduced

• Section 5.4 includes a table showing summary of trig functions of special angles

• Section 5.5 has improved coverage of secant and cosecant graphs

• Section 5.6 has a strengthened connection between y = tan x and y = (sin x)/cos(x)

• Section 5.7 has an improved introduction to transformations, and a clearer distinction between phase angle and

phase shift

CHAPTER 6 Trigonometric Identities, Inverses, and Equations

• Section 6.1 has an increased emphasis on what an identity is (the definition of an identity), as well as an additional

example of quadrant and sign analysis

• Section 6.2 has a better introduction to clarify goals, as well as an improved format for verifying identities

• Section 6.3 has improved coverage of the co-function identities, as well as extended coverage of the sum and

difference identities

• Section 6.5 has a strengthened connection between inverse functions and drawn diagrams, improved coverage on

evaluating the inverse trig functions, and more real-world applications of inverse trig functions

CHAPTER 7 Applications of Trigonometry

• Section 7.1 has consolidated coverage of the ambiguous case

• Section 7.2 has expanded coverage of computing areas using trig , as well as six new contextual applications of

triangular area using trig

• Section 7.3 has improved discussion, coverage, and illustrations of vector subtraction, and stronger connections

between solutions using components, and solutions using the law of cosines.

• Section 7.5 has additional real-world applications of complex numbers (AC circuits)

cob19529_fm_i-xl.indd Page xix 1/2/09 5:55:25 PM usercob19529_fm_i-xl.indd Page xix 1/2/09 5:55:25 PM user /Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10/Users/user/Desktop/Temp Work/January/02-12-09/VGP_02-01-09/MHBR104-10