CIMA - C3 Fundamentals Of Business Mathematics
Подождите немного. Документ загружается.

370 Answer bank
(iii) P(None owned) = (1 – P(H)) (1 – P(C)) (1 – P(TV))
= 0.5
× 0.4 × 0.1
= 0.02
(d) (i)
40%
(ii)
66.67%
Workings
Out of every 100 respondents, 50 own their own homes and 50 do not. This gives the bottom row of
the contingency table shown below. Similarly the total column is determined by the 60% of
respondents who own cars. Of the 50 home owners, 40 people (80%
× 50) also own cars. The table
can now be completed as follows.
Home owner
Not home owner
Total
Car
40
20
60
No car
10
30
40
Total
50
50
100
(i) Of the 50 respondents who do not own homes, only 20 own cars = 20/50
× 100% = 40%
(ii) Of the 60 who own cars, only 40 are home owners = 40/60
× 100% = 66.7%
53 B Expected profit = (15,000
× 0.2) + (19,000 × 0.6) + (–1,000 × 0.1)
= 3,000 + 11,400 – 100
= $14,300
If you selected option A, you have totalled all profits.
If you selected option C, you have selected the option with the highest probability.
If you selected option D, you have averaged the profits without using the probabilities.
54 B The diagram is a Venn diagram illustrating two mutually exclusive outcomes.
55 D The rule of addition for two events which are not mutually exclusive = P(A or B)
= P(A) + P(B)
– P(A and B)
If you selected option A, this is the rule of addition for two mutually exclusive events.
Option B is the simple multiplication or AND law.
56 C I, II and III are limitations of expected values.
57 A =
1
B =
6
C =
128
Answer bank 371
Workings
Red
Yellow
Green
Total
Good
25 – 1 = 24
50 – 6 = 44
75 – 15 = 60
128
Faulty
4%
× 25 = 1
12%
× 50 = 6
20% × 75 = 15
22
Total
25
50
75
150
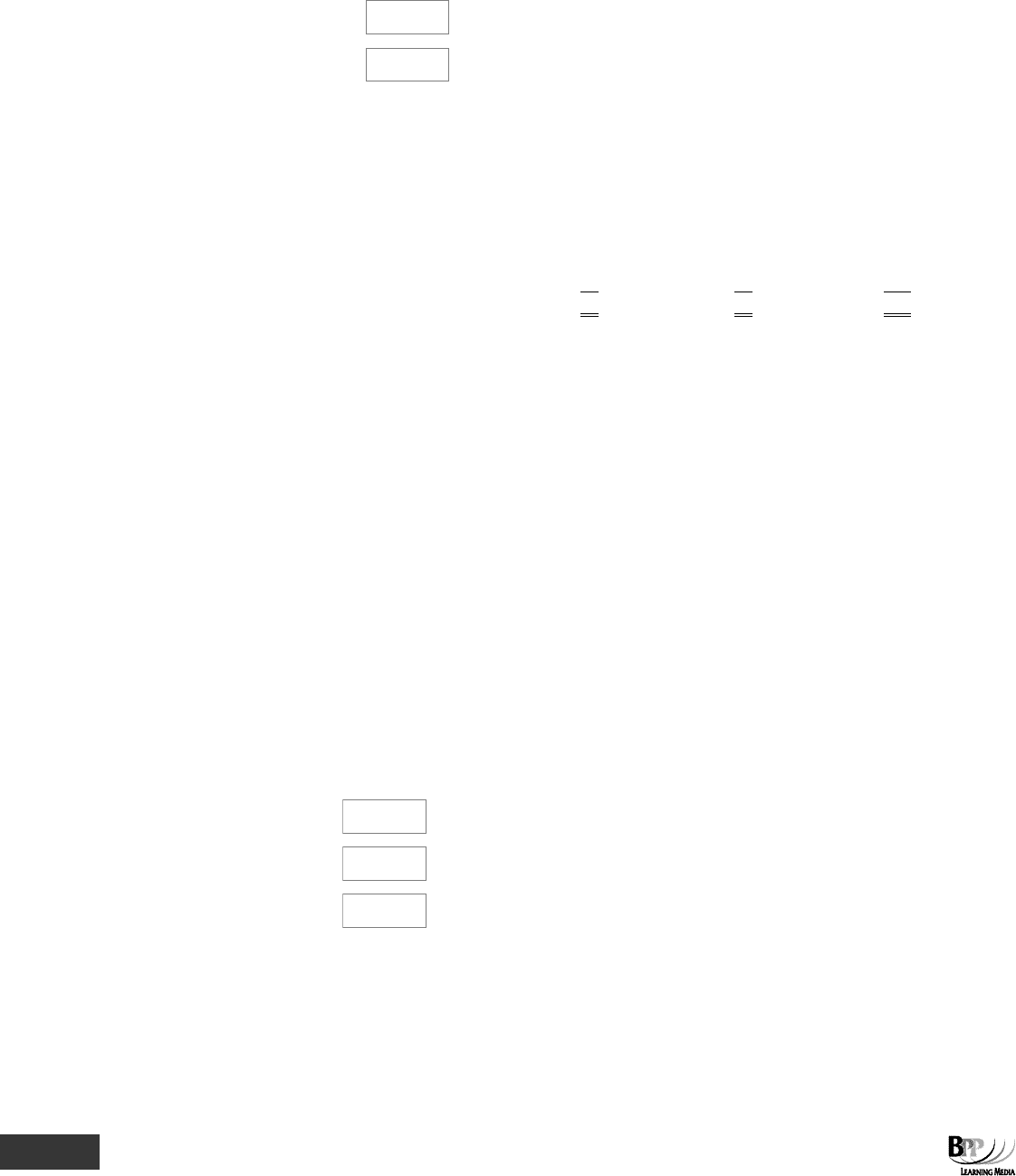
58 D
Pr(elephants weigh between 5,200 kg and 6,000 kg) = 0.5 – 0.0314
= 0.4686
From normal distribution tables, 0.4686 corresponds to a z value of 1.86.
If z =
σ
μx −
1.86 =
σ
200,5000,6 −
σ =
86.1
200,5000,6 −
= 430 kg
The correct answer is therefore D.
Make sure that you draw a sketch of the area that you are interested in when answering an objective
test question such as this. It will help to clarify exactly what you are trying to do.
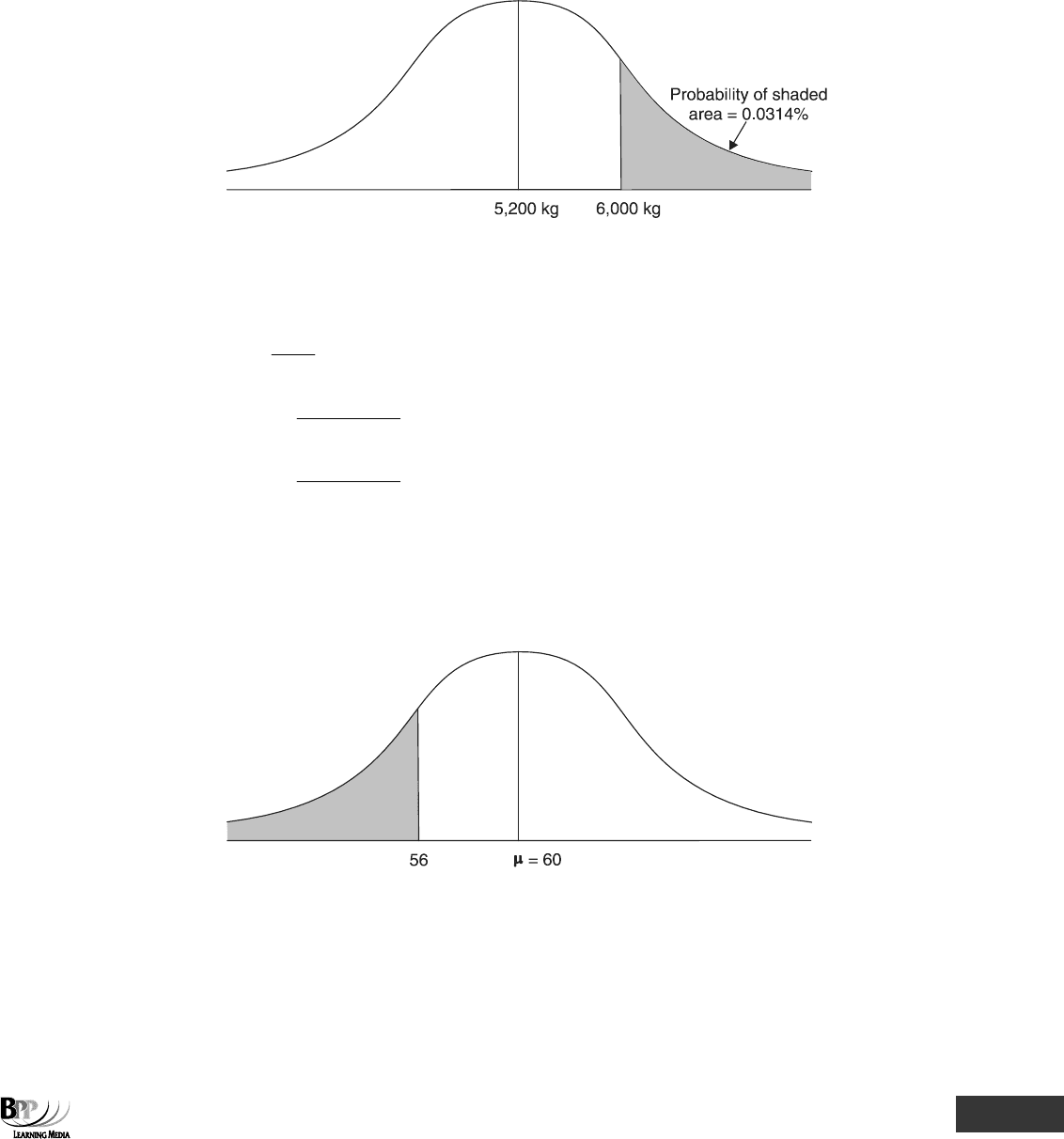
59 D
We are interested in the shaded area of the graph above, which we can calculate using normal
distribution tables.

372 Answer bank
z =
σ
μ−x
=
8.3
6056 −
= 1.05
A z value of 1.05 corresponds to a probability of 0.3531.
The shaded area has a corresponding probability of 0.5 – 0.3531 = 0.1469 or 0.15 or 15%.
Option A is incorrect because it represents the probability of getting a score of 56 or more.
Option B represents the probability of getting a score of 60 or less, ie 50% (the mean represents the
point below which 50% of the population lie and above which 50% of the population lie).
If you selected option C, you forgot to deduct your answer from 0.5.
60 D 28.23% of the population lies between 900 and the mean. Therefore 0.2823 corresponds to a z value
of 0.78 (from normal distribution tables).
If z =
σ
μx −
0.78 =
90
900 μ−
0.78 × 90 = 900 – μ
70.2 = 900 – μ
μ = 900 – 70.2
= 829.8 or 830
Draw a sketch of the area we are concerned with in this question if you had difficulty understanding the
answer.
61 A
We need to find the point z standard deviations above the mean such that 20% of the frequencies
are above it and 30% (50% – 20%) of the frequencies lie between the point z and the mean.
From normal distribution tables, it can be seen that 30% of frequencies lie between the mean and
the point 0.84 standard deviations from the mean.
Answer bank 373
If z =
σ
μx −
0.84 =
600,1
200x −
=
40
200x −
33.6 = x – 200
x = 200 + 33.6
= 233.6 or 234 (to the nearest whole number)
If you selected option B, you incorrectly added one standard deviation to the mean instead of 0.84
standard deviations.
If you selected option C you have added 1.28 standard deviations to the mean instead of 0.84
standard deviations.
If you selected option D, you have found 200 + (1.96 × 40) which accounts for 47.5% of the area
from 200 to point z.
62 (a)
1
(b)
2
+
3
(c)
4
(d)
2
+
3
+
4
=
2
+ 0.5
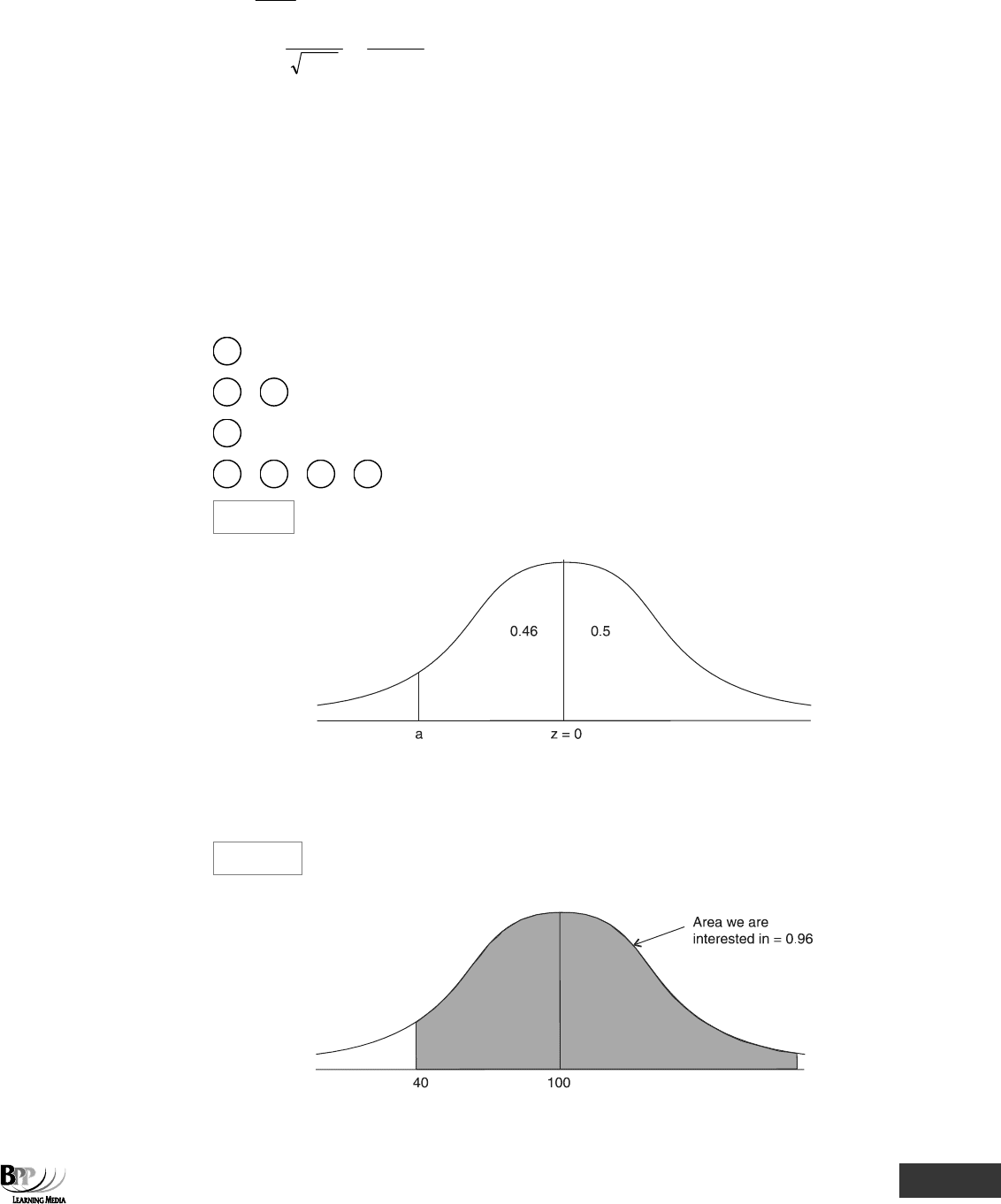
63 (a)
–1.75
Working
The normal distribution graph above shows the point a above which 96% of the population lies.
Normal distribution tables show that, a z value of 1.75 corresponds to a probability of 0.46. Since a
is less than 0, its value is –1.75.
(b)
34.3 hours
Working
374 Answer bank
Using z =
σ
μx −
where z = 1.75
x = 40
μ = 100
1.75 =
σ
− 10040
σ =
75.1
10040 −
= 34.3 hours (to 1 decimal place)
(c)
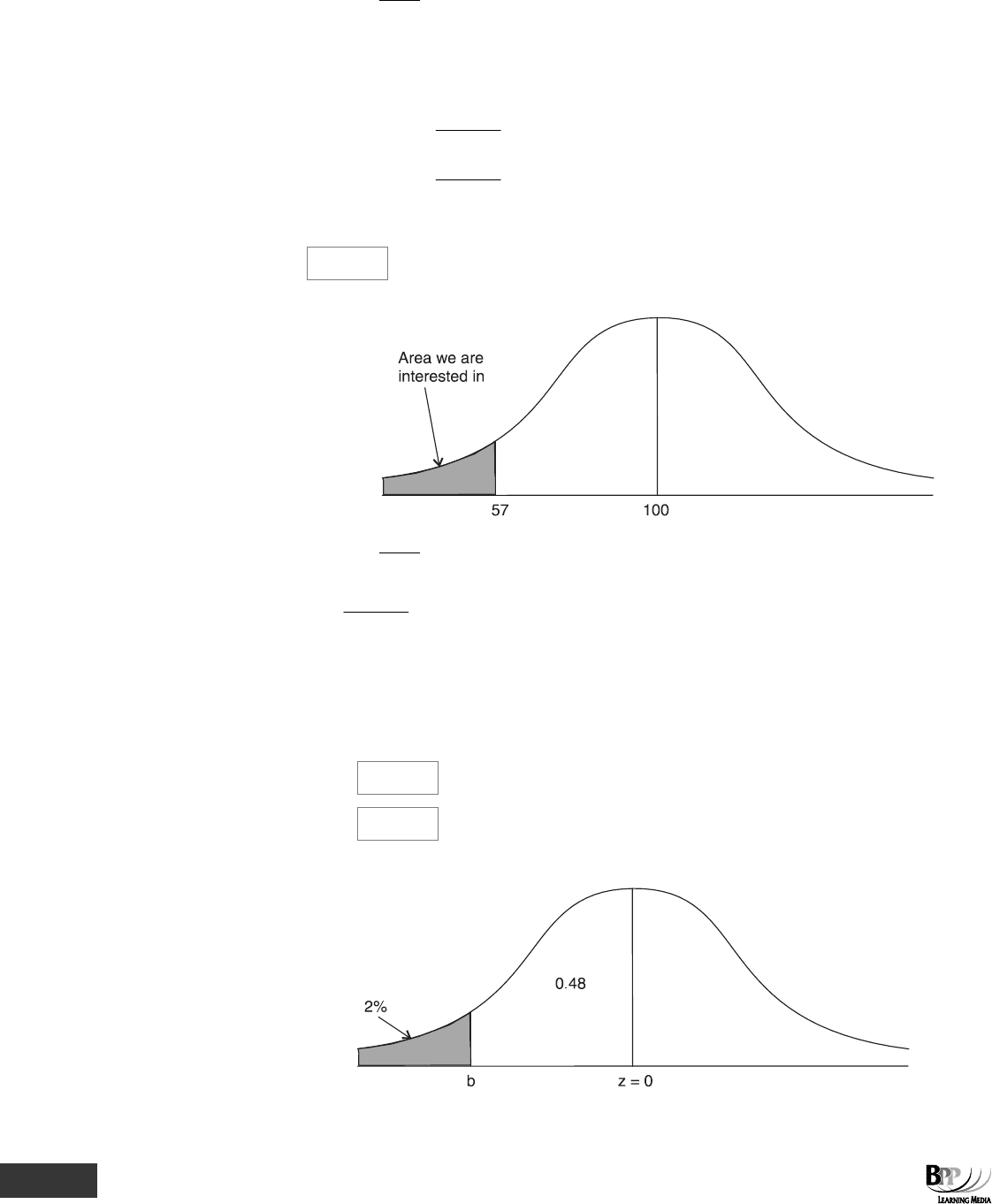
10.6%
Working
Using z =
σ
μ−x
z =
3.34
10057 −
z = 1.25
When z = 1.25, the proportion of batteries lasting between 57 and 100 hours is 0.3944 (from normal
distribution tables). The area that we are interested in is the area to the left of 57 hours (shaded area
on the graph) = 0.5 – 0.3944 = 0.1056 = 10.6% (to 1 decimal place).
(d) (i)
–2.05
(ii)
111.8
Workings
(1)
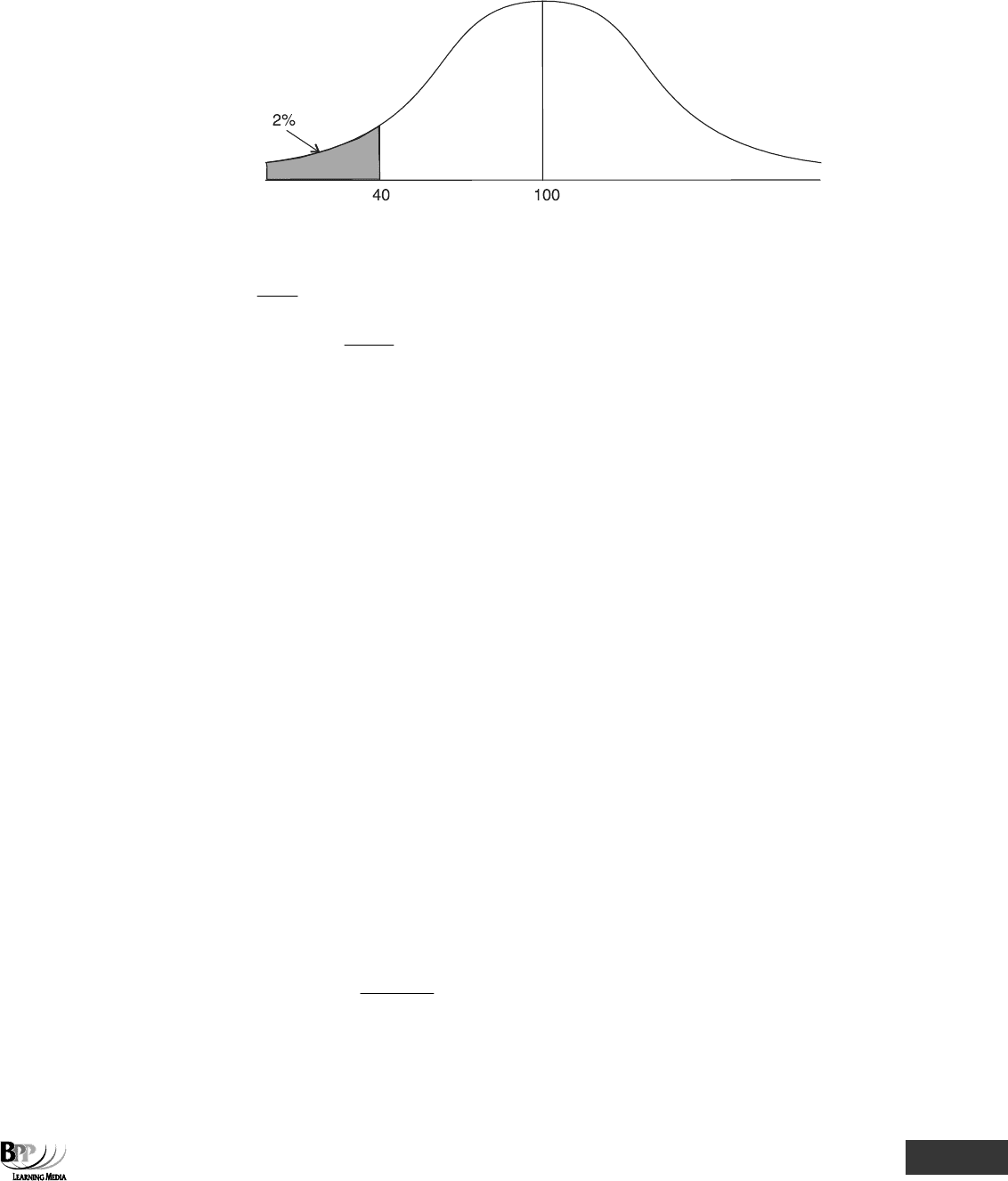
Answer bank 375
The area between b and 0 = 48% (0.48), and so from normal distribution tables, the value of
b shown in the graph above is –2.05.
(2)
We want 2% of batteries to last for less than 40 hours. From normal distribution tables, this
corresponds to a z value of –2.05.
If z =
σ
μ−x
–2.05 =
35
40 μ−
(–2.05 × 35) = 40 – μ
–71.75 = 40 – μ
μ = 40 + 71.75
= 111.75
= 111.8 (to 1 decimal place
64 C S = X + nrX
= $2,000 + (8 × 0.12 × $2,000)
= $2,000 + $1,920
= $3,920
If you selected option A, you calculated the interest element only and forgot to add on the original
capital value.
If you selected option B, you used n = 7 instead of n = 8.
If you selected option D, you used the compound interest formula instead of the simple interest
formula.
65 B If S = X + nrX
S – X = nrX (note S – X = interest element)
$1,548 – $900 = nrX
4 years = 4 × 12 = 48 months = n
∴ $648 = 48 r × $900
∴ r =
900£48
648£
×
r = 0.015 or 1.5%
If you selected option C, you misinterpreted 0.015 as 15% instead of 1.5%.

376 Answer bank
If you selected option D, you calculated the annual rate of interest instead of the monthly rate.
66 C $3,000 = 120% of the original investment
∴ Original investment =
120
100
× $3,000
= $2,500
∴ Interest = $3,000 – $2,500
= $500
Make sure that you always tackle this type of question by establishing what the original investment
was first.
If you selected option D, you simply calculated 20% of $3,000 which is incorrect.
67 C If a cost declines by 8% per annum on a compound basis, then at the end of the first year it will be
worth 0.92 the original value.
Now = $12,000
End of year 1 = $12,000 × 0.92
End of year 2 = $12,000 × 0.92
2
End of year 3 = $12,000 × 0.92
3
∴ At the end of year 3, $12,000 will be worth
$12,000 × (0.92)
3
= $9,344
If you selected option A, you calculated the value after four years, not three.
If you selected option B, you have assumed that the cost will decline by 8% × 3 = 24% over 3 years
therefore leaving a value of $12,000 – ($12,000 × 24%) = $12,000 – $2,880 = $9,120.
If you selected option D, you calculated the value after two years, not three.
68 A If house prices rise at 3% per calendar month, this is equivalent to
(1.03)
12
= 1.426 or 42.6% per annum
If you selected option B, you forgot to take the effect of compounding into account, ie 3% × 12 =
36%.
If you selected option C, you incorrectly translated 1.426 into 14.26% instead of 42.6% per annum.
If you selected option D, you forget to raise 1.03 to the power of 12, instead you multiplied it by 12.
69 (a)
15.87
%
Working
15% per annum (nominal rate) is 3.75% per quarter. The effective annual rate of interest is
[1.0375
4
– 1] = 0.1587 = 15.87%
(b)
26.82
%
Working
24% per annum (nominal rate) is 2% per month. The effective annual rate of interest is
[1.02
12
– 1] = 0.2682 = 26.82%

Answer bank 377
70 (a)
$2,941
Working
Value after 3 years = $8,000 × 1.11
3
= $10,941 (to the nearest $)
∴ Interest = $10,941 – $8,000
= $2,941
(b)
$13,881
Working
Value after 5 years = $15,000 × 1.14
5
= $28,881 (to the nearest $)
∴ Interest = $28,881 – $15,000
= $13,881
(c)
$3,601
Working
Value after 4 years = $6,000 × 1.1
2
× 1.15
2
= $9,601 (to the nearest $)
∴ Interest = $9,601 – $6,000
= $3,601
(d)
10.78%
Working
Rate of interest = 5.25% every six months
∴Six monthly ratio = 1.0525
∴Annual ratio = 1.0525
2
= 1.1078
∴Effective annual rate = 10.78% (to 2 decimal places)
(e)
12.68%
Working
Rate of interest = 1% every month
∴ Monthly ratio = 1.01
∴ Annual ratio = 1.01
12
= 1.1268
∴ Effective annual rate = 12.68% (to 2 decimal places)

378 Answer bank
(f)
12.55%
Working
Rate of interest = 3% per quarter
∴ Quarterly ratio = 1.03
∴ Annual ratio = 1.03
4
= 1.1255
∴ Effective annual rate = 12.55% (to 2 decimal places)
71 C Present value of the lease for years 1 – 9 = $12,000 × 5.328
Present value of the lease for years 0 – 9 = $12,000 × (1 + 5.328)
= $12,000 × 6.328
= $75,936
If you selected option A, you calculated the PV of the lease for years 1-9 only. If the first payment is
made now, you must remember to add 1 to the 5.328.
Option B represents the PV of the lease for years 1-10 (the first payment being made in a one year's
time), ie $12,000 × 5.650 = $67,800.
Option D is incorrect because it represents the PV of the lease for years 1-10 plus an additional
payment now (ie $12,000 × (1 + 5.650) = $79,800.
72 D Let A = annual repayments
These repayments, A are an annuity for 15 years at 9%.
Annuity (A) =
factor Annuity
mortgage ofPV
PV of mortgage = $60,000
Annuity factor = 8.061 (9%, 15 years from CDF tables)
∴ Annuity =
061.8
000,60$
= $7,440 (to the nearest $10)
If you selected option A you have confused mortgages with sinking funds and have calculated the PV
of the mortgage as if it occurred at time 15 instead of time 0.
If you selected option B you have forgotten to take account of the interest rates (ie 9% for 15 years).
You have simply divided $60,000 by 15.
If you selected option C, you have not taken into account the fact that the repayments happen at the
year end and that the first repayment is in one year's time and not now.
73 C The IRR can be calculated using the following formula.
IRR = a +
()
%ab
NPVNPV
NPV
b
a
a
⎥
⎦
⎤
⎢
⎣
⎡
−×
−

Answer bank 379
where a = 10%
b = 24%
NPV
a
= $460
NPV
b
= $320
IRR = 10% +
()
%1024
320$460$
460$
⎥
⎦
⎤
⎢
⎣
⎡
−×
−
= 10% + 46%
= 56%
If you selected option A, you have calculated the arithmetic mean of 10% and 24% instead of using
the IRR formula.
If you selected option B you have used an NPV of –$320 instead of +$320 in your calculation.
If you selected option D, you must realise that it is possible to use either two positive or two
negative NPVs as well as a positive and negative NPV. Using the former method, however, the
results will be less accurate.
74 B The payments made on a credit card are an annuity of $x per month.
From cumulative present value tables (3%, 12 periods) the annuity factor is 9.954.
If Annuity =
factor Annuity
annuityof PV
Annuity =
954.9
000,21$
= $2,110
If you selected option A, you have simply divided $21,000 by 12 without any reference to
discounting.
If you selected option C, you have misread the cumulative present value tables (and used the annuity
factor for 12% and 3 periods instead of 12 periods and 3%).
If you selected option D, you have calculated the present value of $21,000 in 12 time periods at a
discount rate of 3% instead of finding the monthly annuity whose present value over 12 months at
3% gives $21,000.
75 A Project A has the highest NPV. When comparing projects it is the NPV of each project which should
be calculated and compared. The correct answer is therefore A.
Mutually exclusive projects should not be selected by comparing the IRRs – Option D is therefore
incorrect, even though it has the highest IRR, it does not have the highest NPV.
Projects B and C do not have the highest NPVs either and so options B and C are incorrect.
