Christ M., Kenig C.E., Sadosky C. Harmonic Analysis and Partial Differential Equations: Essays in Honor of Alberto P. Calderon

Подождите немного. Документ загружается.

Chapter 1: Calderdn and Zygmund's Theory of Singular Integrals

[40]

Sur l'interpolation d'operations, C. R. Acad. Sci. Paris 208

(1939a), 1272-1273.

[41] ,

Sur les multiplicateurs des series de Fourier, Studia Math. 8

(1939b), 78-91.

[42] J. Marcinkiewicz and A. Zygmund, On a theorem of Lusin, Duke Math.

J. 4 (1938), 473-485.

[43] J. McNeal and E. M. Stein, The Szego projection in convex domains,

Math. Zeit 224 (1997), 519-553.

[44] Y. Meyer, Ondelettes et Operateurs (vols. I and II), Hermann, 1990.

[45] Y. Meyer and R. R. Coifman, Ondelettes et Operateurs (vol. III), Her-

mann, 1991.

[46] D. Muller, F. Ricci, and E. M. Stein, Marcinkiewicz multipliers and

multi-parameter structure on Heisenberg groups I, Inv. Math. 119 (1995),

199-233.

[47] A. Nagel, F. Ricci, and E. M. Stein, 1999, in preparation.

[48] A. Nagel, J. P. Rosay, E. M. Stein, and S. Wainger, Estimates for the

Bergman and Szego kernels in C2, Ann. of Math. 129 (1989), 113-149.

[49] J. Nash, Continuity of solutions of parabolic and elliptic equations, Amer.

J. of Math. 80 (1958), 931-954.

[50] 0. Nikodym, Sur les ensembles accessibles, Fund. Math.

10 (1927),

116-168.

[51] R. Palais, Seminar on the Atiyah-Singer index theorem, Ann. of Math.

Study no. 15, Princeton Univ. Press, 1965.

[52] D. H. Phong and E. M. Stein, Hilbert integrals, singular integrals and

Radon transforms I, Acta Math. 157 (1986), 99-157.

[53] I. Privalov, Sur une generalisation du theoreme de Fatou, Mat. Sbornik

3 (1923), 232-235.

[54] M. Riesz, Sur les fonctions conjuguees, Mat. Zeit. 27 (1927), 218-244.

[55] F. Ricci and E. M. Stein, Multiparameter singular integrals and maximal

functions, Ann. Inst. Fourier 42 (1992), 637-670.

Stein

[56] L. P. Rothschild and E. M. Stein, Hypoelliptic differential operators and

nilpotent groups, Acta Math. 137 (1976), 247-320.

[57] S. Saks, On the strong derivatives of functions of intervals, Fund. Math.

25 (1935), 235-252.

[58) A. Seeger, Singular integral operators with rough kernels, Jour. of the

A.M.S. 9 (1996), 95-106.

[59] R. T. Seeley, Singular integrals on compact manifolds, Amer. J. of Math.

81 (1959), 658-690.

[60] , Refinement

of the functional calculus of CaIderdn and Zygmund,

Indag. Math 27 (1965), 167-204.

[61]

, Elliptic

singular integrals, Proc. Symp. Pure Math. 10 (1967),

308-315.

[62] D: C. Spencer, A function-theoretic identity, Amer.

J. of Math. 65

(1943), 147-160.

[63] E. M. Stein, Singular Integrals and Differentiability Properties of Func-

tions, Princeton University Press, 1970.

[64]

Some problems in harmonic analysis suggested by symmetric

spaces and semisirnple groups, in Proc.

Int.

Congress Math. Nice I,

173-189, Gauthier-Villars, 1970.

[65]

, The

development of square functions in the work of A. Zyg-

mund, Bull. Amer. Math. Soc. 7 (1982), 359-376.

[66] , Harmonic

Analysis. Princeton University Press, 1992.

[67] E. M. Stein and S. Wainger, Problems in harmonic analysis related to

curvature, Bull. Amer. Math. Soc. 84 (1978), 1239-1295.

[68] T. Tao, Three regularity results in harmonic analysis, Ph.D. dissertation,

Princeton University.

[69] E. C. Titchmarsh, On conjugate functions, Proc. London Math. Soc.

29 (1929), 49-80.

[70] J. Unterberger and J. Bokobza, Les operateurs de Calderdn-Zygmund

precises, C. R. Acad. Sci. Paris 259 (1964), 1612-1614.

Chapter 1: Calderon and Zygmund's Theory of Singular Integrals

[71] A. Zygmund, On the differentiability of multiple integrals, Fund. Math.

23 (1934), 143-149.

[72]

, Complex

methods in the theory of Fourier Series, Bull. A.M.S.

49 (1943), 805-822.

[73]

On a theorem of Marcinkiewicz concerning interpolation of op-

erations, Jour. de Math. 35 (1956), 223-248.

[74] , Trigonometric Series, 2d ed., Cambridge Univ. Press, 1953.

Chapter 2

Transference Principles in

Ergodic Theory

Alexandra Bellow

The purpose of this paper is not to give a survey, but rather to discuss a few

selected topics having to do with transference in Ergodic Theory.

2.1 Classical Transference Principle

Let me first give some historical background.

Birkhoff's proof of the Pointwise Ergodic Theorem appeared in 1931 [1]

and received a great deal of attention. There have been many proofs of

Birkhoff's Pointwise Ergodic Theorem, in particular many proofs via the

Maximal Ergodic Inequality. Let me mention two of the early ones, both

going back to 1939: the Kakutani-Yosida paper [2] giving a sharp form of

the Maximal Ergodic Inequality and Wiener's celebrated paper on the Dom-

inated Ergodic Theorem [3] giving the dominated estimate in LP for the

Ergodic Maximal Function. More on Wiener's paper later.

Now there is another celebrated paper that preceded Birkhoff's Proof

of the Ergodic Theorem by one year, namely the Hardy-Littlewood paper

in Acta Mathematica, 1930 [4] (the years 1930, 1931 were spectacular years

for analysis). In their fundamental paper, Hardy and Littlewood introduced

what is now known as the Hardy-Littlewood Maximal Function and proved

various maximal inequalities, dominated estimates in LP, 1 < p < oo, and

much more. Having some knowledge of the game of cricket helps in develop-

ing a feeling for the "basic inequality" in the early part of the paper. For

Research partially supported by NSF grant DMS-9303327.

Chapter 2: Transference Principles in Ergodic Theory

it was the game of cricket-Hardy's "other" great passion-- that motivated

this "basic inequality." For Hardy, who practiced Mathematics for the inter-

nal esthetics of Mathematics, this may be the closest he ever came to practical

applications in his mathematical work. At any rate, one quickly realizes that

this "basic inequality" implies what is now known as the Hardy-Littlewood

Weak-Type Maximal Inequality, even though the Weak-Type Maximal In-

equality is not explicitly stated as such in the Hardy-Littlewood paper.

If one thinks of the set of integers Z as a totally or-finite measure space

with mass 1 at each point, then translation by 1 is a measure-preserving

transformation and the Hardy-Littlewood Maximal Function is precisely the

Maximal Ergodic Function. Thus the Weak-Type Inequality and the Dom-

inated Estimates in L" for the Hardy-Littlewood Maximal Function follow

from the corresponding estimates for the Ergodic Maximal Function.

The remarkable fact is that the converse is also true: The Maximal Er-

godic Inequality and the Dominated Ergodic Estimates in LI can be derived

from the corresponding results for the Hardy-Littlewood Maximal Function.

This is now known as the basic "transference principle" in Ergodic Theory.

To the best of my knowledge, the story of the transference principle begins in

1939 with N. Wiener's paper on the Dominated Ergodic Theorem. Wiener

first proves a version of the Hardy-Littlewood Maximal Inequality using a

Vitali-type covering argument and then uses a transference argument to de-

rive the corresponding Maximal Inequality in the abstract dynamical system.

The technique is roughly this: consider the function along the orbit, one or-

bit at a time, then integrate and apply Fubini's Theorem making use of the

measure-preserving character of the transformations. My guess is that this

was a natural approach for Wiener to try. As a very young man, he had

spent some time at Cambridge University, where he came under Hardy's in-

fluence. Wiener greatly admired Hardy, whom he considered his "master in

mathematical training." So it should not come as a surprise that when he

learned about the Maximal Ergodic Inequality, he tried to derive it from the

Hardy-Littlewood Inequality.

It appears that Aurel Wintner was also aware of the possibility of trans-

ferring a deterministic inequality to the ergodic setting. Wintner suggested

the idea to Hartman who implemented it in yet another proof of the Maximal

Ergodic Inequality [51; in fact, he derived the Maximal Ergodic Inequality

from F. Riesz's Sunrise Lemma, via a transference argument.

Finally, in 1968, Alberto Calderdn formulated the general Transference

Principle in Ergodic Theory in his beautiful paper "Ergodic Theory and

translation-invariant operators" [6J. His motivation was to derive the Ergodic

Hilbert Transform of Cotlar from the ordinary Hilbert Transform.

People in Harmonic Analysis were quick to grasp the Calderdn Transfe-

rence Principle. Motivated also by the Calderon-Zygmund work on singular

integrals (in particular the rotation method that allowed the transference

Bellow

of results about the Hilbert Transform from the 1-dimensional to the n-

dimensional setting), and beginning in the early 1970s, R. Coifman and G.

Weiss embarked on a remarkable program to extend the framework of the

transference principle further: they replaced the group Z or JR by a more gen-

eral (locally compact, amenable) group G and the measure-preserving action

by a continuous representation of G acting on some Banach space. This has

been a very active area of research, in which Earl Berkson and his collab-

orators also figure prominently. But I shall not dwell on this, because this

is somewhat outside the scope of the paper. I would like to recall, however,

that Coifman and Weiss [7] showed how to transfer the strong-type (p, p)

maximal inequality in the case when the operators of the representation are

positivity-preserving, using the lovely transference argument of A. de la Torre

[8]. For transference of strong-type (p, p) maximal inequalities, respectively

weak-type maximal inequalities in the case of separation-preserving represen-

tations, see [9] (this paper also contains some interesting counterexamples),

respectively [10]; see also [11].

It is a curious fact that the Calderon Transference Principle took longer

to reach the ergodic circles per se. There were still books on Ergodic Theory

appearing in the 1980s-excellent books otherwise-that barely mentioned

Calderbn's paper, unaware of the transference principle. In the 1980s I recall

hearing J. Bourgain lecture on the proof of the remarkable ergodic theorems

along the sequence of squares and along the sequence of primes ([12], [13],

[14]) and making use of transference arguments-that was a novelty in those

days. I learned about the Calderon Transference Principle in 1987. Calderon

gave me a reprint of his paper and said: "You may find this of some inter-

est." Did I ever! By now the Calderon Transference Principle has become a

standard tool, a classic in Ergodic Theory, and I am happy to report that

singular integrals are a "household word" in Ergodic Theory as well.

Transference techniques, in various guises, have become common prac-

tice in Ergodic Theory.

Let me give a small sample.

In [15], Jones and

Olsen show how to transfer a maximal inequality from one positive invertible

isometry on LP to any other, while later, in [16], Jones, Olsen, and Wierdl

show how to transfer oscillation (variational) inequalities from the integers

to positive invertible isometries.

This allows them, using Akcoglu's Dila-

tion Theorem, to extend various pointwise ergodic theorems, known in the

measure-preserving case, to Dunford-Schwartz operators [15], respectively,

to positive contractions on LP [16]. Another interesting ergodic theorem ob-

tained via transference arguments is given in [17]. See also the important,

beautiful recent work on ergodic theorems in the case of non-abelian groups

of Nevo [18] and Nevo and Stein [19].

I shall now state the Calderon Transference Principle not in its full gen-

erality, but in the discrete case, in a form which suffices for our purposes.

Chapter 2: Transference Principles in Ergodic Theory

Let p be a probability on Z. If cp E £'(Z) define

(pip)(p) = Ep(j)co(p+ j),

for

p E Z.

jEZ

Let (X, A, m, T) be an abstract dynamical system (that is, (X, A, m)

is a probability space and r

: X --> X an invertible measure-preserving

transformation). If f E Ll = L' (X) define

(pf)(x) =

p(j)f(r x), for x E X.

jEZ

Note that we use Greek letters to denote functions on Z and ordinary

letters to denote functions on X.

Theorem 2.1 (Transference Principle). Let (p,,) be a sequence of prob-

ability measures on Z. Consider the assertions:

(i) There is a constant C > 0 such that

# {jEZ : SuP(pnOu) > Al <

IIWjIi for all cp E 2+(Z), A > 0

(that is, we have a weak-type (1,1) inequality on R1(Z))

(ii) There is a constant C > 0 such that for every abstract dynamical

system (X, A, m, r) we have

m(xEX :sup(pnf)(x)>A} IIfI11 for all fEL+(X),A>0

It n

(that is, we have a weak-type (1, 1) inequality in the ergodic context, i.e., on

LI(X))

Then (i) = (ii).

Comments:

1. The constant C is the same in (i) and (ii).

2. One can remove the restriction on the abstract dynamical system that

the total mass m(X) = 1; one can allow a-finite measure spaces (X, A, m)

and one obtains an equivalent statement.

3. The Transference Principle also applies if we replace the weak-type

(1, 1) estimate by a "weak-type (p, p) estimate" (respectively a "strong-

type (p, p) estimate") for 1 < p < oo. The moral of the story is that

if we have the estimate for the dynamical system of the integers with

translations, we can derive it for any other dynamical system.

Bellow

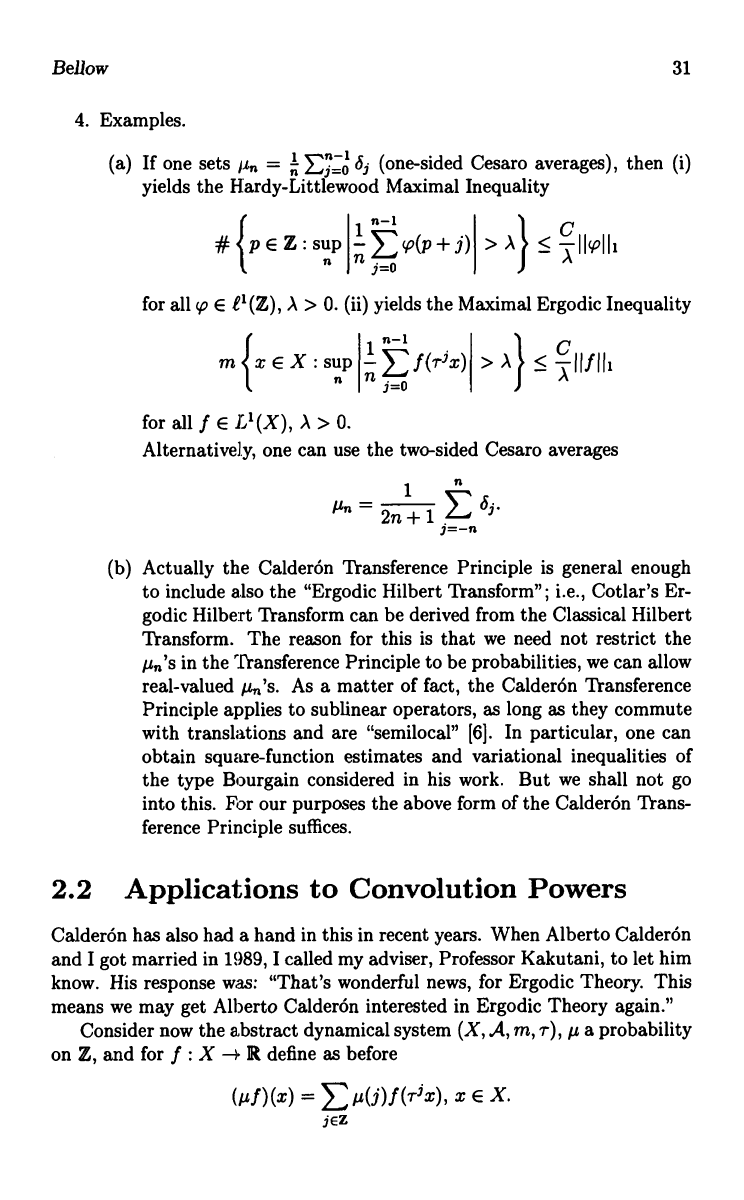
4. Examples.

(a) If one sets jLn = n Ej o 6j (one-sided Cesaro averages), then

yields the Hardy-Littlewood Maximal Inequality

(i)

1 n-1 C

# PEZ:suP -EcP(P+j) >A <-IkPIIi

n n

j=o

for all cp E el(Z), A > 0. (ii) yields the Maximal Ergodic Inequality

m{xEX:sup

ll n

l rnn

6j_

j=-n

(b) Actually the Calderon Transference Principle is general enough

to include also the "Ergodic Hilbert Transform"; i.e., Cotlar's Er-

godic Hilbert Transform can be derived from the Classical Hilbert

Transform. The reason for this is that we need not restrict the

An's in the Transference Principle to be probabilities, we can allow

real-valued As a matter of fact, the Calderon Transference

Principle applies to sublinear operators, as long as they commute

with translations and are "semilocal" [6]. In particular, one can

obtain square-function estimates and variational inequalities of

the type Bourgain considered in his work. But we shall not go

into this. For our purposes the above form of the Calderon Trans-

ference Principle suffices.

2.2

Applications to Convolution Powers

Calderon has also had a hand in this in recent years. When Alberto Calderon

and I got married in 1989, I called my adviser, Professor Kakutani, to let him

know. His response was: "That's wonderful news, for Ergodic Theory. This

means we may get Alberto Calderon interested in Ergodic Theory again."

Consider now the abstract dynamical system (X, A, m, ,r), p a probability

on Z, and for f : X -+ R define as before

(p f) (x) = E p(j) f (T1 x), x E X.

n-1

f (Tix)

j=0

>A}<

1011

for all f EL'(X), A > 0.

Alternatively, one can use the two-sided Cesaro averages

jEZ

Chapter 2: Transference Principles in Ergodic Theory

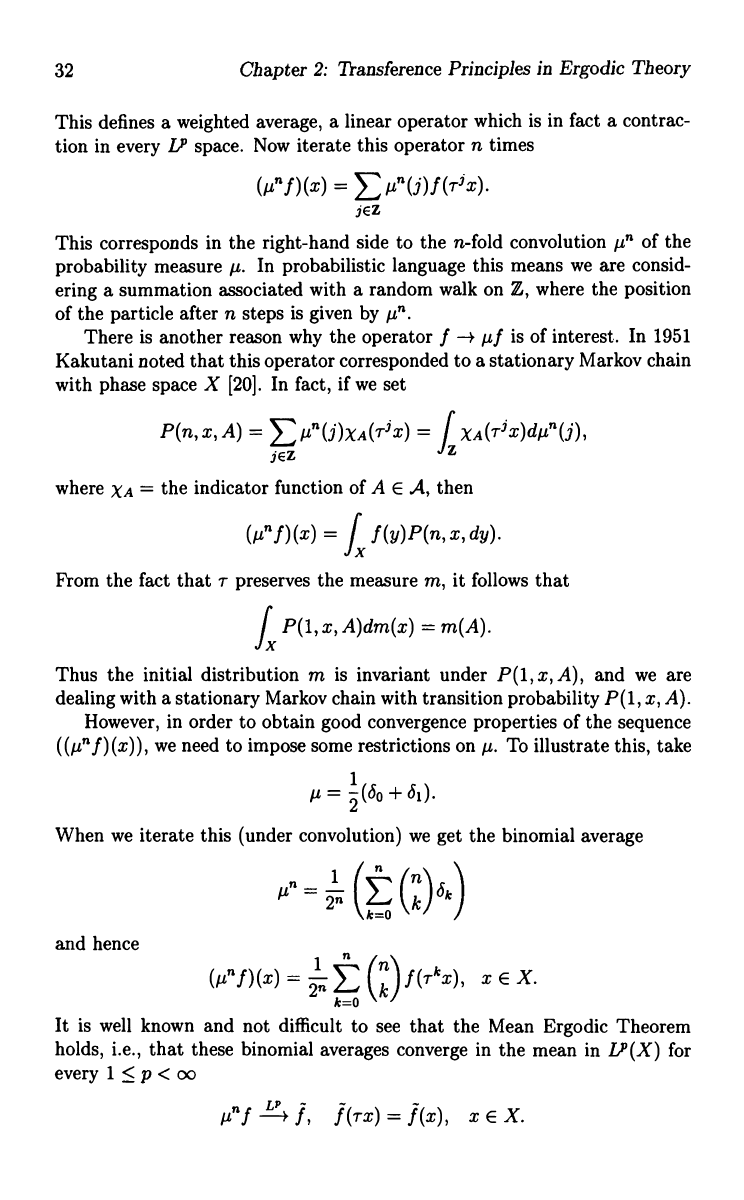
This defines a weighted average, a linear operator which is in fact a contrac-

tion in every LP space. Now iterate this operator n times

(pnf)(x) =

pn(7)f(T'x)

jEZ

This corresponds in the right-hand side to the n-fold convolution An of the

probability measure p. In probabilistic language this means we are consid-

ering a summation associated with a random walk on Z, where the position

of the particle after n steps is given by pn.

There is another reason why the operator f -+ p f is of interest. In 1951

Kakutani noted that this operator corresponded to a stationary Markov chain

with phase space X [20]. In fact, if we set

P(n, x, A) = 1: pn(i)XA(r'x) =

fxA(r'x)diz(i)where

XA = the indicator function of A E A, then

(pnf)(x) =

jf(y)P(n,x,dY).

From the fact that T preserves the measure m, it follows that

P(1, x, A)dm(x) = m(A).

Thus the initial distribution m is invariant under P(1, x, A), and we are

dealing with a stationary Markov chain with transition probability P(1, x, A).

However, in order to obtain good convergence properties of the sequence

((pn f)(x)), we need to impose some restrictions on p. To illustrate this, take

2(60+61).

When we iterate this (under convolution) we get the binomial average

= 2n

(()ok)

k=0

and hence

(pnf)(x) =

(k)

f (Tkx), X E X.

k=0

It is well known and not difficult to see that the Mean Ergodic Theorem

holds, i.e., that these binomial averages converge in the mean in LP(X) for

every 1 < p < 00

pnf L f, f(Tx) = f(x),

x E X.

Bellow

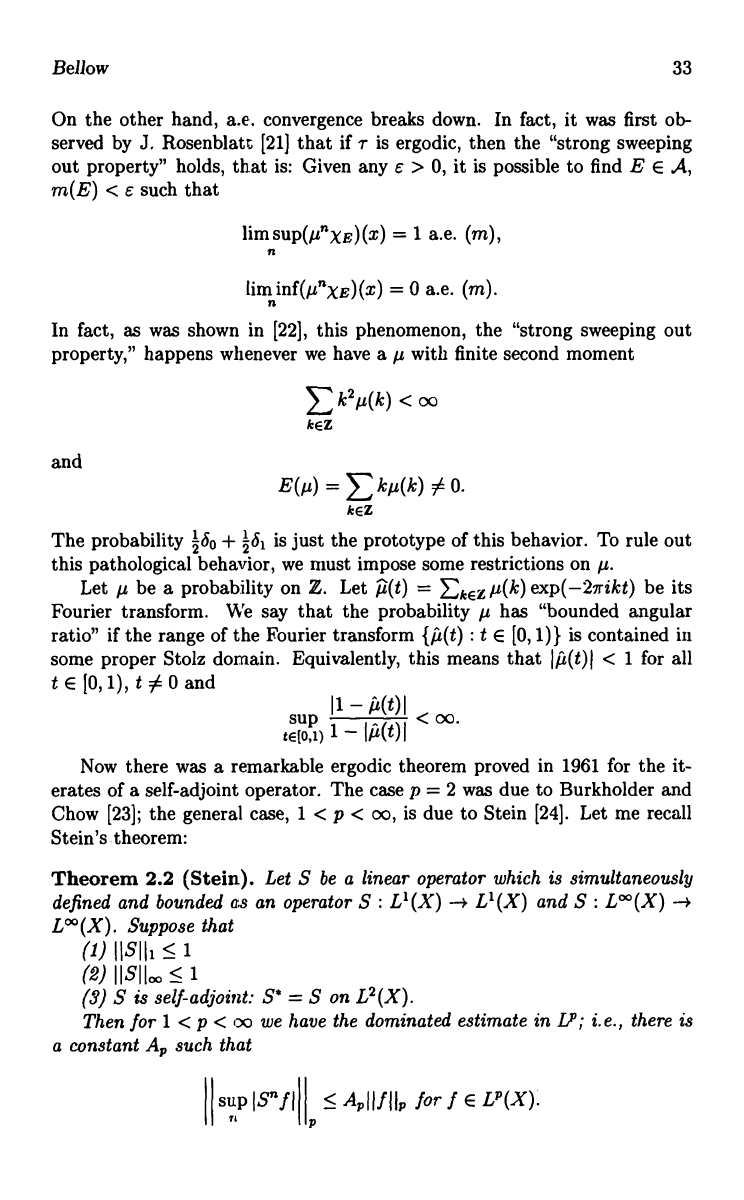
On the other hand, a.e. convergence breaks down. In fact, it was first ob-

served by J. Rosenblatt [21] that if r is ergodic, then the "strong sweeping

out property" holds, that is: Given any e > 0, it is possible to find E E A,

m(E) < e such that

limsup(p'XE)(x) = 1 a.e. (m),

liminf(p"XE)(x) = 0 a.e. (m).

In fact, as was shown in [22], this phenomenon, the "strong sweeping out

property," happens whenever we have a p with finite second moment

E k2p(k) < 00

kEZ

and

E(p) = kp(k) # 0.

kEZ

The probability 2So + 1bl is just the prototype of this behavior. To rule out

this pathological behavior, we must impose some restrictions on A.

Let p be a probability on Z. Let µ(t) = >kEZ p(k) exp(-21rikt) be its

Fourier transform. We say that the probability p has "bounded angular

ratio" if the range of the Fourier transform {µ(t) : t E [0, 1)} is contained in

some proper Stolz domain. Equivalently, this means that IA(t)[ < 1 for all

tE 10,I), t960 and

sup

11 - A(t)I

< 00.

tEl0,1) 1 - (p(t)I

Now there was a remarkable ergodic theorem proved in 1961 for the it-

erates of a self-adjoint operator. The case p = 2 was due to Burkholder and

Chow [23]; the general case, 1 < p < oo, is due to Stein [24]. Let me recall

Stein's theorem:

Theorem 2.2 (Stein). Let S be a linear operator which is simultaneously

defined and bounded as an operator S : L'(X) -+ Ll(X) and S : L1* (X) -+

L°°(X). Suppose that

(1) IISII1 < 1

(2) IISII,c < 1

(9) S is self-adjoint: S' = S on L2(X).

Then for 1 < p < oo we have the dominated estimate in LP; i.e., there is

a constant AP such that

supISnfl

<API[f{[p for f E LP(X).