Chiodi M. An innovative 3D-CFD-Approach towards Virtual Development of Internal Combustion Engines

Подождите немного. Документ загружается.

156 10 3D-CFD-Modeling of the Wall Heat-Transfer

In order to ensure the same “thermodynamic conditions” during the comparison (see Figure

10.5), the necessary average values of the variables in the real working-process analysis have

been automatically provided by the evaluation tool implemented in

QuickSim (see Chapter 7.4).

In this work, the heat-transfer models chosen in the real working-process analysis are:

Bargende’s correlation during the compression and expansion stroke (working cycle).

Hohenberg’s correlation during the intake and exhaust stroke (gas exchange period).

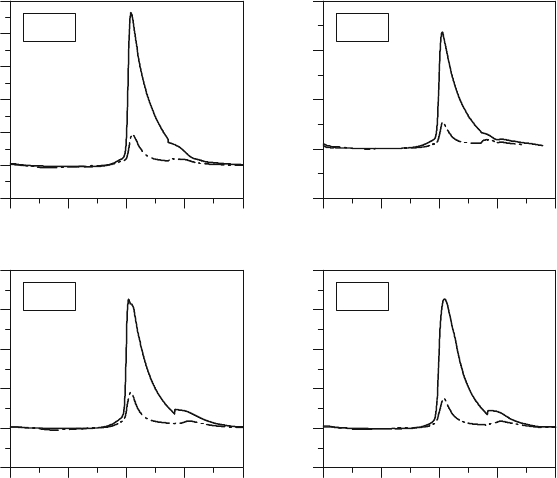
Figure 10.6: Comparison between the 3D-CFD-heat-transfer (WF: wall-function model) and

the real working-process analysis (WP) under the same “thermodynamic conditions”.

Here a representative selection of numerous comparisons is reported. In cases 1 und 2 (see Table

10.1) two operating conditions of an old mass production engine are reported. Case 3 and 4 refer

to test engines with very simple combustion chamber geometries, so that it is possible to

implement a 3D-CFD-mesh with a high structure quality (low warpage angle, optimal internal

Case 1

Hea

ans

M, J

-1.0

0.0

1.0

2.0

3.0

4.0

5.0

Crank an

, de

TDC BDC FTDC BDC TDC

Case 2

Hea

ans

M, J

-1.0

0.0

1.0

2.0

3.0

Crank an

, de

TDC BDC FTDC BDC TDC

Case 3

Hea

ans

M, J

-1.0

0.0

1.0

2.0

3.0

4.0

Crank an

, de

TDC BDC FTDC BDC TDC

Hea

ans

M, J

-1.0

0.0

1.0

2.0

3.0

4.0

Crank an

, de

TDC BDC FTDC BDC TDC

Case 4

10.2 State-of the-Art of Engine Heat-Transfer Calculation in the 3D-CFD-Simualtion 157

face angle, optimal aspect ratio and a better fulfilling of the



-condition [56]) at any piston

position.

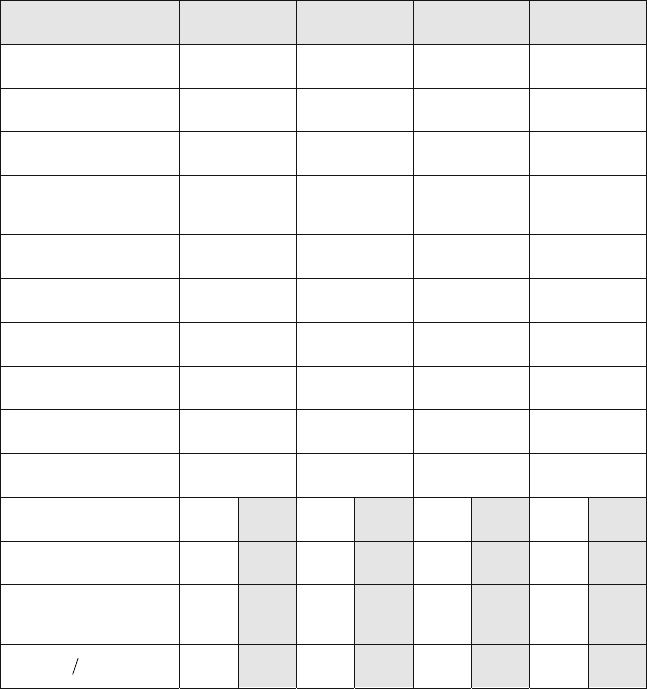
Table 10.1: Comparison: 3D-CFD wall heat-transfer calculation

with wall function models (WF) vs. real working-process analysis (WP).

Specifications: Case 1 Case 2 Case 3 Case 4

Engine MB M102-E23 MB M102-E23 Test-Engine Test-Engine

No. of valves/cyl.- 2 2 2 4

Cyl. displacement, cm

575 575 480 480

Combustion chamber

shape

Bowl +

squish area

Bowl +

squish area

Pent-roof Flat

rpm,- 3,000 1,500 4,000 3,000

Load, - WOT Low WOT WOT

imep, bar 10.8 3.4 10.5 9.5

3D-CFD-Mesh,

Cells

15,000 15,000 45,000 50,000

Cell type, - Hexahedral Hexahedral Hexahedral Hexahedral

Simulated fuel, - C

WF WP WF WP WF WP WF WP

Heat release

, J/cycle

1684 1727 621 619 1455 1455 1324 1311

Heat transfer

J/cycle

62.3 352.4 61.5 202.4 77.1 286.6 66.2 308.1

Ratio

QQ , %

3.7 20.4 9.9 32.7 5.3 19.7 5.0 23.5

The four cases show that the heat-transfer prediction using the wall function is in any case not

plausible. Due to the high mesh-structure quality, even in cases 3 and 4 no remarkable

improvements have been achieved. These results confirm the analyses of other authors [11]. The

158 10 3D-CFD-Modeling of the Wall Heat-Transfer

reason of this inaccuracy, as explained at the beginning of this chapter, lays in an inadequate

physical formulation and numerical implementation. In particular the turbulence in the near-wall

region cannot be predicted correctly. Since the turbulence variables are the most predominant

inputs in the heat-transfer formulation based on the wall function, it becomes evident that even

an improved formulation will not be able to achieve a general validation until its inputs are

reliable.

10.2.4.1 Sensitivity Analysis of the 3D-CFD-Heat-Transfer calculated with a

Wall-Function Model

A sensitivity analysis of the 3D-CFD-heat-transfer calculation with a traditional wall-function

model (WF) using as test object a HYDRA-research-engine from Ricardo with four different

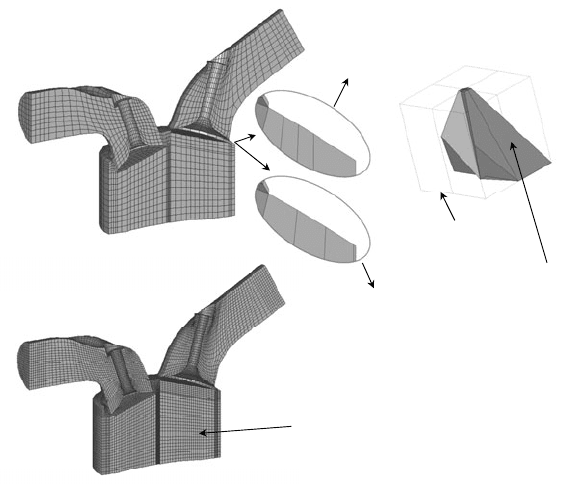
mesh structures or refinement degrees has been performed (see Figure 10.7).

Figure 10.7: Sensitivity analysis with four different mesh structures or refinement degrees

(HYDRA research-engine – 2 valves).

Standard mesh (coarse)

Fine mesh (Sim. 4)

Standard

spark plug

Deformed

spark plug

(Sim. 3)

Thin cell

layer (0.1 mm)

(Sim. 2)

Normal cell layer

(Sim. 1)

Half discretization length

than coarse mesh

10.2 State-of the-Art of Engine Heat-Transfer Calculation in the 3D-CFD-Simualtion 159

The simulated engine has a simple combustion chamber shape with a very high mesh quality so

that a modification of the mesh can be well targeted and accurate investigations of their effects

on the simulation results can be performed. The four meshes of the two-valve research engine

differ as follows:

(Sim. 1 – “Standard”) – Standard coarse mesh with ca. 15,000 cells in the combustion

chamber.

(Sim. 2 – “Thin Layer”) – Standard coarse mesh with a thin cell layer of 0.1 mm at the

cylinder liner.

(Sim. 3 – “Bad SP”) – Standard coarse mesh with a deformed spark plug (“bad” spark

plug with locally high aspect ratios and warpage-angles of the cells).

(Sim. 4 – “Fine”) – Fine mesh with half discretization length compared to the standard

coarse one (

~120,000 cells in the combustion chamber).

The simulated operating condition reported here is 2,000 rpm at WOT with the same boundary

conditions. The turbulence is modeled by a

H

standard model.

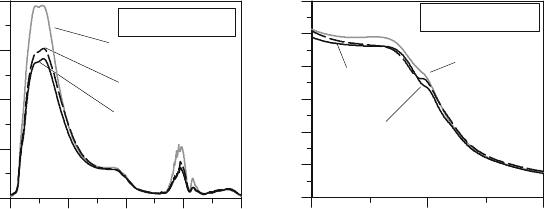
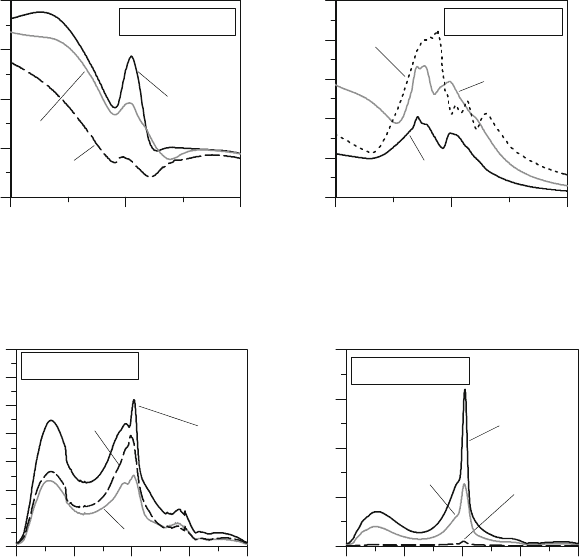
As expected, during the exchange period the average cylinder turbulence TKE



, due to high

local velocity gradients, shows remarkable differences only with the simulation of the fine mesh

(see Figure 10.8). In contrast the profile of



during the working period, in all cases, shows

much smaller discrepancies. Since the influence of the turbulence on the heat-transfer and

consequently on the engine energy-balance is moderate during the exchange period, the overall

variable



can be assumed as a reliable input for heat-transfer modeling.

Figure 10.8: Turbulence TKE in the cylinder during the whole cycle

and the working period.

2000 rpm - WOT

Thin layer

Fine

Standard &

Bad SP

Cyl. turb. kinetik energy, m

120

160

Crank an

, de

TDC BDC FTDC BDC TDC

2000 rpm - WOT

Thin

layer

Fine

Standard &

Bad SP

urb. k

k energy, m

Crank an

le M, de

630 FTDC 810

160 10 3D-CFD-Modeling of the Wall Heat-Transfer

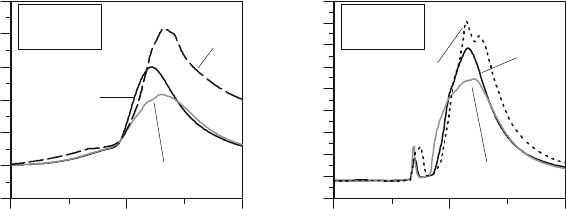
Looking now at the local values of the turbulence within the cells at the wall where the wall

function is applied (boundary layer cells), the differences become more considerable (see Figures

10.9 and 10.10). Focusing on the working period, the influences of dimension, orientation and

structure quality of the wall-cells on the turbulence becomes evident. In a moving complex

geometry there is no possibility to control any cell and eventually isolate “perturbations” on the

local turbulence calculation. Therefore the simulation of the local heat-transfer with a wall-

function approach is very often practically “out of control” since one of its most important input

variables is not reliable.

Figure 10.9: Average TKE at the

cylinder-liner wall-cells.

Figure 10.10: Average TKE at the

spark-plug wall-cells.

Figure 10.11: Average y

-value of the cylinder

wall-cells.

Figure 10.12: Average y

-value of the

cylinder-liner wall-cells.

2000 rpm - WOT

Thin

layer

Fine

Standard &

Bad SP

ner T

E, m

Crank an

le M, de

630 FTDC 810

2000 rpm - WOT

Bad SP

Fine

Standard &

Thin Layer

E a

he spark p

ug, m

Crank an

le M, de

630 FTDC 810

Standard &

Bad SP

Fine

Thin

layer

2000 rpm - WOT

Cyl. wall cells y

, -

100

150

200

250

300

350

Crank an

le M, de

TDC BDC FTDC BDC TDC

2000 rpm - WOT

Thin

layer

Fine

Standard &

Bad SP

ner wa

s y

, -

250

500

750

1000

Crank an

le M, de

TDC BDC FTDC BDC TDC

10.2 State-of the-Art of Engine Heat-Transfer Calculation in the 3D-CFD-Simualtion 161

Figure 10.13: Percentage of

cylinder wall-cells with



<30.

Figure 10.14: Percentage of

cylinder wall-cells with



>100.

The optimal dimension for the wall-function approach is when the dimensionless wall-distance



assumes values between 30 and 100 units (see Chapter 10.2.1). Here the analysis of



for

the four simulations points out the differences to this assumption and the mesh sensitivity on the

results (see Figures 10.11-10.14). The



-value depends not only on the local mesh structure

but also on the flow motion whose transient state varies locally and temporally with high

gradients. Nevertheless the flow motion in general depends on the engine operating condition.

Therefore if it were possible to tune the mesh structure and the mesh motion for fulfilling the



-conditions at any location and time step, this process would be definitely compromised at a

different operating condition. For the above mentioned reasons the wall function approach in

internal combustion engines cannot rely on optimal



-conditions.

Figure 10.15: Average heat-transfer flux through the cylinder walls during the whole cycle

and the working period.

Standard &

Bad SP

Fine

Thin

layer

2000 rpm - WOT

Cyl. wall cells y

<30, %

100

Crank an

le M, de

TDC BDC FTDC BDC TDC

Standard &

Bad SP

Fine

Thin

layer

2000 rpm -

WOT

Cyl. wall cells y

>100, %

100

Crank an

le M, de

TDC BDC FTDC BDC TDC

2000 rpm -

WOT

Thin layer

Fine

Standard &

Bad SP

. hea

ansfe

ux, MW/m

-0.2

0.0

0.2

0.4

0.6

0.8

1.0

Crank an

, de

TDC BDC FTDC BDC TDC

2000 rpm -

WOT

Thin

layer

Fine

Standard &

Bad SP

. hea

ansfe

ux, M

-0.2

0.0

0.2

0.4

0.6

0.8

1.0

Crank an

, de

630 FTDC 810

162 10 3D-CFD-Modeling of the Wall Heat-Transfer

Figure 10.16: Average heat-transfer flux

through the cylinder-liner walls.

Figure 10.17: Average heat-transfer flux

through the spark plug walls.

Concluding the analysis of the heat-transfer flux using the wall-function approach (see Figures

10.11-10.12) reports the extreme high sensitivity of the heat-transfer flux with the mesh. The

results of the different simulations show variations of about 500% in the prediction of the local

heat-flux (in certain cases even more) and up to 100% in the prediction of the overall heat-

transfer energy

(J/cycle). The resulting predictability in the calculation of the heat-transfer

and the energy balance is obviously not acceptable for a reliable engine analysis.

10.2.5 QuickSim’s Approach: A new Phenomenological Heat-

Transfer Model in the 3D-CFD-Simulation

In this work a three-dimensional phenomenological heat-transfer coefficient



MD ,x

, which is

based on the before described Re-Nu-correlations (see Chapters 10.2.3.3 and 10.2.3.4), is

proposed for both the working cycle



MD ,

and the charge changing period



MD ,

. This

model is implemented locally, like the wall function, in only a single wall-cells layer, so that

computing overheads are not caused. The classic wall function is still used for assuring the no-

slip velocity condition and the temperature condition at the wall.

The modeling of the heat-transfer coefficients chosen for the 3D-CFD-simulation, similarly to

the real working-process analysis, is based on the following correlations [21,22]:

Bargende’s correlation during the compression and expansion stroke (working cycle).

Hohenberg’s correlation during the intake and exhaust stroke (gas exchange period).

2000 rpm -

WOT

Thin

layer

Fine

Standard &

Bad SP

HT f

ux, M

-0.2

0.0

0.2

0.4

0.6

0.8

1.0

Crank an

, de

630 FTDC 810

2000 rpm -

WOT

Standard &

Thin layer

Fine

Bad SP

. spa

k p

ug HT f

ux, M

-0.2

0.0

0.2

0.4

0.6

0.8

1.0

1.2

1.4

1.6

Crank an

, de

630 FTDC 810

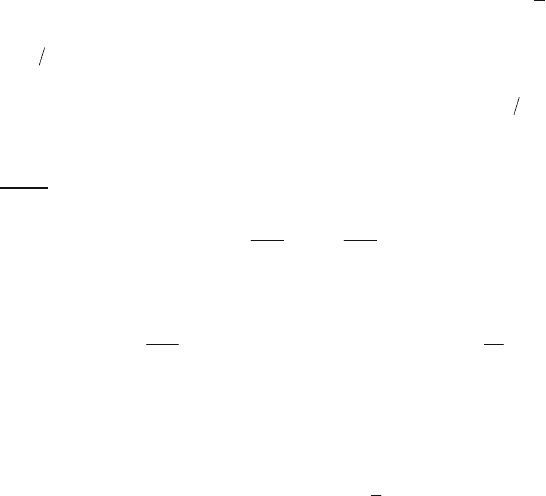
10.2 State-of the-Art of Engine Heat-Transfer Calculation in the 3D-CFD-Simualtion 163

10.2.5.1 The Heat-Transfer during the Working Cycle

The effects of the heat transfer on the working cycle behavior are remarkable because, e.g. the

ratio of the heat losses to the total heat transfer usually amounts to more than 80% and it is still

more than 45% during the combustion period only.

The aim of this work is to develop a three-dimensional heat transfer model



MD ,

, which,

first of all, ensures a high accuracy in predicting the overall heat transfer rate



CFD

ddQ M

at every time step. This condition is a prerequisite for a thorough

prediction of the engine energy balance. In this manner, the overall heat transfer rate

calculated from the real working-process analysis (WP)



ddQ M

is taken

as the representative value.

Target

CFD

(10.18)

with

 

MMD

jjWjCj

CFD

AxTxTx

&&&

(10.19)

where



is the temperature at the center node of a cell

adjacent to the wall with the

surface

and a constant temperature



over the time.

Starting from the known formulation of Bargende:





' MD



,,,,,),(

78.0477.078.0073.0

wTpVafx

(10.20)

where

a is a corrector factor for the local implementation and having the detailed results of the

flow field in the 3D-CFD-simulation at disposal, it is now necessary to find out how to set

appropriately the variables for each wall-cell

in this formulation.

This is a critical choice which requires not only to identify the phenomenon-relevant value for

each variable but also to avoid unreliable variables (e.g. local values of

k and H of wall-cells –

see Chapter 10.2.4.1). Many trial-and-error adjustments in setting the variables in



D ,

have been tested in order to reach the best compromise between overall and local heat transfer

rate prediction, high accuracy on different engines, high accuracy at different engine operating

conditions and low influence of the mesh structure on the results.

164 10 3D-CFD-Modeling of the Wall Heat-Transfer

Model Development Step

The development tests of



D ,

have been performed by using both the evaluation tool and

the real working-process analysis program (WP) implemented in

QuickSim via user-subroutines

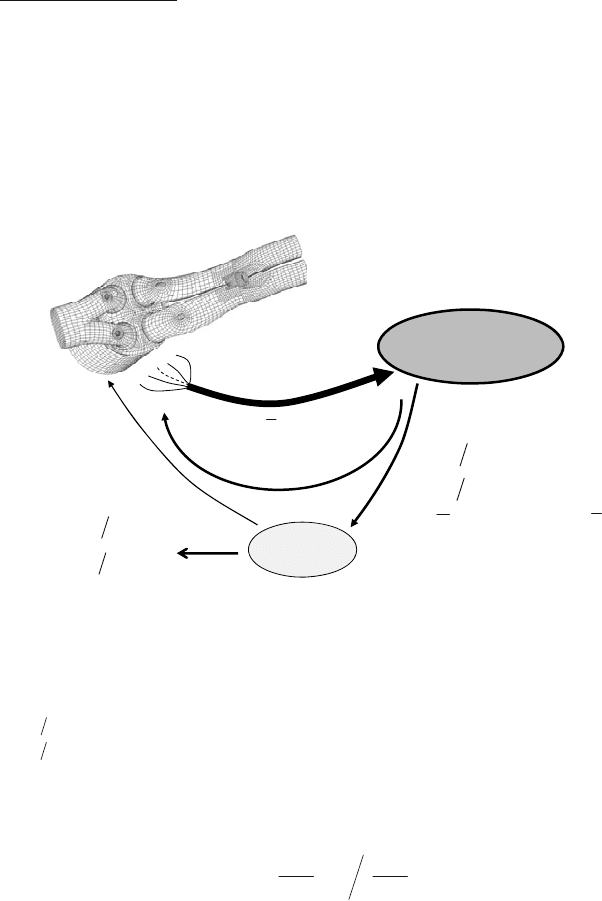
(see Chapter 7). This becomes an "internal 3D-CFD-WP coupling" (see Figure 10.18) as the next

feasible step of the result comparison shown in Figure 10.5. This approach ensures not only the

same “thermodynamic conditions” between 3D-CFD and WP, but also provides, at every time

step, the necessary average values of the variables used in the real working-process analysis to

the three-dimensional simulation.

Figure 10.18: The decisive step: the “internal coupling” between a 3D-CFD-code and

the real working-process analysis.

Choosing a variant of



D ,

, which is mostly a combination of global (averaged overall

variables) and local variables, respectively, the heat-transfer rate of the CFD-simulation



CFD

ddQ M

has been calculated and compared to that of the working-process analysis



ddQ M

. If they differed, at every time step, an overall correction variable



c (see

Eq. 10.21) is determined and set in the 3D-CFD-simulation (see Eq. 10.22), in order to correct

the wall heat flux (see Eq. 10.23). In this manner at least, during the model developing phase, a

representative overall heat transfer rate has been imposed as a boundary condition.



CFD

(10.21)

Evaluation

Tool



,

ddQ







&&&

,,,,

xvxT



,,,,,,,

kwVVTTp

ddQ

BBUG

CFD

3D-CFD-

Simulation



 

...,,

10.2 State-of the-Art of Engine Heat-Transfer Calculation in the 3D-CFD-Simualtion 165

With the calculation of



the heat-transfer coefficient has to be corrected:



D

D ,,

11 jHT

corr

xcx

(10.22)

so that:



, WP

CFD

corrCFD

M

(10.23)

The phenomenological model was not validated until the heat-transfer correction variable



became approximately a constant

, so that this value can be included in the constant

of Eq.10.20, leading to:





' MD



,,,,,),(

78.0477.078.0073.0

wTpVafx

(10.24)

where:

caa 

(10.25)

In addition to the above mentioned approach that uses the validated model with a constant value

, the user of QuickSim has also the possibility to run a simulation with an automatic

adjustment of



. In this case the profile of the calculated 3D-CFD-heat-transfer (actual

value) will always equal that one of the real working-process analysis (set value).

Final Formulation

In this section the numerical implementation procedure of each variable in the phenomenological

model is described for the final version of



D ,

Volume

The instantaneous cylinder volume



is taken as the characteristic geometrical entity in

which the heat-transfer process takes place.

Pressure

The pressure in the combustion chamber of an internal combustion engine can be assumed as

uniform. The local value of the pressure in the wall-cell



is taken as the relevant value.