Астафьева Н.М. Вейвлет-анализ. Основы применения и примеры применения

Подождите немного. Документ загружается.

ÆÇÕÂÎË Ô ÏÂÔÛÕÂÃÂÏË ÑÍÑÎÑ 40 ÎÇÕ (Ä ÍÑÐÙÇ ÓâÆÂ ÑÐË

ÏÇÐÇÇ ËÐÕÇÐÔËÄÐÞ). £ ÔÇÓÇÆËÐÇ ÓËÔÖÐÍÂ àÕÂ 40-ÎÇÕÐââ

ÒÇÓËÑÆËÚÐÑÔÕß ÒÓÇÓÞÄÂÇÕÔâ ì ÊÆÇÔß ÄËÆÐÞ ÐÇÔÍÑÎßÍÑ

ÆÇÕÂÎÇÌ Ô ÏÂÔÛÕÂÃÂÏË ÑÍÑÎÑ 25 ë 30 ÎÇÕ, ÍÑÕÑÓÞÇ ×ÑÓÏË-

ÓÖáÕ ÆÄÇ ÍÓÖÒÐÑÏÂÔÛÕÂÃÐÞÇ ÆÇÕÂÎË (ÒÓËÃÎËÊËÕÇÎßÐÑ

85 ë 90 ÎÇÕ). ¬ÓÑÏÇ ÕÑÅÑ, ÏÑÉÐÑ ÑÕÏÇÕËÕß ÐÇÔÍÑÎßÍÑ

ÆËÂÒÂÊÑÐÑÄ ÎÑÍÂÎßÐÞØ ÒÇÓËÑÆËÚÐÑÔÕÇÌ Ô ÏÂÔÛÕÂÃÂÏË

ÑÕ 8 ÆÑ 11 ÎÇÕ (Ë Ô ÇÜÇ ÏÇÐßÛËÏË).

®ÂÔÛÕÂÃ 9 ë 10 ÎÇÕ ÑÃÞÚÐÑ ÔÄâÊÞÄÂáÕ Ô 18,6-ÎÇÕÐËÏ

ÎÖÐÐÞÏ ÙËÍÎÑÏ, ÍÑÕÑÓÞÌ ÒÓËÔÖÕÔÕÄÖÇÕ Ä ÆËÐÂÏËÍÇ

ÊÂÔÖØ, ÐÂÄÑÆÐÇÐËÌ Ë Ä ØÑÆÇ ÕÇÏÒÇÓÂÕÖÓÞ Ä ³ÇÄÇÓÐÑÌ

¡ÏÇÓËÍÇ [24]. ®ÂÔÛÕÂÃ ÑÍÑÎÑ 40 ÎÇÕ ÏÑÉÇÕ ÃÞÕß ÔÄâÊÂÐ

Ô 70 ë 80-ÎÇÕÐËÏ ÙËÍÎÑÏ ÔÑÃÞÕËÌ ¿Îß-¯ËÐßÑ, ÑÕÏÇÚÇÐ-

ÐÞÏ Ä [20, 25]; ÒÓËÚËÐÞ ÅÇÐÇÓÂÙËË àÕÑÅÑ ÙËÍÎÂ ÒÑÍÂ

ÐÇâÔÐÞ. ±ÑÒÞÕÍË ÔÄâÊÂÕß ÇÅÑ Ô ÄÖÎÍÂÐËÚÇÔÍÑÌ ÆÇâÕÇÎßÐÑ-

ÔÕßá [26] ÐÇ ÒÓÇÆÔÕÂÄÎâáÕÔâ ÑÃÑÔÐÑÄÂÐÐÞÏË. ´ÓÇÕËÌ

ØÂÓÂÍÕÇÓÐÞÌ ÏÂÔÛÕÂÃ, ÑÍÑÎÑ 150 ÎÇÕ (ÇÏÖ ÔÑÑÕÄÇÕ-

ÔÕÄÖáÕ ÕÓË ÔÂÏÞÇ ÍÓÖÒÐÑÏÂÔÛÕÂÃÐÞÇ ÆÇÕÂÎË ÐÂ

ÓËÔ. 13Ã), ÐÂØÑÆËÕÔâ ÐÂ ÅÓÂÐËÙÇ ÕÑÚÐÑÔÕË ËÊ-ÊÂ ÍÑÐÇÚÐÑÌ

ÆÎËÐÞ ÂÐÂÎËÊËÓÖÇÏÑÅÑ ÓâÆÂ Ë ÏÑÉÇÕ ÃÞÕß ÒÓËÊÐÂÐ

ÐÇÆÑÔÕÑÄÇÓÐÞÏ.

´ÂÍËÏ ÑÃÓÂÊÑÏ, ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËÇ, ÑÆÐÂ ËÊ

ÔËÎßÐÞØ ÔÕÑÓÑÐ ÍÑÕÑÓÑÅÑ ÔÑÔÕÑËÕ Ä ÄÑÊÏÑÉÐÑÔÕË ÂÐÂ-

ÎËÊÂ ÔÕÓÖÍÕÖÓÞ ÐÇÑÆÐÑÓÑÆÐÞØ ÒÓÑÙÇÔÔÑÄ, ÒÑÍÂÊÂÎÑ

ÔÎÇÆÖáÜÇÇ. ³ÖÜÇÔÕÄÖáÕ ÎÑÍÂÎßÐÞÇ ÒÇÓËÑÆËÚÐÑÔÕË

ÔÑÃÞÕËÌ ¿Îß-¯ËÐßÑ Ô ÏÂÔÛÕÂÃÂÏË ÑÕ 8 ÆÑ 11 ÎÇÕ;

ÐÇÑÃØÑÆËÏÑ ÆÑÒÑÎÐËÕÇÎßÐÑÇ ËÊÖÚÇÐËÇ ÄÑÒÓÑÔÂ, ÔÄâÊÂÐÞ

ÎË ÑÐË Ô 18,6-ÎÇÕÐËÏ ÎÖÐÐÞÏ ÙËÍÎÑÏ ËÎË 22-ÎÇÕÐËÏ

ÙËÍÎÑÏ ÔÑÎÐÇÚÐÑÌ ÂÍÕËÄÐÑÔÕË. ° ÏÂÔÛÕÂÃÂØ ÏÇÐßÛÇ 7 ë

8 ÎÇÕ ÒÓË ÅÑÆËÚÐÞØ ÆÂÐÐÞØ ÅÑÄÑÓËÕß ÐÇ ËÏÇÇÕ ÔÏÞÔÎÂ.

£ÇÌÄÎÇÕ-ÂÐÂÎËÊ ÐÇ ÒÑÍÂÊÞÄÂÇÕ ÖÔÕÑÌÚËÄÑÅÑ 70 ë 80-

ÎÇÕÐÇÅÑ ÙËÍÎÂ ÔÑÃÞÕËÌ ¿Îß-¯ËÐßÑ: ÔÖÜÇÔÕÄÖÇÕ ÐÇÔÍÑÎß-

ÍÑ ÒÓÑÆ ÑÎÉËÕÇÎßÐÞØ àÒÑØ ÒÑÄÕÑÓâáÜÇÌÔâ ÆÎËÕÇÎßÐÑÔÕË

(ÑÍÑÎÑ 40 ÎÇÕ), ÒÇÓËÑÆËÚÐÑÔÕß ÍÑÕÑÓÞØ ÒÓÇÓÞÄÂÇÕÔâ Ä

ÔÇÓÇÆËÐÇ ÓâÆÂ. ±ÓËÚËÐÞ àÕÑÅÑ ÒÓÇÓÞÄÂÐËâ ÒÑÍÂ ÐÇâÔÐÞ.

°ÐÑ ÏÑÉÇÕ ÃÞÕß ÑÃÖÔÎÑÄÎÇÐÑ Ë ÑÃÝÇÍÕËÄÐÞÏË ×ËÊËÚÇ-

ÔÍËÏË ÒÓËÚËÐÂÏË, Ë ÔÖÃÝÇÍÕËÄÐÞÏË: ÂÐÂÎËÊËÓÖÇÏÞÇ

ÆÂÐÐÞÇ ÓÇÍÑÐÔÕÓÖËÓ ÑÄÂÐÞ ÂÄÕÑÓÑÏ ÓÂÃÑÕÞ [21] ÒÑ ÃÑÎß-

ÛÑÏÖ ÐÂÃÑÓÖ ÍÑÔÄÇÐÐÞØ ÔÄËÆÇÕÇÎßÔÕÄ, Ë ÑÕÔÖÕÔÕÄËÇ

ÐÇÍÑÕÑÓÞØ ËÊ ÐËØ (ÊÂ ÔÑÑÕÄÇÕÔÕÄÖáÜËÌ ÔÇÓÇÆËÐÇ ÓâÆÂ

ÒÇÓËÑÆ ÄÓÇÏÇÐË) ÏÑÅÎÑ ÒÓËÄÇÔÕË Í ËÔÍÂÉÇÐËá ÍÂÓÕËÐÞ.

¯ÇÍÑÕÑÓÞÇ ÒÓËÊÐÂÍË (ÐÂÒÓËÏÇÓ, ÔÑØÓÂÐÇÐËÇ ×ÂÊÞ ÍÑÎÇ-

ÃÂÐËâ) ÖÍÂÊÞÄÂáÕ ÐÂ ÄÑÊÏÑÉÐÑÔÕß ÔÖÜÇÔÕÄÑÄÂÐËâ ÖÔÕÑÌ-

ÚËÄÑÅÑ 70 ë 80-ÎÇÕÐÇÅÑ ÙËÍÎÂ ÔÑÃÞÕËÌ ¿Îß-¯ËÐßÑ. °

ÃÑÎÇÇ ÆÎËÕÇÎßÐÞØ àÒÑØÂØ ÐÇ ÒÑÊÄÑÎâÇÕ ÅÑÄÑÓËÕß ÍÑÐÇÚ-

ÐÂâ ÆÎËÐÂ ÓâÆÂ.

±ÓË ËÐÕÇÓÒÓÇÕÂÙËË ÓÇÊÖÎßÕÂÕÑÄ ÐÇÑÃØÑÆËÏÑ

ÒÑÏÐËÕß, ÚÕÑ ÂÐÂÎËÊËÓÖÇÏÞÇ ÆÂÐÐÞÇ ÓÇÍÑÐÔÕÓÖËÓÑÄÂÐÞ

ÒÑ ÍÑÔÄÇÐÐÞÏ ÔÄËÆÇÕÇÎßÔÕÄÂÏ Ë, ÍÓÑÏÇ ÕÑÅÑ, ÐÇ ÔÑÆÇÓÉÂÕ

ËÐ×ÑÓÏÂÙËË ÐË Ñ ÆÎËÕÇÎßÐÑÔÕË, ÐË ÑÃ ËÐÕÇÐÔËÄÐÑÔÕË

ÒÓÑÙÇÔÔÂ (ÑÐË ÑÕÓÂÉÂáÕ ÕÑÎßÍÑ ÐÂÎËÚËÇ ËÎË ÑÕÔÖÕÔÕÄËÇ

ÔÑÃÞÕËâ ¿Îß-¯ËÐßÑ Ä ÕÇÚÇÐËÇ ÅÑÆÂ) Ë ÐÇ ÔÑÆÇÓÉÂÕ

ËÐ×ÑÓÏÂÙËË ÑÃ ÂÐÕË-¿Îß-¯ËÐßÑ, ËÎË Â-¯ËÐßÂ, âÄÎâá-

ÜÇÅÑÔâ ÐÇÑÕÝÇÏÎÇÏÑÌ ÚÂÔÕßá ÒÓÑÙÇÔÔÂ À¬¿¯. ®ÐÑÅÑ

ÃÑÎßÛÇ Ë ÅÑÓÂÊÆÑ ÃÑÎÇÇ ÆÑÔÕÑÄÇÓÐÑÌ ËÐ×ÑÓÏÂÙËË ÔÑÆÇÓ-

ÉÂÕ ÆÂÐÐÞÇ ÐÂÃÎáÆÇÐËÌ ÊÂ ËÊÏÇÐÇÐËâÏË ËÐÆÇÍÔÂ

ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ ÊÂ ÒÑÔÎÇÆÐÇÇ ÔÕÑÎÇÕËÇ.

6.3. ³ÓÇÆÐÇÏÇÔâÚÐÞÇ ÊÐÂÚÇÐËâ

ËÐÆÇÍÔÂ ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ

¯ÂÒÑÏÐËÏ, ÚÕÑ ËÐÆÇÍÔ ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ Ct ì àÕÑ

ÑÒÓÇÆÇÎÇÐÐÞÏ ÑÃÓÂÊÑÏ ÐÑÓÏËÓÑÄÂÐÐÂâ ÓÂÊÐÑÔÕß ÂÕÏÑ-

Ô×ÇÓÐÑÅÑ ÆÂÄÎÇÐËâ, ËÊÏÇÓâÇÏÑÅÑ ÐÂ ÖÓÑÄÐÇ ÏÑÓâ ÐÂ

ÔÕÂÐÙ ËâØ, ÓÂÔÒÑÎÑÉÇÐÐÞØ ÄÃÎËÊË ÙÇÐÕÓÑÄ ÆÇÌÔÕÄËâ

ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ. °ÃÞÚÐÑ ÑÐ ÄÞÚËÔÎâÇÕÔâ ÔÎÇÆÖá-

ÜËÏ ÑÃÓÂÊÑÏ. ¢ÇÓÖÕÔâ ÄÓÇÏÇÐÐ



yÇ ÓâÆÞ ÆÂÄÎÇÐËâ ÔÕÂÐ-

ÙËÌ ´ÂËÕË Ë ¥ÂÓÄËÐÂ p

y; m

(ËÐÆÇÍÔÞ y, m ÑÃÑÊÐÂÚÂáÕ ÅÑÆ Ë

ÏÇÔâÙ). ±Ñ ÐËÏ ÄÞÚËÔÎâáÕÔâ ÓâÆÞ ÐÑÓÏÂÎËÊÑÄÂÐÐÑÅÑ

ÆÂÄÎÇÐËâ ÔÕÂÐÙËÌ P

y; m



y; m

ÿ hp



=e, ÊÆÇÔß hp

i ì

ÔÓÇÆÐÇÇ ÏÐÑÅÑÎÇÕÐÇÇ ÊÐÂÚÇÐËÇ (ÐÑÓÏÂ), ÄÞÚËÔÎÇÐÐÑÇ ÒÑ

ÔÓÇÆÐÇÏÇÔâÚÐÞÏ ÆÂÐÐÞÏ ÊÂ ÒÇÓËÑÆ 1951 ë 1980 ÅÅ.; e ì

ÔÕÂÐÆÂÓÕÐÑÇ ÑÕÍÎÑÐÇÐËÇ, ÄÞÚËÔÎÇÐÐÑÇ ÒÑ ÄÔÇÏ ÊÐÂÚÇÐËâÏ

ÂÐÑÏÂÎ ËÌ ÆÂÄÎÇÐËâ ÊÂ ÒÇÓËÑÆ 1951 ë 1980 ÅÅ. ©ÂÕÇÏ

ÄÞÚËÔÎâáÕÔâ ÐÑÓÏÂÎËÊÑÄÂÐÐÞÇ ÂÐÑÏÂÎËË ÆÂÄÎÇÐËâ

ÔÕÂÐÙËÌ ´ÂËÕË (ËÐÆÇÍÔ ´) Ë ¥ÂÓÄËÐÂ (ËÐÆÇÍÔ D) d

y; m



 P

y; m

ÿ P

y; m

. ª, ÐÂÍÑÐÇÙ, ÄÞÚËÔÎâáÕÔâ ÊÐÂÚÇÐËâ

ËÐÆÇÍÔÂ ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ: C

y; m

 d

y; m

=d (d ì ÔÕÂÐ-

ÆÂÓÕÐÑÇ ÑÕÍÎÑÐÇÐËÇ ÄÔÇØ ÓÂÊÐÑÔÕÇÌ d

y; m

ÊÂ ÕÑÕ ÉÇ

ÃÂÊÑÄÞÌ ÒÇÓËÑÆ ÄÓÇÏÇÐË Ô 1951-ÅÑ ÒÑ 1980 ÅÅ.). °ÕÓËÙÂ-

ÕÇÎßÐÞÇ ÊÐÂÚÇÐËâ àÕÑÅÑ ËÐÆÇÍÔÂ ÕÇÔÐÑ ÔÄâÊÂÐÞ Ô ÔÑÃÞ-

ÕËâ ÏË ¿Îß-¯ËÐßÑ, ÒÑÎÑÉËÕÇÎßÐÞÇ, ÔÑÑ ÕÄÇÕÔÕÄÇÐÐÑ, Ô

Â-¯ËÐßÂ.

¯Â ÓËÔÖÐÍÇ 14 ÒÓÇÆÔÕÂÄÎÇÐÞ ËÊÏÇÐÇÐËÇ ÔÓÇÆÐÇÏÇÔâÚ-

ÐÞØ ÊÐÂÚÇÐËÌ ËÐÆÇÍÔÂ Ct ÊÂ ÒÑÔÎÇÆÐËÇ 108 ÎÇÕ (ÓËÔ. 14Â)

Ë ÍÂÓÕËÐÂ ÍÑà××ËÙËÇÐÕÑÄ ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËâ

(ÓËÔ. 14Ã) Ä ÕÂÍÑÏ ÆËÂÒÂÊÑÐÇ ÏÂÔÛÕÂÃÑÄ, ÚÕÑÃÞ ÑØÄÂ-

ÕËÕß ÒÑ ÄÑÊÏÑÉÐÑÔÕË ÄÔÇ ÍÓÖÒÐÑÏÂÔÛÕÂÃÐÞÇ ÆÇÕÂÎË

ÒÓÑÙÇÔÔÂ, ÑÒËÔÞÄÂÇÏÞÇ àÕËÏ ÍÑÐÇÚÐÞÏ ÓâÆÑÏ (ÏÂÔÛÕÂÃ

ÎËÐÇÌÐÑ ÓÂÔÕÇÕ ÄÐËÊ ÆÑ 97 ÎÇÕ). ¯Â ÓËÔÖÐÍÇ 14Ä ÒÓÇÆÔÕÂ-

ÄÎÇÐÑ ÓÂÔÒÓÇÆÇÎÇÐËÇ ÒÎÑÕÐÑÔÕË àÐÇÓÅËË ÔÓÇÆÐÇÏÇÔâÚÐÞØ

ÊÐÂÚÇÐËÌ ËÐÆÇÍÔÂ Ct Ä ÃÑÎÇÇ ÏÇÎÍÑÏÂÔÛÕÂÃÐÑÌ ÑÃÎÂ-

ÔÕË, ÏÂÔÛÕÂÃ ÏÇÐâÇÕÔâ ÆÑ 10,5 ÎÇÕ.

°ÃÓÂÜÂáÕ ÐÂ ÔÇÃâ ÄÐËÏÂÐËÇ ÆÄÂ ÍÓÖÒÐÑÏÂÔÛÕÂÃÐÞØ

ÏËÐËÏÖÏÂ ÒÑÚÕË ÐÂ ÅÓÂÐËÙÂØ ÓâÆÂ Ë ÄÇÕÄâÜÇÇÔâ "ÆÇÓÇÄÑ"

1910

1940

1970

¤ÑÆÞ

ÎÇÕ

10,5

ÎÇÕ

²ËÔ. 14. ³ÓÇÆÐÇÏÇÔâÚÐÞÇ ÊÐÂÚÇÐËâ ËÐÆÇÍÔÂ ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ (Â),

ÍÂÓÕËÐÂ ÍÑà××ËÙËÇÐÕÑÄ (Ã), ÍÂÓÕËÐÂ ÓÂÔÒÓÇÆÇÎÇÐËâ ÒÎÑÕÐÑÔÕË àÐÇÓ-

ÅËË (Ä), Â ÕÂÍÉÇ ÍÂÓÕËÐÂ ÍÑà××ËÙËÇÐÕÑÄ (Å) ÆÎâ ×ÓÂÅÏÇÐÕÂ, ÄÊâÕÑÅÑ Ä

ÓÂÏÍÖ ÐÂ ÓËÔ. Ã.

´. 166, å 11] £¦«£¦´-¡¯¡ª© 1165

ÒÑÎÑÉËÕÇÎßÐÞØ àÍÔÕÓÇÏÖÏÑÄ ÏÇÉÆÖ ÐËÏË. ³ÕÄÑÎ ÆÇÓÇÄÂ

Ë ÆÄÇ ÇÅÑ ÒÑÚÕË ÑÆÐÑÄÓÇÏÇÐÐÑ ÐÂÚËÐÂáÜËÇÔâ ÄÇÕÄË

ÄÞÆÇÎâáÕ ÆÄÂ ÒÓÂÍÕËÚÇÔÍË ÑÆËÐÂÍÑÄÞØ ÒÇÓËÑÆÂ ì

ÏÇÉÆÖ ¿Îß-¯ËÐßÑ ÐÂ ÅÓÂÐËÙÂØ ÆÇÓÇÄÂ Ë Ä ÇÅÑ ÔÇÓÇÆËÐÇ

ÒÑ 39,8 ÎÇÕ (ÚÕÑ ÔÑÅÎÂÔÖÇÕÔâ Ô ÏÂÔÛÕÂÃÑÏ ÑÍÑÎÑ 40 ÎÇÕ

ÆÎâ 500-ÎÇÕÐËØ ÆÂÐÐÞØ Ë ÔÄËÆÇÕÇÎßÔÕÄÖÇÕ Ä ÒÑÎßÊÖ

ÔÖÜÇÔÕÄÑÄÂÐËâ 75 ë 80-ÎÇÕÐÇÅÑ ÙËÍÎÂ).

²ÂÔÒÓÇÆÇÎÇÐËÇ ÒÎÑÕÐÑÔÕË àÐÇÓÅËË (ÓËÔ. 14Ä) ÒÑÍÂÊÞ-

ÄÂÇÕ, ÚÕÑ ÏÇÎÍÑÏÂÔÛÕÂÃÐÂâ ÚÂÔÕß ÒÓÑÙÇÔÔÂ ÆÇÏÑÐÔÕÓË-

ÓÖÇÕ ÐÂÎËÚËÇ ÆËÂÒÂÊÑÐÑÄ ÎÑÍÂÎßÐÞØ ÒÇÓËÑÆËÚÐÑÔÕÇÌ Ë,

ÍÓÑÏÇ ÕÑÅÑ, ÍÂÚÇÔÕÄÇÐÐÑÇ ÑÕÎËÚËÇ ÔÕÓÖÍÕÖÓÞ ÒÓÑÙÇÔÔÂ Ä

ÑÕÓÇÊÍË ÄÓÇÏÇÐË, ÒÓËÐÂÆÎÇÉÂÜËÇ Ë ÐÇ ÒÓËÐÂÆÎÇÉÂÜËÇ

ÆÇÓÇÄÖ. £ÐÖÕÓÇÐÐââ ÚÂÔÕß ÆÇÓÇÄÂ ÔÑÆÇÓÉËÕ, Ä ÑÔÐÑÄÐÑÏ,

ÆÇÕÂÎË Ô ØÂÓÂÍÕÇÓÐÞÏË ÏÂÔÛÕÂÃÂÏË ÑÍÑÎÑ 30 Ë 12

ÏÇÔâÙÇÄ, Â ÄÐÇÛÐËÇ ÚÂÔÕË ÆÇÓÇÄÂ ÔÑÔÕÂÄÎÇÐÞ, Ä ÑÔÐÑÄ-

ÐÑÏ, ËÊ ÆÇÕÂÎÇÌ Ô ÏÂÔÛÕÂÃÑÏ ÑÍÑÎÑ 18 ÏÇÔâÙÇÄ.

£ÑÊÏÑÉÐÑ, ÐÂÎËÚËÇÏ àÒÑØ Ô ÓÂÊÐÑÌ ÄÓÇÏÇÐÐo

Ì ÔÕÓÖÍ-

ÕÖÓÑÌ ÏÑÉÐÑ ÑÃÝâÔÐËÕß ÑÕÏÇÚÂÇÏÑÇ ÏÐÑÅËÏË ÂÄÕÑÓÂÏË

ËÊÏÇÐÇÐËÇ ØÂÓÂÍÕÇÓÂ ÔÙÇÐÂÓËÇÄ ¿Îß-¯ËÐßÑ ÒÑÔÎÇ ÔÇÓÇ-

ÆËÐÞ 70-Ø ÅÑÆÑÄ. £ àÕÑÌ ÔÄâÊË ÔÎÇÆÖÇÕ ÔÍÂÊÂÕß, ÚÕÑ ÒÓË

ËÊÖÚÇÐËË ÒÓÑÙÇÔÔÂ À¬¿¯ (Ë, ÄÑÊÏÑÉÐÑ, ÐÇ ÕÑÎßÍÑ

À¬¿¯) ÐÇ ÔÎÇÆÖÇÕ ÆÇÎÂÕß ÔÎËÛÍÑÏ ÍÂÕÇÅÑÓËÚÇÔÍËØ

ÄÞÄÑÆÑÄ, ÑÒËÓÂâÔß Ä ÂÐÂÎËÊÇ ÐÂ ÐÂÃÎáÆÇÐËâ Ä ÕÇÚÇÐËÇ

ÒÑÔÎÇÆÐËØ ÐÇÔÍÑÎßÍËØ ÆÇÔâÕËÎÇÕËÌ. ¯ÇÑÃØÑÆËÏ ÂÐÂÎËÊ

ÅÑÓÂÊÆÑ ÃÑÎÇÇ ÒÓÇÆÔÕÂÄËÕÇÎßÐÞØ ÆÂÐÐÞØ (Ä ÐÂÛÇÏ ÔÎÖ-

ÚÂÇ, ÐÂÒÓËÏÇÓ, ÓâÆÞ ÐÂÃÎáÆÇÐËÌ ÊÂ ÒÑÔÎÇÆÐËÇ 30 ÎÇÕ

ÑÍÂÊÂÎËÔß ÃÞ ÐÇÆÑÔÕÂÕÑÚÐÞÏË).

°ÆÐÂÍÑ ÄÇÓÐÇÏÔâ Í ÔÕÓÖÍÕÖÓÇ ÍÂÓÕËÐÞ ÍÑà××ËÙËÇÐ-

ÕÑÄ. ¥ÄÂ ÍÓÖÒÐÑÏÂÔÛÕÂÃÐÞØ ÏËÐËÏÖÏÂ ÐÂ ÅÓÂÐËÙÂØ

ÆÇÓÇÄÂ Ë ÑÆËÐ ÏÇÉÆÖ ÇÅÑ ÄÇÕÄâÏË ÔÑÑÕÄÇÕÔÕÄÖáÕ ËÐÕÇÐ-

ÔËÄÐÞÏ Ë ÒÓÑÆÑÎÉËÕÇÎßÐÞÏ âÄÎÇÐËâÏ ¿Îß-¯ËÐßÑ 1899 ë

1902, 1940 ë 1941 Ë 1982 ë 1983 ÅÑÆÑÄ. ±ÓË ÔÓÂÄÐÇÐËË Ô 500-

ÎÇÕÐËÏË ÆÂÐÐÞÏË ÏÑÉÐÑ ÖÃÇÆËÕßÔâ, ÚÕÑ àÕË ÔËÎßÐÇÌÛËÇ

ÊÂ ÒÑÔÎÇÆÐÇÇ ÔÕÑÎÇÕËÇ ¿Îß-¯ËÐßÑ âÄÎâáÕÔâ ÄÒÑÎÐÇ

ÑÓÆËÐÂÓÐÞÏË ÔÑÃÞÕËâÏË. ¯ÇÔÏÑÕÓâ ÐÂ ÕÑ ÚÕÑ 500-

ÎÇÕÐËÇ ÆÂÐÐÞÇ ÐÇ ÔÑÆÇÓÉÂÕ ËÐ×ÑÓÏÂÙËË ÑÃ ËÐÕÇÐÔËÄÐÑ-

ÔÕË ÒÓÑÙÇÔÔÂ Ë ÖÔÓÇÆÐÇÐÞ ÒÑ 12 ÏÇÔâÙÂÏ, ÆÇÕÂÎË ÍÂÓÕËÐ

ØÑÓÑÛÑ ÔÑÅÎÂÔÖáÕÔâ. ®ÑÉÐÑ ÔÚËÕÂÕß, ÚÕÑ ÓÇÍÑÐÔÕÓÖËÓÑ-

ÄÂÐÐÂâ ÊÂ ÒÑÔÎÇÆÐËÇ 500 ÎÇÕ ØÓÑÐÑÎÑÅËâ ÔÑÃÞÕËÌ ¿Îß-

¯ËÐßÑ ÐÇÒÎÑØÑ ÑÒËÔÞÄÂÇÕ ÆËÐÂÏËÍÖ ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ

ÐÂ ÃÑÎßÛËØ ÄÓÇÏÇÐÂØ (ÒÑÓâÆÍÂ ÐÇÔÍÑÎßÍËØ ÆÇÔâÕËÎÇÕËÌ).

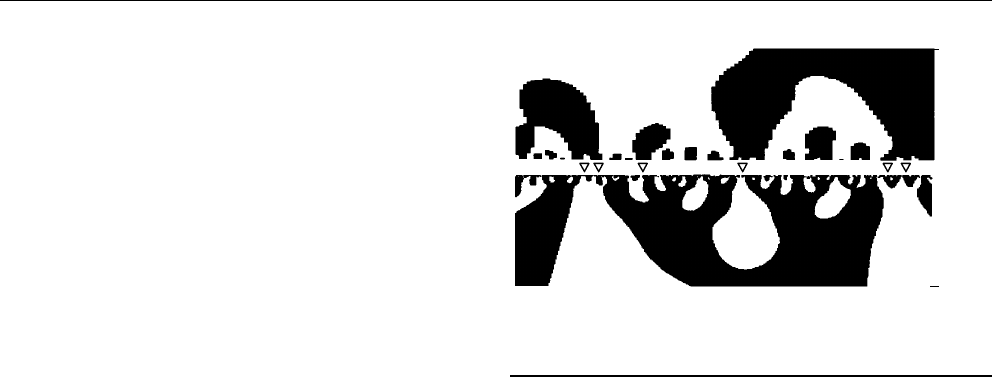
¢ÑÎÇÇ ÒÑÆÓÑÃÐÑ ÄÇÕÄË ÆÇÓÇÄÂ ÒÑÍÂÊÂÐÞ ÐÂ ÓËÔ. 14Å:

ÊÆÇÔß ÏÂÔÛÕÂÃ ÓÂÔÕÇÕ ÆÑ 29 ÎÇÕ. ¬ÂÓÕËÐÞ ÍÑà××ËÙËÇÐÕÑÄ

(Ë ÎËÐËÌ ÎÑÍÂÎßÐÞØ ÏÂÍÔËÏÖÏÑÄ) ÆÇÏÑÐÔÕÓËÓÖáÕ ÐÇÒÓÑ-

ÔÕÖá ËÇÓÂÓØËÚÇÔÍÖá ÔÕÓÖÍÕÖÓÖ. ¦ÔÎË Ä ÑÔÐÑÄÂÐËË ÆÇÓÇÄÂ

ÏÞ ÑÕÏÇÕËÎË ÖÍÓÖÒÐÇÐËÇ ÏÂÔÛÕÂÃÂ Ä ÄËÆÇ ÖÆÄÑÇÐËâ

ÒÇÓËÑÆÂ, ÕÑ ÖÉÇ ÆÄÇ ÅÎÂÄÐÞÇ ÄÇÕÄË ÆÇÓÇÄÂ ÄÇÕÄâÕÔâ ÒÑ-

ÓÂÊÐÑÏÖ ì ÎÇÄÂâ ÐÂ ÕÓË, Â ÒÓÂÄÂâ ÐÂ ÆÄÇ ÄÇÕÄË. ¯Â ÃÑÎÇÇ

ÏÇÎÍËØ ÏÂÔÛÕÂÃÂØ ÒÓÑÆÑÎÉÂÇÕÔâ, ÍÂÍ ÍÂÉÇÕÔâ, ÐÇÓÇÅÖ-

ÎâÓÐÑÇ ÚÇÓÇÆÑÄÂÐËÇ ÖÆÄÑÇÐËâ Ë ÖÕÓÑÇÐËâ ÎËÐËÌ ÎÑÍÂÎß-

ÐÞØ ÏÂÍÔËÏÖÏÑÄ. £ÔÕÓÇÚÂáÕÔâ Ë ÄÇÕÄÎÇÐËâ ÕËÒÂ ÄÑÆÑ-

ÓÂÊÆÇÎÂ, ÍÑÅÆÂ Ä ÅÎÂÄÐÖá ÎËÐËá ÎÑÍÂÎßÐÞØ ÏÂÍÔËÏÖÏÑÄ

ÒÑ ÏÇÓÇ ÓÑÔÕÂ ÏÂÔÛÕÂÃÂ ÄÎËÄÂáÕÔâ ÒÑÔÎÇÆÑÄÂÕÇÎßÐÑ

ÐÇÔÍÑÎßÍÑ ÒÑÃÑÚÐÞØ.

£ÐÇÛÐÇ ÒÑØÑÉËÇ ÄÇÕÄÎÇÐËâ ÏÑÉÐÑ ÔÏÑÆÇÎËÓÑÄÂÕß

ÍÂÐÕÑÓÑÄÔÍËÏ ÏÐÑÉÇÔÕÄÑÏ, ÍÑÅÆÂ ËÊ ÇÆËÐËÚÐÑÅÑ

ÑÕÓÇÊÍÂ (Ö ÐÂÔ àÕÑ ÒÓËÏÇÓÐÑ 80 ÎÇÕ) ÖÆÂÎâÇÕÔâ ÔÓÇÆÐââ

ÕÓÇÕß, ËÊ ÑÔÕÂÄÛËØÔâ ÚÂÔÕÇÌ ÕÂÍÉÇ ÖÆÂÎâáÕÔâ ÔÓÇÆÐËÇ

ÕÓÇÕË Ë Õ.Æ. (ÏÑÆÇÎß ÖÆÄÑÇÐËâ ÎËÐËÌ ÎÑÍÂÎßÐÞØ ÏÂÍÔË-

ÏÖÏÑÄ); ËÎË ÖÆÂÎâáÕÔâ ÆÄÇ ËÊ ÒâÕË ÚÂÔÕÇÌ ÇÆËÐËÚÐÑÅÑ

ÑÕÓÇÊÍÂ Ë Õ.Æ. Ä ÍÂÉÆÑÏ ÔÎÇÆÖáÜÇÏ ÒÑÍÑÎÇÐËË ËÊ ÍÂÉÆÑÌ

ÚÂÔÕË (ÏÑÆÇÎß ÖÕÓÑÇÐËâ).

£ ÐÂÛÇÏ ÔÎÖÚÂÇ ÒÓÑÙÇÔÔ ÔÎÑÉÐÇÇ ÒÑ ÍÓÂÌÐÇÌ ÏÇÓÇ Ä

ÆÄÖØ ÑÕÐÑÛÇÐËâØ. £Ñ-ÒÇÓÄÞØ, ÄÔÕÓÇÚÂáÕÔâ ÚÇÓÇÆÖá-

ÜËÇÔâ ÐÇÓÇÅÖÎâÓÐÞÏ ÑÃÓÂÊÑÏ ÄÇÕÄÎÇÐËâ ÆÄÖØ (ËÎË ÆÂÉÇ

ÃÑÎÇÇ) ÕËÒÑÄ ì ÕÂÍ ÖÔÕÓÑÇÐÞ ÐÇÑÆÐÑÓÑÆÐÞÇ ËÎË ÏÖÎß-

ÕË×ÓÂÍÕÂÎßÐÞÇ ÏÐÑÉÇÔÕÄÂ. £Ñ-ÄÕÑÓÞØ, Ä ÑÕÎËÚËÇ ÑÕ

ÔÕÂÐÆÂÓÕÐÑÌ ÒÓÑÙÇÆÖÓÞ ÒÑÔÕÓÑÇÐËâ ÍÂÐÕÑÓÑÄÔÍÑÅÑ ÏÐÑ-

ÉÇÔÕÄÂ, ÅÆÇ ÓÂÊ ÖÆÂÎÇÐÐÂâ ÚÂÔÕß ÑÕÓÇÊÍÂ ÐÇ ÄÑÔÔÕÂÐÂÄÎË-

ÄÂÇÕÔâ ÐË Ä ÍÂÍÑÌ ÔÄÑÇÌ ÚÂÔÕË, ÊÆÇÔß ÒÓÑÆÑÎÉÂáÕÔâ

ÄÇÕÄÎÇÐËâ ÎËÐËÌ ÎÑÍÂÎßÐÞØ àÍÔÕÓÇÏÖÏÑÄ ÑÃÑËØ ÊÐÂÍÑÄ,

Õ.Ç. Ä ÖÆÂÎÇÐÐÑÏ ÑÕÓÇÊÍÇ ÐÂ ÔÎÇÆÖáÜËØ àÕÂÒÂØ ÍÂÍ ÃÞ

ÄÑÔÔÕÂÐÂÄÎËÄÂáÕÔâ ÐÇÍÑÕÑÓÞÇ ÇÅÑ ÚÂÔÕË. ¢ÖÆÇÕ ÎË ÓÇ-

ÊÖÎßÕÂÕÑÏ ÕÂÍÑÅÑ ÍÂÔÍÂÆÐÑÅÑ ÒÓÑÙÇÔÔÂ ÍÂÐÕÑÓÑ ÄÔÍÂâ

ÒÞÎß ËÎË ËÐÑÇ ×ÓÂÍÕÂÎßÐÑÇ ÏÐÑÉÇÔÕÄÑ, ÊÂÄËÔËÕ ÑÕ

ÔÑÄÑÍÖÒÐÑÔÕË ÒÓÂÄËÎ ÄÇÕÄÎÇÐËâ, ÄÞâÔÐËÕß ÍÑÕÑÓÞÇ ÒÑ

ÑÅÓÂÐËÚÇÐÐÑÏÖ ÓâÆÖ ÆÂÐÐÞØ ÊÂÕÓÖÆÐËÕÇÎßÐÑ.

´ÂÍËÏ ÑÃÓÂÊÑÏ, ÄÇÌÄÎÇÕ-ÂÐÂÎËÊ ÓâÆÂ ÔÓÇÆÐÇÏÇÔâÚÐÞØ

ÊÐÂÚÇÐËÌ ËÐÆÇÍÔÂ ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ ÄÞâÄËÎ ÂÄÕÑÏÑ-

ÆÇÎßÐÖá ÔÕÓÖÍÕÖÓÖ ÆÂÐÐÞØ Ë ÐÂÎËÚËÇ ÒÑÆÑÃÐÑÅÑ ÍÂÔÍÂÆ-

ÐÑÏÖ ÒÓÑÙÇÔÔÂ ÐÂ ÏÂÔÛÕÂÃÂØ ÑÕ ÏÇÔâÙÂ ÆÑ ÐÇÔÍÑÎßÍËØ

ÆÇÔâÕËÎÇÕËÌ (ÆÑ 70 ë 80 ÎÇÕ). ÁÄÎâÇÕÔâ ÎË ÒÑÎÖÚÇÐÐÂâ

ÆÓÇÄÑÄËÆÐÂâ ÍÂÓÕËÐÂ ÎËÐËÌ ÎÑÍÂÎßÐÞØ ÏÂÍÔËÏÖÏÑÄ

ÔÄËÆÇÕÇÎßÔÕÄÑÏ ÕÑÅÑ, ÚÕÑ ÔËÔÕÇÏÂ ÆÇÏÑÐÔÕÓËÓÖÇÕ ÍÂÔÍÂÆ

ÖÄÇÎËÚÇÐËÌ (ÖÆÄÑÇÐËÌ, ÖÕÓÑÇÐËÌ Ë Õ.Æ.) ÒÇÓËÑÆÂ, ÍÄÂÊËÒÇ-

ÓËÑÆËÚÇÔÍÑÇ ËÎË ËÐÑÇ ÒÑÄÇÆÇÐËÇ, âÄÎâÇÕÔâ ÎË ÒÓÑÆÑÎ-

ÉÂáÜÇÇÔâ ÐÂ ÏÂÎÞØ ÄÓÇÏÇÐÐÞØ ÏÂÔÛÕÂÃÂØ ÄÇÕÄÎÇÐËÇ

ÎËÐËÌ ÎÑÍÂÎßÐÞØ àÍÔÕÓÇÏÖÏÑÄ ÑÃÑËØ ÊÐÂÍÑÄ ÔÄËÆÇÕÇÎß-

ÔÕÄÑÏ ÐÂÎËÚËâ ÍÂÔÍÂÆÐÑÅÑ ÒÓÑÙÇÔÔÂ ÐÂ ÏÂÔÛÕÂÃÂØ

ÒÑÓâÆÍÂ ÏÇÔâÙÂ Ë ÏÇÐÇÇ, ÒÑÍÂÉÖÕ ÆÂÎßÐÇÌÛËÇ ËÔÔÎÇÆÑÄÂ-

ÐËâ; ÆÎâ àÕÑÅÑ ÐÇÑÃØÑÆËÏ, Ä ÚÂÔÕÐÑÔÕË, ÂÐÂÎËÊ ÓâÆÑÄ Ô

ÃÑÎÇÇ ÏÇÎÍËÏ ÓÂÊÓÇÛÇÐËÇÏ.

6.4. ³ÖÕÑÚÐÞÇ ÊÐÂÚÇÐËâ ËÐÆÇÍÔÂ ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ

¡ÐÂÎ ËÊËÓÖ ÇÏÞÌ ÓâÆ ÔÖÕÑÚÐÞØ Ê ÐÂÚÇÐËÌ ËÐÆÇÍÔÂ Ct

ÑØÄÂÕÞÄÂÇÕ ÆÇÔâÕËÎÇÕÐ ËÌ ÑÕÓÇÊ ÑÍ ÄÓÇÏ ÇÐË (Í ÔÑÉÂÎÇ-

ÐËá, ÐÇ ÒÓËÐÂÆÎÇÉÂÜËÌ ÆÇÓÇÄÖ, ÑÒËÔÂÐÐÑÏÖ Ä ÓÂÊÆÇÎÇ

6.3). ¯Â ÓËÔÖÐÍÇ 15Â, Ã ÒÓÇÆÔÕÂÄÎÇÐÞ ÓâÆ ÔÖÕÑÚÐÞØ

ÊÐÂÚÇÐËÌ ËÐÆÇÍÔÂ Ct Ë ÍÂÓÕËÐÂ ÍÑà××ËÙËÇÐÕÑÄ ÇÅÑ

ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËâ (ÏÂÔÛÕÂÃ ÓÂÔÕÇÕ ÆÑ 3 ÎÇÕ).

³ÓÂÄÐÇÐËÇ ÒÑÍÂÊÞÄÂÇÕ, ÚÕÑ ÔÓÇÆÐÇÏÇÔâÚÐÞÇ ÊÐÂÚÇÐËâ

ÑÚÇÐß ØÑÓÑÛÑ ÑÒËÔÞÄÂáÕ ÏÇÉÅÑÆÑÄÖá ËÊÏÇÐÚËÄÑÔÕß

ÅÑÆÂ

ÅÑÆ

²ËÔ. 15. ³ÖÕÑÚÐÞÇ ÊÐÂÚÇÐËâ ËÐÆÇÍÔÂ ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ ÊÂ ÑÕÏÇÚÇÐ-

ÐÞÌ ÎËÐËÇÌ ÐÂ ÓËÔ. 14Ã ÒÇÓËÑÆ, ÍÂÓÕËÐÂ ÍÑà××ËÙËÇÐÕÑÄ Ë ÓÂÔÒÓÇÆÇ-

ÎÇÐËÇ ÒÎÑÕÐÑÔÕË àÐÇÓÅËË.

1166 ¯.®. ¡³´¡¶¾¦£¡ [µ¶¯ 1996

ÒÓÑÙÇÔÔÂ Ë ÆÑÔÕÂÕÑÚÐÑ ØÑÓÑÛÑ ì ÅÑÆÑÄÑÌ ØÑÆ. £ÐÖÕÓË-

ÅÑÆÑÄÂâ ËÊÏÇÐÚËÄÑÔÕß ÅÑÓÂÊÆÑ ÎÖÚÛÇ ÑÒËÔÞÄÂÇÕÔâ ÒÑÆ-

ÓÑÃÐÞÏ ÓâÆÑÏ, ÑÆÐÂÍÑ ÐÂËÃÑÎÇÇ ØÂÓÂÍÕÇÓÐÞÇ ÆÇÕÂÎË

ÒÓÑÔÎÇÉËÄÂáÕÔâ ÐÂ ÍÂÓÕËÐÂØ ÍÑà××ËÙËÇÐÕÑÄ ÄÇÌÄÎÇÕ-

ÒÓÇÑÃÓÂÊÑÄÂÐËâ ÑÃÑËØ ÓâÆÑÄ.

¬ÂÓÕËÐÂ ÓÂÔÒÓÇÆÇÎÇÐËâ ÒÎÑÕÐÑÔÕË àÐÇÓÅËË ËÐÆÇÍÔÂ

ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ (ÓËÔ. 15Ä, ÊÆÇÔß ÏÂÔÛÕÂÃ ÏÇÐâÇÕÔâ ÆÑ

1 ÅÑÆÂ) ÒÑÍÂÊÞÄÂÇÕ âÓÍÑ ÄÞÓÂÉÇÐÐÞÌ ÅÑÆÑÄÑÌ ØÑÆ Ë

ÔÖÜÇÔÕÄÇÐÐÑ ÐÇÔÕÂÙËÑÐÂÓ ÐÖá ÔÕÓÖÍÕÖÓÖ ÒÓÑÙÇÔÔÂ ÐÂ

ÏÇÐßÛËØ ÄÓÇÏÇÐÐÞØ ÏÂÔÛÕÂÃÂØ. ±ÑÆÓÑÃÐÞÌ ÂÐÂÎËÊ

ÄÞâÄÎâÇÕ Ä àÕÑÌ ÐÇÔÕÂÙËÑ ÐÂÓÐÑÌ ÔÕÓÖÍÕÖÓÇ ÆËÂÒÂÊÑÐÞ

ÎÑÍÂÎßÐÞØ ÒÇÓËÑÆË ÚÐÑÔÕÇÌ ÒÓÑÙÇÔÔÂ Ô ÏÂÔÛÕÂÃÂÏË

ÑÍÑÎÑ ÐÇÆÇÎË (ÃÑÎßÛËÐÔÕÄÑ ÏÂÍÔËÏÖÏÑÄ ÐÂÃÎáÆÂÇÕÔâ

ÐÂ ÏÂÔÛÕÂÃÂØ  5 Ë  9 ÆÐÇÌ), ÏÇÔâÙÂ ( 25 ÆÐÇÌ), 8 ë 9

ÏÇÔâÙÇÄ Ë ÑÍÑÎÑ 2 ÎÇÕ ( 22 ÏÇÔâÙÂ).

¿ÕË Ë ÑÒËÔÂÐÐÞÇ Ä ÓÂÊÆÇÎÂØ 6.2 Ë 6.3 ÏÂÔÛÕÂÃÞ ÏÑÉÐÑ

ÑÃÐÂÓÖÉËÕß ÐÂ ÔÒÇÍÕÓÂØ ÏÑÜÐÑÔÕË, ÒÓÇÆÔÕÂÄÎÇÐÐÞØ ÐÂ

ÓËÔ. 16. ©ÆÇÔß ÒÑÍÂÊÂÐÞ ÔÒÇÍÕÓÞ ÏÑÜÐÑÔÕË, ÓÂÔÔÚËÕÂÐ-

ÐÞÇ ÒÑ ÍÑà××ËÙËÇÐÕÂÏ ÒÓÇÑÃÓÂÊÑÄÂÐËâ ¶ÖÓßÇ E

(ÓËÔ. 16Â) Ë ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËâ E

(ÓËÔ. 16Ã) ÓâÆÑÄ

ÔÓÇÆÐÇÏÇÔâÚÐÞØ (ÛÕÓËØÑÄÞÇ ÎËÐËË) Ë ÔÖÕÑÚÐÞØ (ÔÒÎÑÛ-

ÐÞÇ ÎËÐËË) ÊÐÂÚÇÐËÌ Ct. ³ÒÇÍÕÓ ÏÑÜÐÑÔÕË E

(ÔÏ.

×ÑÓÏÖÎÖ (28)) ÔÑÑÕÄÇÕÔÕÄÖÇÕ ÔÅÎÂÉÇÐÐÑÏÖ ÐÂ ÍÂÉÆÑÏ

ÏÂÔÛÕÂÃÇ ÔÒÇÍÕÓÖ ¶ÖÓßÇ. °ÃÂ ÔÒÇÍÕÓÂ ËÏÇáÕ ÆÑÄÑÎßÐÑ

ÃÑÎßÛËÇ ÑÕÓÇÊÍË ÔÑ ÔÕÇÒÇÐÐÞÏ ÒÑÄÇÆÇÐËÇÏ ÐÂ ÏÂÔÛÕÂ-

ÃÂØ ÑÕ ÐÇÔÍÑÎßÍËØ ÆÐÇÌ ÆÑ ÏÇÔâÙÂ Ë ÑÕ ÆÄÖØ-ÕÓÇØ ÏÇÔâÙÇÄ

ÆÑ ÅÑÆÂ.

´ÂÍËÏ ÑÃÓÂÊÑÏ, ÑÒËÔÞÄÂÇÏÞÌ ÄÓÇÏÇÐÐÞÏ ÓâÆÑÏ Ct

ÒÓÑÙÇÔÔ, ÍÂÍ Ë ÃÑÎßÛËÐÔÕÄÑ ÒÓÑÙÇÔÔÑÄ Ä ÒÓËÓÑÆÇ, ÒÓÑËÔ-

ØÑÆËÕ Ä ÑÚÇÐß ÛËÓÑÍÑÏ ÆËÂÒÂÊÑÐÇ ÄÓÇÏÇÐÐÞØ ÏÂÔÛÕÂ-

ÃÑÄ. ³ÒÇÍÕÓÞ ¶ÖÓßÇ ÂÐÂÎËÊËÓÖÇÏÑÅÑ ÓâÆÂ ÆÑÔÕÂÕÑÚÐÑ

ÊÂÛÖÏÎÇÐÞ, ÑÆÐÂÍÑ ÐÂ ÐËØ ÄÞÆÇÎâáÕÔâ ÒËÍË ÄÃÎËÊË

ÄÞÆÇÎÇÐÐÞØ ØÂÓÂÍÕÇÓÐÞØ ÒÇÓËÑÆÑÄ (ÒÓÂÍÕËÚÇÔÍË ÕÇØ ÉÇ,

ÚÕÑ Ë ÐÂ ÔÒÇÍÕÓÂØ ÏÑÜÐÑÔÕË, ÒÑÎÖÚÇÐÐÞØ ÒÑ ÍÑà××ËÙËÇÐ-

ÕÂÏ ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËâ). ¬ÂÓÕËÐÞ ÓÂÔÒÓÇÆÇÎÇÐËâ

ÒÎÑÕÐÑÔÕË àÐÇÓÅËË E

a; b, ÒÓÇÆÔÕÂÄÎÇÐÐÞÇ ÄÞÛÇ,

ÆÇÏÑÐÔÕÓËÓÖáÕ ÔÎÑÉÐÑÇ ÐÇÔÕÂÙËÑÐÂÓÐÑÇ ÒÑÄÇÆÇÐËÇ ÒÓÑ-

ÙÇÔÔÂ, ÐÂÎËÚËÇ ÒÇÓËÑÆËÚÇÔÍËØ Ë ÐÇÒÇÓËÑÆËÚÇÔÍËØ ÔÑÔÕÂ-

ÄÎâáÜËØ ÐÂ ÓÂÊÐÞØ ÏÂÔÛÕÂÃÂØ.

´ÂÍÑÌ ÒÓÑÙÇÔÔ ÏÑÉÇÕ ÃÞÕß ÍÑÏÒÑÊËÙËÇÌ ÔÕÑØÂÔÕËÚÇ-

ÔÍÑÌ ÍÑÏÒÑÐÇÐÕÞ Ë ÐÇÔÍÑÎßÍËØ ÓÇÅÖÎâÓÐÞØ ÍÑÏÒÑÐÇÐÕ.

£ÑÔÒÑÎßÊÖÇÏÔâ ÒÓÑÙÇÆÖÓÑÌ ´ÂÍÇÐÔÂ (ÕÇÑÓËÇÌ ÄÎÑÉÇÐËâ

ÐÇÎËÐÇÌÐÞØ ÆËÐÂÏËÚÇÔÍËØ ÔËÔÕÇÏ [27]) ÆÎâ ÍÑÐÔÕÓÖËÓÑ-

ÄÂÐËâ ×ÂÊÑÄÑÅÑ ÒÓÑÔÕÓÂÐÔÕÄÂ ÒÑ ÄÓÇÏÇÐÐo

Ì ÓÇÂÎËÊÂÙËË Ë

ÒÑÔÕÓÑÇÐËâ ÄÑÊÏÑÉÐÑÅÑ ÂÕÕÓÂÍÕÑÓÂ.

³ÎÇÆÖâ ´ÂÍÇÐÔÖ, ÔÍÑÐÔÕÓÖËÓÖÇÏ ËÊ Ct m-ÍÑÏÒÑÐÇÐÕ-

ÐÞÇ ÄÇÍÕÑÓÞ ÔÑÔÕÑâÐËâ ÔÎÇÆÖáÜËÏ ÑÃÓÂÊÑÏ:





t

; x

t

; . . . ; x

t





©ÆÇÔß x

t

  x

 k ÿ 1t



Ë t ì ÄÓÇÏÇÐÐÂâ ÊÂÆÇÓÉÍÂ.

²Â ÔÒÓÇÆÇÎÇÐËÇ Ä ÇÍÕÑÓÑÄ ÔÑÔÕÑâÐËâ ÔÑÔ ÕÂÄÎâÇÕ ÓÇÍÑÐ-

ÔÕÓÖËÓÑÄÂÐÐÑÇ ×ÂÊÑÄÑÇ ÒÓÑÔÕÓÂÐÔÕÄÑ ÓÂÊÏÇÓÐÑÔÕË m.

¥ÄÖÏÇÓÐÞÇ ÒÓÑÇÍÙËË ÒÑÎÖÚÇÐÐÞØ ÕÓÂÇÍÕÑÓËÌ ÒÑÍÂ-

ÊÂÐÞ ÐÂ ÓËÔ. 17 ÆÎâ ÓÂÊÐÞØ ÊÐÂÚÇÐËÌ ÒÂÓÂÏÇÕÓÂ ÄÓÇ-

ÏÇÐÐÑÌ ÊÂÆÇÓÉÍË t. ±ÇÓÄÞÇ ÕÓË ÒÑÓÕÓÇÕÂ ÒÑÎÖÚÇÐÞ ÐÂ

ÑÔÐÑÄÇ ÓâÆÂ ÔÖÕÑÚÐÞØ ÊÐÂÚÇÐËÌ, ÆÄÂ ÒÑÔÎÇÆÐËØ ì ËÊ ÓâÆÂ

ÔÓÇÆÐÇÏÇÔâÚÐÞØ ÊÐÂÚÇÐËÌ. £ÔÇ ÕÓÂÇÍÕÑÓËË ÑÅÓÂÐËÚÇÐÞ,

ÑÆÐÂÍÑ ÐÇ ÒÑÍÂÊÞÄÂáÕ âÄÐÑ ÒÇÓËÑÆËÚÇÔÍÑÌ ÔÕÓÖÍÕÖÓÞ.

·ÂÓÂÍÕÇÓÐÞÇ ÒÇÕÇÎßÍË ÄËÆÐÞ ÐÂ ÆÄÖØ ÒÇÓÄÞØ ÒÑÓÕÓÇÕÂØ,

ÒÓÑÒÂÆÂáÕ ÒÓË ÊÂÆÇÓÉÍÇ Ä ÑÆËÐ ÏÇÔâÙ Ë ÔÐÑÄÂ

ÒÑâÄÎâáÕÔâ ÒÓË t  3 ÏÇÔ. £ ÑÔÕÂÎßÐÑÏ ÒÑÓÕÓÇ ÕÞ Ô

ÊÂÆÇÓÉÍÑÌ ÓÂÄÐÑÌ ÑÆÐÑÏÖ Ë ÕÓÇÏ ÏÇÔâÙÂÏ ÒÓÂÍÕËÚÇÔÍË

ÒÑÄÕÑÓâáÕ ÆÓÖÅ ÆÓÖÅÂ.

±ÑÓÕÓÇÕ Ô ÊÂÆÇÓÉÍÑÌ t  12 ÏÇÔ., Õ.Ç. ÒÑÔÕÓÑÇÐÐÞÌ ÐÂ

ÒÓÇÆÇÎÇ ËÎË ÖÉ ÔÑÄÔÇÏ ÊÂ ÒÓÇÆÇÎÂÏ Ë ÄÑÊÏÑÉÐÑÔÕÇÌ

ÆÎËÐÞ ËÏÇáÜÇÌÔâ Ö ÐÂÔ ÓÇÂÎËÊÂÙËË, ÑÕÎËÚÂÇÕÔâ ÑÕ

ÒÓÇÆÞÆÖÜËØ Ë ËÐÕÇÓÇÔÇÐ ÕÇÏ, ÚÕÑ ÕÓÂÇÍÕÑÓËâ àÕÑÅÑ

ÂÕÕÓÂÍÕÑÓÂ ËÏÇÇÕ ÕÓË ÑÔË (ÏÑÉÐÑ ÒÓÇÆÔÕÂÄËÕß ÔÇÃÇ

ÆËÐÂÏËÚÇÔÍÖá ÔËÔÕÇÏÖ Ô ÕÓÇÏâ ÔÕÂÙËÑÐÂÓÐÞÏË ÓÇÛÇ-

ÐËâÏË).

°ÅÓÂÐËÚÇÐÐÑÔÕß ÕÓÂÇÍÕÑÓËÌ Ë ÐÇ ÔÎËÛÍÑÏ ÃÑÎßÛÂâ

ÓÂÊÏÇÓÐÑÔÕß ÔËÔÕÇÏÞ ÅÑÄÑÓâÕ Ñ ÄÑÊÏÑÉÐÑÔÕË ÒÑÔÕÓÑÇ-

ÐËâ ÏÑÆÇÎË ÒÓÑÙÇÔÔÂ Ë ØÑÕâ ÃÞ Ñ ÒÓËÐÙËÒËÂÎßÐÑÌ

ÄÑÊÏÑÉÐÑÔÕË ÒÓÇÆÔÍ ÂÊÂÐËâ ÇÅÑ ÒÑÄÇÆÇÐËâ ÐÂ ÐÇ ÔÎËÛ-

ÍÑÏ ÃÑÎßÛËØ ÄÓÇÏÇÐÂØ. ÁÔÐÑ, ÑÆÐÂÍÑ, ÚÕÑ ÆÎËÐÂ ËÏÇá-

ÜËØÔâ ÓÇÂÎËÊÂÙËÌ ÐÇ ÒÑÊÄÑÎâÇÕ ÆÇÎÂÕß ÍÂÕÇÅÑÓËÚÇÔÍËØ

ÄÞÄÑÆÑÄ, Â ×ÑÓÏÂ ÒÑÔÎÇÆÐÇÌ ÒÓÑÇÍÙËË ÅÑÄÑÓËÕ ÒÑ ÍÓÂÌ-

ÐÇÌ ÏÇÓÇ Ñ ÐÇÑÃØÑÆËÏÑÔÕË ÂÐÂÎËÊÂ ÃÑÎÇÇ ÒÓÇÆÔÕÂÄËÕÇÎß-

ÐÞØ ÆÂÐÐÞØ.

0,001 0,01 0,1 1 10

0,01

0,1

¹ÂÔÕÑÕÂ, (ÏÇÔ.)

ÿ1

0,001 0,01 0,1 1 10

0,01

0,1

¹ÂÔÕÑÕÂ, (ÏÇÔ.)

ÿ1

²ËÔ. 16. ³ÒÇÍÕÓÞ ÏÑÜÐÑÔÕË (Â) Ë ÔÍÂÎÑÅÓÂÏÏÞ (Ã) ÆÎâ ÔÓÇÆÐÇÏÇÔâÚ-

ÐÞØ (ÛÕÓËØÑÄÞÇ ÎËÐËË) Ë ÔÖÕÑÚÐÞØ (ÔÒÎÑÛÐÞÇ ÎËÐËË) ÊÐÂÚÇÐËÌ Ct.

x  xt

xt  t



xt  t



xt  t



xt  t



²ËÔ. 17. ±ÓÑÇÍÙËË ×ÂÊÑÄÑÅÑ ÒÓÑÔÕÓÂÐÔÕÄÂ, ÒÑÎÖÚÇÐÐÞÇ ÐÂ ÑÔÐÑÄÇ

ÓâÆÑÄ ÔÖÕÑÚÐÞØ Ë ÔÓÇÆÐÇÏÇÔâÚÐÞØ ÊÐÂÚÇÐËÌ Ct; t

 1, t

 3,

 30 ÔÖÕ.; t

 3 ÏÇÔ.

´. 166, å 11] £¦«£¦´-¡¯¡ª© 1167

6.5. ¤ÎÑÃÂÎßÐÞÇ ÕÇÏ ÒÇÓÂÕÖÓÞ Ë À¬¿¯

¯Â ÓËÔÖÐÍÇ 18 ÒÓÇÆÔÕÂÄÎÇÐÞ ÅÓÂ×ËÍ ËÊÏÇÐÇÐËâ ÂÐÑÏÂÎËÌ

ÔÓÇÆÐÇÅÑÆÑÄÞØ ÊÐÂÚÇÐËÌ ÒÓËÒÑÄÇÓØÐÑÔÕÐÑÌ ÕÇÏÒÇÓÂ-

ÕÖÓÞ ÄÑÊÆÖØÂ ÊÂ ÒÇÓËÑÆ Ô 1850-ÅÑ ÒÑ 1990 ÅÅ. Ë ÔÑÑÕÄÇÕ -

ÔÕÄÖáÜÂâ ÍÂÓÕËÐÂ ÍÑà××ËÙËÇÐÕÑÄ ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂ-

ÐËâ Ä ÆËÂÒÂÊÑÐÇ ÏÂÔÛÕÂÃÑÄ, ÑØÄÂÕÞÄÂáÜÇÏ ÒÓÂÍÕËÚÇÔÍË

ÄÔÇ ÍÓÖÒÐÑÏÂÔÛÕÂÃÐÞÇ ÆÇÕÂÎË ÒÓÑÙÇÔÔÂ, ÑÒËÔÞÄÂÇÏÞÇ

ÓâÆÑÏ (ÏÂÔÛÕÂÃ ÎËÐÇÌÐÑ ÓÂÔÕÇÕ ÆÑ 85 ÎÇÕ).

°ÃÓÂÜÂÇÕ ÐÂ ÔÇÃâ ÄÐËÏÂÐËÇ ÕÑÕ ×ÂÍÕ, ÚÕÑ ÏÂÔÛÕÂÃ

25 ë 30 ÎÇÕ ÓÂÊÆÇÎâÇÕ ÍÂÓÕËÐÖ ÍÑà××ËÙËÇÐÕÑÄ ÐÂ ÆÄÇ

ÊÂÏÇÕÐÑ ÓÂÊÎËÚÂáÜËÇÔâ ÑÃÎÂÔÕË. £ ÐËÉÐÇÌ ÚÂÔÕË ÍÂÓ-

ÕËÐÞ ÄËÆÐÞ ÄÔÇÅÑ ÎËÛß ÆÄÇ ÍÓÖÒÐÑÏÂÔÛÕÂÃÐÞÇ ÆÇÕÂÎË.

³ÑÄÇÓÛÇÐÐÑ ÑÕÎËÚÐÂâ ÔÕÓÖÍÕÖÓÂ ÐÂÃÎáÆÂÇÕÔâ Ä ÄÇÓØÐÇÌ

ÚÂÔÕË ÍÂÓÕËÐÞ, ÅÆÇ ÔÑÔÓÇÆÑÕÑÚÇÐÂ ÒÓÂÍÕËÚÇÔÍË ÄÔâ ÄÓÇ-

ÏÇÐÐÂâ ÆËÐÂÏËÍÂ ÂÐÂÎËÊËÓÖÇÏÞØ ÆÂÐÐÞØ.

¯ËÉÐââ ÚÂÔÕß ÍÂÓÕËÐÞ ÍÑà××ËÙËÇÐÕÑÄ ÑÕÓÂÉÂÇÕ

ÐÂÎËÚËÇ Ä ÂÐÂÎËÊËÓÖÇÏÞØ ÆÂÐÐÞØ ÍÓÖÒÐÑÏÂÔÛÕÂÃÐÑÌ

ÍÑÏÒÑÐÇÐÕÞ, ÒÑØÑÉÇÌ ÐÂ ÒÑÎÑÉËÕÇÎßÐÞÌ ÕÓÇÐÆ, ì

ÔÄÇÕÎÂâ ÑÃÎÂÔÕß ÔÎÇÄÂ ÔÑÑÕÄÇÕÔÕÄÖáÕ ÑÕÓËÙÂÕÇÎßÐÞÏ

ÊÐÂÚÇÐËâÏ ÍÑà××ËÙËÇÐÕÑÄ, ÍÑÕÑÓÞÇ Ô ÕÇÚÇÐËÇÏ ÄÓÇÏÇÐË

(ÒÓË ÆÄËÉÇÐËË ÐÂÒÓÂÄÑ) ÄÑÊÓÂÔÕ ÂáÕ, Ë Ä ÒÓÂÄÑÌ ÐËÉÐÇÌ

ÚÂÔÕË ÍÂÓÕËÐÞ ×ÑÓÏËÓÖÇÕÔâ ÒÑÚÕË ÓÂÄÐÑÙÇÐÐÂâ ÑÃÎÂÔÕß

ÒÑÎÑÉËÕÇÎßÐÞØ ÊÐÂÚÇÐËÌ ÍÑà××ËÙËÇÐÕÑÄ (ÕÇÏÐÂâ).

³ÕÓÖÍÕÖÓÂ ÅÑÓÂÊÆÑ ÃÑÎÇÇ ÔÎÑÉÐÂâ Ë, ÐÂ ÒÇÓÄÞÌ

ÄÊÅÎâÆ, ÃÇÊ ÍÂÍÑÅÑ-ÎËÃÑ ÊÂÏÇÕÐÑÅÑ ÒÑÓâÆÍÂ ÐÂÃÎáÆÂ-

ÇÕÔâ ÐÂ ÏÂÔÛÕÂÃÂØ ÆÑ 25 ë 30 ÎÇÕ. ¢ÑÎÇÇ ÒÑÆÓÑÃÐÑÇ

ÓÂÔÔÏÑÕÓÇÐËÇ ÒÑÊÄÑÎâÇÕ, ÑÆÐÂÍÑ, ÄÞÆÇÎËÕß ÒÑÄÕÑÓâá-

ÜËÇÔâ ØÂÓÂÍÕÇÓÐÞÇ ÆÇÕÂÎË: ÔÖÜÇÔÕÄÖÇÕ ÆËÂÒÂÊÑÐ ÄÓÇÏÇÐ-

ÐÞØ ÏÂÔÛÕÂÃÑÄ, ÐÂ ÍÑÕÑÓÞØ ÍÂÓÕËÐÂ ËÏÇÇÕ ÒÓÂÍÕËÚÇÔÍË

ÍÄÂÊËÒÇÓËÑÆËÚÇÔÍÖá ÔÕÓÖÍÕÖÓÖ. ®ÑÉÐÑ ÔÍÂÊÂÕß, ÚÕÑ ÇÔÎË

ÔÂÏÑÌ ÍÓÖÒÐÑÏÂÔÛÕÂÃÐÑÌ ÔÑÔÕÂÄÎâáÜÇÌ ÂÐÂÎËÊËÓÖÇ-

ÏÑÅÑ ÒÓÑÙÇÔÔÂ âÄÎâÇÕÔâ ÒÑÚÕË ÎËÐÇÌÐÞÌ ÒÑÎÑÉËÕÇÎß-

ÐÞÌ ÕÓÇÐÆ, ÕÑ ÐÂ "ÏÇÊÑÏÂÔÛÕÂÃÂØ" ÒÓÑÙÇÔÔ ÒÓÇÆÔÕÂ-

ÄÎâÇÕÔâ ÔÑÔÕÂÄÎÇÐÐÞÏ ËÊ ÐÇÃÑÎßÛÑÅÑ ÐÂÃÑÓÂ ÅÂÓÏÑÐËÚÇ-

ÔÍËØ ÍÑÎÇÃÂÐËÌ.

¯Â ÓËÔÖÐÍÇ 19 Ä ÕÑÏ ÉÇ ÏÂÔÛÕÂÃÇ Ë ÊÂ ÕÑÕ ÉÇ ÒÇÓËÑÆ

ÄÓÇÏÇÐË ÒÓÇÆÔÕÂÄÎÇÐÞ ÅÓÂ×ËÍË ÂÐÑÏÂÎËÌ ÔÓÇÆÐÇÅÑÆÑ-

ÄÞØ ÊÐÂÚÇÐËÌ ÒÓËÒÑÄÇÓØÐÑÔÕÐÑÌ ÕÇÏÒÇÓÂÕÖÓÞ ÄÑÊÆÖØÂ

ÑÕÆÇÎßÐÑ ÆÎâ ³ÇÄÇÓÐÑÅÑ (ÓËÔ. 19Â) Ë ÀÉÐÑÅÑ (ÓËÔ. 19Ã)

ÒÑÎÖÛÂÓËÌ Ë ÍÂÓÕËÐÞ ÍÑà××ËÙËÇÐÕÑÄ ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂ-

ÊÑÄÂÐËâ ÒÑÎÖÛÂÓÐÞØ ÆÂÐÐÞØ: ÐÂ ÓËÔ. 19Ä ÒÑÍÂÊÂÐÂ

ÍÂÓÕËÐÂ ÍÑà××ËÙËÇÐÕÑÄ ÆÎâ ³ÇÄÇÓÐÑÅÑ ÒÑÎÖÛÂÓËâ, ÐÂ

ÓËÔ. 19Å ì ÆÎâ ÀÉÐÑÅÑ ÒÑÎÖÛÂÓËâ.

¬ÂÓÕËÐÞ ÍÑà××ËÙËÇÐÕÑÄ ÒÑÎÖÛÂÓÐÞØ ÆÂÐÐÞØ ËÏÇáÕ

ÍÂÚÇÔÕÄÇÐÐÑ ÔØÑÉÖá ÔÕÓÖÍÕÖÓÖ. ¯ÂËÃÑÎÇÇ ÊÂÏÇÕÐÑÇ

ÓÂÊÎËÚËÇ ÏÇÉÆÖ ÒÑÎÖÛÂÓËâÏË ÔÑÔÕÑËÕ Ä ÊÂÒÂÊÆÞÄÂÐËË

ÕÓÇÐÆÂ Ä ÀÉÐÑÏ ÒÑÎÖÛÂÓËË: ÅÎÑÃÂÎßÐÑÇ ÒÑÕÇÒÎÇÐËÇ Ä

³ÇÄÇÓÐÑÏ ÒÑÎÖÛÂÓËË Ë ÐÂÚËÐÂÇÕÔâ ÓÂÐßÛÇ, Ë ÃÑÎÇÇ

ÊÐÂÚËÕÇÎßÐÑ, ÚÇÏ Ä ÀÉÐÑÏ. °ÔÐÑÄÐÑÌ ÒÓËÚËÐÑÌ ÕÂÍÑÅÑ

ÓÂÊÎËÚËâ âÄÎâÇÕÔâ, ÒÑ-ÄËÆËÏÑÏÖ, ÐÇÓÂÄÐÑÏÇÓÐÑÇ ÓÂÔÒÓÇ-

ÆÇÎÇÐËÇ ÔÖÛË, ÃÑÎßÛÇÇ ÍÑÎËÚ ÇÔÕÄÑ ÍÑÕÑÓÑÌ ÒÓËØÑÆËÕÔâ

ÐÂ ³ÇÄÇÓÐÑÇ ÒÑÎÖÛÂÓËÇ. ¯ÇÎßÊâ ÔÃÓÂÔÞÄÂÕß ÔÑ ÔÚÇÕÑÄ Ë

ÂÐÕÓÑÒÑÅÇÐÐÞÌ ×ÂÍÕÑÓ, ÄÎËâÐËÇ ÍÑÕÑÓÑÅÑ ÃÑÎÇÇ ÊÂÏÇÕÐÑ

ÕÂÍÉÇ Ä ³ÇÄÇÓÐÑÏ ÒÑÎÖÛÂÓËË. ¯ÇÑÃØÑÆËÏÑ ÒÓËÐËÏÂÕß

ÄÑ ÄÐËÏÂÐËÇ ÕÂÍÉÇ ÕÑÕ ×ÂÍÕ, ÚÕÑ ÏÇÕÇÑÔÕÂÐÙËÌ Ä ÀÉÐÑÏ

ÒÑÎÖÛÂÓËË ÏÇÐßÛÇ Ë, ÄÑÊÏÑÉÐÑ, ÆÂÐÐÞÇ ÏÇÐÇÇ ÒÓÇÆÔÕÂ-

ÄËÕÇÎßÐÞ. °ÕÏÇÕËÏ, ÚÕÑ ÕÓÇÐÆ, ÒÑÎÖÚÇÐÐÞÌ ÒÑÔÎÇ ÄÞÚË-

ÕÂÐËâ ËÊ ËÔØÑÆÐÞØ ÆÂÐÐÞØ ÓÇÍÑÐÔÕÓÖËÓÑÄÂÐÐÑÅÑ Ô ÒÑÏÑ-

Üßá ÑÃÓÂÕÐÑÅÑ ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËâ ÓâÆÂ, ÐÇ ËÏÇÇÕ Ä

ÐÂÚÂÎÇ ÔÕÑÎÇÕËâ ØÂÓÂÍÕÇÓÐÑÅÑ ËÊÎÑÏÂ, ÔÄâÊÞÄÂÇÏÑÅÑ

ÑÃÞÚÐÑ Ô ÕÇØÐÑÅÇÐÐÑÌ ÐÂÅÓÖÊÍÑÌ.

´ÂÍ ÉÇ, ÍÂÍ Ë ÆÎâ ÂÐÑÏÂÎËÌ ÅÎÑÃÂÎßÐÑ Ì ÕÇÏÒÇÓÂÕÖÓÞ

ÄÔâ ÄÓÇÏÇÐÐÂâ ÆËÐÂÏËÍÂ ÂÐÂÎË ÊËÓÖÇÏÞØ ÒÑÎÖÛÂÓÐÞØ

ÆÂÐÐÞØ ÔÑÔÓÇÆÑÕÑÚÇÐÂ ÐÂ ÏÂÔÛÕÂÃÂØ ÒÓËÃÎËÊËÕÇÎßÐÑ

ÆÑ 25 ë 30 ÎÇÕ.

1880 1910 1940 1970 ¤ÑÆÞ

ÎÇÕ

²ËÔ. 18. ¡ÐÑÏÂÎËË ÔÓÇÆÐÇÅÑÆÑÄÑÌ ÅÎÑÃÂÎßÐÑÌ ÕÇÏÒÇÓÂÕÖÓÞ Ë ÍÂÓÕË-

ÐÂ ÍÑà××ËÙËÇÐÕÑÄ ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËâ.

1880 1910 1940 1970 ¤ÑÆÞ

¤ÑÆÞ19101880

ÎÇÕ

²ËÔ. 19. ¡ÐÑÏÂÎËË ÔÓÇÆÐÇÅÑÆÑÄÑÌ ÕÇÏÒÇÓÂÕÖÓ Þ ÆÎâ ³ÇÄÇÓÐÑÅÑ Ë

ÀÉÐÑÅÑ ÒÑÎÖÛÂÓËÌ Ë ÔÑÑÕÄÇÕÔÕÄÖáÜËÇ ÍÂÓÕËÐÞ ÍÑà××ËÙËÇÐÕÑÄ

ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËâ.

ÎÇÕ

²ËÔ. 20. ¶ÓÂÅÏÇÐÕÞ ÍÂÓÕËÐ ÍÑà××ËÙËÇÐÕÑÄ ÆÎâ ³ÇÄÇÓÐÑÅÑ Ë ÀÉÐÑÅÑ

ÒÑÎÖÛÂÓËÌ.

1168 ¯.®. ¡³´¡¶¾¦£¡ [µ¶¯ 1996

¯Â ÓËÔÖÐÍÇ 20 ÒÑÍÂÊÂÐÞ ×ÓÂÅÏÇÐÕÞ ÒÑÎÖÛÂÓÐÞØ

ÍÂÓÕËÐ Ô ÃÑÎÇÇ ÒÑÆÓÑÃÐÞÏ ÓÂÊÓÇÛÇÐËÇÏ ÒÑ ÏÂÔÛÕÂÃÖ:

ÏÂÔÛÕÂÃ ÓÂÔÕÇÕ ÆÑ 30 ÎÇÕ ÐÂ ÓËÔ. 20Â Ë ÆÑ 8 ÎÇÕ ÐÂ ÓËÔ. 20Ã

(ÄÓÇÏÇÐÐa

â ÑÔß ÑÔÕÂÎÂÔß ÕÑÌ ÉÇ, ÚÕÑ Ë ÐÂ ÓËÔ. 18, 19).

¹ÕÑÃÞ ÖÆÑÃÐÇÇ ÃÞÎÑ ÔÓÂÄÐËÄÂÕß ÒÑÎÖÛÂÓÐÞÇ ÆÂÐÐÞÇ,

ÍÂÓÕËÐÞ ÔÑÄÏÇÜÇÐÞ ÏÂÎÞÏË ÏÂÔÛÕÂÃÂÏË ì Ä ÐËÉÐÇÌ

ÒÑÎÑÄËÐÇ ÍÂÉÆÑÅ Ñ ÓËÔÖÐÍÂ ÒÑÍÂÊÂÐÂ ÍÂÓÕËÐÂ ÍÑà××Ë-

ÙËÇÐÕÑÄ ÆÎâ ÀÉÐÑÅÑ ÒÑÎÖÛÂÓËâ, Â Ä ÄÇÓØÐÇÌ ÒÑÎÑÄËÐÇ

ÒÑÍÂÊÂÐÂ ÊÇÓÍÂÎßÐÑ ÑÕÓÂÉÇÐÐÂâ ÍÂÓÕËÐÂ ÆÎâ ³ÇÄÇÓÐÑÅÑ

ÒÑÎÖÛÂÓËâ (ÏÂÔÛÕÂÃ Ä ÄÇÓØÐÇÌ ÒÑÎÑÄËÐÇ, ÔÑÑÕÄÇÕ-

ÔÕÄÇÐÐÑ, ÓÂÔÕÇÕ ÄÄÇÓØ).

·ÑÓÑÛÑ ÊÂÏÇÕÐÂâ ÙËÍÎËÚÐÑÔÕß ÒÇÓËÑÆÑÄ ÒÑÕÇÒÎÇÐËâ

Ë ÒÑØÑÎÑÆÂÐËâ ÐÂ ÏÂÔÛÕÂÃÂØ ÑÍÑÎÑ 10 ë 11 ÎÇÕ (ÓËÔ. 20Ã)

×ÑÓÏËÓÖÇÕ ÃÑÎÇÇ ÆÎËÕÇÎßÐÞÇ àÒÑØË ÒÑÕÇÒÎÇÐËâ Ë ÒÑØÑ-

ÎÑÆÂÐËâ ÍÎËÏÂÕÂ. ±ÑÄÕÑÓâáÜËÇÔâ ÑÕÐÑÔËÕÇÎßÐÑ ÍÓÖÒ-

ÐÑÏÂÔÛÕÂÃÐÞÇ ÆÇÕÂÎË ÐÂ ÓËÔ. 20Â ÔÄËÆÇÕÇÎßÔÕÄÖáÕ Ñ

ÕÑÏ, ÚÕÑ ÃÑÎÇÇ ËÎË ÏÇÐÇÇ ÆÎËÕÇÎßÐÞÇ àÒÑØË (ÒÓÑÆÑÎÉË-

ÕÇÎßÐÑÔÕßá ÑÕ > 10 ÆÑ < 30 ÎÇÕ) Ô ÒÑÄÞÛÇÐËÇÏ Ë

ÒÑÐËÉÇÐËÇÏ ÔÓÇÆÐÇÅÑÆÑÄÑÌ ÕÇÏÒÇÓÂÕÖÓÞ ÔÏÇÐâÎË ÆÓÖÅ

ÆÓÖÅÂ Ä ÕÇÚÇÐËÇ ÄÔÇÅÑ ÂÐÂÎËÊËÓÖÇÏÑÅÑ ÒÇÓËÑÆÂ ÄÓÇÏÇÐË

ÃÇÊ âÓÍÑ ÄÞÓÂÉÇÐÐÑÌ ÓÇÅÖÎâÓÐÑÔÕË. ´ÂÍ, àÒÑØË ÒÑÕÇ-

ÒÎÇÐËâ ÏÑÉÐÑ ÐÂÃÎáÆÂÕß ÒÓËÏÇÓÐÑ Ä 60-Ç Ë 80-Ç ÅÑÆÞ

ÒÓÑÛÎÑÅÑ ÔÕÑÎÇÕËâ Ë ÐÂ ÓÖÃÇÉÇ ÔÕÑÎÇÕËÌ, Ä ÔÇÓÇÆËÐÇ

ÐÂÛÇÅÑ ÔÕÑÎÇÕËâ Ë Ä ÍÑÐÙÇ ÓâÆÂ.

©ÆÇÔß, ÍÂÍ Ë Ä ÓÇÊÖÎßÕÂÕÂØ ÂÐÂÎËÊÂ ØÂÓÂÍÕÇÓËÔÕËÍ

À¬¿¯, ÄÞÆÇÎâáÕÔâ ÎÑÍÂÎßÐÞÇ ÒÇÓËÑÆËÚÐÑÔÕË. °ÕÐÑÔË-

ÕÇÎßÐÑ ÖÔÕÑÌÚËÄÞÌ 10 ë 11-ÎÇÕÐËÌ ÙËÍÎ ÐÂÃÎáÆÂÇÕÔâ Ä

ÐÂÚÂÎÇ ÓâÆÂ. ©ÂÕÇÏ ÔÕÓÖÍÕÖÓÂ àÕÑÅÑ ÙËÍÎÂ ÐÂÚËÐÂÇÕ

ÒÓÇÕÇÓÒÇÄÂÕß ÐÇÍÑÕÑÓÞÇ ËÊÏÇÐÇÐËâ, ÐÑ ÏÑÉÐÑ ÐÂÃÎá-

ÆÂÕß ÖÔÕÂÐÑÄÎÇÐËÇ ÑÕÐÑÔËÕÇÎßÐÑ ÖÔÕÑÌÚËÄÑÅÑ 5 ë 6-ÎÇÕ-

ÐÇÅÑ ÙËÍÎÂ (ÑÔÑÃÇÐÐÑ Ä ÍÂÓÕËÐÇ ÆÎâ ³ÇÄÇÓÐÑÅÑ ÒÑÎÖÛÂ-

ÓËâ). ¿ÕÑÕ ÒÇÓËÑÆ ÄÓÇÏÇÐË ÔÑÑÕÄÇÕÔÕÄÖÇÕ ÄÇÕÄâÏ ÆÇÓÇÄÂ

ÐÂ ÍÂÓÕËÐÇ ÍÑà××ËÙËÇÐÕÑÄ ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËâ ÓâÆÂ

ÊÐÂÚÇÐËÌ ËÐÆÇÍÔÂ ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ (ÓÂÊÆÇÎ 6.3). ¢ÑÎÇÇ

ÍÓÖÒÐÑÏÂÔÛÕÂÃÐÂâ ÆËÐÂÏËÍÂ ÔÓÇÆÐÇÅÑÆÑÄÞØ ÂÐÑÏÂÎËÌ

ÕÇÏÒÇÓÂÕÖÓÞ ÐÇ ÒÑÍÂÊÞÄÂÇÕ ÍÂÍÑÌ-ÎËÃÑ ÖÔÕÑÌÚËÄÑÌ

ÒÇÓËÑÆËÚÐÑÔÕË.

¢ÑÎßÛÑÌ ËÐÕÇÓÇÔ Í ËÔÕÑÓËË À¬¿¯ ÔÄâÊÂÐ ÕÂÍÉÇ Ô

ÕÇÏ, ÚÕÑ ÚÂÔÕÑÕÂ ÒÓÑÙÇÔÔÂ ¿Îß-¯ËÐßÑ ËÐÑÅÆÂ ÂÔÔÑÙËË-

ÓÖÇÕÔâ Ô ÅÎÑÃÂÎßÐÞÏ ÒÑÕÇÒÎÇÐËÇÏ ÍÎËÏÂÕÂ [28]. ¡ÄÕÑÓ

[21] ÑÕÏÇÚÂÇÕ, ÑÆÐÂÍÑ, ÚÕÑ ÕÇÔÐÑÌ ÔÄâÊË ÏÇÉÆÖ ÚÂÔÕÑÕÑÌ

ÔÑÃÞÕËÌ ¿Îß-¯ËÐßÑ Ë ÅÎÑÃÂÎßÐÞÏ ÒÑÕÇÒÎÇÐËÇÏ ËÏ ÐÇ

ÊÂÏÇÚÇÐÑ.

¯Â ÔÂÏÑÏ ÆÇÎÇ, Ñ ÒÓâÏÑÌ ÔÄâÊË ÏÇÉÆÖ ÚÂÔÕÑÕÑÌ

ÔÑÃÞÕËÌ ¿Îß-¯ËÐßÑ Ë ÅÎÑÃÂÎßÐÞÏ ÒÑÕÇÒÎÇÐËÇÏ ÔÖÆËÕß

ÔÎÑÉÐÑ, ÒÑÔÍÑÎßÍÖ ÄÂÉÐÂ, ÒÑ-ÄËÆËÏÑÏÖ, ÐÇ ÕÑÎßÍÑ

ÚÂÔÕÑÕÂ, ÐÑ Ë ÆÎËÕÇÎßÐÑÔÕß Ë ËÐÕÇÐÔËÄÐÑÔÕß ÔÑÃÞÕËÌ.

¬ÂÍ ÖÉÇ ÖÒÑÏËÐÂÎÑÔß, ËÊÏÇÐÇÐËÇ àÕËØ ØÂÓÂÍÕÇÓËÔÕËÍ

âÄÎÇÐËâ ¿Îß-¯ËÐßÑ ØÑÓÑÛÑ ÑÒËÔÞÄÂÇÕÔâ ÊÐÂÚÇÐËâÏË

ËÐÆÇÍÔÂ ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ.

¯Â ÓËÔÖÐÍÇ 21 ÆÎâ ÔÓÂÄÐÇÐËâ Ä ÑÆËÐÂÍÑÄÑÏ ÏÂÔÛÕÂÃÇ

ÒÓËÄÇÆÇÐÞ ÍÂÓÕËÐÂ ÍÑà××ËÙËÇÐÕÑÄ ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑ-

ÄÂÐËâ ÓâÆÂ ÂÐÑÏÂÎËÌ ÔÓÇÆÐÇÅÑÆÑÄÑÌ ÕÇÏÒÇÓÂÕÖÓÞ

ÀÉÐÑÅÑ ÒÑÎÖÛÂÓËâ (ÑÐÂ ÓÂÔÒÑÎÑÉÇÐÂ Ä ÄÇÓØÐÇÌ ÚÂÔÕË

ÓËÔÖÐÍÂ, ÏÂÔÛÕÂÃ ÓÂÔÕÇÕ ÄÄÇÓØ) Ë ÍÂÓÕËÐÂ ÍÑà××ËÙËÇÐ-

ÕÑÄ ÆÎâ ËÐÆÇÍÔÂ ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ (ÑÐÂ Ä ÐËÉÐÇÌ ÚÂÔÕË,

ÏÂÔÛÕÂÃ ÓÂÔÕÇÕ ÄÐËÊ). ¬ÂÓÕËÐÞ ÚÇÓÐÑ-ÃÇÎÞÇ; ÚÇÓÐÞÇ

ÑÃÎÂÔÕË ÔÑÑÕÄÇÕÔÕÄÖáÕ ÒÑÎÑÉËÕÇÎßÐÞÏ ÊÐÂÚÇÐËâÏ

ÍÑà××ËÙËÇÐÕÑÄ (Ë ÔÄâÊÂÐÞ Ô ÕÇÒÎÞÏË àÒÑØÂÏË Ä ÍÂÓÕËÐÇ

ÆÎâ ÂÐÑÏÂÎËÌ ÕÇÏÒÇÓÂÕÖÓÞ), ÃÇÎÞÇ ì ÑÕÓËÙÂÕÇÎßÐÞÏ

(ÐÂÒÑÏÐËÏ, ÚÕÑ ÑÕÓËÙÂÕÇÎßÐÞÇ ÊÐÂÚÇÐËâ ËÐÆÇÍÔÂ Ct

ÂÔÔÑÙËËÓÖáÕÔâ Ô ÔÑÃÞÕËâÏË ¿Îß-¯ËÐßÑ).

´ÓÇÖÅÑÎßÐËÍÂÏË ÑÕÏÇÚÇÐÞ ÎËÛß ÐÂËÃÑÎÇÇ ÊÐÂÚËÕÇÎß-

ÐÞÇ ÊÂ àÕÑÕ ÒÇÓËÑÆ ÔÑÃÞÕËâ ¿Îß-¯ËÐßÑ 1899 ë 1900, 1902,

1913 ë 1914, 1940 ë 1941, 1970 Ë 1982 ë 1983 ÅÑÆÑÄ. ÇÅÍÑ

ÄËÆÇÕß, ÚÕÑ ÄÔÇ ÃÑÎÇÇ ËÎË ÏÇÐÇÇ ÒÓÑÆÑÎÉËÕÇÎßÐÞÇ ÕÇÒÎÞÇ

ÒÇÓËÑÆÞ ÔÄâÊÂÐÞ Ô ÐÂËÃÑÎÇÇ ËÐÕÇÐÔËÄÐÞÏË ÔÑÃÞÕËâÏË

¿Îß-¯ËÐßÑ (ÆÂÉÇ ÔÕÓÖÍÕÖÓÂ ÚÇÓÐÞØ ÆÇÕÂÎÇÌ Ä ÄÇÓØÐÇÌ

ÍÂÓÕËÐÇ ÒÑÄÕÑÓâÇÕ ÔÕÓÖÍÕÖÓÖ ÃÇÎÞØ Ä ÐËÉÐÇÌ). °ÆÐÂÍÑ

ÚÂÔÕÑÕÂ ÔÑÃÞÕËÌ ¿Îß-¯ËÐßÑ, ÒÑ-ÄËÆËÏÑÏÖ, ÐÇ ÑÍÂÊÞÄÂÇÕ

ÓÇÛÂáÜÇÅÑ ÄÎËâÐËâ ÐÂ ÅÎÑÃÂÎßÐÑÇ ÒÑÕÇÒÎÇÐËÇ.

£ÑÑÃÜÇ ÉÇ ¿Îß-¯Ë ÐßÑ ÏÑÅÖÕ ÑÍÂÊÞÄÂÕß ÐÇ ÕÑÎßÍÑ

ÐÇÒÑÔÓÇÆÔÕÄÇÐÐÑÇ ÄÎËâÐËÇ ÐÂ ÍÎËÏÂÕ, ÐÑ Ë ÑÒÑÔÓÇÆÑÄÂÐ-

ÐÑÇ. ¯ÇÒÑÔÓÇÆÔÕÄÇÐÐÑÇ ÄÎËâÐËÇ (ÚÇÓÇÊ ÂÕÏÑÔ×ÇÓÖ) ÒÓÑËÔ-

ØÑÆËÕ ÐÂ ÄÓÇÏÇÐÐ



yØ ÏÂÔÛÕÂÃÂØ ÒÑÓâÆÍÂ 1 ë 2 ÎÇÕ ì Ä

ÕÇÚÇÐËÇ ÆÇÌÔÕÄËâ ÔÑÃÞÕËâ ¿Îß-¯ËÐßÑ. °ÒÑÔÓÇÆÑÄÂÐÐÑÇ

ÄÎËâÐËÇ (ÚÇÓÇÊ ÑÍÇÂÐ), ÍÂÍ ÑÍÂÊÂÎÑÔß [29], ÏÑÉÇÕ ÒÓÑËÔ-

ØÑÆËÕß ÐÂ ÄÓÇÏÇÐÂØ ÒÑÓâÆÍÂ ÆÇÔâÕËÎÇÕËâ Ë ÆÂÉÇ, ÄÑÊ-

ÏÑÉÐÑ, ÃÑÎßÛËØ ÄÓÇÏÇÐÐ



yØ ÏÂÔÛÕÂÃÂØ.

³ÒÖÕÐËÍÑÄÞÇ ÐÂÃÎáÆÇÐËâ ÒÑÍÂÊÂÎË [29], ÚÕÑ ÄÑÎÐÞ

ÒÎÂÐÇÕÂÓÐÑÅÑ ÏÂÔÛÕÂÃÂ, ÑÕÓÂÉÇÐÐÞÇ ÑÕ ÂÏÇÓËÍÂÐÔÍÑÅÑ

ÒÑÃÇÓÇÉßâ ÄÑ ÄÓÇÏâ ÑÚÇÐß ËÐÕÇÐÔËÄÐÑÅÑ ¿Îß-¯ËÐßÑ

1982 ë 1983 ÅÅ., ÒÇÓÇÔÇÍÎË ´ËØËÌ ÑÍÇÂÐ Ë ÆÇÔâÕßá ÅÑÆÂÏË

ÒÑÊÉÇ ÑÍÂÊÂÎË ÄÑÊÆÇÌÔÕÄËÇ ÐÂ ¬ÖÓÑÔËÑ, Ä ÓÇÊÖÎßÕÂÕÇ ÚÇÅÑ

ÃÑÎßÛÑÇ ÍÑÎËÚÇÔÕÄÑ ÕÇÒÎÑÌ ÄÑÆÞ ÓÂÔÕÇÍÎÑÔß ÑÕ áÉÐÑÅÑ

ÒÑÃÇÓÇÉßâ ÁÒÑÐËË Ä ÔÓÇÆÐËÇ ÛËÓÑÕÞ ´ËØÑÅÑ ÑÍÇÂÐÂ Ë

ÒÓËÄÇÎÑ Í ÊÂÏÇÕÐÑÏÖ ÓÑÔÕÖ ÒÑÄÇÓØÐÑÔÕÐÑÌ ÕÇÏÒÇÓÂÕÖÓÞ

ÐÂ ÄÞÔÑÍËØ ÛËÓÑÕÂØ Ä ÔÇÄÇÓÑ-ÊÂÒÂÆÐÑÌ ÚÂÔÕË ´ËØÑÅÑ

ÑÍÇÂÐÂ. ´ÂÍËÏ ÑÃÓÂÊÑÏ, ÒÇÓÇÓÂÃÑÕÂÐÐÞÇ ÑÍÇÂÐÑÏ

ÒÑÔÎÇÆÔÕÄËâ ÔÑÃÞÕËÌ ¿Îß-¯ËÐßÑ ÏÑÅÖÕ ÑÍÂÊÂÕßÔâ ÑÚÇÐß

ÆÑÎÅÑÉËÄÖÜËÏË.

7. ©ÂÍÎáÚÇÐËÇ

ªÕÂÍ, ÐÂ ÒÓÑÔÕÞØ ÒÓËÏÇÓÂØ ÒÓËÏÇÐÇÐËâ ÄÇÌÄÎÇÕ-ÒÓÇÑÃ-

ÓÂÊÑÄÂÐËâ ÆÎâ ÂÐÂÎËÊÂ ÑÆÐÑÏÇÓÐÞØ ×ÖÐÍÙËÌ Ô ØÑÓÑÛÑ

ËÊÄÇÔÕÐÞÏË ÔÄÑÌÔÕÄÂÏË ÒÑÍÂÊÂÐÞ ÐÇÍÑÕÑÓÞÇ ÄÑÊÏÑÉÐÑ-

ÔÕË àÕÑÅÑ ÑÕÐÑÔËÕÇÎßÐÑ ÐÑÄÑÅÑ ÏÂÕÇÏÂÕËÚÇÔÍÑÅÑ ÂÒÒÂ-

ÓÂÕÂ, ÒÑÊÄÑÎâáÜÇÅÑ ÄÞâÄËÕß Ë ÐÂÅÎâÆÐÑ ÒÑÍÂÊÂÕß ÔÕÓÖÍ-

ÕÖÓÖ (ÍÄÂÊËÒÇÓËÑÆËÚÇÔÍÖá, ÂÄÕÑÏÑÆÇÎßÐÖá Ë Õ.Æ.) ÑÒË-

ÔÞÄÂÇÏÑÅÑ ÂÐÂÎËÊËÓÖÇÏÑÌ ×ÖÐÍÙËÇÌ ÒÓÑÙÇÔÔÂ Ë ÆÂáÜÇÅÑ

ËÐ×ÑÓÏÂÙËá Ñ ØÂÓÂÍÕÇÓÐÞØ ÏÂÔÛÕÂÃÂØ ÒÓÑÙÇÔÔÂ.

´Ñ, ÚÕÑ ÏÑÆÇÎßÐÞÇ ÒÓËÏÇÓÞ ÃÞÎË ÑÆÐÑÏÇÓÐÞÏË

ÓâÆÂÏË, ÐÇ ÐÂÓÖÛÂÇÕ ÑÃÜÐÑÔÕË ÒÓËÏÇÐÇÐËâ ÄÇÌÄÎÇÕ-

ÒÓÇÑÃÓÂÊÑÄÂÐËâ: ÑÒÓÇÆÇÎÇÐËâ Ë ÔÄÑÌÔÕÄ Â ÒÓÇÑÃÓÂÊÑÄÂ-

ÐËâ ÎÇÅÍÑ ÒÇÓÇÐÑÔâÕÔâ ÐÂ ÏÐÑÅÑÏÇÓÐÞÇ ÔÎÖÚÂË, Â ÐÇÊÂÄË-

ÔËÏÂâ ÒÇÓÇÏÇÐÐÂâ (Ö ÐÂÔ ì ÄÓÇÏâ) ÏÑÉÇÕ ÃÞÕß ÎáÃÑÌ

ÔÍÂÎâÓÐÑÌ ËÎË ÄÇÍÕÑÓÐÑÌ ÄÇÎËÚËÐÑÌ.

®ÂÔÛÕÂÃÐÑ-ÄÓÇÏÇÐÐa

â ÓÂÊÄÇÓÕÍÂ, ÒÑÎÖÚÂáÜÂâÔâ Ä

ÓÇÊÖÎßÕÂÕÇ ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËâ ÔËÅÐÂÎÂ, ÒÑÊÄÑÎâÇÕ

ÄÞâÄËÕß ÐÇ ÕÑÎßÍÑ ÑÔÙËÎÎâÙËË Ô ØÑÓÑÛÑ ×ËÍÔËÓÑÄÂÐÐÞÏ

50 ÎÇÕ

²ËÔ. 21. ¶ÓÂÅÏÇÐÕÞ ÍÂÓÕËÐ ÍÑà××ËÙËÇÐÕÑÄ ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËâ

ÔÓÇÆÐÇÏÇÔâÚÐÞØ Ê ÐÂÚÇÐËÌ ËÐÆÇÍÔÂ ÀÉÐÑÅÑ ¬ÑÎÇÃÂÐËâ Ë ÂÐÑÏÂÎËÌ

ÔÓÇÆÐÇÅÑÆÑÄÞØ ÊÐÂÚÇÐËÌ ÕÇÏÒÇÓÂÕÖÓÞ ÆÎâ ÀÉÐÑÅÑ ÒÑÎÖÛÂÓËâ.

ÒÇÓËÑÆÑÏ, ÐÑ Ë ÐÇÔÕÂÙËÑÐÂÓÐÞÇ ÑÔÙËÎÎâÙË Ë, ÎÑÍÂÎËÊÑ-

ÄÂÐÐÞÇ ÒÇÓËÑÆËÚÐÑÔÕË Ë Õ.Ò.

¿ÐÇÓÅËâ (ËÎË ÆËÔÒÇÓÔËâ) ÍÑà××ËÙËÇÐÕÑÄ ÄÇÌÄÎÇÕ-

ÒÓÇÑÃÓÂÊÑÄÂÐËâ E

a ÒÓÑÒÑÓÙËÑÐÂÎßÐÂ ÆËÔÒÇÓÔËË ÂÐÂ-

ÎËÊËÓÖÇÏÞØ ÆÂÐÐÞØ Ë ÆÂÇÕ ÓÂÔÒÓÇÆÇÎÇÐËÇ àÐÇÓÅËË ÒÓÑ-

ÙÇÔÔÂ ÒÑ ÏÂÔÛÕÂÃÂÏ. £ÑÊÏÑÉÐÑÔÕß ÒÑÎÖÚÇÐËâ àÕÑÌ

ØÂÓÂÍÕÇÓËÔÕËÍË ÎÑÍÂÎßÐÑ ÒÑÊÄÑÎâÇÕ, ÐÂÒÓËÏÇÓ, ÒÓË

ÂÐÂÎËÊÇ ÕÖÓÃÖÎÇÐÕÐÞØ ÒÓÑÙÇÔÔÑÄ ÐÇ ÕÑÎßÍÑ ÒÑÎÖÚËÕß

ÐÂÃÑÓ ØÂÓÂÍÕÇÓÐÞØ ÏÂÔÛÕÂÃÑÄ, ÐÑ Ë ÑÃÝÇÍÕËÄÐÑ ÑÒÓÇÆÇ-

ÎËÕß ÏÂÔÛÕÂÃÞ, ÔÄâÊÂÐÐÞÇ Ô ÍÑÅÇÓÇÐÕÐÞÏË ÔÕÓÖÍÕÖÓÂÏË,

Ë ËÔÔÎÇÆÑÄÂÕß ÒÇÓÇÏÇÉÂÇÏÑÔÕß ÒÓÑÙÇÔÔÂ.

±Ñ ÍÑà××ËÙËÇÐÕÂÏ ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËâ, Â ÕÂÍÉÇ

ÒÑ ÊÐÂÚÇÐËâÏ ÎÑÍÂÎßÐÞØ àÍÔÕÓÇÏÖÏÑÄ ÏÑÉÐÑ ÄÞÚËÔÎËÕß

ÓÂÊÏÇÓÐÑÔÕß ÂÐÂÎËÊËÓÖÇÏÑÅÑ ÏÐÑÉÇÔÕÄÂ ËÎË ÔÒÇÍÕÓ

ÓÂÊÏÇÓÐÑÔÕÇÌ, ÇÔÎË ÑÐÑ ÏÖÎßÕË×ÓÂÍÕÂÎßÐÑ.

¶ËÎßÕÓÂÙËÑÐÐÞÇ Ë ÓÇÍÑÐÔÕÓÖÍÙËÑÐÐÞÇ ÔÄÑÌÔÕÄÂ ÒÓÇ-

ÑÃÓÂÊÑÄÂÐËâ ÒÑÊÄÑÎâáÕ ÑÒÇÓËÓÑÄÂÕß ËÐ×ÑÓÏÂÙËÇÌ

(ÔÅÎÂÉËÄÂÐËÇ, ÓÂÊÎÑÉÇÐËÇ ÐÂ ÍÑÏÒÑÐÇÐÕÞ, ÔÄÇÓÕÍÂ Ë

Õ.Ò.) ÃÇÊ ÒÑÕÇÓË ÊÐÂÚËÏÞØ ÆÇÕÂÎÇÌ. ²ÂÊÓÞÄÞ ÐÇÒÓÇÓÞÄÐÑ-

ÔÕË, ÔÍÂÚÍË Ë ÆÓÖÅËÇ ÑÔÑÃÇÐÐÑÔÕË, ÄÑÊÐËÍÂáÜËÇ ËÊ-ÊÂ

ÄÂÓËÂÙËÌ ËÊÏÇÓâÇÏÑÌ ØÂÓÂÍÕÇÓËÔÕËÍË Ë ÔÃÑÇÄ ËÎË ÛÖÏÂ

ËÐÔÕÓÖÏÇÐÕÑÄ ËÊÏÇÓÇÐËâ, ÎÇÅÍÑ ÆÇÕÇÍÕËÓÖáÕÔâ, ÎÑÍÂÎ Ë-

ÊÖáÕÔâ Ë ÒÓË ÐÇÑÃØÑÆËÏÑÔÕË ÏÑÅÖÕ ÃÞÕß ÖÔÕÓÂÐÇÐÞ ËÎË

ÔÍÑÓÓÇÍÕËÓÑÄÂÐÞ.

£ÑÊÏÑÉÐÑÔÕË ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËâ ÒÑÍÂÊÂÐÞ

ÕÂÍÉÇ ÐÂ ÒÓËÏÇÓÂØ ÂÐÂÎËÊÂ ÐÇÍÑÕÑÓÞØ ÐÂÕÖÓÐÞØ ÄÓÇ-

ÏÇÐÐ



yØ ÏÇÕÇÑÓÑÎÑÅËÚÇÔÍËØ ÓâÆÑÄ.

£ÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËÇ ÒÓÇÆÔÕÂÄÎâÇÕÔâ ÑÚÇÐß ÒÇÓ-

ÔÒÇÍÕËÄÐÞÏ ÏÂÕÇÏÂÕËÚÇÔÍËÏ ÂÒÒÂÓÂÕÑÏ ÐÇ ÕÑÎßÍÑ ÆÎâ

ÊÂÆÂÚ, ÔÄâÊÂÐÐÞØ Ô ÂÐÂÎËÊÑÏ ÔËÅÐÂÎÑÄ ÓÂÊÎËÚÐÑÌ ÒÓË-

ÓÑÆÞ, ÐÑ Ë ÆÎâ ÓÇÛÇÐËâ ÖÓÂÄÐÇÐËÌ, ÑÒËÔÞÄÂáÜËØ

ÔÎÑÉÐÞÇ ÐÇÎËÐÇÌÐÞÇ ÒÓÑÙÇÔÔÞ Ô ÄÊÂËÏÑÆÇÌÔÕÄËâÏË Ä

ÛËÓÑÍËØ ÆËÂÒÂÊÑÐÂØ ÏÂÔÛÕÂÃÑÄ.

³ÎÇÆÖÇÕ ÔÍÂÊÂÕß, ÚÕÑ ÄÇÌÄÎÇÕ-ÒÓÇÑÃÓÂÊÑÄÂÐËÇ ÐË Ä

ÍÑÇÏ ÔÎÖÚÂÇ ÐÇ âÄÎâÇÕÔâ ÊÂÏÇÐÑÌ ÅÂÓÏÑÐËÚÇÔÍÑÅÑ ÂÐÂ-

ÎËÊÂ Ë ÐÇ ÖÏÂÎâÇÕ ÇÅÑ ÆÑÔÕÑËÐÔÕÄ. °ÐÑ ÒÓÑÔÕÑ ËÐÑÇ Ë

ÒÑÊÄÑÎâÇÕ ÒÑÔÏÑÕÓÇÕß ÐÂ ÂÐÂÎËÊËÓÖÇÏÞÌ ÒÓÑÙÇÔÔ Ô

ÐÇÔÍÑÎßÍÑ ËÐÑÌ ÕÑÚÍË ÊÓÇÐËâ ì Ô ÕÑÚÍË ÊÓÇÐËâ ÆÓÖÅÑÅÑ

ÂÐÂÎËÊÂÕÑÓÂ (ÔÇÏÇÌÔÕÄÂ ÂÐÂÎËÊÂÕÑÓÑÄ).

£ ÊÂÍÎáÚÇÐËÇ ÂÄÕÑÓ ÃÎÂÅÑÆÂÓËÕ ²ÑÔÔËÌÔÍËÌ ×ÑÐÆ

×ÖÐÆÂÏÇÐÕÂÎßÐÞØ ËÔÔÎÇÆÑÄÂÐËÌ ÊÂ ÚÂÔÕËÚÐÖá ÒÑÆ-

ÆÇÓÉÍÖ (ÅÓÂÐÕ å 93-01-17342) Ë À.¡. ¬ÓÂÄÙÑÄÂ ÊÂ

ÄÐËÏÂÐËÇ Í ÓÂÃÑÕÇ Ë ÙÇÐÐÞÇ ÊÂÏÇÚÂÐËâ.

³ÒËÔÑÍ ÎËÕÇÓÂÕÖÓÞ

1. Grossmann A, Morlet J SIAM J. Math. Anal. 15 723 (1984)

2. Wavelets (Eds J M Combes, A Grossmann, P Tchamitchian)

(Berlin: Springer-Verlag, 1989)

3. Wavelets and Their Applications (Ed. R Coifman) (Boston: Jones

and Barlett Publ., 1992)

4. Wavelet Analysis and Its Applications (Vol. 1: An Introduction to

Wavelets Vol. 2: Wavelets: A Tutorial in Theory and Applications)

(San Diego: Academ. Press Inc., 1992)

5. Daubechies I Comm. Pure Appl. Math. 41 906 (1988); IEEE Trans.

Inform. Theory 36 961 (1990); Ten Lectures on Wavelets (CBMS

Lecture Notes Series) (Philadelphia: SIAM, 1991)

6. Farge M Ann. Rev. Fluid Mech. 24 395 (1992)

7. Poulighy B et al. J. Appl. Cryst. 24 526 (1991); Muzy J F, Bacry E,

Arneodo A Phys. Rev. Lett. 67 3515 (1991)

8. Paul T J. Math. Phys. 25 (11) 3252 (1984)

9. Mallat S G Trans. Am. Math. Soc. 315 69 (1989); IEEE Trans. Patt.

Anal. Mach. Int. 31 674 (1989)

10. Lemarie P G, Meyer Y Rev. Math. Ibero-Americana 2 1 (1986)

11. Battle G Commun. Math. Phys. 110 601 (1987)

13. Beylkin G, Coifman R, Rokhlin V Comm. Pure Appl. Math. 44 141

(1991)

14. Holschneider M J. Stat. Phys. 50 963 (1988)

15. Arneodo A, Grasseau G, Holschneider M Phys. Rev. Lett. 61 2281

(1988)

16. Collineau S, Brunet Y Boundary-Layer Meteorology 65 357 (1993)

17. ¡ÔÕÂ×ßÇÄÂ ¯ ®, ±ÓÇÒÓËÐÕ å 1891 (®ÑÔÍÄÂ: ª¬ª ²¡¯, 1994);

±ÓÇÒÓËÐÕ å 1946 (®ÑÔÍÄÂ: ª¬ª ²¡¯, 1996)

18. Wyrtki K J. Phys. Oceanogr. 5 572 (1975)

19. MacKenzie D New Scientist (16 April) 16 (1987)

20. ³ËÆÑÓÇÐÍÑÄ ¯ ³ ´Ó. ¤ËÆÓÑÏÇÕÇÑÙÇÐÕÓÂ ³³³² (316) 31 (1991)

21. Wang Shaowu Acta Meteorologica Sinica 6 (1) 47 (1992)

22. ¡ÔÕÂ×ßÇÄÂ ¯ ®, ³ÑÐÇÚÍËÐ ¥ ® ¥¡¯ 344 (4) 539 (1995)

23. JPCC, 1990: Climate Change. The JPCC Scientific Assessment (Eds

J T Houghton, F J Jenkins, J J Ephraums ) (Cambridge: Cambridge

University Press, 1990)

24. Currie R G J. Geophys. Res. 89 7213 (1984)

25. Quinn W H, Neal V T, Antunez de Maylo S E J. Geophys. Res. 92

14449 (1987)

26. Haudler R, Andsager K Inter. J. Climato. 10 413 (1990)

27. Takens F Lecture Notes in Mathematics (Eds D Rand, L-S Young)

(New York: Springer-Verlag, 1981) p. 366

28. Enéeld D B, Cids L TOGA Notes (1) 1 (1990)

29. Jacobs G A et al. Nature (London) 370 360 (1994)

Wavelet analysis: basic theory and some applications

N.M. Astaf'eva

Space Research Institute, Russian Academy of Sciences

ul. Profsoyuznaya 84/32, 117810 Moscow, Russia

Tel. (7-095) 333-21 45

E-mail: ast@nat.iki.rssi.ru, ast@iki.rssi.ru

The basic theory of the wavelet transform, an effective investigation tool for inhomogeneous processes involving widely different scales

of interacting perturbations, is presented. In contrast to the Fourier transform, with the analysing function extending over the entire axis

of time, the two-parametric analysing function of the one-dimensional wavelet transform is well localised in both time and frequency.

The potential of the method is illustrated by analysing familiar model series (such as harmonic, fractal, and those with various types of

singularities) and the long-term variation of some meteorologic characteristics (Southern oscillation index and global and hemispheric

temperatures). The analysis of a number of El-Nino events and of the temporal behaviour of the Southern oscillation index reveals

periodic components, local periodicity features, and time scales on which self-similarity structures are seen. On the whole, both

stochastic and regular components seem to be present. The global and hemispheric temperatures are qualitatively similar in structure,

the main differen ce ì presumably due to the greater amount of land and stronger anthropogenic factor ì being that the warming trend

in the Northern hemisphere is slightly stronger and goes érst in time.

PACS numbers: 02.30.±f, 02.90.+p, 92.60.±e, 92.60.Ry

Bibliography ì 29 references

Received 23 May 1996, revised 18 July 1996

1170 ¯.®. ¡³´¡¶¾¦£¡ [µ¶¯ 1996