Antontsev S.N., Kazhikhov A.V., Monakhov V.N. Boundary Value Problems in Mechanics of Nonhomogeneous Fluids
Подождите немного. Документ загружается.


70
Chapter
2
p
=
p0(x),
u
=
u0(x),
e
=
eo(x>
for
t
=
0.
(7.7)
It
is
still
assumed that
bounded functions.
Conditions (7.5) mean that in Ehler variables boundaries of flow
domain (solid thermoisolated walls) move by the given law and the
given set up in gas dynamics
is
called "problem on piston" [117].
The basic difference in comparison with the case considered above
po
=
p1
f
0
is
connected with obtaining the
first
energy estimate
of the form (2.91, the rest of the reasoning remains the same. The
problem
is
reduced to uniform boundary values by the extension bo-
undary functions
p0
and
p,
to the interior of the rectangle
.L
=
=
(0,
1)
x
(0,
'l').
The evaluation
of
the form (2.9) in problem
with conditions
(7.5)
appears to be obtained
if
an extension
is
a
special one. Describe this construcJion. Assume boundary functions
po(t),
p,(t)
to satisfy the condition
po(x)
and
S0(x)
are highly positive and
and initial density
p
(x)
(7.8)
This requirement means, that the distance between moving domain
boundaries of flow in hler variables
is
highly more than zero and,
consequently,
is
assumed
to
be hysically reasonable. (Note that
condition (7.8) in example (7.47
is
not satisfied for
a
<
0
1.
The first equation
of
the system (1.10) written in the
form
a
1
au
at
p
ax
-
(
-
)=
-
being integrated over
x
then over
t
gives
I
dx
f--
-
l(t)
2
6
>
0.
0
P(X,
t)
(7.9)
This correlation
is
similar to property (2.1) in the problem
adhesion condition. Besides,
it
follows from (7.9) that on some
curve
a
=
a(t)
density values are equal to
l-'(t)
with
and
it
is
an analogue
of
equality
(2.2).
Now introduce
an
auxiliary function
v(t>
x
a5
l(t)
0
P(5,
t>
-
u,(x,
t)
=
--
f
-
+
wo(t>,
(7.10)
where
v(t>
=
p,(t)
-
po(t).
(7.11)
and
It
is
evident that
u,(x,
t>
takes the given values
po(t)
p,(t>
for
x
=
0
and
x
=
1
respectively.

Correctness
of
Boundary Problems
79
Assume
u(x,
t)
=
ul(x,
t)
+
w(x,
t) (7.12)
Then a new sought function w(x, t) equals zero for
x
=
0,
1
and
equation system (1.10)
is
transformed:
ap
v
at
ax
+YP
=O,
-+
P
Tiiultiplying the second equation
w
swing
with the third and in-
tegrating over
x
we come to the relation
dil
v2 kv
I
-
I
(
-w2
+@I&=---
f
0dx
-
dto
2
-
1
10
(7.14)
v2
I
x
d<
v1
1
v
xdf
--JwJ-
dx
-
-
I
W'~X
-
J[(
-
)'I-
+
pJJvJdx.
l2
0 0
P(5,
t>
lo
0
1
OP(5,
t)
-
x
d<
0
P(<,
t>
Applying the property
0
<-
f
-
<l(t),the boundedness v(t)
POW
and 1-'(t>, using the Gronwall lemma deduce the
re-
quired evaluation
After that the rest of calculations from theorem 1.1 are repeated.
Finally, we obtain the following result in problem on piston.
Theorem
7.2.
If
initial data
(7.7)
satisf the conditions of
theorem 1.1, and boundary functions
po(tY
andpl(t)
following smoothness
(po(t>, p,(t)>
E
LVi(0,
TI,
po(O>
=
uo(0),
@,(O>
=
uo(l>,
and satisfy condition
(7.81,
then the problem (1.101, (7.5)
-
(7.7) has a unique solution.
3.
Inhomogeneous problems for temperature
Now
consider the
case
when nonzero boundary conditions are given
vor values characterizing the thermal regime
of
flow,
i.e.
for
tem-
perature
or
thermal
flow.
Three versions of set up physically re-
asonable are possible (see [187]):
possess the

80
Chapter
2
1
)
a thermal flow
is
given
where
it
is
assumed that
3)
The third type conditions for temperature are given
(7.20)
Here the
known
functions
pert ies
(ki,
ail,
i
=
0,
1
possess the pro-
ki(t)
I
0,
ai(t)
I
0,
(ki,
ai)
E
H:(O,
T),
i
=
0,
1.
(7.21)
In all versions listed above the basic theorem 1.1 admits the
f
o
11
owing general
i
zat ion.
Theorem 7.3.
If
requirements (".IT), (7.191, (7.21) are satisfied
boundary value problems with conditions (7.161, (7.18)
or
(7.20)
for temperature and (7.1) or (7.5) for velocity are uniquely
sol-
vable.
The case 2) when boundary tenperature values are given
is
consi-
dered to be the most interesting. Consider
it
in
detail.
Examine the version when on boundaries
x
=
0,
x
=
1
zero strain
is
given. Thus,
it
is
required to find the solution of equation
system (1.10) with the following boundary value conditions:
au
(P
-
-
ax
au
(P
-
-
ax
Similarly
i=O,I
remain the
1
to the previous,
p
=
kpQ
and
satisfy assumptions (7.19). Initial conditions (1.12)
same
:
Xi(t)
E
W,(0,
T),

Correctness
of
Boundary Problems
81
As
it
was mentioned above inhomogeneity of boundary values only
introduces one peculiarity:
it
impedes to deduce the
first
energy
estimate.
In
situations considered above, when condition
ae/ax
=
0
is
laid down on boundaries, in all cases including, obtaining
energy estimate the property
of
non-negativeness
of
temperatm.?
0(x,
t>
2
0
similar way the peculiar case, when zero values
of
temperature are
given On boundaries
x
=
0,
x
=
1
tive,
1s
investigated.
If
inhomogeneous boundary value problem
is
considered for temperature with conditions
(7.22)
and,
if
this
problem
is
reduczrl to zero boundary values by subtracting an
un-
known function
8
admitting values
xo
and
x
for
x
=
0
and
x
=
la
non-nekativeness property cannot be guaranteed for the
difference
0
-
0
This circumstance make
us
use other methods of
obtaining evaluation for a total energy.
Introduce an ausiliary function
@,(x,
t)
as a solution
of
boun-
dary value problems
provided by maximum principle has been used. In a
and initial values are nonnega-
a@
1
a0,
a
--
-h-(p
-
at
ax
ax
Owing to
maximum
principle for
6,(x,
t>
correlations are satis-
f
ied
0
<
m
5
8,(x,
t)
5
bi
<
m.
Other smoothness properties are not required from function
B,(x,t>
yet, but note, that atronger properties result further from ob-
taming estimates for density
Introduce a new sought function
cp
instead of
8
O,(X,
t>
o(x,
t)
=
@,(x, t>cp(x,
t),
(7.25)
C'lhich
is
likely to equal
1
on
boundaries
x
=
9,
1.
Equation sys-
tem
(1.10)
is
transformed:
au
f
p2-z
0,
aP
at
-
ax
-
au
a
au
a
at
ax
ax
ax
(P
-
1
-
k
-
(8,Pcp),
--
--
(7.26)

82
Chapter
2
@-=A-
(Olp
-L
)
+
Ap
2
.
-
+
p(
-
)‘-
’at
ax
ax
ax
ax
ax
Boundary conditions
au
ax
x
o
-
I
= =
kXo(t)#
Transform equations
(7.22) can be written
in
the form
(7.26). The second equation
is
multiplied by
u the third by
1
-
(4-l
then
add
=
(0,
I)
(0,
t).
Here
apply the
acp
a
-
(I
-
9-l)
=
-
[ol((p
-
ln
cp
at
at
81
a@,
ae
a
P-*-
(1
-
cp-l)
=
-
b(cp
-
ax ax ax
and integrate over
Q
=
evident equalities
-
111
-
~(cp
-
lncp
-
I),
In
cp
-
1)
-
]
-
-
(p
-)
1
a0,
a
a01
ax ax ax
x(cp
-
In
cp
-
1)
and equation (7.23)
for
determining
Ol(x,
t)
then use boundary
conditions (7.27).
As
a result a correlation appears
t
U,(u(t>, cp(t>>
+
I
V,(U(T),
P(T),
cp(z))dz=
0
(7.28)
t
I
au
o o
lax
=
Ul(Uo,
cpo)
+
k
I I
p0
-
dx
dz
,
in which
I
p
au
~e
acp
Vl(u,
p,
cp)
=
1
[
-
(
-
)2
+h
(
-
)‘JdX.
o
cp
ax
cp2
ax
The second integral in the right side (7.28)
is
bounded fpom above:
Consequently, due
to
boundedness
8,
we have from (7.28)

Correctness
of
Boundary Problems
It
20
Ul(t>
+-I
Now
momentum equation
a3
(7.29)
au
a
au
at
ax ax
PI
-
b--
--_
is first integrated over
x
from
0
to
a random point
au
a
In
p
ax
at
Then substitute
p-
and
integrate overt and potentiate:
by
-
-
from the first equation (7.26)
t
X
P
exp(kI
PB
dT)=
poexp
{
1
(uo
-
u)d5
1
0
0
Having multiplied both parts by
k
0
taking a logarithm, we come
to
equality
integrating over t then
Using the definition (7.25) of function
CP
evaluate the right side
(7.30)
by quantity
Then use elementary inequality
b
(7.32)
ln(1
+
ae
)
5
a
+
b
Va
2
0,
b
2
0,
assuming
t
a
=
C
I
q(x,
t)dT,
b
=
max
Ilu(z)ll
0
0
<T
It
(7.33)
After that apply inequality (see the proof of inequalities
(6.14)
from lemma
6.1)
1
1
0
0
I
q(x, t)k
5
C[
I
+
I
~,(q
-
lncp
-
I)&].
(7.34)
Then, (7.29) with the help
of
(7.30)
-
(234)
function
for non-negative

84
Chapter
2
it
is
easy to obtain thew relation
t
-0
Z(t>
I
C
[
1
+I
Z(z)dz
J,
from which follows boundedness
Z(t),
and the necessary energy esti-
mate accordingly. Stress that when formula (7.30) being deduced,
we bear in mind the fact that strin values
u
=
p
-
-
p
are known
at least on one boundary
x
=
0
or
x
=
1.
A
more complicated
si-
tuation occurs,
if
on boundaries
x
=
0,l
velocity values are
rather
given than strain values. Consider
this
case assuming, for
simplicity, boundary values for velocity to be equal
to
zero:
au
ax
ulx=o
=
uIx=,
=
0,
olx=o
=
X,(t>,
@I,=,
=
X,(t).
(7.35)
As
in the previous case relations (7.28) and (7.29) are valid, but
(7.30)
is
not. Instead of
it
one can obtain another auxiliary for-
mula.
To
do this the momentum equation
au
a0
all
at
ax
ax
,u=p--
kp
Q
(7.36)
-- --
is
integrated by time
t
from
0
to an arbitrary
t
E
LO,
TI
t
I
~(x,
t)d
z.
u
-
u0
=
ax
o
Now
the given equality
is
integrated over x from a fixed oint to
xo(t)
E
[O,
11
to be chosen below
to
an arbitrary
x
E
PO,
1J
X
t
t
(U
-
uo)d<=
U(X,
t)dt-
f
o(x,(t),
T)d'C.
(7.37)
0
xO
0
From the
first
equation (7.26) and (7.36) we have
t
t
I
u(X,
z)dt
=
-
In
p(x,
t)
-
k
I
p(x,
z)O(x,
z)dz
+
In
p0(x).
0
0
Therefore potentiating (7.37)
come
to
equality
t
X
t
P
exp(k
I
pBdz}
=
p'exp
{
I
(uo
-
dd.5
-
I
u(xo(t),
t)dz}.
0
0
X
0
(7.38)
Choose
xo(t)
in accordance with the following assumption.
Lemma 7.1. For any
t
E
[0,
Tj
there exists at least one point
xo
=
xo(t>
E
[o,
11
such
that

Correctness
of
Boundary Problems
85
Proof. From equation (7.36) follows the existence of such function
(7.40)
+(x,
t)
that
a+
a+
ax
at
yu=-
u=-
from definition
CJ
it
follows that
+
satisfies equation
a+ a'+
at
ax
--
-
p
7
-
kp8.
Transform
this
equation dividing
it
intop then
writi
it
in
a
divergent form. Use the
first
equation (7.26) and (7.48:
a
'+
a+
a
-(-)--
at
P
ax ax
(u+)
=
7
-
k
0
-
u'.
Integrate this equality over
\
=
(0,
I)
x
(0,
t)
taking into
account that
u
=
+x
=
0
for
x
=
0,
1
Remember that the following correlation follows from (7.26)
An adequate choice of infinite values (see
equal to unit. Then
from
(7.41) it.follo:vs that for anyt
E
LO,
TJ
there exists
a
point
~0
=
xo(t)
E
LO,
11
1)
provides
it
to
be
such that
4J(xo(t>,t>
=
M(t>
Actually,
if
one assumes that
Bx
E
LO,
1
J
having multiplied
this
correlation by
p-l(x,
to)
and integrated
over
x
from
0
to
1
with
(7.42) in view we obtain
a
contradiction
to (7.41). Further,
from
definition (7.40) of
the
function +(x,
t)
we
have
+(x, to)
>
%(to)
or
+(x,
to)
<
n(tO),
t
X
Jl(x,
t)
=
/
a(x,
z)dT+
uo(<)d5
0
0
Hence obtain equality (7.39), where the
first
addent in
the
right

06
Chapter
2
side (7.41)
is
to be integrated by parts. 17e are likely to have
from
(7.39)
(7.431
But then having multiplied by
k0
and integrated over
t
we find
from (7.38)
k
I
pods
I
ln[l
+
C
I
a(x,
r)exp
{IluCt)II+
C
I
U,(s)ds}
dt]
5
t
t
t
0
0
0
Using again inequalities (7.32) and (7.34) conclude:
Strengthening inequality (7.29) in the way similar to the previous
case we obtain the required estimate
We emphasize again that inhomogeneous boundary value problems
differ from problem (1.10)
-
(1.12)
in
the proof of estimate of
the form (7.44).
As
soon
as
this evaluation
is
obtained, auxiliary
relations (2.7). (2.111, (2.12)
will
give bounds
from
above and
from below for density and for derivative
ap/dx
in
L,(O,
T;
L2(51))
considered
as
parabolic and boundary conditions can be made homo-
geneous after the corresponding substitution.
As
a
result, in
equations there appear only lower terms, which don’t create any
additional difficulties.
Therefore equations for velocity and temperature can be
8.
STABILIZATION
OP
SOLUTIONS
UNDZR
INFINITE
TIILE
INCREASE
Stabiliza ion of viscous
as
equation solutions has been studied
in
works
156, 59,
69,
1394.
It
should be noted that the model of
barotropic liquid in the case
of
monotonous state equation
P(
P)
has
only been fully investigated. The fact of stabilization in the
Cauchy problem has been proved in [69]. Estimates of convergence
velocity have also been obtained in [56, 1391.
In considering stabilization problems, the proof of
a
priori evalu-
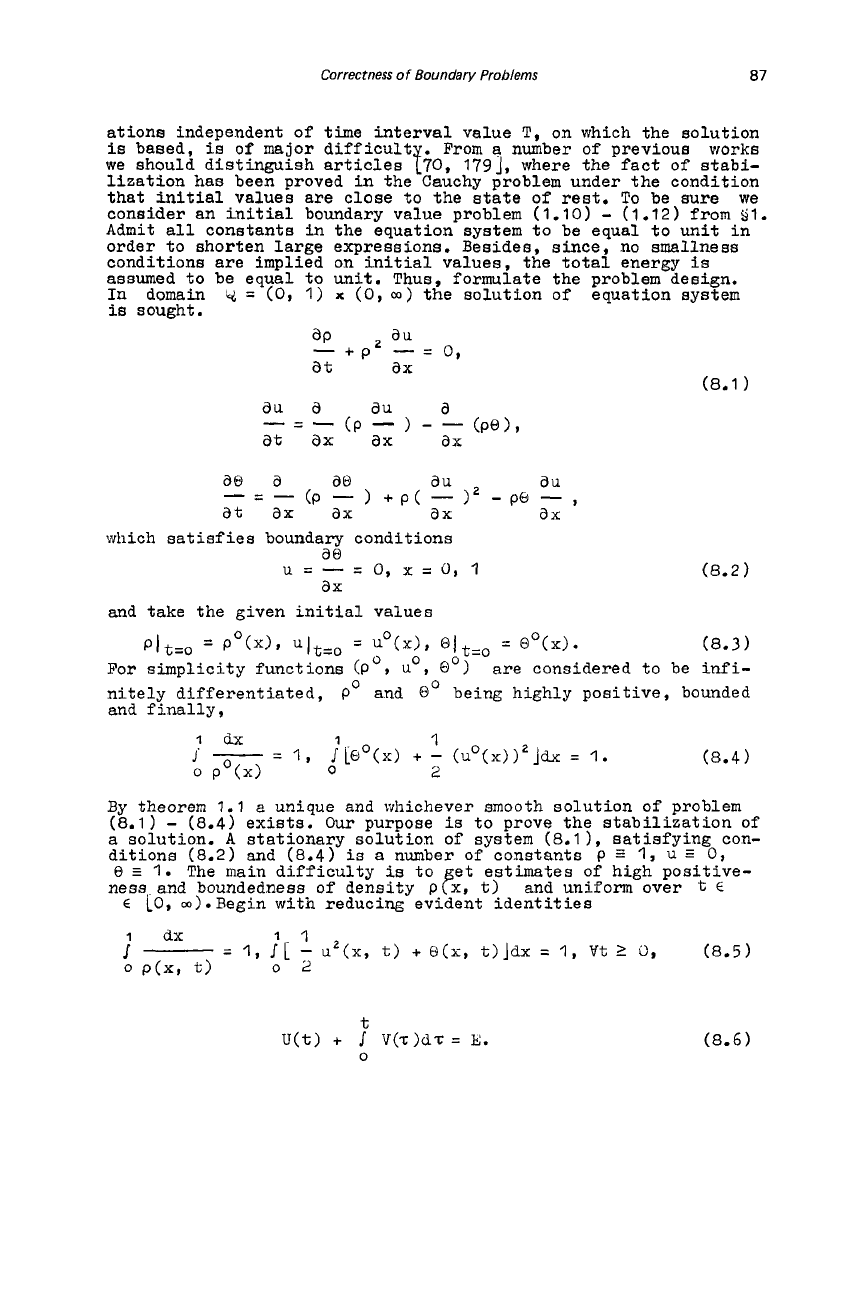
Correctness
of
Boundary
Problems
a7
ations independent of time interval value
T,
on which the solution
is
based,
is
of
major difficult
.
From a number of previous works
we should distinguish articles
f70,
1791,
where the fact
of
stabi-
lization has been proved in the Cauchy problem under the
condition
that initial values are close to the state of rest.
To
be sure we
consider an initial boundary value problem
(1.10)
-
(1.12)
from
$1.
Admit all constants in the equation system to be equal to unit in
order to shorten large expressions. Besides, since,
no
smallness
conditions are implied on initial values, the total energy
is
assumed to be equal to unit. Thus, formulate the problem design.
In domain
Q
=
(0,
1)
x
(0,
m)
the solution of equation system
is
sought.
aP
2
au
-+p
-=
0,
at
ax
(8.1
au
a
au
a
--
--((p-)--
at
ax
ax
ax
(P@
1
,
ae
a
ae
au
au
--_
-
(P-)+P(-)z-pb-,
at ax ax ax
ax
which satisfies boundary conditions
a0
ax
u=-=
0,
x
=
0,
1
(8.2)
and take the given initial values
(8.3)
For simplicity functions
(Po,
uo,
0')
are considered
to
be
infi-
nitely differentiated,
Po
and
0'
being highly positive, bounded
and finally,
0
0
Plt=o
=
P
(XI,
UJt,O
=
(x),
01
t=o
=
Oo(x).
(8.4)
By theorem
1.1
a
unique and whichever smooth solution of problem
(8.1)
-
(8.4)
exists.
Our
purpose
is
to prove the stabilization of
a
solution.
A
stationary solution
of
system
(8.11,
satisfying con-
ditions
(8.2)
and
(8.4)
is
a
number
of
constants
p
=
1,
Us
0,
0
=
1.
The main difficulty
is
to et estimates of high positive-
ness and boundedness
of
density pfx,
t)
and uniform over
t
€
E
LO,
m)
Regin with reducing evident identities
