Ammari H., Benkirane A., Touzani A. (editors) Recent Developments in Nonlinear Analysis
Подождите немного. Документ загружается.

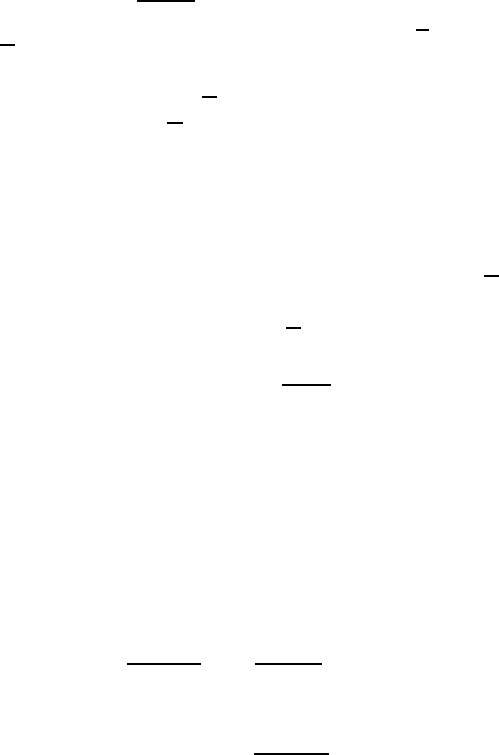
December 28, 2009 12:15 WSPC - Proceedings Trim Size: 9in x 6in recent
210
For all u ∈ W
1,p(x)
(Ω) the following relations hold
(2.1) kuk > 1 ⇒ kuk
p
−
≤ I
p(x)
(u) ≤ kuk
p
+
;
(2.2) kuk < 1 ⇒ kuk
p
+
≤ I
p(x)
(u) ≤ kuk
p
−
.
Finally, we remember some embedding results regarding variable exponent
Lebesgue-Sobolev spaces. For the continuous embedding between variable
exponent Lebesgue-Sobolev spaces we refer to (Theorem 1.1, Fan et al.
8
):
if p : Ω → IR is Lipschitz continuous and p
+
< N , then for any q ∈ L
∞
+
(Ω)
with p(x) ≤ q(x) ≤
Np(x)
N−p(x)
, there is a continuous embedding W
1,p(x)
(Ω) ,→
L
q(x)
(Ω). In what concerns if N < p
−
= ess inf
x∈
Ω
p(x) ≤ p(x) for any
x ∈ Ω, by Theorem 2.2 in Fan et al.
9
we deduce that W
1,p(x)
(Ω) is contin-
uously embedded in W
1,p
−
(Ω). Since N < p
−
it follows that W
1,p
−
(Ω) is
compactly embedded in C(
Ω). Thus, we obtain that W
1,p(x)
(Ω) is contin-
uously embedded in C(Ω).
From now on, E is the space W
1,p(x)
(Ω) with the norm
kuk =
Z
Ω
|∇u|
p(x)
+ |u|
p(x)
dx
1/p(x)
,
which is clearly equivalent to the usual one, on the space C(
Ω) we consider
the norm kuk
∞
= sup
x∈
¯
Ω
|u(x)|. When p
−
> N, Sobolev’s Theorem implies
that E is compactly embedded in C(Ω), hence
(2.3) c = sup
u∈E\{0}
kuk
∞
kuk
< +∞.
Proposition 2.1. Let I : E → E
∗
be the operator defined by
I(u)ϕ =
Z
Ω
|∇u|
p(x)−2
∇u∇ϕ + |u|
p(x)−2
uϕ
dx
for all u, ϕ ∈ E. Then I admits a continuous inverse on E
∗
.
Proof.
Denoting by h., .i the usual inner product in IR
N
. We have for some positive
constant C
1
hI(u), ui
kuk
≥ C
1
I
p(x)
(u)
kuk
≥ C
1
kuk
p
−
−1
.
Hence,
lim
kuk→+∞
hI(u), ui
kuk
= +∞.

December 28, 2009 12:15 WSPC - Proceedings Trim Size: 9in x 6in recent
211
Then I is coercive. Moreover, by simple arguments we can easily verify
that I is hemicontinuous. It remains to show that I is uniformly monotone.
Indeed, recall first the well known inequality
|x − y|
m
≤ 2
m
h|x|
m−2
x −|y|
m−2
y, x − yi, ∀x, y ∈ IR
N
, ∀m ≥ 2.
Thus, it is easy to see that
hI(u
1
) − I(u
2
), u
1
− u
2
i ≥
1
2
p
+
Z
Ω
|∇u
1
− ∇u
2
|
p(x)
+ |u
1
− u
2
|
p(x)
dx
≥ c(p
+
)ku
1
− u
2
k
p
+
,
for every u
1
and u
2
belonging to E. This means that I is uniformly mono-
tone operator in E. Therefore, the conclusion of Proposition 2.1 follows
directly from the result (Theorem 26. A) of Zeidler.
19
Our main result are the following
Theorem 2.1. Suppose p
−
> N and let α satisfying (1.2). Assume that
f : Ω × IR → IR is a function which is measurable in Ω and C
1
in IR
satisfying (1.1), (1.3) and (1.4). Then, there exist an open interval A of
]0, +∞[ and a positive real number t such that, for every λ ∈ A and every
function g : Ω × IR → IR which is measurable in Ω and C
1
in IR satisfying
(1.5), there exists σ > 0 such that for each µ ∈ [0, σ] the system (P
1
) has
at least three weak solutions whose norms in E are less than t.
3. Proof of the main result
We begin by setting
Φ(u) =
Z
Ω
1
p(x)
(|∇u|
p(x)
+ |u|
p(x)
) dx
J(u) = −
Z
Ω
F (x, u) dx
and
Ψ(u) =
Z
Ω
1
γ(x)
|u|
γ(x)
dx
for each u ∈ E. It is well known that Φ and J are well defined and contin-
uously Gˆateaux differentiable with
Φ
0
(u)ϕ =
Z
Ω
(|∇u|
p(x)−2
∇u∇ϕ + |u|
p(x)−2
uϕ) dx

December 28, 2009 12:15 WSPC - Proceedings Trim Size: 9in x 6in recent
212
and
J
0
(u)ϕ = −
Z
Ω
f(x, u)ϕ dx
for all u, ϕ in E. Note that J
0
is compact and Φ is clearly weakly lower
semi-continuous and bounded on each bounded subset of E. Proposition
2.1 ensures that Φ
0
admits a continuous inverse on E
∗
. Moreover, it is easy
to see that
lim
kuk−→+∞
(Φ(u) + λJ(u)) = +∞
for all λ ∈]0, +∞[. Indeed, since E is embedded in C
0
(
Ω), we have
J(u) ≥ −|Ω|(
c
1
α
−
kuk
α
+
∞
+ c
2
kuk
∞
),
where |Ω| denotes the measure of Ω. Then, the coercivity of Φ + λJ can be
easily deduced from (2.1) since α
+
< p
−
and λ > 0 and from the fact that
kuk
∞
≤ Dkuk for some positive constant D. Then (i) is verified.
In the sequel, we will verify the conditions (ii) and (iii) of Theorem 1.1.
By (1.3) and (1.4), it follows that F (x, t) is increasing for t ∈ (1, ∞) and
decreasing for t ∈ (0, 1), uniformly with respect to x and F (x, 0) = 0 is
obvious. Also, by (1.4), we can choose δ > 1 such that
F (x, u) ≥ 0 = F (x, 0) ≥ F (x, τ), ∀u > δ, τ ∈]0, 1[.
Let b, d be two numbers such that 0 < b < min{1, c}, with c given by (2.3)
and d > δ such that d
p
−
|Ω| > 1. Then we obtain
Z
Ω
sup
0≤u≤b
F (x, u) ≤ 0 <
1
c
p
+
b
p
+
d
p
−
Z
Ω
F (x, d) dx.
Define the real number r by
r =
1
p
+
b
c
p
+
.
By choosing
u
0
(x) = 0 and u
1
(x) = d for every x ∈ Ω
we have
Φ(u
0
) = J(u
0
) = 0, Φ(u
1
) =
Z
Ω
1
p(x)
|d|
p(x)
dx
and
J(u
1
) = −
Z
Ω
F (x, d) dx.
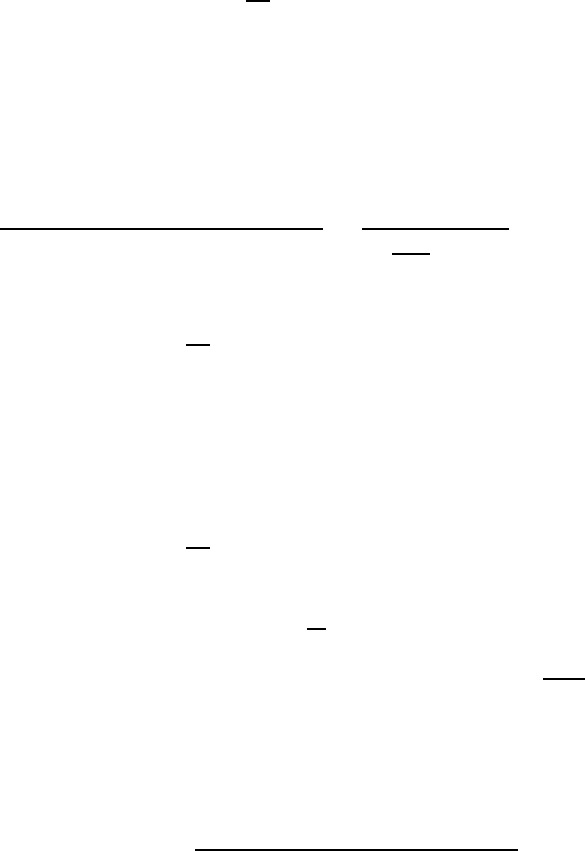
December 28, 2009 12:15 WSPC - Proceedings Trim Size: 9in x 6in recent
213
Then we clearly have
Φ(u
1
) ≥
1
p
+
d
p
−
|Ω| > r.
Thus we deduce that
Φ(u
0
) < r < Φ(u
1
).
Then (ii) in Theorem 1.1 is verified.
On the other hand, we have
−
(Φ(u
1
) − r)J(u
0
) + (r − Φ(u
0
))J(u
1
)
Φ(u
1
) − Φ(u
0
)
= r
Z
Ω
F (x, d) dx
Z
Ω
1
p(x)
|d|
p(x)
dx
> 0.
We also have
1
p
+
I
p(x)
(u) ≤ Φ(u),
which implies that
I
p(x)
(u) ≤ p
+
r < 1,
for all x ∈ Ω and for all u ∈ E such that Φ(u) ≤ r. Using (2.1), we get
kuk ≤ 1. This implies that
1
p
+
kuk ≤ Φ(u) ≤ r.
Taking into account that
|u(x)| ≤ c(p
+
r)
1
p
+
< b
for all x ∈ Ω and for all u ∈ E such that Φ(u) ≤ r, with c = sup
u∈E
kuk
∞
kuk
.
It follows
− inf
u∈Φ
−1
(]−∞,r])
J(u) = sup
Φ(u)≤r
−J(u) ≤
Z
Ω
sup
0≤u≤b
F (x, u) dx ≤ 0.
Consequently, we obtain
inf
u∈Φ
−1
(]−∞,r])
J(u) >
(Φ(u
1
) − r)J(u
0
) + (r − Φ(u
0
))J(u
1
)
Φ(u
1
) − Φ(u
0
)
.
This means that condition (iii) in Theorem 1.1 is verified.
Moreover, since the function G : Ω × IR −→ IR is a measurable in
Ω and C
1
in IR × IR satisfying the condition (1.5), then the functional
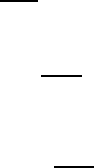
December 28, 2009 12:15 WSPC - Proceedings Trim Size: 9in x 6in recent
214
Ψ(u) =
Z
Ω
Z
u(x)
0
g(x, t) dt
!
dx is well defined and continuously Gˆateaux
differentiable on E, with compact derivative, and one has
Ψ
0
(u)ϕ =
Z
Ω
g(x, u(x))ϕ(x) dx
for all u, ϕ ∈ E. So, in view of Proposition 2.1 and Theorem 1.1, the proof
of Theorem 2.1 is achieved.
Corollary 3.1. Suppose p
−
> N. Let α satisfying (1.2). Then there exists
an open interval A of ]0, +∞[ and a positive real number t such that, for
every λ ∈ A and every γ, with γ > 1 there exists σ > 0 such that for each
µ ∈ [0, σ] the system (P
1
)
0
has at least two weak nonzero solutions whose
norms in E are less than t.
Proof.
Let
Φ(u) =
Z
Ω
1
p(x)
(|∇u|
p(x)
+ |u|
p(x)
) dx
J(u) = −
Z
Ω
(
1
α(x)
|u|
α(x)
− u) dx
and
Ψ(u) =
Z
Ω
1
γ(x)
|u|
γ(x)
dx
for each u ∈ E. It is well known that Φ and J are well defined and contin-
uously Gˆateaux differentiable with
Φ
0
(u)ϕ =
Z
Ω
(|∇u|
p(x)−2
∇u∇ϕ + |u|
p(x)−2
uϕ) dx
and
J
0
(u)ϕ = −
Z
Ω
|u|
α(x)−2
uϕ dx
for all u, ϕ in E. Note that J
0
is compact and Φ is clearly weakly lower
semi-continuous and bounded on each bounded subset of E. Proposition
2.1 ensures that Φ
0
admits a continuous inverse on E
∗
. Moreover, it is easy
to see that
lim
kuk−→+∞
(Φ(u) + λJ(u)) = +∞
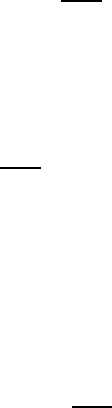
December 28, 2009 12:15 WSPC - Proceedings Trim Size: 9in x 6in recent
215
for all λ ∈]0, +∞[. Consider now
H(x, u) =
1
α(x)
|u|
α(x)
− u.
Since α(x) > 0 for all x ∈ Ω we have
lim
|u|→∞
H(x, u) = +∞.
Choose δ > 1 such that
1
α(x)
|u|
α(x)
− u > 0
for all u > δ. Hence we get
H(x, u) ≥ 0 = H(x, 0) ≥ H(x, τ), ∀u > δ, τ ∈]0, 1[.
Let us choose b, d two real numbers and u
0
and u
1
the same as in the
proof of Theorem 2.1. Then we obtain (ii) and (iii) of Theorem 1.1 when
adapting the techniques given in the proof of Theorem 2.1. On the other
hand, the functional
Ψ(u) =
Z
Ω
1
γ(x)
|u|
γ(x)
dx
is continuously Gˆateaux differentiable on E, with compact derivative. So,
in view of Theorem 1.1, the proof is completed.
Acknowledgement
The research of S. El Manouni is supported by Al-Imam University project
No. 28/12.
References
1. G. Anello and G. Cordaro, Existence of solutions of the Neumann problem
involving the p-Laplacian via variational principle of Ricceri. Arch. Math.
(Basel)79 (2002)274-287.
2. G. Bonanno, A minimax inequality and its applications to ordinary differen-
tial equations. J. Math. Anal. Appli. 270(2002) 210-219.
3. G. Bonanno, Some remarks on a three critical points theorem, Nonlinear
Anal. 54(2003) 651-665.
4. G. Bonanno and P. Candito, Three solutions to a Neumann problem for
elliptic equations involving the p-Laplacian. Arch. Math. 80(2003) 424-429.
5. G. Dai, Three solutions for a Neumann-type differential inclusion problem
involving the p(x)-Laplacian, Nonlinear Anal. to appear
6. S. El Manouni and M. Kbiri Alaoui, A result on elliptic systems with Neu-
mann conditions via Ricceri’s three critical points theorem, Nonlinear Anal.
to appear
December 28, 2009 12:15 WSPC - Proceedings Trim Size: 9in x 6in recent
216
7. O. Kov´aˇcik and J. R´akosn´ık, On spaces L
p(x)
and W
1,p(x)
, Czechoslovak
Math. J. 41(1991) 592-618.
8. X. L. Fan, J. Shen and D. Zhao, Sobolev embedding theorems for spaces
W
k,p(x)
(Ω), J. Math. Anal. Appl. 262(2001) 749-760.
9. X. L. Fan and D. Zhao, On the spaces L
p(x)
(Ω) and W
m,p(x)
(Ω), J. Math.
Anal. Appl. 263(2001) 424-446.
10. M. Mihˇailescu, Existence and multiplicity of solutions for a Neumann prob-
lem involving the p(x)-Laplace operator, Nonlinear Anal., 67(2007) 1419-
1425.
11. J. Musielak, Orlicz spaces and modular spaces. Lecture Notes in Mathemat-
ics, 1034. Springer-Verlag, Berlin (1983).
12. B. Ricceri, On three critical points theorem, Arch. Math. (Basel) 75 (2000),
220-226.
13. B. Ricceri, A three critical points theorem revisited. Nonlinear Anal. to ap-
pear (2008).
14. B. Ricceri, A general variational principle and some of its applications. J.
Comput. Appl. Math. 113(2000) 401-410.
15. M. Ruzicka, Electrorheological fluids: Modelling and Mathematical Theory,
Lecture Notes in Math., Springer-Verlag, Berlin (2002).
16. M. Ruzicka, Flow of shear dependent electrorheological fluids. C. R. A. S.
Sci. Paris. S´er. I. Math. 329(1999) 393-398.
17. X. Shi and X. Ding, Existence and multiplicity of solutions for a general
p(x)-Laplacian Neumann problem, Nonlinear Anal. to appear
18. J. Simon, r´egularit´e de la solution d’une equation non lin´eaire dans IR
N
.
LMN 665, P. Benilan ed., Berlin-Heidelberg-New York 1978.
19. E. Zeidler, Nonlinear functional analysis and its applications. Vol. II/B.
Berlin-Heidelberg-New York 1978.

December 28, 2009 12:15 WSPC - Proceedings Trim Size: 9in x 6in recent
217
Positive solutions with changing sign energy to nonhomogeneous
elliptic problem of fourth order
M. Talbi
∗
and N. Tsouli
†
D´epartement de Math´ematiques et Informatique Facult´e des Sciences
Universit´e Mohamed 1, Oujda, Morocco
E-mails:
∗
talbi
md@yahoo.fr,
†
tsouli@hotmail.com
In this paper, we study the existence for two positive solutions to a nonhomoge-
neous elliptic equation of fourth order with a parameter λ such that 0 < λ <
ˆ
λ.
The first solution has a negative energy while the energy of the second one is
positive for 0 < λ < λ
0
and negative for λ
0
< λ <
ˆ
λ. The values λ
0
and
ˆ
λ
are given under variational form and we show that every corresponding critical
point is solution of the nonlinear elliptic problem (with a suitable multiplicative
term).
Keywords: Ekeland’s principle; p-Laplacian operator; Palais-Smale condition.
1. Introduction
We consider the problem with Navier boundary conditions
(P
λ
)
∆
2
p
u = λ|u|
q−2
u + |u|
r−2
u in Ω
u = ∆u = 0 on ∂Ω.
Here Ω is a smooth domain in R
N
(N ≥ 1), ∆
2
p
is the p-biharmonic operator
defined by ∆
2
p
u = ∆(| ∆u |
p−2
∆u), λ is a positive parameter, p, q and r
are reals such that
1 < q < p < r < p
∗
2
, where
p
∗
2
=
Np
N − 2p
if p < N/2,
p
∗
2
= +∞ if p ≥ N/2.
Such kind of problems with combined concave and convex nonlinearities
were studied recently by several authors
2–7,9–11,17
in the case of operator
∆
p
. Our main results here can be summarized as follows:
Let us put X = W
2,p
0
(Ω) ∩ W
2,p
(Ω). We find two characteristic values λ
0
December 28, 2009 12:15 WSPC - Proceedings Trim Size: 9in x 6in recent
218
and
ˆ
λ (λ
0
<
ˆ
λ) under variational form, i.e.
(V )
λ
0
= C
0
(p, q, r) inf
u∈X\{0}
F (u) and
ˆ
λ =
ˆ
C(p, q, r) inf
u∈X\{0}
F (u),
such that two branches of positive solutions to (P
λ
) exist for λ ∈]0,
ˆ
λ[ (the
functional F will be given below). Moreover, the energy of the first positive
solution is negative for λ ∈]0,
ˆ
λ[ while the energy of the second positive
solution changes sign at λ
0
, i.e. it is positive forλ ∈]0, λ
0
[ and negative for
λ ∈]λ
0
,
ˆ
λ[. Notice that these two positive solutions are found simultaneously
and that our approach does not use the mountain-pass lemma.
On the other hand, we show that every solution of (V ) is a solution of
the problem (P
λ
) (with a suitable multiplicative term). This second point
lets expect that the first nonlinear eigenvalue ζ of (V ), i.e.
ζ = sup{λ > 0 : (P
λ
) has a nonnegative solution}
may satisfy a variational problem similar to (V ) (see
4
for p = 2). Let us
precise that
ˆ
λ coincides with ζ when q → p and that
ˆ
λ constitutes a good
minoration of ζ in the general case 1 < q < p.
We consider the transformation of Poisson problem used by P.Dr´abek
and M.ˆotani (cf.
12
):
We recall some properties of the Dirichlet problem for the Poisson equation:
−∆u = f in Ω,
u = 0 on ∂Ω.
(1)
It is well known that (1) is uniquely solvable in W
2,p
(Ω) ∩ W
1,p
0
(Ω) for all
f ∈ L
p
(Ω) and for any p ∈]1, +∞[.
We denote by :
X = W
2,p
(Ω) ∩ W
1,p
0
(Ω),
kuk
p
= (
Z
Ω
|u|
p
dx)
1/p
the norm in L
p
(Ω),
kuk
2,p
= (k∆uk
p
p
+ kuk
p
p
)
1/p
the norm in X,
kuk
∞
the norm in L
∞
(Ω),
and < ., . > is the duality bracket between L
p
(Ω) and L
p
0
(Ω), where p
0
=
p/(p −1). Denote by Λ the inverse operator of −∆ : X → L
p
(Ω).
The following lemma gives us some properties of the operator Λ (cf.,
1216
)
Lemma 1.1.
(i) (Continuity): There exists a constant c
p
> 0 such that
kΛfk
2,p
≤ c
p
kfk
p

December 28, 2009 12:15 WSPC - Proceedings Trim Size: 9in x 6in recent
219
holds for all p ∈]1, +∞[ and f ∈ L
p
(Ω).
(ii) (Continuity) Given k ∈ N
∗
, there exists a constant c
p,k
> 0 such that
kΛfk
W
k+2,p ≤ c
p,k
kfk
W
k,p
holds for all p ∈]1, +∞[ and f ∈ W
k,p
(Ω).
(iii) (Symmetry) The following identity:
Z
Ω
Λu · vdx =
Z
Ω
u ·Λvdx
holds for all u ∈ L
p
(Ω) and v ∈ L
p
0
(Ω) with p ∈]1, +∞[.
(iv) (Regularity) Given f ∈ L
∞
(Ω), we have Λf ∈ C
1,α
(
¯
Ω) for all α ∈]0, 1[;
moreover, there exists c
α
> 0 such that
kΛfk
C
1,α
≤ c
α
kfk
∞
.
(v) (Regularity and Hopf-type maximum principle) Let f ∈ C(
¯
Ω) and f ≥ 0
then w = Λf ∈ C
1,α
(
¯
Ω), for all α ∈]0, 1[ and w satisfies: w > 0 in
Ω,
∂w
∂n
< 0 on ∂Ω.
(vi) (Order preserving property) Given f, g ∈ L
p
(Ω) if f ≤ g in Ω, then
Λf < Λg in Ω.
Remark 1.1. (∀u ∈ X)(∀v ∈ L
p
(Ω)) v = −∆u ⇐⇒ u = Λv.
Let us denote N
p
the Nemytskii operator defined by
N
p
(v)(x) = |v(x)|
p−2
v(x) if v(x) 6= 0
N
p
(v)(x) = 0 if v(x) = 0,
and we have ∀v ∈ L
p
(Ω), ∀w ∈ L
p
0
(Ω) :
N
p
(v) = w ⇐⇒ v = N
p
0
(w).
We define the functionals P, Q, R : L
p
(Ω) → R as follows:
P (v) = kvk
p
p
, Q(v) = ||Λv||
q
q
and R(v) = ||Λv||
r
r
.
The operator Λ enables us to transform problem (P
λ
) to an other problem
which we will study in the space L
p
(Ω).
Definition 1.1. We say that u ∈ X \ {o} is a solution of problem (P
λ
), if
v = −∆u is a solution of the following problem
(P
0
λ
)
Find v ∈ L
p
(Ω) \ {o}, such that
N
p
(v) = λΛ(N
q
(Λv)) + Λ(N
r
(Λv)) in L
p
0
(Ω).
