Ames W.F., Harrel E.M., Herod J.V. (editors). Differential Equations with Applications to Mathematical Physics
Подождите немного. Документ загружается.


270
L.
E.
Payne
where
X
is the appropriate Poincark constant. But
(3.1)
implies that
Il
u(t)llZ,
2
Ilu(O)llZ,
exp(2vXt) (3-2)
which leads to
a
contradiction since ujui cannot remain bounded
as
t
--t
00.
Thus for
T
=
00
our theorem is vacuous. In fact, for
arbitrary
T
we have, letting
t
--t
T
in (3.2)
WID1
2
{
J
D
fifidz}exP(2vw,
M:
2
ID[-'{
J
D
f;
f;dz} exp(2vXT).
(3.3)
when
101
is the volume measure of
D.
This means that for given
f;,
T,
v
and
D,
M1
cannot be arbitrary but, in fact, must satisfy
(3.4)
The same inequality must clearly be satisfied by
Adz
with
fi
replaced
by
h.
Another way of looking at this is that given any
f;,
v,
D
and
MI
with
M:
>
max
fi
fi
(3.5)
D
then our Theorem
1
will hold only
if
This is
a
necessary but not
a
sufficient condition. The point to be
made is that given
v,
D,
and
f;,
then the bound
MI
and the time
T
cannot be chosen independently. Thus
if
we wish to develop
a
numerical scheme for finding
an
approximate solution of
(1.1)-(1.3)
we must be careful
to
choose the Mi's sufficiently large. There is
of
course
a
trade
off
since the larger the
M;
the larger the constants
K
in the theorem and the smaller the exponent
6.
As was pointed out in
[3],
[5],
and
[GI
the continuous dependence
results stated in Theorems
1-3
could actually have been derived un-
der somewhat less restrictive constraint set restrictions. The
L,
constraints could have in some cases been replaced by
L,
constraints
for suitable values of
p.
In this case, however, it becomes somewhat
more difficult to make the inequalities explicit.

On Stabilizing
Ill-Posed
Cauchy
Problems
271
Bibliography
[l]
P.
S.
Crooke and L.
E.
Payne,
Continuous dependence
on
geom-
etry
for
the backward heat equation,
Math. Meth. in Appl. Sci.,
6
1984,
pp.
433-438.
[2]
G.
P.
Galdi and
B.
Straughan,
Stability
of
solutions to the
Navier-Stokes equations backward
in
time,
Arch. Rat. Mech.
Anal.,
101
1988,
pp.
107-114.
[3]
R.
J.
Knops and
L.
E.
Payne,
On the stability
of
solutions
of
the
Navier-Stokes equations backward
in
time,
Arch. Rat. Mech.
Anal.,
29
19G8,
pp.
331-335.
[4]
L.
E.
Payne,
Improperly posed problems
in
partial diflerential
equations,
Regional Conference Series in Applied Mathematics
#22,
SIAM, Philadelphia,
1975.
[5]
L.
E.
Payne,
Some remarks on ill-posed problems
for
viscous
fluids,
Int.
J.
Eng. Sci., to appear,
1992.
[GI
L.
E.
Payne,
Continuous dependence on spatial geometry
for
solutions
of
the Navier-Stokes equations backward
in
time,
to
appear.
[7]
L.
E.
Payne and
B.
Straughan,
Comparison
of
viscous flows
backward in time with small data,
hit.
J.
Non-Linear Mech.,
24
1989,
pp.
209-214.
[8]
J.
Persens,
On
stabilizing ill-posed problems
for
partial difleren-
tial equations under perturbations
of
the geometry
of
the domain,
Ph.D. thesis, Cornell University,
1986.
[9]
B.
Straughan,
Backwad uniqueness and unique continuation
for
solutions to the Navier-Stokes equations on an exterior domain,
J.
Math. Pures et Appl.,
62
1983,
pp.
49-62.

This page intentionally left blank

Evans' Function, Melnikov's
Integral, and Solitary
Wave
Instabilities
Robert
L.
Peg0
*
Department
of
Mathematics
University
of
Maryland
College Park,
MD
20742
Michael
I.
Weinstein
*
Department
of
Mathematics
University
of
Michigan
Ann Arbor,
h4I
48109
1
Introduction
In this note we discuss recent results on:
(i)
a
method for detecting the eigenvalues
of
systems
of
ordinary
differential equations with asymptotically constant coefficients, and
(ii) applications of this method to the detection of instabilities
and transitions to instability of solitary wave solutions to equations
which model long wave propagation in dispersive media.
We shall illustrate and apply this theory for solitary waves of
a
generalization of the Korteweg-de Vries equation (gKdV):
where
f(u)
=
uP+'/(p
t
l),
and
p
2
1
is real.
A
more detailed discussion including applications to generaliza-
tions of
a
Boussinesq equation (Bou),
a
regularized long wave equa-
tion of Benjamin, Bona
SC
Ma.honey (BBM), and
a
KdV-Burgers
equation can be found
in
[15,
141.
The technique developed to study
the spectrum of the linexized operator about the solitary wave plays
*Partially
supported
by
grants
from
the National Science Foundation.
Differential Equations with Copyright
@
1993
by Academic Press,
Inc.
Applications to Mathematical
All
rights
of
reproduction
in
any
form
reserved.
Physics
ISBN
0-12-056740-7
273
274
Robert
L.
Peg0
and
Michael
I.
Weinstein
an important role in the proof of
asymptotic
stability of solitary waves
of gKdV
[16,
171.
The method we use is based
on
the study of Evans’ function
D(X),
and in particular, new formulas for the derivatives of
D(X). D(X)
was
introduced by
J.
W. Evans in his study of the stability of traveling
wave solutions of reaction-diffusion systems that model nerve impulse
propagation
IS].
In addition to discussing how unstable eigenvalues
are detected using
D(X),
we discuss how:
(iii)
D(X)
detects “resonance poles”. These are pole singulari-
ties of
a
suitably defined resolvent operator, which play
a
role in the
mechanism of transition to instability. This mechanism is quite dif-
ferent from that seen in transitions to instability in finite dimensional
Hamiltonian systems. Resonance poles arise in quantum scattering
theory (e.g. Augur states for the helium atom [IS]) and in plasma
physics (Landau damping for the Vlasov-Poisson system
[5,
61).
Finally, we point out:
(iv)
a
connection between our expression for
D’(X)
and the
Mel-
nikov integral
(see
[lo,
13]),
which was introduced to study the order
of splitting, under perturbation, of the stable and unstable manifolds
of
a
homoclinic point of
an
autonomous system of
ODE’S.
2
Solitary Waves and Linearized Stability
The generalized KdV equation admits solitary wave solutions for any
c
>
0,
of the form
u(z,t)
=
u,(z
-
ct)
where
u,(z)
=
asech2/P(yz)
with
a
=
(c(p
+
l)(p
+
2)/2)l/p,
y
=
pc1i2/2. The wave profile
uc(z)
decays to zero exponentially as
IzI
+
00.
To consider the stability of such waves, we study the evolution
of small perturbations of such waves, writing
u(z,t)
=
uc(z
-
ct)
+
v(z
-
ct,t).
Neglecting terms nonlinear in the perturbation
v,
the
linearized evolution equation for the perturbation of the wave is
a,.
+
ax((u:
-
C).)
+
a,v
=
0
.
(2)
We look for solutions of the form
v
=
extY(z),
where
X
E
C
and
Y
satisfies
aXL,Y
=
XY
,
(3)

Evans' Function, Melnikov's Integral, Solitary Wave Instabilities
275
where
L,
=
-6':
+
c
-
ug.
This eigenvalue problem takes the form of
an eigenvalue problem commonly associated with linear Hamiltonian
sys tems:
JLY
=XY,
where
J
is skew symmetric and
L
is self-adjoint. If
(3)
admits
a
square integrable solution for some
X
with ReX
#
0,
we call
X
an
unstable eigenvalue for
(3)
and
Y
the associated eigenfunction. (By
reflection symmetry,
-A
is an eigenvalue if
X
is.)
Previous work ([a,
3,
4,
12, 20, 21, 221, see also
[9])
has shown
that
u,
is nonlinearly stable in
H'
(modulo spatial translations) if
and unstable if
d
dc
--"u,]
<
0
.
(5)
Here the functional
N[u]
=
J-",
u2
dx
is
a
generalized momen-
tum associated with the IIaniiltonian structure of (gKdV), and is
independent of time for solutions.
For
the particular example
at
hand,
(4)
holds
if
and only
if
y
<
4.
The stability proofs rely on
establishing that
u,
is
a
local minimizer of
a
conserved energy func-
tional, subject to the constraint of fixed momentum.
Here we discuss how:
If
the instability condition
(5)
holds, a real
unstable eigenvalue exists with
X
>
0.
This gives rise
to
a
non-
oscillatory and exponentially growing solution
of
the linearized evo-
lution equation.
The same was proved in
[15],
for generalizations of
the BBM and Boussinesq equations. These results clarify the mecha-
nisms
for
the instability proved for gKdV and gBBM in
[4]
and
[19];
see [12] for an alternative approach to studying linear exponential
instability. Our result concerning gBou seems to be the first regard-
ing the stability
or
instability of the solitary waves
of
this equation.
The methods used in the works mentioned above apparently fail to
decide stability in this equation.

276
Robert
L.
Peg0
and
Michael
I.
Weinstein
3
Evans' Function and Unstable Eigenvalues
The method we use to study the existence of eigenvalues for (3)
is related to the study of eigenvalues in boundary value problems
for ordinary differential operators. As
1x1
--+
00,
the coefficients in
equations (3) converge rapidly to those of the following constant
coefficient equation
For
ReX
>
0,
this equation has solutions
Y(x,X)
=
epj"
for
j
=
1,2,3, where the
pj,
which depend on
A,
satisfy
RepI(X)
<
0
<
Repj(X)
for
j
=
2,3.
Correspondingly, for equation (3) there is
a
1-dimensional subspace of
solutions which decay as
z
+
00,
spanned by
a
function
Y+(x,
A)
and
2-dimensional subspace of solutions which decay to zero
as
x
+
-00,
spanned by functions
Y;(z,
A)
and
YF(x,
A).
In particular,
Y+
may
be normalized
so
that
X
is an eigenvalue when these subspaces meet nontrivially. The angle
between these subspaces may be measured by
a
Wronskian-like ana-
lytic function
D(X),
named Evans' function by Alexander, Gardner
&
Jones
[l],
after
J.
W. Evans who pioneered its
use
in the study
of stability of nerve impulses [S]. In
[ll,
11,
a
geometric/topological
approach using Evans' function is developed to study the stability
of
traveling waves of singularly perturbed reaction diffusion systems.
We now obtain
D(X)
in the present application to KdV. Consider,
for
ReX
>
0,
the solution
Y+(z,X)
as
x
+
00.
Then
D(X)
may be
defined by the relation
This interpretation of
D(X)
as
a
transmission
coefficient is exploited
in [23].

Evans’ Function, Melnikov’s Integral, Solitary Wave Instabilities
277
In equation
(3),
for Rex
>
0,
if
D(X)
vanishes, then
X
is an
eigenvalue, and conversely. (It also then follows that
-A
<
0
is an
eigenvalue, though quite possibly not
a
zero of
D(X).)
What about ReX
=
O?
In this case, it turns out that Rep2(X)
=
0,
and therefore the vanishing of
D(X)
on the imaginary
axis
implies
the existence of
a
solution which is exponentially decaying as
x
--$
too,
but which is merely bounded for
x
+
-oo
(cf.
(8)).
In fact one
can show, by
a
perturbation argument about the operator in
(6)
that
the imaginary axis
is
covered by essential spectrum. Using symmetry
properties of gKdV we can
in
fact show that zeros of
D(X)
embedded
in the essential spectrum are eigenvalues of
(3).
Theorem
1
(i)
D(X)
is defined and is analytic in the half-plane
R
=
{A
:
Re
X
>
-€},
for some
E
>
0.
(ii) If ReX
2
0,
then
X
is
a
zero of
D(X)
if
and
only
if
X
is an
eigenvalue for the problem
(3).
The corresponding eigenfunction
decays exponentially as x
+
foo.
(iii)
D(X)
-+
1
as
1x1
-+
oo
in
Q.
(iv)
D(X)
is
real
for real
A.
It happens naturally that
D(0)
=
0
when linearizing about
a
traveling wave: for
X
=
0
the function
Y(x)
=
&u,
satisfies
(3).
This follows from translation invariance in
x.
The crux of our method is that we have new integral formulae
for derivatives of
D(X).
A
special case of these formulae which we
use is:
Theorem
2
For
all
X
E
0,
Here, Y+(x,X) is the solution
of
(3)
satisfying
(7),
which decays as
x
+
+oo,
and Z-(x,X)
is
a
solution of the adjoint of
(3)
which
decays as x
--f
-m.
These solutions
are
taken
with
a
suitable nor-
maliza
t
ion.
278
Robert
L.
Peg0
and
Michael
I.
Weinstein
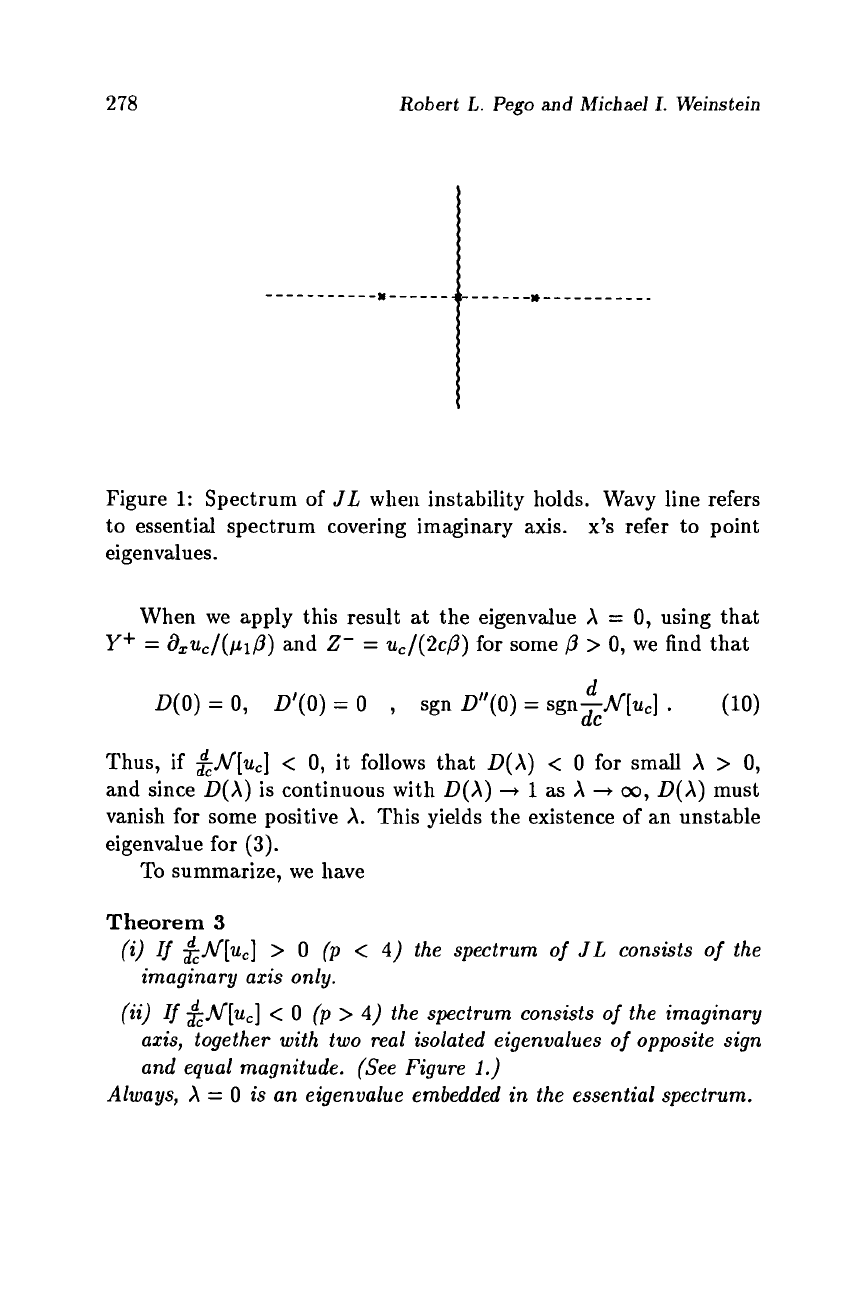
Figure
1:
Spectrum of
JL
when instability holds. Wavy line refers
to essential spectrum covering imaginary
axis.
x’s refer
to
point
eigenvalues.
When we apply this result
at
the eigenvalue
X
=
0,
using that
Y+
=
&u,/(p~P)
and
2-
=
uC/(2cP)
for some
P
>
0,
we find that
d
dc
D(O)
=
0,
D’(O)
=
O
,
sgn
D”(O)
=
sgn-N[u,]
.
(10)
Thus, if &N[u,]
<
0,
it
follows that
D(X)
<
0
for small
X
>
0,
and since
D(X)
is
continuous with
D(X)
+
1
as
X
+
00,
D(X)
must
vanish for some positive
A.
This yields the existence
of
an unstable
eigenvalue for
(3).
To
summarize, we have
Theorem
3
(i)
If
$N[u,]
>
0
(p
<
4)
the spectrum
of
JL
consists
of
the
imaginary axis only.
(ii)
If
$N[uc]
<
0
(p
>
4)
the spectrum consists
of
the imaginary
axis, together with two real isolated eigenvalues
of
opposite sign
and equal magnitude. (See Figure
1.)
Always,
X
=
0
is an eigenvalue embedded in the essential spectrum.

Evans’ Function, Melniliov’s Integral, Solitary Wave Instabilities
279
4
Transition to Instability; Resonance
Poles
to Eigenvalues
As
noted in section
3,
a
pair of real eigenvalues appear for
p
>
4,
yielding the linearized exponential instability of the solitary wave.
What is the origin of these unstable eigenvalues? Is there some trace
of them in the stable regime
p
<
4?
For
gKdV (and other equations) we study the transition to insta-
bility by considering the Taylor expansion of
D(X,p)
in
a
neighbor-
hood of the transition point
(X,p)
=
(0,pcrjt)
=
(0,4).
(For
gKdV
with
a
power nonlinearity the transition point does not depend on
the wave speed
c,
due to
a
scaling property of the equation.)
We have:
Theorem
4
The
Taylor
expunsion
ofD(X,p)
at
(0,pcrit)
is
with
a
a,”D(O,pcrjt)
#
0
and
6
3
aPaiD(0,pcrjt)
#
0.
Therefore, the mechanism for transition from stability to insta-
bility may be described as follows:
as
p
varies from below
pcr;t
to
above
pcrit,
a
real root
A,-&)
of
D(X,p)
=
0
crosses from the negative
real
axis
A0
<
0
to the positive real axis
A0
>
0,
with
X(pcrjt)
=
0.
Xo
is
a
locally analytic function of
p,
and
aPXo(pcrjt)
#
0.
Once the
root
Xo
is nonnegative
it
is an eigenvalue of
(3).
Its existence implies
the existence of
a
symmetrically placed eigenvalue of
(3)
at
-XO.
In finite dimensional Hamiltonian systems the mechanism for the
emergence of two real eigenvalues
as
a
parameter varies is quite sim-
ple and standard: If rcrjt denotes the value
at
which the transition
from stability
(r
<
rcrit)
to instability
(T
>
rcrjt),
then for
T
<
a
pair of pure imaginary eigenvalues exists which coalesce at the ori-
gin for
T
=
rcrjt
and branch
off
symmetrically about the origin on
the real
axis
for
T
>
rCrjt.
See figure
2.
This scenario is
called
an
“exchange of stability”
[7].
By contrast, the transition to instability here does not involve any
purely imaginary eigenvalues. An interpretation of when neg-
ative is that it corresponds to what is known in quantum scattering
