Allman E.S., Rhodes J.A. Mathematical Models in Biology: An Introduction

Подождите немного. Документ загружается.

166 Modeling Molecular Evolution

b. Suppose these sequences came from currently living species whose

evolutionary relationships we would like to deduce. Draw the evo-

lutionary tree that you believe best describes the relationship. Ex-

plain how you have used the distance data in your reasoning. Note:

This problem is the subject of the next chapter.

4.5.16. a. Show that the formula d

K 2

=−

ln(1 − 2β − 2γ ) −

ln(1 −

4γ ) for the Kimura 2-parameter model can be derived from the

formula for d

K 3

by setting δ = γ .

b. Suppose we have two aligned sequences S

and S

. Explain why the

proportion p

of sites that undergo transitions is a good estimate for

β and why the proportion of sites p

that undergo transversions is

a good estimate for 2γ . Use this to derive the Kimura 2-parameter

distance formula for sequence data that is given in the text.

4.5.17. Derive the formula for the Kimura 3-parameter distance as follows.

Refer to exercise 4.4.21 of the last section, which gives formulas for

the parameters β



, γ



, and δ



in M(β



,γ



,δ



) = M(α, β, γ )

a. Show that

1 − 2β



− 2δ



= (1 − 2β − 2δ)

1 − 2β



− 2γ



= (1 − 2β − 2γ )

and

1 − 2γ



− 2δ



= (1 − 2γ − 2δ)

b. Use part (a) to show

ln(1 − 2β



− 2δ



) + ln(1 − 2β



− 2γ



) + ln(1 − 2γ



− 2δ



)

= t

(

ln(1 − 2β − 2δ) + ln(1 − 2β − 2γ ) + ln(1 − 2γ − 2δ)

)

c. Assuming β, γ , and δ are all small, use the approximation ln(1 +

x) ≈ x for x ≈ 0 in the last equation to get

ln(1 − 2β



− 2δ



) + ln(1 − 2β



− 2γ



) + ln(1 − 2γ



− 2δ



)

≈−4t

(

β + γ + δ

)

d. Explain why β + γ + δ should be interpreted as the total rate of

base substitution, and thus why it is reasonable to deﬁne

K 3

=−



ln(1 − 2β



− 2δ



) + ln(1 − 2β



− 2γ



)

+ln(1 − 2γ



− 2δ



)



4.5. Phylogenetic Distances 167

4.5.18. (Linear Algebra) The goal of this problem is to show that the Jukes-

Cantor distance is a special case of the log-det distance. You will need

to know the following two facts about determinants of k × k matrices

that are proved in a Linear Algebra course:

i) det(cA) = c

det(A).

ii) det(A) = the product of A’s k eigenvalues.

a. Suppose two sequences, S

and S

, of length N , were compared and

the frequency table F was found to be exactly described by a Jukes-

Cantor matrix M(α) with base distribution (1/4, 1/4, 1/4, 1/4) for

. Show that F =

M(α).

b. Explain why f

= f

= (N /4, N/4, N/4, N /4).

c. Use the facts above to show that, in this case, d

, S

) =

, S

4.5.19. (Linear Algebra) Proceeding as in the last problem, show that the

Kimura 3-parameter distance is a special case of the log-det distance.

4.5.20. (Linear Algebra) Show the log-det distance formula is symmetric

and additive through the following steps. You will need to know the

following three facts about determinants of k × k matrices that are

proved in a Linear Algebra course:

i) det(A

) = det( A), where A

, the transpose of A, is a matrix

whose (i, j ) entry is the ( j, i) entry of A.

ii) det(AB) = det(A) det(B).

iii) If the (i, j ) entries of D are all zero for i = j, then

det(D) = D(1, 1) · D(2, 2) ···D(k, k).

a. Use fact (i) to show the log-det distance is symmetric.

b. For the situation in Figure 4.2, with initial base distribution p

in S

, explain why p

= M

0→1

and p

= M

1→2

are the base

distributions in S

and S

, respectively.

c. For the vector p

= (a, b, c, d), let







√

a 000

√

b 00

√

c 0

000

√







Then, for each pair i, j with 0 ≤ i < j ≤ 2, deﬁne the matrix

i→j

= D

−1

i→j

168 Modeling Molecular Evolution

Show N

1→2

0→1

= N

0→2

, and use fact (ii) to conclude

(

det(N

1→2

)

+ ln

(

det(N

0→1

)

= ln

(

det(N

0→2

)

d. Show the relative frequency array for comparing S

to S

is G

i→j

, and then use fact (ii) to show

ln(det(G

i→j

)) = ln(det(N

i→j

)) + ln(det(D

)).

e. Combine parts (c) and (d), and fact (iii) to show the log-det distance

is additive.

Projects

1. Investigate how the Jukes-Cantor distance formula performs on simulated

sequence data produced according to the Jukes-Cantor model.

The MATLAB program mutate can be used to simulate DNA mu-

tations according to any speciﬁed Markov model of base substitution.

Then, the Jukes-Cantor distance can be computed for the sequences so

produced. However, seldom does the Jukes-Cantor distance exactly re-

cover the value of αt used in the simulation.

Explore the performance of the Jukes-Cantor distance formula for

recovering αt on data that is produced by the Jukes-Cantor model under

varying circumstances.

Suggestions

So that the derivation of the Jukes-Cantor distance formula is valid,

pick a small value of the mutation rate, such as α = .001, to use in all

your simulations.

Use compseq to compare sequences and distJC to compute

distances.

For some ﬁxed value of t, say around 300, perform a number of

simulations for various values of N, and compute the Jukes-Cantor

distances. Compare these to αt. Do you see a pattern in how accuracy

varies with N ?

For some ﬁxed value of N , say around 400, perform a number of

simulations for various values of t, and compute the Jukes-Cantor

distances. Compare these to αt. Do you see a pattern in how accuracy

varies with t?

In comparing the computed values of d

to αt , you could consider

either d

− αt or d

/(αt). What do each of these mean?

4.5. Phylogenetic Distances 169

For a ﬁxed choice of N and t, do many simulations. Then present

your results by plotting a histogram of the values you ﬁnd for d

.Do

they appear to cluster around αt? How spread out is the histogram?

Compute means and standard deviations.

Repeat the last step, changing N or t to a new value.

What conclusions can you draw about using the Jukes-Cantor formula

for real data? Does it appear to be most accurate when sequences are

long or short? When the length of elapsed time is large or small? Can

you give an intuitive explanation of why this should be the case?

2. Investigate how the various distance formulas perform on simulated se-

quence data produced according to models different from the one under-

lying the distance formula.

This is an important issue, because real sequence evolution is at best

only approximately described by any of these models.

If, for instance, one sequence evolved from another according to the

Kimura 2-parameter model with β = γ , then we should not expect the

Jukes-Cantor distance to be a valid reconstruction of the amount of mu-

tation. However, it should be close if β and γ are close to one another.

As in the project above, the MATLAB program mutate can simulate

DNA mutations according to a speciﬁed Markov model of base substi-

tution. Then the various distances can be computed for the sequences so

produced, using compseq, distJC, distK2 and distLD.

Suggestions

Explore the performance of the Jukes-Cantor and Kimura 2-parameter

distance formulas for recovering the total amount of mutation on data

that is produced by various Kimura 2-parameter matrices. You should

keep the parameters β and γ very small, and use a large number of

time steps for mutate.Ifβ/γ = κ, how different can κ be from 1 for

the Jukes-Cantor formula to be close to correct?

Explore the performance of the Jukes-Cantor distance formula for re-

covering αt on data that is produced by a model using Jukes-Cantor

matrices, but with an initial base distribution other than the Jukes-

Cantor one. (Keep α small and use a large value of t for mutate.)

How different can the initial base distribution be before the distance

seems unreliable?

Repeat the last item for the Kimura 2-parameter model.

If data are produced according to some general Markov model, how

close to a Kimura 2-parameter matrix must the model matrix be for the

log-det distance and Kimura 2-parameter distance to be close?

170 Modeling Molecular Evolution

Even if data are produced according to the Jukes-Cantor model, do the

Kimura distances and log-det distances give the same results as the

Jukes-Cantor distance? Shouldn’t they?

Explain how you would decide which distance formula to use if you

were given two sequences.

Constructing Phylogenetic Trees

Having modeled the evolution of DNA in the last chapter, we are now ready

to use these models to make important deductions from real DNA data. We

will see how a model of molecular evolution, together with some new math-

ematical techniques, can be used to deduce evolutionary history.

Let’s consider a well-studied, yet still compelling question: What is the

relationship of humans to the modern apes? More speciﬁcally, which of the

gorilla, chimpanzee, orangutan, and gibbon are our closest evolutionary kin,

or are all these apes more closely related to each other than they are to us?

Early evolutionists viewed the chimpanzee and gorilla as our closest rela-

tives. Humans and these African apes were believed to form one evolutionary

grouping, which had split from other ape lineages in the more distant past. A

bit later, the dominant view became that all the modern apes were more closely

related to one another than to humans. Two possible diagrams that represent

more detailed versions of these competing views of hominoid evolution are

shown in Figure 5.1.



Since the chimpanzee and gorilla are African, whereas the orangutan

and gibbon are Asian, what, if anything, would each of these trees

indicate about the likely location of the appearance of the ﬁrst humans?

How can we choose which of these or the many other possible evolution-

ary trees is the best description of hominoid descent? One approach involves

Human

Chimp

Gibbon

Gorilla

Orangutan

Human

Chimp

Gibbon

Gorilla

Orangutan

Figure 5.1. Two possible hominoid phylogenies.

171

172 Constructing Phylogenetic Trees

ﬁrst choosing a particular gene that all the apes and humans share, but whose

DNA sequence shows variation from species to species. Assuming this gene is

shared and similar in all the hominoids because it arose from a common ances-

tor (i.e., the sequences are orthologous), then the variations in the sequences

among the species should contain information about their evolutionary

history.

For instance, the 898 base-pair HindIII sequences of mitochondrial DNA

from these hominoids and seven other primates have been reported in

(Hayasaka et al., 1988), which draws on work in (Anderson et al., 1981)

and (Brown et al., 1982). These sequences are in agreement at between 67%

and 97% of the sites, depending on which two are compared. (To see the

sequences for yourself, type primatedata in MATLAB, and then who to

see the names of the variables in which they are stored.)

We would like to infer a phylogenetic tree, such as one of those in Figure

5.1, showing how all the apes evolved from a common ancestor. But how does

the data indicate a “best” tree, or even a good tree, to describe the evolutionary

descent?

Of course scientists have drawn trees showing suspected evolutionary re-

lationships between species since well before the advent of DNA sequencing.

Morphological similarities between species are one source of clues as to

which trees accurately describe the descent. The identiﬁcation of common

ancestors from fossils is another. Now sequence data provides a new source

of information about evolutionary history, but using it to infer phylogenetic

trees requires the development of new mathematical tools.

5.1. Phylogenetic Trees

Before we begin developing methods to deduce phylogenetic trees, though, we

will need some terminology. Because the sequences we might want to relate

could come from different species, as in the hominoid example, or instead

from different subspecies, populations, or even individuals, we will refer to

each source of the DNA sequence as a taxon (pl. taxa). An equivalent term

in common use is operational taxonomic unit, usually abbreviated as OTU.

We hope to draw a diagram consisting of line segments that represents the

evolutionary history of the taxa. Each of the line segments in the diagram is

referred to as an edge. A diagram such as those above, in which there are no

loops formed by the edges, is called a tree.



Why is it reasonable to assume evolutionary relationships can be mod-

eled by drawing trees? What would it mean if there were a loop of

edges?

5.1. Phylogenetic Trees 173

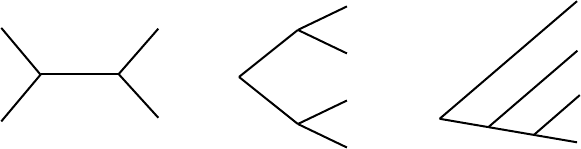
Figure 5.2. An unrooted tree (L) and two rooted versions (C,R).

Because lateral gene transfer can occur, for instance when viral DNA is

permanently incorporated into that of a host, trees cannot describe all evolu-

tionary relationships. They provide the simplest model, which is nonetheless

fully adequate for most uses.

The meeting point of several edges is called an interior vertex (pl. vertices),

while the end of an edge at a taxon is called a terminal vertex or a leaf. The

vertex where the common ancestor of all the taxa would be located is referred

to as the root.

A tree is said to be bifurcating if at each interior vertex three edges meet

and at the root two edges meet, as in the trees in Figure 5.1. Although it is

conceivable biologically that a tree other than a bifurcating one might describe

an evolutionary lineage, it is usual to ignore that possibility.



What would the evolutionary meaning be of a vertex in a tree where

four edges meet (i.e., where one edge splits into three)? Can you think

of plausible circumstances under which several species might diverge

in this way?

Although ideally every phylogenetic tree would have a root showing the

common ancestor of the taxa, sometimes we have to do without one. Some

methods of phylogenetic tree construction yield unrooted trees. For example,

Figure 5.2 shows an unrooted tree and several of the rooted trees that agree

with it. The two trees on the right could each be bent and stretched to look

like the tree on the left; only the location of the root distinguishes them.

Topological trees. A tree relating a number of taxa can actually specify

several different types of information about their relationships. First, if we

do not specify the lengths of edges, and hence only look at the branching

structure, we are considering only the topology of the tree. We consider two

trees to be topologically the same if we can bend and stretch the edges of

either one to get the other. We are not, however, allowed to cut off an edge

and reattach it elsewhere; doing that may give us a tree that is topologically

distinct from the original one.

174 Constructing Phylogenetic Trees

Tree T

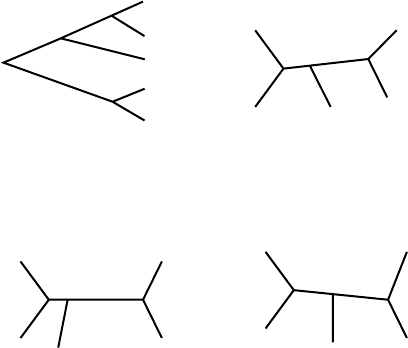
Figure 5.3. Four topological trees; as unrooted trees, all but lower right are identical.

In Figure 5.3, trees T

, T

, and T

are all topologically the same as unrooted

trees, because if any of these ﬁgures were made of rubber it could be deformed

into the other ones without either cutting or gluing pieces of it together. Tree

, on the other hand, is topologically distinct from T

, T

, and T

For rooted trees, we use a similar concept. Two rooted trees are topologi-

cally the same if one can be deformed into the other without moving the root.

Edge lengths can be changed, but not the branching structure.



In Figure 5.3, where can you place a root on T

so that it is not topo-

logically the same as T

as a rooted tree? So that it is topologically the

same as T

as a rooted tree?

A topological tree, even an unrooted one, tells us quite a lot about the

evolutionary history of the taxa it relates. For instance, all the trees in Figure

5.2 indicate that taxa A and B are related by a single split in lineage, as are C

and D. However, several bifurcations of lineage occurred during the course

of A and D evolving from a common ancestor, as two other taxa arose in the

process.

Knowing the location of the root conveys more information and may give

a better sense of the ordering of events in time. For instance, the tree on the far

right in Figure 5.2 clearly indicates the order in which bifurcations occurred:

5.1. Phylogenetic Trees 175

The common ancestor gave rise to two taxa, one of which may have evolved

further to become A. The other subsequently gave rise to B and a third taxon.

This third taxon then gave rise to both C and D.

The tree in the middle of Figure 5.2 can be interpreted similarly. The

common ancestor gave rise to two taxa, one of which gave rise to both A

and B, while the other gave rise to C and D. Note, however, that with only a

topological tree, we cannot say which of these last two bifurcations occurred

ﬁrst: Did the most recent common ancestor of A and B exist more recently

than that of C and D? We have no way to tell from this tree.

The number of different topological trees that might relate several terminal

taxa increases rapidly with the number of taxa. For instance, there is only 1

unrooted topological tree relating 3 taxa, but there are 3 unrooted topologically

distinct trees relating 4 taxa.



Draw the one unrooted topological tree that might relate terminal taxa

A, B, and C. Draw the three unrooted topological trees that might relate

terminal taxa A, B, C, and D.

For 5 terminal taxa, there are 15 such trees. Thus, ignoring the root location,

there are 13 more trees that might relate the 5 hominoids than were presented in

the chapter introduction. For 6 terminal taxa, there are more than 100 possible

unrooted trees. As the number of taxa increases, the number of trees quickly

grows to astronomical size. In the exercises, you will ﬁnd precise formulas

giving the number of unrooted and rooted trees relating n taxa. You will also

see just how large these numbers are, even for a relatively small number

of taxa. The large number of trees is unfortunate, because it means some

approaches to ﬁnding a good tree to relate taxa will be slow. If a method ﬁnds

the “best” tree by looking individually at each possible tree, then using it will

be extremely time-intensive if there are more than a handful of taxa involved.

Metric trees. In addition to a topological structure, a tree may have a

metric structure; each edge may be assigned a certain length. This metric

structure might be speciﬁed by writing numbers for the lengths next to the

edges (see Figure 5.4 (L)), or it may be merely suggested by drawing the

tree with edges of those lengths, yet not explicitly numbering them. Thus, a

topological tree and an unlabeled metric tree can be hard to tell apart. (For

clarity, in this book, we will always label edges with their lengths when the

tree is intended to be a metric one.)

Generally, the lengths of edges in a phylogenetic tree constructed from

DNA sequence data somehow represent the amount of mutation that occurred