AckermannTh. (ed) Wind Power in Power Systems

Подождите немного. Документ загружается.

//INTEGRAS/KCG/P AGIN ATION/ WILEY /WPS /FINALS_1 4-12- 04/0470855088_ 24_CHA23 .3D – 521 – [505–522/18]

17.12.2004 10:47PM

[30] WSH (Wind Service Holland) (2003), ‘WSH-Offshore wind energy’, http://home.wxs.nl/windsh/

offshore. html, accessed 10th November 2004.

[31] Zittel, W., Niebauer, P. (1988) ‘Identification of Hydrogen By-product Sources in the European Union’,

Ludwig-Bo

lkow-Systemtechnik GmbH, Ottobrunn, funded by the European Union, contract 5076-92-11

EO ISP D Amendment 1.

Wind Power in Power Systems 521

//INTEGRAS/KCG/P AGIN ATION/ WILEY /WPS /FINALS_1 4-12- 04/0470855088_ 24_CHA23 .3D – 522 – [505–522/18]

17.12.2004 10:47PM

//INTEGRAS/KCG/P AGIN ATION/ WILEY /WPS /FINALS_1 4-12- 04/0470855088_ 25_CHA24 .3D – 523 – [523–554/32]

17.12.2004 10:48PM

Part D

Dynamic Modelling

of Wind Turbines for

Power System

Studies

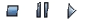
//INTEGRAS/KCG/P AGIN ATION/ WILEY /WPS /FINALS_1 4-12- 04/0470855088_ 25_CHA24 .3D – 524 – [523–554/32]

17.12.2004 10:48PM

//INTEGRAS/KCG/P AGIN ATION/ WILEY /WPS /FINALS_1 4-12- 04/0470855088_ 25_CHA24 .3D – 525 – [523–554/32]

17.12.2004 10:48PM

Introduction to the Modelling

of Wind Turbines

Hans Knudsen and Jørgen Nyga

rd Nielsen

Dedicated to our friend Vladislav Akhmatov, whose never-failing endeavour, zeal

and perseverance during his PhD project brought the world of wind turbine aero-

dynamics to us.

24.1 Introduction

This chapter presents a number of basic considerations regarding simulations for wind

turbines in electrical power systems. Though we focus on the modelling of wind

turbines, the general objective is also to look at the wind turbine as one electrotechnical

component among many others in the entire electrical power system.

The chapter starts with a brief overview of the concept of modelling and simulation

aspects. This is followed by an introduction to aerodynamic modelling of wind turbines.

We will present general elements of a generic wind turbine model and some basic

considerations associated with per unit systems, which experience shows are often

troublesome. Mechanical data will be discussed together with a set of typical mechanical

data for a contemporary sized wind turbine. We will give an example of how to convert

these physical data into per unit data. These per unit data are representative for a wide

range of sizes of wind turbine and are therefore suitable for user applications in a

number of electrical simulation programs. Finally, various types of simulation phenom-

ena in the electrical power system are discussed, with special emphasis on what to

consider in the different types of simulation.

Wind Power in Power Systems Edited by T. Ackermann

Ó 2005 John Wiley & Sons, Ltd ISBN: 0-470-85508-8 (HB)

//INTEGRAS/KCG/P AGIN ATION/ WILEY /WPS /FINALS_1 4-12- 04/0470855088_ 25_CHA24 .3D – 526 – [523–554/32]

17.12.2004 10:48PM

We will not address the issues related to model implementation in various simulation

programs except for some specific properties related to dynamic stability compared with

full transient generator models.

24.2 Basic Considerations regarding Modelling and Simulations

Computer simulation is a very valuable tool in many different contexts. It makes it

possible to investigate a multitude of properties in the design and construction phase as

well as in the application phase. For wind turbines (as well as other kinds of complex

technical constructions), the time and costs of development can be reduced consider-

ably, and prototype wind turbines can be tested without exposing physical prototype

wind turbines to the influence of destructive full-scale tests, for instance. Thus, com-

puter simulations are a very cost-effective way to perform very thorough investigations

before a prototype is exposed to real, full-scale, tests.

However, the quality of a computer simulation can only be as good as the quality of the

built-in models and of the applied data. Therefore it is strongly recommended to define

clearly the purpose of the computer simulations in order to make sure that the model and

data quality are sufficiently high for the problem in question and that the simulations will

provide adequate results. Otherwise, the results may be insufficient and unreliable.

Unless these questions are carefully considered there will be an inherent risk that

possible insufficiencies and inaccuracies will not be discovered – or will be discovered

too late – and subsequent important decisions may be made on a faulty or insufficient

basis. Hence, computer simulations require a very responsible approach.

Computer simulations can be used to study many different phenomena. The require-

ments that a specific simulation program has to meet, the necessa ry level of modelling

detail and requirements regarding the model data may differ significantly and depend on

the objective of the investigation. Different parts of the system may be of varying

importance, depending on the objective of the investigation, too. All this should be

taken into consideration before starting the actual computer simulation work.

However, in order to take into account all this, it is necessa ry to have a general

understanding of the wind turbine (or of any other system on which one wants to

perform a computer simulation) and how the various parts of the wind turbine can be

represented in a computer model. This basic overview will be provided in the subsequent

sections of this chapter. After that, the different types of simulations and various

requirements regarding accuracy will be discussed in more detail.

24.3 Overview of Aerodynamic Modelling

The modelling of different types of generators, converters, mechanical shaft systems and

control systems is all well-documented in the literature. In the case of wind turbines it is

therefore primarily the aerodynamic system that may be unfamiliar to those who work

with electrotechnical simulation programs. For that reason, an introduction to the basic

physics of the turbine rotor and the various ways in which the turbine rotor is commonly

represented will be outlined. For more details, see, for example, Akhmatov (2003),

where the subject is treated in great detail and where a comprehensive list of references

526 The Modelling of Wind Turbines

//INTEGRAS/KCG/P AGIN ATION/ WILEY /WPS /FINALS_1 4-12- 04/0470855088_ 25_CHA24 .3D – 527 – [523–554/32]

17.12.2004 10:48PM

regarding wind power in general is included. Some of the most recommendable refer-

ences in this context are Aagaard Madsen (1991), Freris (1990), Hansen (2000), Heier

(1996), Hinrichsen (1984), Johansen (1999), Øye (1986), Snel and Lindenburg (1990),

Snel and Schepers (1995), Sørensen an d Koch (1995), and Walker and Jenkins (1997).

24.3.1 Basic description of the turbine rotor

From a physical point of view, the static characteristics of a wind turbine rotor can be

described by the relationships between the total power in the wind and the mechanical

power of the wind turbine. These relationships are readily described starting wi th the

incoming wind in the rotor swept area. It can be shown that the kinetic energy of a

cylinder of air of radius R travelling with wind speed V

WIND

corresponds to a total wind

power P

WIND

within the rotor swept area of the wind turbine. This power, P

WIND

, can

be expressed by:

WIND



AIR

 R

WIND

; ð24:1Þ

where 

AIR

is the air density (¼1.225 kg/m

), R is the rotor radius and V

WIND

is the wind

speed.

It is not possible to extract all the kinetic energy of the wind, since this would mean

that the air would stand still directly behind the wind turbine. This would not allow the

air to flow away from the wind turbine, and clearly this cannot represent a physical

steady-state condition. The wind speed is only reduced by the wind turbine, which thus

extracts a fraction of the power in the wind. This fraction is denominated the power

efficiency coefficient, C

, of the wind turbine. The mechanical power, P

MECH

, of the

wind turbine is therefore – by the definition of C

– given by the total power in the wind

WIND

using the following equation:

MECH

¼ C

WIND

: ð 24 :2Þ

It can be shown that the theoretical static upper limit of C

is 16/27 (approximately

0.593); that is, it is theoretically possible to extract approximately 59% of the kinetic

energy of the wind. This is known as Betz’s limit. For a comparison, modern three-

bladed wind turbines have an optimal C

value in the range of 0.52–0.55 when measured

at the hub of the turbine.

In some cases, C

is specified with respect to the electrical power at the generator

terminals rather than regarding the mechanical power at the turbine hub; that is, the

losses in the gear and the generator are deducted from the C

value. When specified in

this way, modern three-bladed wind turbines have an optimal C

value in the range of

0.46–0.48. It is therefore necessary to unde rstand whether C

values are specified as a

mechanical or as an electrical power efficiency coefficient.

If the torque T

MECH

is to be applied instea d of the power P

MECH

, it is conveniently

calculated from the power P

MECH

by using the turbine rotational speed !

turb

MECH

turb

: ð24:3Þ

Wind Power in Power Systems 527

//INTEGRAS/KCG/P AGIN ATION/ WILEY /WPS /FINALS_1 4-12- 04/0470855088_ 25_CHA24 .3D – 528 – [523–554/32]

17.12.2004 10:48PM

It is clear from a physical point of view that the power, P

MECH

, that is extracted from

the wind will depend on rotational speed, wind speed an d blade angle, . Therefore,

MECH

and hence also C

must be expected to be functions of these quantities.

MECH

¼ f

MECH

ð!

turb

; V

wind

;Þ: ð24:4Þ

Now, the forces of the wind on a blade section – and thereby the possible energy

extraction – will depend on the angle of incidence ’ between the plane of the moving rotor

blades and the relative wind speed V

rel

(see Figure 24.1) as seen from the moving blades.

Simple geometrical considerations, which ignore the wind turbulence created by the

blade tip (i.e. a so-called two-dimensional aerodynamic representation) show that the

angle of incidence ’ is determined by the incoming wind speed V

WIND

and the speed of

the blade. The blade tip is moving with speed V

tip

, equal to !

turb

R. This is illustrated in

Figure 24.1. Another commonly used term in the aerodynamics of wind turbines is the

tip-speed ratio, , which is define d by:

 ¼

turb

WIND

: ð24:5Þ

The highest values of C

are typic ally obtained for  values in the range around 8 to 9

(i.e. when the tip of the blades moves 8 to 9 times faster than the incoming wind). This

means that the angle between the relative air speed – as seen from the blade tip – and the

rotor plane is rather a sharp angle. Therefore, the angle of incide nce ’ is most con-

veniently calculated as:

’ ¼ arctan





¼ arctan

WIND

turb



: ð24:6Þ

It may be noted that the angle of incidence ’ is defined at the tip of the blades, and that

the local angle will vary along the length of the blade, from the hub (r ¼0) to the blade

tip (r ¼R) and, therefore, the local value of ’ will depend on the position along the

length of the blade.

On modern wind turbines, it is possible to adjust the pitch angle  of the entire blade

through a servo mechanism. If the blade is turned, the angle of attack  between the

blade and the relative wind V

rel

will be changed accordingly. Again, it is clear from a

tip

=–ω

turb

× R

rel

tip

WIND

Figure 24.1 Illustration of wind conditions around the moving blade. Note: V

tip

¼tip speed;

turb

¼turbine rotational speed; R ¼rotor radius; V

rel

¼relative wind speed; V

WIND

¼wind speed;

 ¼angle of attach; ’ ¼angle of incidence between the plane of the rotor and V

rel

;  ¼blade angle

528 The Modelling of Wind Turbines

//INTEGRAS/KCG/P AGIN ATION/ WILEY /WPS /FINALS_1 4-12- 04/0470855088_ 25_CHA24 .3D – 529 – [523–554/32]

17.12.2004 10:48PM

physical perspective that the forces of the relative wind on the blade, and thereby also

the energy extraction, will depend on the angle of attack  between the moving rotor

blades and the relative wind speed V

rel

as seen from the moving blades.

It follows from this that C

can be expressed as a function of  and :

¼ f

ð; Þ: ð24:7Þ

is a highly nonlinear power function of  and . It should be noted that one main

advantage of an approach including C

,  and  is that these quantities are normalised

and thus comparable, no matter what the size of the wind turbine.

On older and simpler wind turbines, the blades have a fixed angular pos ition on the

hub of the wind turbine, which means the blade angle  is constant (

const

). This is called

stall (or passive stall) control, because the turbine blades will stall at high wind speeds

and thus automatically reduce the lift on the turbine blades. With a fixed pitch angle of

the blades, the relation between the power efficiency coefficient C

(, 

const

) and the

tip-speed ratio, , will give a curve similar in shape to the one shown in Figure 24.2(a).

Assuming a constant wind speed, V

WIND

, the tip-speed ratio, , will vary proportion-

ally to the rotational speed of the wind turbine rotor. Now, if the C

–  curve is known

for a specific wind turbine with a turbine rotor radius R it is easy to construct the curve

of C

against rotational speed for any wind speed, V

WIND

. The curves of C

against

rotational speed will be of identical shape for different wind speeds but will vary in terms

of the ‘stretch’ along the rotational speed axis, as illustrated in Figure 24.2(b). There-

fore, the optimal operational point of the wind turbine at a given wind speed V

WIND

is,

as indicated in Figure 24.2(a), determined by tracking the rotor speed to the point 

opt

The optimal turbine rotor speed !

turb, opt

is then found by rewriting Equation (24.5) as

follows:

turb; opt



opt

WIND

: ð24:8Þ

The optimal rotor speed at a given wind speed can also be foun d from Figure 24.2(b).

Observe that the optimal rotational speed for a specific wind speed also depends on the

turbine radius, R, which increases with the rated power of the turbine. Therefore, the

larger the rated power of the wind turbine the lower the optimal rotational speed.

These basic aerodynamic equations of wind turbines provide an understanding that

fixed-speed wind turbines have to be designed in order for the rotational speed to match

the most likely wind speed in the area of installation. At all other wind speeds, it will not

be possible for a fixed-speed wind turbi ne to maintain operation with optimised power

efficiency.

In the case of variable-speed wind turbines, the rotational speed of the wind turbine is

adjusted over a wide range of wind speeds so that the tip-speed ratio  is maintained at



opt

. Thereby, the power efficiency coefficient C

reaches its maximum and, conse-

quently, the mechanical power output of a variable-speed wind turbine will be higher

than that of a similar fixed-speed wind turbine over a wider range of wind speeds. At

higher wind speeds, the mechanical power is kept at the rated level of the wind turbine

by pitching the turbine blades.

Wind Power in Power Systems 529

//INTEGRAS/KCG/P AGIN ATION/ WILEY /WPS /FINALS_1 4-12- 04/0470855088_ 25_CHA24 .3D – 530 – [523–554/32]

17.12.2004 10:48PM

opt

(λ, β

const

)

(a)

(b)

0 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30

4 m/s

5 m/s

6 m/s

7 m/s

8 m/s

9 m/s

10 m/s

11 m/s

12 m/s

13 m/s

14 m/s

15 m/s

16 m/s

Rotational speed (rpm)

Power efficiency

0.60

0.55

0.50

0.45

0.40

0.35

0.30

0.25

0.20

0.15

0.10

0.05

– 0.05

–0.10

–0.15

–0.20

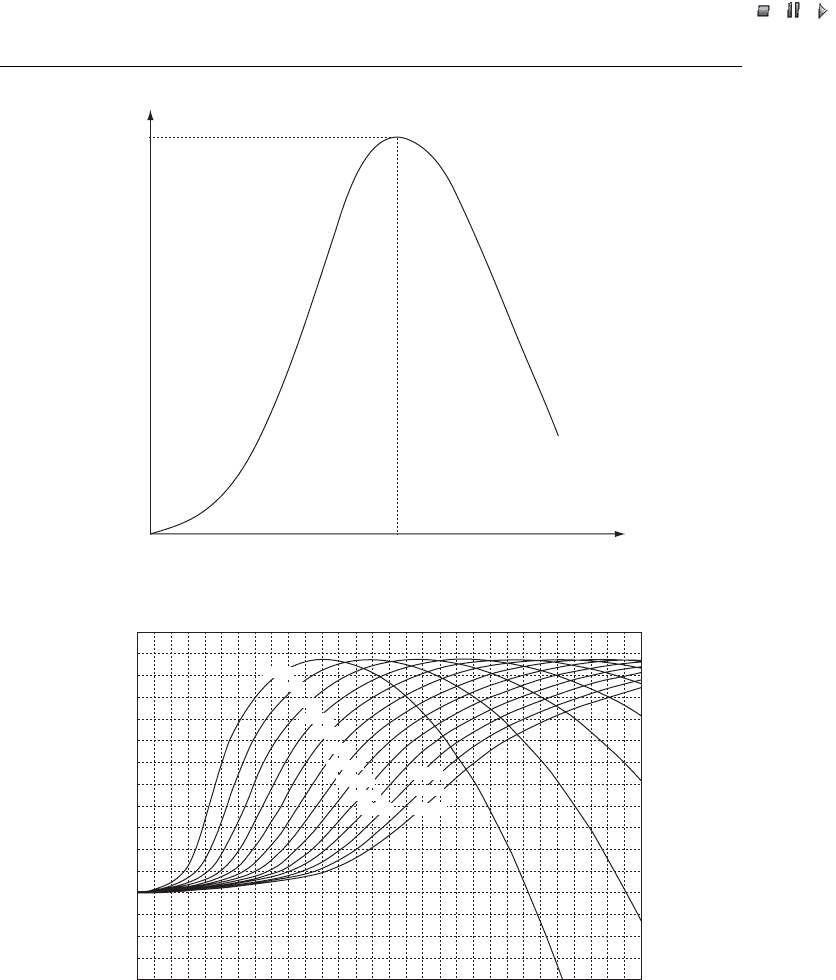
A variable speed 2 MW wind speed-aerodynamics

Figure 24.2 Power efficiency coefficient, C

, for a fixed blade angle (a) C

as a function of tip-

speed ratio, , and (b) C

as a function of rotational speed for various wind speeds (4–16 M/S).

Reproduced from Wind Engineering, volume 26, issue 2, V. Akhmatov, ‘Variable Speed Wind

Turbines with Doubly-fed Induction generators, Part I: Modelling in Dynamic Simulation Tools’,

pp. 85–107, 2002, by permission of Multi-Science Publishing Co. Ltd

530 The Modelling of Wind Turbines