Accardi L., Freudenberg W., Obya M. (Eds.) Quantum Bio-informatics IV: From Quantum Information to Bio-informatics

230

The

relation (23)

can

be

regarded

as

the

quantum

analogue

of

classi-

cal Bayesian relation.

In

the

extend

classical information

theory

to

quantum

system

on

the

base

of

the

quantum

Bayesian re

lation

(23).

5.

Mutual

entropy

and

conditional

entropy

vs.

quantum

entanglement

In classical description of a physical

compound

system

its correlation

can

be

represented by a

joint

probability measure

or

a conditional

probability

measure. In classical information

theory

we have

proper

criteria

to

estimate

such correlation, which

are

so-called

the

mutual

entropy

and

the

conditional

entropy

given by Shannon.

The

mutual

entropy

is given as

the

relative

entropy

of

correlated joint probability

and

non-correlated one.

This

means

that

it

represents

the

correlation included in

the

joint

probability

Tij

as

the

distance from

the

non

correlated

product

joint

probability

Piqj,

where

Pi

and

qj

are

marginal probabilities, in

the

sense

of

relative entropy. In

other

words,

the

mutual

e

ntropy

means

the

common

information included

in marginal

random

variables X

and

Y.

On

the

other

hand,

the

conditional

entropy

is given by

the

average

of

the

entropy

of

conditional probability

which means

the

uncertainty

still remaining in X (resp. Y)

after

observing

Y (resp.

X).

We

extend

the

classical entropies

to

a

quantum

system.

Definition

5.1.

Let ¢

be

an

entanglement

mapping

with

p = ¢

(1)

and

0"

= ¢* (1).

One

defines

4

,5

1¢(p,

0")

==

8(8¢,

p®

O")

= Tr8 ¢ (log 8 ¢ - log p ®

0")

(24)

where

8 (',

.)

is

the

Araki-Umegaki relative entropy. One calls 1¢

(p,

0")

the

quantum

mutual

entropy. 7,

10.

The

quantum

mutual

e

ntropy

can

be

computed

as follows:

1¢

(p,

0")

= Tr8¢ (log8¢

-logp

®

0")

= Tr8 ¢ (log 8 ¢ - log p ® 1 - log 1 ®

0")

=

-8

(8¢) -

Tr

2

8¢ log p ® 1 -

Tr

1

8¢ log 1 ®

0"

=8(p)+8(0")-8(8

¢)

.

(25)

231

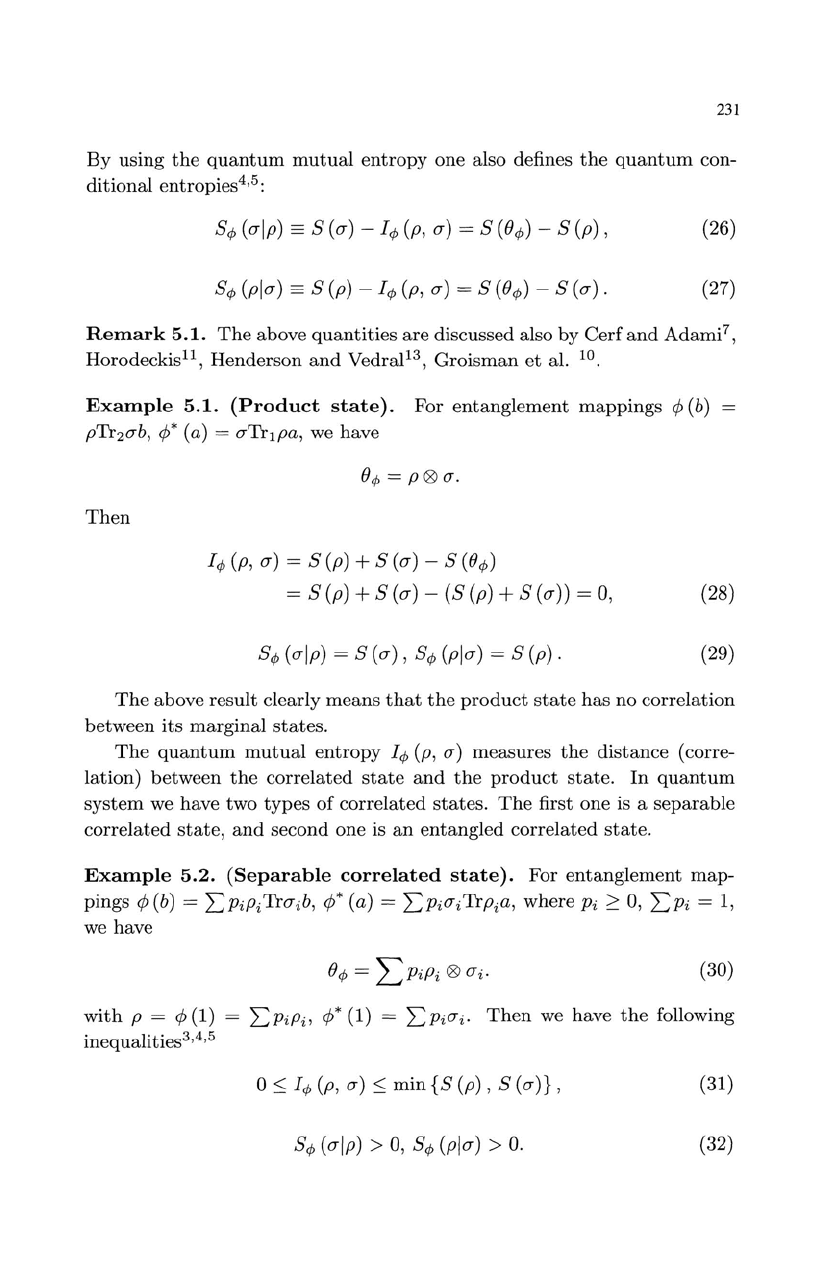
By

using

the

quantum

mutual

entropy one also defines

the

quantum

con-

ditional entropies

4

,5:

S</>

(alp)

==

S (a) -

I</>

(p,

a) = S

(8</»

- S

(p)

, (26)

S</>

(pia)

==

S

(p)

-

I</>

(p,

a) = S

(8</»

- S (a) .

(27)

Remark

5.1.

The

above quantities are discussed also

by

Cerf

and

Adami

7

,

Horodeckisll, Henderson

and

Vedral

13

, Groisman

et

al.

10.

Example

5.1.

(Product

state).

For entanglement mappings ¢

(b)

pTr2ab, ¢* (a) = aTr1pa, we have

8</>

= p 0 a.

Then

I

</>

(p

, a) = S

(p)

+ S (a) - S

(8</»

=S(p)+S(a)-(S(p)+S(a))=O,

(28)

S

</>

(alp) = S (a) , S

</>

(pia) = S

(p)

.

(29)

The

above result clearly means

that

the

product

state

has no correlation

between

its

marginal

stat

e

s.

The

quantum

mutual

e

ntropy

I</>

(p,

a) measures

the

distance (corre-

lation)

betw

een

the

corre

lated

state

and

the

product

state.

In

quantum

system we have two types of correlated states.

The

first one is a separable

correlated

state,

and

second one is

an

entangled correlated

state.

Example

5.2.

(Separable

correlated

state).

For entanglement map-

pings

¢

(b)

=

'2:.

Pi

Tra

ib, ¢* (a) = '2:.PWiTrpia, where

Pi

~

0,

'2:.P

i = 1,

we have

(30)

with

p = ¢ (1) =

'2:.PiP

i' ¢* (1) = '2:.PWi.

Then

we have

the

following

inequalities

3

,4,5

0::;

I</>

(p,

a)

::;

min {S

(p)

, S (a)} ,

(31)

S

</>

(alp) >

0,

S

</>

(p

ia) >

0.

(32)

232

Example

5.3.

(Separable

perfect

correlated

state).

For entangle-

ment

mappings

¢(b)

=

LPi

lei) (eil

(fi,bi

i

),

¢* (a) =

LPi

1M

(fil (ei,aei)

we

have

8¢

=

I>i

lei) (eil 0 I!i) (fil

with

p = ¢

(1)

=

LPi

lei) (eil,

(J

= ¢*

(1)

=

LPi

1M

(fil.

Then.

1¢

(p,

(J)

= 5

(p)

+ 5

((J)

- 5 (8¢) = 5 (p),

(33)

where 5

(p)

= 5

((J)

= 5 (8¢) = -

LPi

logpi.

This

correlation corresponds

to

a perfect correlation in

the

classical scheme.

Example

5.4.

(Pure

entangled

correlated

state).

For en-

tanglement

mappings

¢(b)

LAi"Xj

lei) (ejl

(fj,bM,

¢* (a)

- 2

LAiAj

1M

(fJl

(ej,aei)

, where

Ai

E C, L

IAil

=

1,

we have

8¢

= L

Ai"Xj

lei) (ej I 0 Iii) (fJ I =

IW)

(WI,

where

IW)

= L Aiei 0 k

and

its marginal

states

are

given by p = ¢

(1)

=

L I

A

il

2

l

e

i) (eil,

(J

=

¢*

(1)

= L I

A

il

2

lii)

(fil.

Then

1¢

(p,

(J)

= 5

(p)

+ 5

((J)

- 5 (8¢)

=

25

(p),

5¢

((Jlp)

= 5¢

(pl(J)

=

-5

(p)

,

where 5

(p)

= 5

((J)

= - L IAil2log

IAil2

.

(35)

In

classical

system

the

mutual

entropy

is always smaller

than

its

mar-

ginal entropies,

and

the

conditional

entropy

is always positive. So

that

the

correlation

of

pure

entangled

state

has

a non-classical property. We

introduce

another

criterion

to

measure

the

correlation of

compound

states.

Definition

5.2. For

an

entanglement

mapping

¢

with

p = ¢ (1),

(J

=

¢* (1), we defines

1

D¢

(pp)

==

1¢

(p,

(J)

-

"2

(5

(p)

+ 5

((J))

1

=

"2

(5

(p)

+ 5

((J))

- 5

(8

¢ )

(36)

(37)

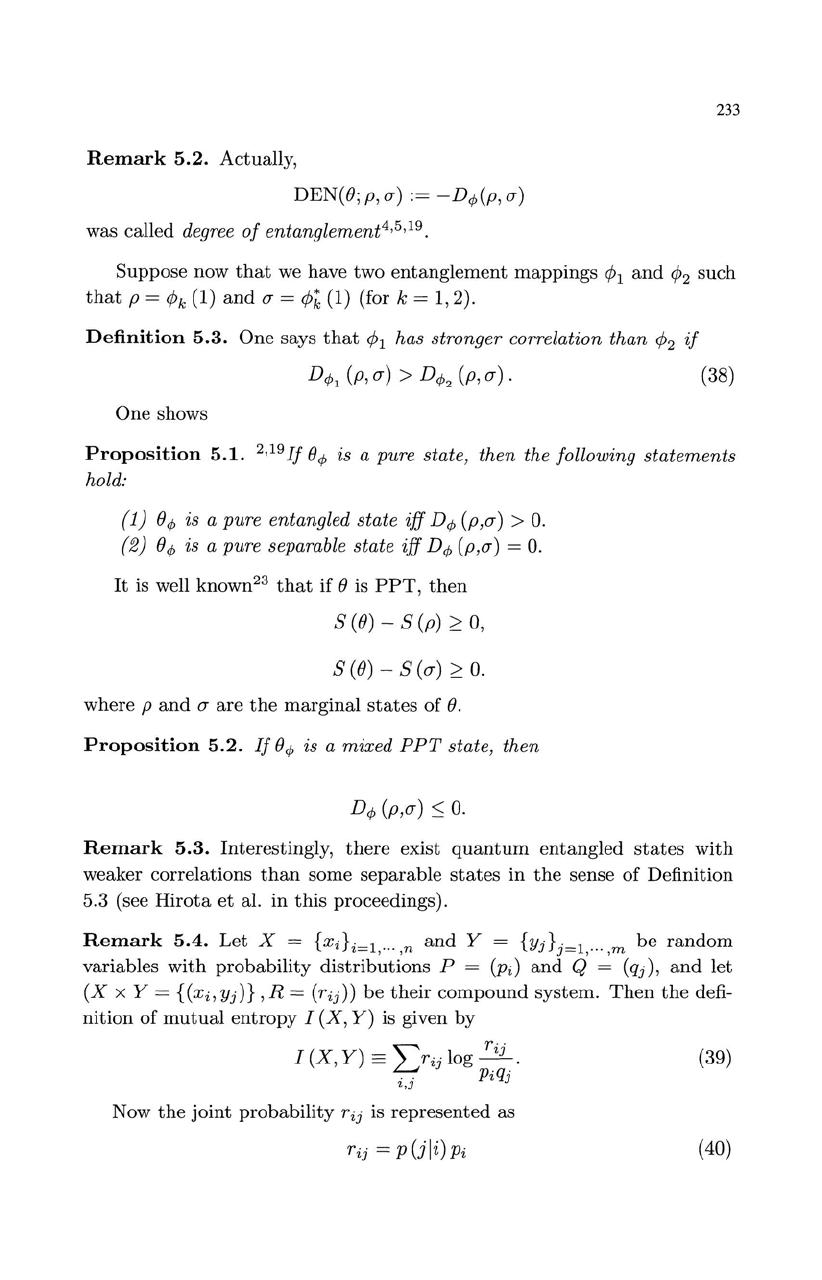
Remark

5.2.

Actually,

DEN(B;

p,

a)

:=

-Dq,(p, a)

was called degree

of

entanglement

4

,5,19.

233

Suppose now

that

we have two

entanglement

mappings

rP1

and

rP2

such

that

p =

rPk

(1)

and

a =

rP~

(1) (for k =

1,2).

Definition

5.3.

One says

that

rP1

has stronger correlation

than

rP2

if

Dq"

(p,a) >

Dq,2

(p,a).

(38)

One

shows

Proposition

5.1.

2,19

If

Bq,

is a pure state,

then

the following

statements

hold:

(1)

Bq,

is a pure entangled state

iff

Dq,

(p,a) >

O.

(2)

Bq,

is a pure separable state

iff

Dq,

(p,a) =

O.

It

is well known

23

that

if B is

PPT,

then

S(B) -

S(p)

~

0,

S(B) -

S(a)

~

O.

where p

and

a are

the

marginal

states

of

B.

Proposition

5.2.

If

Bq,

is a

mixed

PPT

state,

then

Remark

5.3.

Interestingly,

there

exist

quantum

entangled

states

with

weaker correlations

than

some separable

states

in

the

sense

of

Definition

5.3 (see

Hirota

et

al. in

this

proceedings).

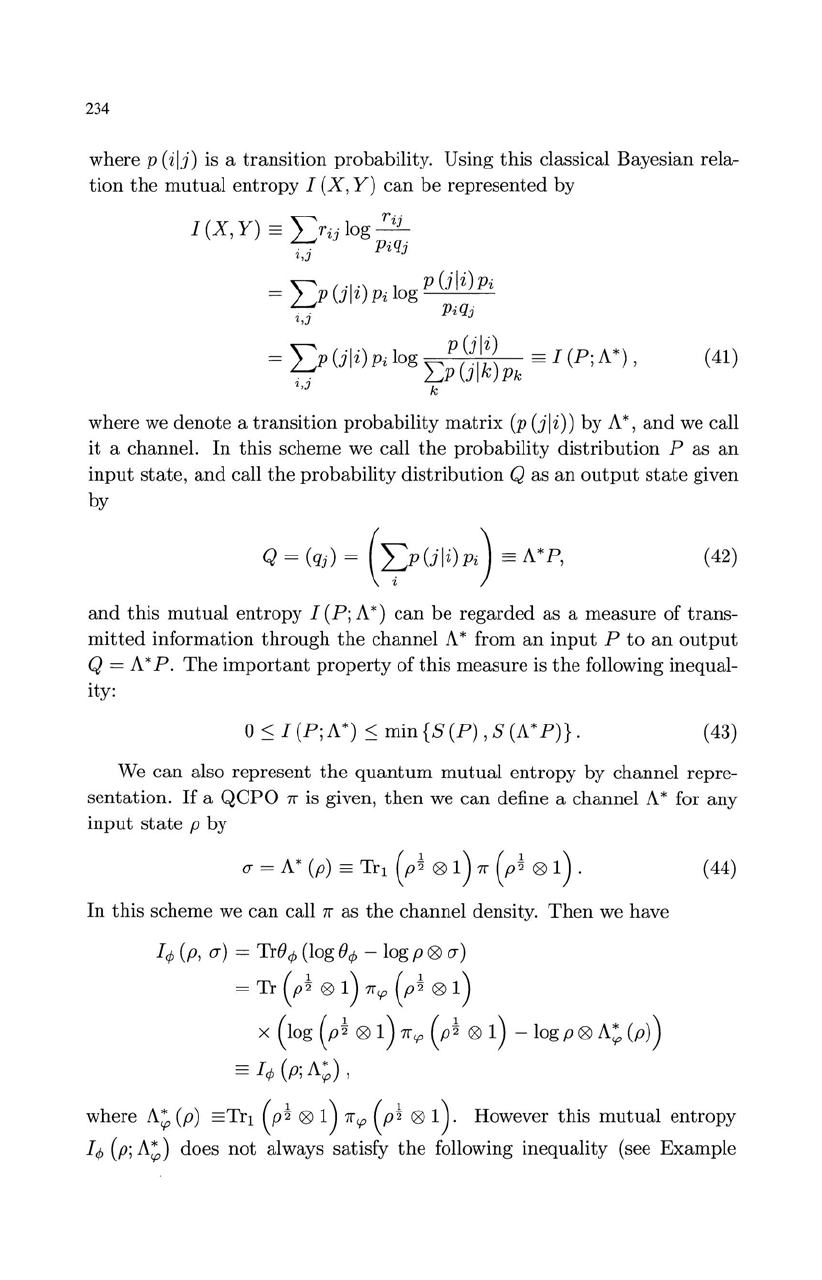
Remark

5.4.

Let

X = {Xi}i=l,.

..

,n

and

Y = {Yj}j=l,

...

,m

be

random

variables

with

probability

distributions

P = (Pi)

and

Q = (qj),

and

let

(X

x Y = {(Xi, Yj)} , R =

(r

ij))

be

their

compound

system.

Then

the

defi-

nition

of

mutual

entropy

I (X,

Y)

is given by

Now

the

joint

probability rij is represented as

rij

=p(jli)Pi

(39)

(40)

234

where P (ilJ) is a

transition

probability, Using

this

classical Bayesian rela-

tion

the

mutual

entropy

I

(X,

Y)

can

be

represented

by

r·

.

I

(X,

Y)

=

Lrij

log

---2.L

· . Piqj

',J

'"

('I

') 1

p(jli)pi

=

~P

J Z

Pi

og

· . Piqj

',J

'"

( 'I·) 1

p(jli)

I(P

A*)

=

~P

J Z

Pi

og

'"

('Ik)

= ; ,

· .

~P

J

Pk

' ,J k

(

41)

where

we

denote

a

transition

probability

matrix

(p

(j Ii)) by A

*,

and

we call

it

a channel.

In

this

scheme we call

the

probability

distribution

P

as

an

input

state

,

and

call

the

probability

distribution

Q

as

an

output

state

given

by

( 42)

and

this

mutual

entropy

I (P; A *)

can

be

regarded

as

a

measure

of

trans-

mitted

information

through

the

channel A * from

an

input

P

to

an

output

Q = A *

P,

The important

property

of

this

measure

is

the

following inequal-

ity:

0::;

I(P;A*)::;

min{S(P)

,

S(A*P)},

(43)

We

can

also

represent

the

quantum

mutual

entropy

by

channel repre-

sentation,

If

a

QCPO

7f is given,

then

we

can

define a channel A * for

any

input

state

p

by

In

this

scheme we

can

call 7f as

the

channel density,

Th

en

we

have

I

4>

(p,

0')

=

Tre4>

(log

e4>

-l

og p 0

0')

= Tr

(p~

0

1)

7f<p

(p~

0

1)

x

(log

(p

~

0

1)

7f

<p

(p

~

0

1)

- log p 0

A~

(p)

)

=

I4>

(p;

A~)

,

(44)

where

A~

(p)

=Tr

1

(p

~

0

1)

7f

<p

(p

~

0

1)

, However

this

mutual

entropy

I

4>

(p;

A~)

does

not

always satisfy

the

following inequality (see

Example

235

5.4):

o

::;

I

q,

(p;

A~)

::;

min

{ S

(p)

, S

(A~

(p))) . ( 45)

From

this

point

of

view

the

quantum

mutual

entropy

1q,

(p,

0')

is

not

a

proper

measure

of

transmitted

information

through

the

channel. Hence, a

further

study

of

this

problem

is needed.

6.

Summary

In

this

paper

we

showed

that

the

quantum

Bayesian

relation

holds

between

the

compound

state

given

by

means

of

a CCP

map

(an

entanglement

map-

ping)

and

the

quantum

conditional

probability

operator

i.e.

QCPO

given

by

means

of a

CP

map.

We discussed

the

role

of

quantum

mutual

entropy,

conditional

entropy

and

its

relation

with

quantum

entanglement.

References

1.

M. Asorey, A. Kossakowski, G.

Marmo,

E.C.G.

Sudarshan,

"Relation

be-

tween

quantum

maps

and

quantum

state",

Open.

Syst.

Info.

Dyn.

12,

319-

329

(2006).

2.

L. Accardi,

T.

Matsuoka,

M.

Ohya,

"Entangled

Markov

chaines

are

indeed

entangled",

Infin. Dim.

Anal.

Quantum

Probab.

Top. 9, 379-390 (2006).

3. L.Accardi,

T.

Matsuoka,

M.

Ohya,

"Entangled

Markov

chain

satisfying

en-

tanglement

condition",

RIMS

1658,

84-94 (2009)

4. V. P. Belavkin, M.

Ohya,

"Quantum

entropy

and

information

in

discrete

entangled

state",

Infin. Dim. Anal.

Quantum

Probab.

Top.

4,

33-59 (2001).

5. V. P. Belavkin, M.

Ohya,

"Entanglement,

quantum

entropy

and

mutual

in-

formation",

Proc.

R. Soc.

London

A

458,

209-231 (2002).

6. V. P. Belavkin, X. Dai,

"An

operational

algebraic

approach

to

quantum

channel

capacity",

Int.

J.

Quantum

Inf. 6, 981 (2008).

7.

N. J.

Cerf

and

C.

Adami,

"Negative

entropy

and

information

in

quantum

mechanics",

Phys.

Rev.

Lett.

79,

5194-5197 (1997).

8. M. D.

Choi,

"Completely

positive

maps

on

complex

matrix",

Lin. Alg. Appl.,

10,

285 (1975).

9.

D. Chrusciiiski, Y.

Hirota,

T.

Matsuoka

and

M.

Ohya,

"Remarks

on

the

degree

of

entanglement"

in

this

proceedings.

10. B.

Groisman,

S.

Popescu

and

A.

Winter,

"Quantum,

classical,

and

total

amount

of

correlations

in a

quantum

state"

Phys.

Rev

A

72,

0323187 (2005).

11. M. Horodecki

and

R.

Horodecki,

"Information-theoretical

aspect

of

quantum

inseparability

of

mixed

states",

Phys.

Rev. A

54,1838-1843

(1996).

12. M. Horodecki, P. Horodecki

and

R. Horodecki, Phy. Lett., A

223,

1 (1996).

13.

Henderson

and

V. Vedral, "Classical,

quantum

and

total

correlation",

J.

Phys.

A

34

6913 (2001)

236

14. A. Jamiolkowski,

"Linear

transformation

which

pres

erve

trace

and

positive

semidefiniteness

of

oprators",

Rep.

Math

.

Phys.

3, 275 (1072).

15. A. Jamiolkowski,

T.

Matsuoka

and

M.

Ohya

,

"Entangling

operator

and

PPT

condition",

TUS

preprint

(2007).

16. G.

Kimura,

A. Kossakowski,

"A

note

on

positive

maps

and

classification

on

states",

Open

Sys. &

Information

Dyn

.

12,

1 (2005).

17.

T.

Matsuoka,

"On

generalized

entangl

e

ment"

,

QP-

PQ

Quantum

Probab.

&

White

Noise Anal.

21

, 170-180 (2007).

18.

W.

A. Majewski,

T.

Matsuoka

and

M.

Ohya

,

"Characterization

of

partial

positive

transposition

states

and

measures

of

entanglement",

J.

Math.

Phys.

50,

113509 (2009).

19.

T.

Matsuoka

, M.

Ohya

,

"Quantum

entangled

state

and

its

characterization",

Foud.

Probab.

Phys

. 3

750

, 298-306 (2005).

20.

M.

Ohy

a,

1.

V. Volovich, Mathematical Foundation

of

Quantum

Information

and

Computation

(

to

be

pabulished

by Springer, New Youk)

21. A. Peres, Phys.Rev.Lett.,

77,

1413 (1996).

22. K.

Urbanik

,

"Joint

probability

distribution

of

observables

in

quantum

me-

chanics",

Stud.

Math.

T.

21,317-323

(1961).

23.

K.G

.H. Vollbrecht, M.M. Wolf,

"Conditional

entropies

and

their

relation

to

entangl

e

ment

criteria"

,

e-print

arXiv

:

quant-ph/0202058v1.

24.

S.

L. Woronowicz, "Positive

maps

of low dimensional

matrix

algebra",

Rep.

Math.

Phys.,

10, 165 (1976)

Quantum

Bio-Informatics

IV

eds.

1.

Accardi,

W.

Freudenberg

and

M.

Ohya

World

Scientific

Publishing

Co.

(pp.

237- 254)

CONSTRUCTING

MULTIPARTITE

ENTANGLEMENT

WITNESSES

MHOSZ MICHALSKI

Institute

of

Physics, Nicolaus Copernicus University

Grudzir;,dzka

5,

87-100 Torun, Poland

E-mail

: milosz@Jizyka.umk.pl

The

method

of

transforming

a

hamiltonian

of

a

composite

quantum

system

into

an

entang

l

ement

witness

,

explored

in

an

ea

rlier

paper

l

,

is

extended

to

the

multipartite

case.

The

witness

can

be

made

not

only

to

detect

genera

l

entang

l

ement

but

also

to

discriminate

among

its

various

multipartite

types.

Partial

knowledge

of

the

lo

west

part

of

energy

spectrum

is suffici

ent

for

approximate

witness

construction.

As

an

examp

le, we

carry

out

analytic

calculations

with

the

hamiltonian

of

a

I-dim

ensiona

l

Heisenberg

XXZ

model.

Keywords:

Multipartite

entanglement,

entang

lement

witness,

quantum

spin

chains,

Heisenberg

models

1.

Introduction

Multipartite

entanglement

is a generic

property

of

quantum

states

of

in-

teracting

many-body

systems. However, already

the

simplest examples in-

dicate

that

it

cannot

be

understood

and

described solely

in

terms

of

more

elementary

bipartite

entanglement.

In

particular,

as counterintuitive as

it

may

seem,

in

a

system

of

3

qubits

there

are

pur

e

states

which

are

separable

against

any

bipartite

split, yet

they

are

not

fully separable

2

,

4.

It

means

that

there

exists genuinely

3-partite

entanglement

which is

not

the

sum

of

any

bipartite

constituents. Moreover,

there

are inequivalent

brands

of

3-partite

entanglement

5

,

as exemplified, for

instance

, by

the

famous IW)

and

IGHZ

)

states,

IW) =

~

(1100)

+

1010)

+

1001))

,

I G H

Z)

=

~

(1

000)

+ 1111)). (1)

Namely,

it

is easy

to

verify

that

tracing

out

one

of

the

qubits

reduces

the

GHZ

state

to

a

separable

mixture, while

the

W

state

is more

robust

-

the

resulting 2-qubit

state

remains entangled. Already this distinction suggests

237

238

that

there

is no

straightforward

generalization

of

the

Schmidt

decomposi-

tion

for

the

3-partite

case,

Indeed,

any

such

I'lj!),

and

IGHZ)

in

particular,

becomes a separable mix-

ture

under

one-party

reduction

, while

the

W

state

does

not

- hence

it

cannot

be

cast

in such a "Schmidt form" by a choice

of

local bases.

This

picture

unfolds into

an

even more complex one

with

the

increase of

the

number

of

subsystems.

Distinguishing

entangled

and

separable

states

of

bipartite

systems re-

mains

to

be

one

of

the

most

challenging

open

problems

in

quantum

infor-

mation

theory.

The

discrimination

among

various genres of

multipartite

entanglement

appears

a still

harder

task.

In

experiments, Bell inequalities

are

among

commonly used tools

to

detect

entanglement

both

in bi-

and

multipartite

settings. Yet Bell inequalities prove

to

be

a faulty

detector

in

general: on

the

one

hand

, even

in

the

bipartite

case,

there

are

entan-

gled

states

not

violating

the

inequalities,

on

the

other

hand

the

degree

of

violation

of

Bell inequalities is

not

sufficiently "monotone" when going

from

"weaker" entangled biseparable

states

to

fully entangled

multipartite

ones

6

,

3.

Consequently,

it

is

not

an

adequate

tool for

the

classification of

various

multipartite

entanglement

patt

erns.

An

alternative

technique, recognized

to

be

quite

fruitful

both

theoretically as well as experimentally, is

the

use

of

entanglement

witnesses

1

,5,6,7,8,9,1O.

While originally7

,9,

entanglement

witnesses were de-

fined

to

distinguish

bipartite

entanglement

from

sep

arability,

they

proved

to

be

equally useful for

the

discrimination

among

various

types

of

multi-

partite

entanglement

ll

,5,6,

or

even as simple

entanglement

measures

a

.

Ac-

tually,

th

ere is a

number

of

interesting

relations between specific witnesses

and

standard

entanglement

measures,

the

former providing various useful

bounds

for

the

latt

er

12.

In

the

present

paper,

we

are

going

to

focus on a special

method

of

constructing

entanglement

witness using a physical observable whose spec-

trum

is

partially

known

10.

In

[1]

we have discussed

an

application of such

a

method

to

construct

entanglement

witness

out

of

the

Hamiltonian

of

a I-dimensional isotropic Heisenberg model. We have

demonstrated

the

aStrictly

speaking

every

witn

ess W gives rise

to

a

pseudo-m

eas

ure

of

entanglement

Ew

(p)

= max{O, -

Tr(W

p)}

rather

than

a

true

measure

, for in

general

it

yields zero

value for

some

e

ntangled

states.

239

usefulness

of

this

approach

with

analytic

calculation of

the

resp

ect

ive en-

tanglement

indicator for a class

of

th

e

rmal

equilibrium

states

of

the

model.

Presently, we

extend

the

res

ults

to

the

case of

an

anisotropic XXZ Heisen-

berg

chain immersed

in

a uniform magnetic field. Varying

the

control pa-

rameters

-

the

anisotropy

constant

and

the

strength

of

magnetic

field -

produces different

types

of

multipartite

e

ntanglement

in

the

ground

state.

This

in

turn

is used as

the

basis of

construction

of

witnesses

detecting

these

specific

entanglement

brands.

The

paper

is organized as follows. Section 2, for convenience

of

the

readers, introduces definitions

and

basic facts

on

multipartite

entangle-

ment.

Section 3 outlines

the

general

methods

of

witness

construction

and

presents

our

procedure

of

obtaining

multipartite

witnesses

of

specific

type

out

of

an

observable. Section 4 describes

the

results

of

analytic

calculations

performed for

the

Heisenberg model.

2.

Multipartite

entanglement

We begin

this

section

with

a review

of

basic facts

on

multipartite

entangle-

ment

of

pur

e

states.

For a more

detail

ed exposition

the

reader

is refe

rred

e.g.

to

[13,

14].

As

it

is well known,

in

bipartite

case H =

HA

@ HB

entanglement

of

pure

states

can

be

compl

ete

ly classified using Schmidt

normal

form,

d

I?p)

= L

Ai

l<P

i) @

l77i)

i=1

where l

?Pi)

E

HA

,

l77i

) E HB

are

orthonormal

systems of vectors a

nd

d

::::;

min{dimH

A,

dimHB}'

The

numbers

Al

~

A2

~

...

~

Ad

~

0,

L i A; =

1,

charact

er

ize

the

entanglement

of

I?p)

entirely, giving rise

to

a

natural

entanglement

measure,

called

the

entr

opy

of

entanglement.

If

d = 1 (i.e. Al = 1),

the

state

I?p)

is

separable.

Observe

that

the

Schmidt

form for I

?p)

is

obtain

ed by

an

appropriate

choice

of

bases

in

HA

and

HB

. In

other

words, I

?p)

is locally

unitarily

(LU)

equivalent

to

any

state

with

similar Schmidt coefficients

Ai.

In

particular,

any

state

of

the

system

of

two

qubits

can

be

brought

by

appropriate

local

unitary

transformations

to

the

form

sinBIOO)

+

cosBlll),

where

the

real

o

::::;

B

::::;

~

parameterizes

all

distinct

entanglement

classes.

Accardi L., Freudenberg W., Obya M. (Eds.) Quantum Bio-informatics IV: From Quantum Information to Bio-informatics

Подождите немного. Документ загружается.