Accardi L., Freudenberg W., Obya M. (Eds.) Quantum Bio-informatics IV: From Quantum Information to Bio-informatics

140

II.

2) Analogue and

non

separable,

Let

X =

{Xt,

t E

Rl}

be

a

system

i.i.d.

ordinary

random

variables, each of

which is

subject

to

the

standard

Gaussian

distribution

N(O, 1).

Then,

the

probability

distribution

v = IItERlLt, where

ILt

is

the

distribution

N(O,

1).

Proposition

2.2.

The probability measure space (RR,

IItB

t

,

v)

is

not

an

abstract Lebesgue space.

With

this

property

we

see

that

the

space L2(RR,

v)

is

quite

different from a

white

noise space,

and

it

is

not

useful

in

the

calculus

of

random

functionals.

3.

Poisson

noise

i) Background.

We

are

going

to

propose a new direction

of

the

treatment

of

random

functions which

are

expressed as functionals

of

Poisson noise,

As was briefly mentioned

in

the

motivation,

we

are

asked

to

introduce

a

method

of

analyzing functionals of Poisson noise.

An

urgent

request

has

come from

the

study

of

random

phenomena

the

probability

distribution

of

which is of fractional power

or

of

long (fat) tail.

Standard

distribution

of

this

kind is

the

stable

distribution.

Suppose

we

are

suggested

to

approximate

the

given fractional power

distribution

by a

stable

distribution.

Th

en

the

is

to

determine

the

power

CY,

which is one of

the

significant characteristics of

the

distribution.

To

this

end, one

may

think

of

the

least

square

method

in statistics.

But

it

is

not

recommended by

many

important

reasons. Here we do

not

go into

details

on

this

problem. A reasonable

method

uses

the

evaluation

of

the

area

given by

the

histogram

of

the

data

over intervals far from

O.

Even

the

power

CY

is

obtained,

we

can

not

investigate

the

structure

of

the

given

random

phenomena

.

In

reality,

we

have d

ete

rmined

only one-

dimensional distribution,

it

does

not

provide enough information for

the

determination

of

the

random

phenomena

in

question.

ii)

Wh

at

we

can

do is

that

we

try

to

discover

the

history

of

the

phe-

nomena,

together

with

its

environment. A favorable case is

that

the

given

stable

distribution

can

be

regarded as

what

is evaluated

at

some

instant

from a

stable

stochastic process.

One

may

think

that

because

of

the

circumstance

of

the

environment,

the

observed

data

might

have come from

the

accumulation

of

independent

data

141

obtained

in

the

past.

Such a case, we say

that

the

observed

data

can

be

embedded

in

a

stable

stochastic

process.

One

may

think

it

is

too

favorable,

but

we know

actual

data

has such a

history.

iii)

Steps

of the

analysis.

Once we

can

find favorable

stable

process, which is a Levy process. We

therefore know

the

famous Levy decomposition. In

the

present case,

there

is

no

Gaussian

component

and

constant

term

can

be

ignored. We, therefore

have

compound

Poisson processes.

To come

to

the

must

make

a few

important

remarks.

a)

The

Levy decomposition says

that

the

stable

process

just

deter-

mined

is consisting of

many

(actually, continuously

many)

inde-

pendent

Poisson

type

processes. (We call Poisson

type

process,

if

the

process

has

the

same

distribution

as

a Poisson process

up

to

constant),

although

they

are

infinitesimal. We need numerical tech-

nique

of

discriminating

those

Poisson

type

processes according

to

different

jumps.

In

other

words, we need

suitable

method

of

ap-

proximation

to

come

to

the

actual

stsps.

b) Having

obtained

a single

component

of

Poisson

type

process,

we

must

fix

the

time,

say

t = 1,

to

have a

system

of

idealized elemental

random

variables. After

that

we

can

imitate

the

steps

of

Gaussian

case

in

order

to

discuss nonli9near functionals

of

them.

c)

Each

component

of

the

stable

process is a Poisson

type

process

which is

parametrized

by

u

the

amount

of

the

jump.

If

the

jump

is

different,

then

they

are

mutually

independent.

We

are

now given a

so-called generalized

stochastic

process

with

independent

values

at

every

point

in

the

sense

of

Gel'fand.

d)

The

self-similarity

of

a

stable

stochastic

process

may

be

rephrased

as

the

duality

between

the

time

t

and

the

amount

u of

the

jump.

In

the

analysis

of

Poisson noise functionals,

this

fact

should

be

taken

into

account.

4.

Calculus

of

Poisson

noise

functionals

Noise, in

this

section, does

not

mean

the

time

derivative,

but

means

deriv-

ative in u

the

space

parameter

of

Poisson

type

processes.

The

parameter

u

denotes

the

amount

of

jump.

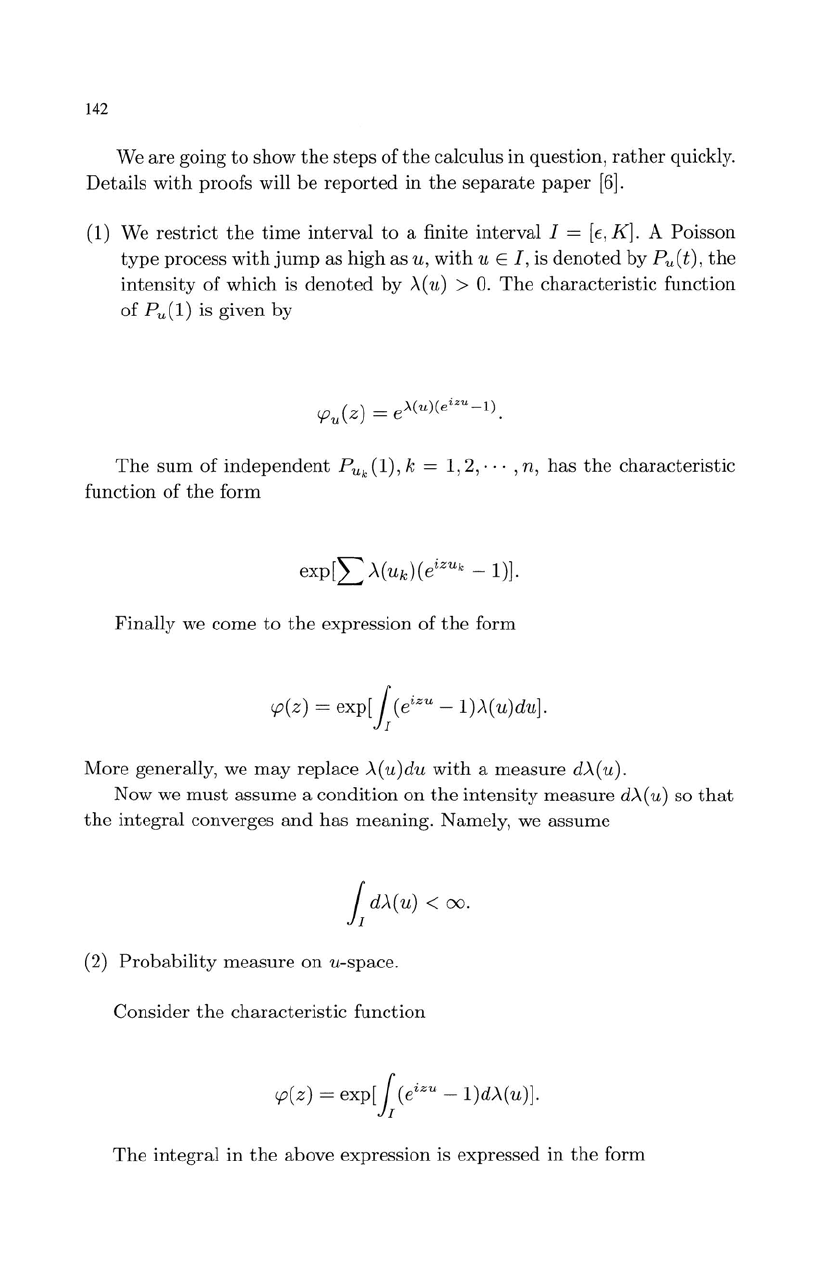
142

We

are

going

to

show

the

steps

of

the

calculus

in

question,

rather

quickly.

Details

with

proofs will

be

reported

in

the

separate

paper

[6].

(1) We

restrict

the

time

interval

to

a finite

interval

I =

[E,

K]. A Poisson

type

process

with

jump

as high

as

u,

with

u E I, is

denoted

by

Pu(t),

the

intensity

of

which is

denoted

by

>.(u)

>

O.

The

characteristic

function

of P

u

(1)

is given

by

The

sum

of

independent

P

Uk

(1), k =

1,2""

,n,

has

the

characteristic

function

of the

form

Finally

we

come

to

the

expression

of

the

form

cp(z)

=

exp[j

(e

izu

- l)>.(u)du].

More generally, we

may

replace

>.(u)du

with

a

measure

d>.(u).

Now we

must

assume

a

condition

on

the

intensity

measure

d>.(u)

so

that

the

integral

converges

and

has

meaning. Namely, we

assume

j

d>.(u)

<

00.

(2)

Probability

measure

on

u-space.

Consider

the

characteristic

function

cp(z)

=

exp[j

(e

izu

- l)d>.(u)].

The

integral

in

the

above expression is expressed

in

the

form

143

1

eiZUd)"(u)

+ canst, Z E

RI.

Noting

that

Z

can

vary

in

RI

arbitrarily,

we

can

make

the

intensity

measure

to

be

the

delta

measure, say

bUD.

This

means

that

one

can

pick

up

an

elemental (atomic) Poisson process

with

jump

uo,

let

it

be

denoted

by

F'(uo)

(3)

The

last

question is how

to

carryon

approximation.

It

is now

the

time

to

remind

the

notion

of

(t,

u)-set

introduced

by

P. Levy

[9]

and

also a

formal expression

of

a

compound

Poisson process

lim

1

(F'(u)

-

~

)dn(u),

p->oo

p>lul>l/p

1 + u

where dn(u) is

the

Levy measure. See

[1]

Section 3.2.

In

the

present

setup,

the

approximation

of

single Poisson

component

does

correspond

to

the

approximation

of

the

delta

measure

on

u-space.

It

is

in

line

with

the

passage from digital

to

analogue.

References

1.

T.

Hida,

Stationary

stochastic

processes.

Princeton

University

Press. 1970.

2.

T.

Hida,

Analysis

of

Brownian

functionals.

Carleton

Math.

Lecture

Notes

no.

13,

Carleton

University, 1975.

3.

T.

Hida,

Brownian

motion.

Springer-Verlag. 1980.

4.

T.

Hida

and

Si Si,

An

innovation

approach

to

random

fields.

Application

of

white

noise theory.

World

Scientific

Pub.

Co. 2004.

5.

T.

Hida

and

Si Si,

Lectures

on

white

noise functionals. World. Sci.

Pub.

Co.

2008.

6.

T.

Hida,

Si Si

and

Win

Htay, A noise

of

Poisson

type

and

its

gdneralized

functionals,

preprint

(submitted).

7.

J.L.

Lions,

The

earth,

planet,

the

role

of

mathematics

and

supercomputers.

(original in

Spanish).

Spanish

Inst.

1990.

8. Si Si, Effective

determination

of

Poisson

noise.

IDAQP

6 (2003), 609-617.

9.

P. Levy,

Theorie

de

l'addition

des

variables

aleatoires.

Gauthier-Villars,

1937,

10. P. Levy,

Processus

stochastiques

et

mouvement

brownien.

Gauthier-Villars.

1948. 2eme ed.

with

supplement

1965.

11. P.

Levy,

Problemes

concrets

d'analyse

fonctionnelle.

Gauthier-Villars.

1951.

12.

W.

Feller,

An

introduction

to

probability

theory

and

its

applications.

vol.1.

Wiley, 1950.

Chapt.

in

particular

Chapt.

III.

13. I.

Ojima,

Levy

process

and

innovation

theory

in

the

context

of

Micro-Macro

duality,

Proc.

The

5th

Nagoya

Levy

Seminar.

2006. 65-69.

This page intentionally left blankThis page intentionally left blank

Quantum

Bio-Informatics

IV

eds. L.

Accardi,

W.

Freudenberg

and

M.

Ohya

World

Scientific

Publishing

Co.

(pp.

145-156)

REMARKS

ON

THE

DEGREE

OF

ENTANGLEMENT

DARIUSZ CHRUSCINSKIl,

YUJI

HIROTA

2

,

TAKASHI MATSUOKA

3

AND

MASANORI OHYA

4

1

Institute

of

Physics, Nicolaus Copernicus University

2

Quantum

Bio-Informatics Center, Tokyo University

of

Science,

3 Department

of

Business

Administration

and Information, Tokyo University

of

Science, Suwa,

4 Department

of

Information

Science, Tokyo University

of

Science

We

analyze

a

measure

of

quantum

entanglement

called

degree

of

entanglement

(DEN).

It

is

shown

how

DEN

behaves

for well

known

classes

of

bipartite

states.

Moreover,

we

compare

DEN

for

quantum

states

having

the

same

marginals.

Con-

trary

to

naive

expectation

it

is

shown

that

separable

state

might

possesses

stronger

correlation

(measured

by

DEN)

than

an

entangled

state.

Keywords:

Quantum

entanglement,

Quantum

entropy

1.

Introduction

In

recent years,

due

to

the

rapid

development of

quantum

information

the-

ory

1

the

necessity of classifying

entangled

states

as

a physical resource is

of

primary

importance.

It

is well known

that

it

is

extremely

hard

to

check

whether

a given

density

matrix

describing a

quantum

state

of

the

compos-

ite

system

is

separable

or

entangled.

There

are

several

operational

criteria

which

enable

one

to

detect

quantum

entanglement

(see e.g. 2 for

the

re-

cent

review).

The

most

famous Peres-Horodecki

criterion

is

based

on

the

partial

transposition:

if

a

state

p is

separable

then

its

partial

transposition

pr

= (ll ®

T)p

is positive.

States

which

are

positive

under

partial

trans-

position

are

called

PPT

states.

Clearly

each

separable

state

is necessarily

PPT

but

the

converse is

not

true.

We

stress

that

it

is easy

to

test

wether

a

given

state

is

PPT,

however,

there

is

no

general

methods

to

construct

PPT

states.

There

are

several

measure

of

entanglement

2.

However,

there

is

no

universal

measure

which shows

that

the

problem

of

quantifying

quantum

entanglement

can

not

be

reduced

to

computing

a single

quantity.

Moreover,

145

146

various

measures

are

not

compatible:

if

EI

and

E2

are

two measures,

then

one

can

find two

states

p

and

pi such

that

EI

(p) <

EI

(pi)

but

E2 (p) >

E2(p').

It

shows

that

various

measures

shows different

aspects

of

quantum

correlations.

In

the

present

paper

we

analyze

a

particular

measure

called degree

of

entanglement

(DEN)

and

based

on

the

mutual

entropy.1O,1l,18 As

other

measures

DEN

uniquely

characterized

the

entanglement

of

pure

states.

However,

it

gives

only

a

partial

answer for

mixed

states.

This

paper

is

organized

as

follows:

in

Section 2 we

introduce

basic prop-

erties

of

DEN.

Sections 3

and

4 provide several

examples

of

quantum

states

for which one easily

compute

DEN.

Moreover, since

they

possess

the

same

marginal

states

(maximally

mixed) one

can

compare

the

corresponding

de-

gree of

entanglement.

Surprisingly,

it

turned

out

that

separable

state

can

have

stronger

correlation

(with

respect

to

DEN)

than

an

entangled

state.

Final

conclusions

are

collected in

the

last

section.

2.

The

Degree

of

Entanglement

We

begin

our

discussion

by

recalling

the

definition of

quantum

entangle-

ment.

Throughout

this

paper,

Hilbert

spaces

are

assumed

to

be

finite

dimensional.

If

e is

the

state

on

the

Hilbert

space

HI

® H

2

,

then

Tr1i2e

denotes

the

partial

trace

of

e

with

regard

to

H

2

.

Definition

2.1.

Let

H

be

a

tensor

product

Hilbert

space

of

two

Hilbert

spaces

HI

and

H2

and

B(H)

the

set

of

bounded

operators

on

H.

(1)

A

state

e

on

B(H) is

said

to

be

separable

if

there

exist

finite se-

quences

of

density

operators

{pJt'-,1 C

B(Hd

and

{O'dt'-,l

C B(H

2

)

such

that

(1)

with

"\,,N

A = 1

and

A > 0

(Vi

= 1

...

N)

~2

2

1,

-

".

(2)

A

state

e

on

B(H) is

said

to

be

entangled

if

it

is

not

separable.

The

classical

example

of

an

entangled

pure

state

is given

by

w =

Jz

(eo ®

el

-

el

® eo) - Bell

state

of

two

qubits.

Definition

2.2.

Let

HI,

H2

be

Hilbert

spaces

and

e a

density

operator

on

HI

®

H2

with

marginal

states

p = Tr1i2e

and

0'

= Tr1il

e.

The

DEN

for e

with

regards

to

p,

a is defined

by

the

following formula

1

D(()

:

p,

a) = 2{S(P) +

S(a)}

- Ie(p, a),

147

(2)

where

Ie

(p,

a) is

the

mutual

entropy

for

()

: Ie(p, a) = tr()(log

()

-log

p

(>9

a).

In

terms

of

von

Neumann

entropy,

Ie

(p,

a)

can

be

rewritten

in

the

form

Ie(p,a) = S(p) +

S(a)

- S(()). Therefore, one

obtains

finally

1

D(()

:

p,

a) = S(()) - 2{S(P) + S(a)}.

(3)

We will often use

this

form

to

calculate

DEN

in

this

and

all

subsequent

sections.

As

an

example

let us calculate

DEN

for

the

singlet

state

w.

The

marginal

states

WI

=

W2

=

~h

and

hence

one

finds D(w :

Wl,W2)

=

-log2

<

O.

Actually,

one

has

the

following

Theorem

2.1.

M.

Ohya and

T.

Matsuoka

18

Let

()

be

a pure state with

marginal states

p,

a.

Then, we have the following classification:

(1)

()

is separable

if

and only

if

D(() :

p,

a)

= 0;

(2)

()

is entangled

if

and only

if

D(() :

p,

a)

<

O.

According

to

the

above

theorem,

DEN

gives us

the

necessary

and

suf-

ficient

condition

for

the

separability

of

pure

states.

For mixed

states

one

has

3

,

10,1l

Theorem

2.2.

Assume

that

the compound state

()

is a

mixed

state.

If

()

is

separable, then D(() :

p,

a)

>

O.

Now, we

compare

quantum

bipartite

states

with

respect

to

DEN.

Definition

2.3.

Let

()1, ()2

be

states

which have

common

marginal

states

p, a.

The

state

()1

possesses

stronger

correlations

than

()2

if

the

following

inequality

holds:

D(()l : p,a) <

D(()2

: p,a). (4)

The

less value

of

DEN

one gets,

the

stronger

correlations

one

has. Here

a

natural

question arises:

Problem

Let

()1

and

()2

be

compound

states

having

the

common

marginal

states

p,

a. Assume

that

D(()l :

p,

a) <

D(()2

:

p,

a).

If

()2 is entangled, is

()1

entangled

as well?

In

what

follows we show

that

it

is

not

the

case.

148

3.

DEN

for

2-qudit

states

3.1.

Circulant

states

We

start

this

section

by

recalling

the

definition of

circulant

state.

12

(see also

Refs.

[13,

14]).

Consider

the

finite-dimensional

Hilbert

space

Cd

(d

E N)

with

the

standard

basis {eo,

el,

...

,ed-d.

Let

~o

be

the

subspace

of

Cd 0 Cd

generated

by

ei 0 ei (i =

0,

1,

...

, d - 1) :

~o

=

span{

eo

0 eo,

el

0

el,

...

,ed-l

0

ed-I}.

(5)

For

any

non-negative

integer

0:,

we define

the

operator

sa

on

Cd by

ek

f---+

ek+a(mod

d),

(k

=

0,1,

...

, d

-1),

and

denote

by

~a

the

image

of

~o

by

Id

0

sa

:

~a

=

(Id

0

sa)~o.

It

turns

out

that

~a

and

~;3

(0:

=I-

{3)

are

orthogonal

to

each

other

and

Cd 0 Cd

~

~o

EB

~l

EB

...

EB

~d-l.

(6)

This

decomposition

is called

the

circulant

decomposition.

Let

Po,

PI'

...

,

Pd-l

be

positive d x d

matrices

with

entries

in

C which

satisfy

tr(po + ... +

Pd-l)

=

1.

(7)

For

each

matrix

Pa

(0:

=

0,1,···

,d

- 1), we define

the

new

linear

operator

p~

on

(C

d

)®2

as

d-l

p~

= L

(ei'

Paej

leij

0

sa

eij

(S")*,

(8)

i,j=O

where eij

means

leil(ejl.

Since

Skeij(Sk)*

= ei+k,j+k, we

note

that

p~

can

be

also

written

as

d-l

p~

= L

(ei'

p"ej

leij

0

eHa,j+a·

i,j=O

One

can

easily check

that

the

sum

of

these

operators

d-l

p"

=

LP~

,,=0

(9)

(10)

defines a

density

matrix

on

(C

d

)®2.

For

further

details

of

circulant

states

we refer

to

Ref. 12.

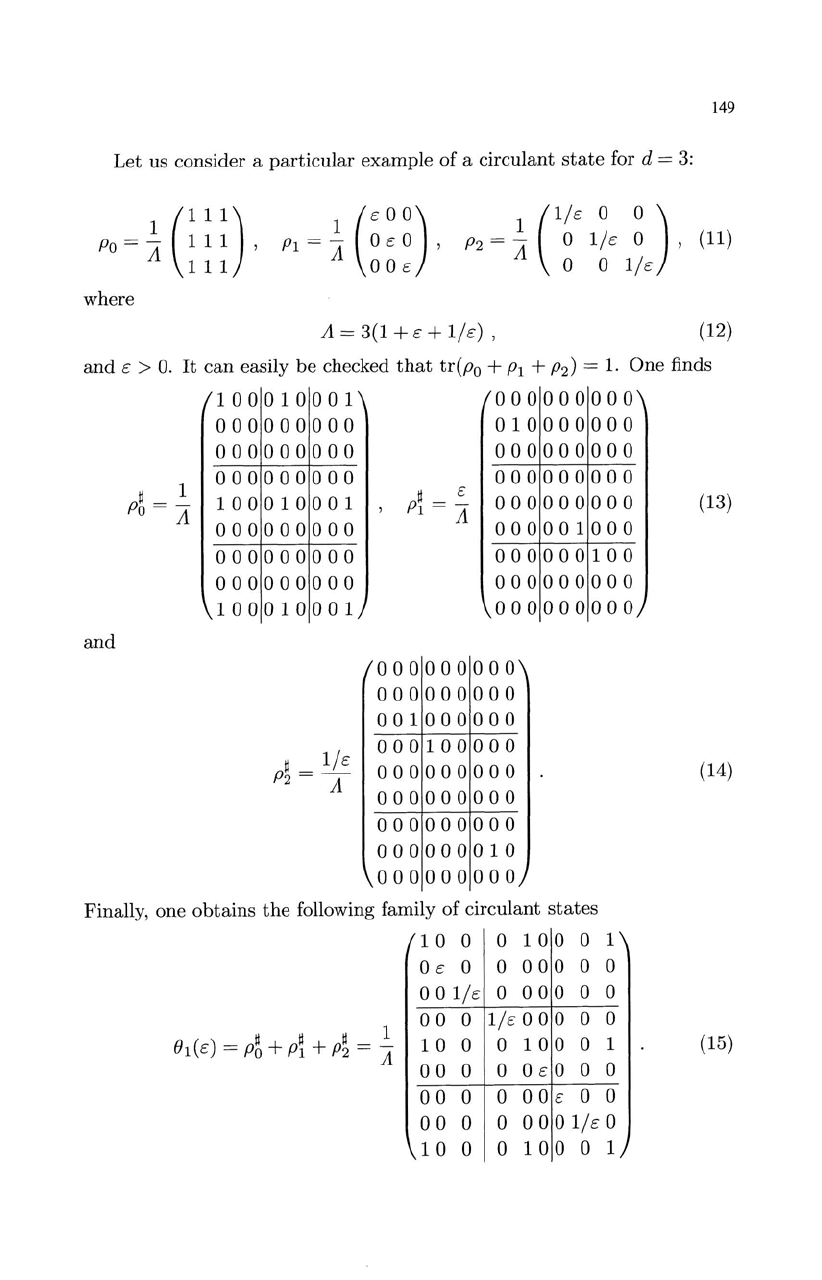
149

Let

us consider a

particular

example

of

a circulant

state

for d =

3:

1

(111)

Po

=

AlII

,

1 1 1

where

(12)

and

E >

o.

It

can

easily

be

checked

that

tr(po +

PI

+

P2)

=

1.

One

finds

and

ti

1

Po

= A

100010001

000000000

010000000

000000000

100010001

000000000

100010001

ti

_

liE

P2-A

ti

_ E

, PI - A

000 000

000

001

000

100

000 000

000

000000000

000001000

000000100

000000000

000

010

000

Finally

, one

obtains

the

following family

of

circulant

states

10

0 0

100

0

1

o E 0

0

000

0

00

liE

o

000

0

00

0

liE

0 0 0

0

10

0

o

100

0

1

00

0 0

OEO

0

00

0

00

E 0 0

00

0 0

00

o

liE

0

10

0

10

001

(13)

(14)

(15)

Accardi L., Freudenberg W., Obya M. (Eds.) Quantum Bio-informatics IV: From Quantum Information to Bio-informatics

Подождите немного. Документ загружается.