Swaminathan N., Bray K.N.C. (eds.) Turbulent Premixed Flames

Подождите немного. Документ загружается.

1.7 Modelling Strategies 23

can be incorporated in closed form. These are very attractive features of the method,

which are described in Section 2.4.

The ﬁnal, so-called mixing, term in Eq. (1.38) containing the molecular-diffusion

coefﬁcient D describes the effect of molecular transport on the PDF; the quantity

D(∂ϕ/∂x

)(∂ϕ/∂x

)|ϕ = ζ is the conditional mean scalar dissipation rate. If the

Damk

ohler number Da is small, that is, if all turbulence scales are smaller than

chemical scales, then the mixing term can be modelled as a function of turbulence

quantities alone, and the equation can be closed. On the other hand, when Da  1,

the smallest scales are chemical, and the gradient ∂ϕ/∂x

approaches that of a laminar

ﬂame, so the mixing term is strongly inﬂuenced by chemical reaction and cannot be

described simply as a function of turbulence quantities; that is, a mean-reaction-rate

closure is again required. This problem is discussed in Section 2.4. Note that, in

the most general case, with three spatial coordinates, three velocity components, N

species, and temperature, the JPDF equation involves 7 + N independent variables.

Conditional moment closure (CMC) models [63–65], which can be derived from

the JPDF transport equation, provide an alternative means of incorporating de-

tailed chemical reaction mechanisms. The basic dependent variables of CMC are

the conditional means of the species mass fractions Y

(x, t) and temperature T (x, t),

conditional on the value of a chosen scalar. In the case of non-premixed combustion,

this is the mixture fraction Z(x, t), whereas the progress variable c(x, t) is selected

[63, 66] for premixed combustion. The conditional mean of Y

(x, t) is then

(ζ; x, t) =Y

(x, t)|c = ζ,

which obeys the transport equation [63, 66, 67]

ρ|ζ

∂Q

∂t

+ρu

|ζ

∂Q

∂x



ρD

∂c

∂x

∂c

∂x





∂

∂ζ

+˙ω

|ζ−˙ω

|ζ

∂Q

∂ζ

+ e

(1.39)

where e

represents other molecular-diffusion terms and e

involves the condi-

tional ﬂuctuation y

(x, t) = Y

(x, t) − Q

(ζ; x, t). Additional source or sink terms will

arise depending on the precise deﬁnition of c, as noted in [66, 67]. The ﬁrst term

on the right-hand side of the equation contains the conditional scalar dissipation

ρD(∂c/∂x

)(∂c/∂x

)|ζ, and the same modelling difﬁculties as in the transported

PDF methods occur when Da  1. A key assumption of CMC is that ﬂuctuations

about the conditional mean are small. In ﬁrst-order CMC, these conditional mean

ﬂuctuations are ignored and the conditional mean reaction rate in Eq. (1.39) is taken

to have the same functional dependence on Q

as that of the instantaneous reaction

rate, i.e., ˙ω

|ζ=˙ω(Q). An allowance for the conditional ﬂuctuations is included

in second-order CMC by including conditional variances and co-variances, which

require further modelling. The CMC method is well advanced for non-premixed

ﬂames, but it is in its early stage for premixed ﬂames, primarily because of the issues

sourrounding the modelling of the conditional scalar dissipation rate. A preliminary

application [68] of this methodology to lean premixed ﬂames is encouraging. How-

ever, in a problem with three spatial coordinates and N +1 scalar variables, there

are N +1 CMC equations, each with four independent variables.

In many situations of practical interest, Da > 1, and regions of chemical reaction

form thin interfaces separating unburned reactants from fully burned combustion

24 Fundamentals and Challenges

products. The mean burning rate can then be speciﬁed as the ﬂame area per unit

volume – the ﬂame surface density (FSD) – multiplied by the rate of conversion

of reactants to products per unit ﬂame area. The surface density function (ζ; x, t),

deﬁned as the mean area per unit volume of the isosurface on which c(x, t) = ζ,isa

distribution function, analogous to the PDF

P(ζ; x, t). As shown in Section 2.2, the

two are related by [69, 70]

(ζ; x, t) =





∂c

∂x

∂c

∂x



1/2



c = ζ

P(ζ; x, t), (1.40)

and (ζ; x, t) obeys the equation [71, 72]

∂

∂t

∂

∂x



+ S

d,c







(

− n

)

∂u

∂x



 +



d,c

∂n

∂x



. (1.41)

Here S

d,c

is the displacement speed of the isosurface c(x, t) = ζ and n

is the compo-

nent of the unit normal vector n, pointing towards small values of c in the direction

of x

. Surface averages are denoted by Q

and are deﬁned as

Q

QG|ζ

G|ζ

where G ≡ [(∂c/∂x

)(∂c/∂x

)]

1/2

is the magnitude of the progress-variable gradient.

Terms on the left-hand side of Eq. (1.41) represent unsteady effects and the inﬂuences

of convection and isosurface propagation, respectively. The ﬁrst term on the right

represents effects of tangential strain that are due to the local velocity ﬁeld, and

the ﬁnal term arises from combined effects of surface curvature and propagation.

Note that the velocity appearing in this equation is the local velocity in the interior

of the thin ﬂame, at locations where c(x, t) = ζ, so extensive modelling is required

to derive a closed version of Eq. (1.41). The FSD may be deﬁned as the value of the

surface density function at a chosen isosurface, for example, the value of c at which

˙ω



(x) =



(ζ; x)dζ =



G|ζP(ζ; x)dζ =





. (1.42)

The local displacement speed S

d,c

is often estimated in terms of the burning velocity

of an unstretched laminar ﬂame, so ρ(ζ)S

d,c

= ρ

An alternative starting point for thin-ﬂamelet combustion models, i.e., for

Da > 1, is provided by the level set or G-equation formalism [41, 74], which is also in-

troduced in Section 2.2. A function G(x, t) is introduced, which s atisﬁes G(x, t) = G

on the thin-ﬂame isosurface. The isosurface is assumed to be an interface between

reactants and products, propagating with a velocity S

relative to reactants, which

is modelled in terms of the strain and curvature of the surface G(x, t) = G

.The

Huygens-type equation, which is a kinematic equation, describes the evolution of G

∂G

∂t

+ u

∂G

∂x

= S



∂G

∂x

∂G

∂x



1/2

. (1.43)

The methodology is developed by Peters [41] and Peters et al. [74] by averaging

Eq. (1.43). Although the function G(x, t) lacks physical signiﬁcance everywhere

1.7 Modelling Strategies 25

except on the surface G(x, t) = G

, averaging involves values for which G(x, t) = G

so a functional form must be speciﬁed. It can be shown [75] that the ﬂame surface

density is related to the G ﬁeld by

(x) =





∂G

∂x

∂G

∂x



1/2



G = G

P(G

; x).

An approximate expression has been derived for

P(G

; x)[75], where P(G; x)isthe

PDF of G.

A striking and important feature of all the schemes for determining mean rates

of chemical reaction introduced here is that a mean or conditional mean scalar

dissipation function plays a central role in each case. In a presumed PDF model

for the progress variable c, the mean dissipation 

strongly inﬂuences the variance





, which, in turn, decides the width of the PDF



P(ζ; x). As may be seen from

Eq. (1.35), this PDF determines the mean rate. And, in the high Damk

ohler limit

that approximates many practical situations, the mean rate is directly proportional

to 

, as shown by Eq. (1.36). This simple expression reminds us that, in a thin

ﬂame, the local rate of reaction must be directly balanced by the rate at which

molecular-transport processes carry heat and reactive species through the preheat

zone at the cold side of the ﬂame. In the transported PDF and CMC models, this

physics is represented in the conditional scalar dissipation or micromixing model.

The surface density function, on which FSD models are based, is deﬁned in terms of

a local gradient, as seen in Eq. (1.40), so the generalised FSD of Eq. (1.42) can be

rewritten as



(ρ

)

1/2

where it is assumed that ρD = ρ

. Finally, the G-equation model of Peters [41]

and Peters et al. [74] involves the scalar dissipation of G. In each of these models, the

accepted practice has generally been to represent the scalar dissipation in terms of a

relatively simple algebraic model, and this has often been borrowed from studies of

non-reactive turbulent ﬂows, namely



= C

ε







where C

is a constant. In circumstances characterised by Da > 1, one must expect

to ﬁnd that 

is inﬂuenced by the laminar ﬂame time δ

in addition to the

turbulence time



k/.Thescalar-dissipation-rate transport approach, described in

Section 2.3, recognises the key role played by the scalar dissipation and determines

it from a closed version of its transport equation containing both characteristic scales.

It is also possible to obtain an algebraic model for the FSD 



(2C

− 1)



1 +







1 +

εδ









using the scalar-dissipation-rate approach [76]. The model parameters deﬁned in

Section 2.3 suggest that C

is of the order of unity, and thus it follows that

εδ

/(C



)  1 for large-Da ﬂames. Hence the FSD scales with δ

rather than

26 Fundamentals and Challenges

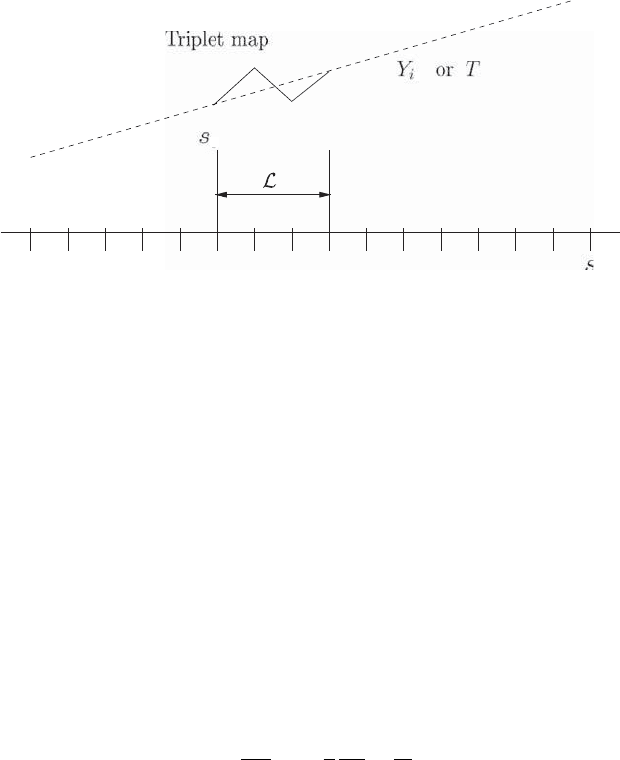
Figure 1.4. Illustration of triplet mapping in a LEM.

with . Earlier models for the FSD scaling with  are discussed in the book edited

by Libby and Williams [10].

From a physical point of view, the evolution of an instantaneous scalar value at a

point inside a turbulent ﬂame is inﬂuenced by the molecular diffusion, reaction, and

convection as conveyed by the last of Eqs. (1.1). Thus, the mean reaction rate is also

expected to be inﬂuenced by these processes, and the closure models previously dis-

cussed attempt to capture these effects by using a simpliﬁed description. In another

approach, known as the linear eddy model (LEM), it was suggested in [77] that these

effects can be accounted for by solving a one-dimensional (1D) unsteady reaction–

diffusion equation along with a stochastic term representing the turbulent mixing or

stirring. This approach was developed, elaborately discussed, and tested by Kerstein

[77–83] for a variety of ﬂows with scalar mixing and chemical reactions. Thus details

can be found in those references. Here, the main features of this approach and its

similarity, where there are any, to other approaches are discussed brieﬂy.

The 1D unsteady equation for the mass fraction of species i is written as

∂Y

∂t

=−

∂J

∂s

˙ω

+ F

stir

, (1.44)

with the stirring term F

stir

included. The direction s is taken to be aligned with

the local normal speciﬁed by the gradient of Y

. The size of this 1D domain is

typically taken to be about 6 in numerical calculations. Because the reaction rate

depends on temperature, Eq. (1.44) is to be supplemented with a similar equation for

temperature T . In these two equations, the diffusion and reaction are described in a

deterministic fashion and the stirring part is modelled with a stochastic approach. The

turbulence is usually expected to increase the scalar gradient and isoscalar surface

area by the action of the compressive principal strain rate on the scalar ﬁeld (for an

elaborate discussion of this physics see Section 2.3). This physics is simulated by a

triplet mapping. A clear exposition of this mapping is given by Kerstein [82] and is

schematically shown in Fig. 1.4, identifying two important parameters, viz., (1) the

location s

for the mapping and (2) its size L. The location s

is chosen randomly

with equal probability for all discrete locations inside the 1D domain. Because F

stir

represents the stirring by turbulent eddies ranging from  to η

, the 1D domain

is discretised according to DNS requirements and the Kolmogorov length scale is

obtained with η

= 4Re

3/4

(



3/2

/ε) in the context of RANS. The mapping size L is

1.7 Modelling Strategies 27

chosen randomly in the range  ≥ L ≥ η

by use of a PDF given by

P(L) =

−8/3

−5/3

− 

−5/3

. (1.45)

This PDF is obtained by drawing an analogy of the stirring event with the random

walk of a marker particle and using the inertial range scaling of Kolmogorov turbu-

lence [82, 84]. This analysis is also used to obtain a frequency, λ

with a dimension

of L

−1

, of this stirring event as

ν Re



(

/η

)

5/3

− 1

1 −

(

/

)

4/3

, (1.46)

where C

is a model parameter. Now, one can see that Eq. (1.44) can be solved using

any standard numerical technique, noting that it is an instantaneous representation,

although an operator-splitting method is useful because of the stiffness associated

with ˙ω

. These solutions are then ensemble or time averaged to obtain the mean

reaction rate and the density or temperature required for the computational ﬂuid

dynamics (CFD) solution. Application of this methodology to turbulent premixed

combustion is discussed in [84–87].

An important application of simulation codes for turbulent combustion is to

estimate the inﬂuence of design changes on emissions of pollutant species and

greenhouse gases, and this necessitates the incorporation of realistic chemical ki-

netic mechanisms. The growing use of non–fossil fuels increases the importance of

this requirement. At present there are two types of approach to this problem. De-

tailed kinetic mechanisms can be directly incorporated into transported PDF, CMC,

and LEM models, although, to reduce computing costs, it is usual to use one of

several available methods (intrinsic low-dimensional manifold, in situ adaptive tab-

ulation, computational singulor perturbation, etc.) to limit the size of the mechanism

[20, 21, 88–90]. Also, as pointed out earlier, our current understanding of possible

chemical kinetic effects on the mixing or scalar dissipation processes in these models

is still incomplete. The alternative approach (FGM, FPI) [89, 91, 92] (also see Sec-

tion 2.2), applicable to the various thin-ﬂamelet models, is to assume combustion to

occur under conditions similar to those to be found in an unstretched laminar ﬂame.

Then data from a laminar ﬂame calculation at appropriate values of mixture frac-

tion, pressure, and initial temperature can be tabulated as a function of the progress

variable, and mean species mass fractions can be estimated from

ρ(x)



(x) =



ρ(ζ)Y

(ζ)P(ζ; x, t)dζ,

where

P(ζ; x, t) is a presumed PDF, calculated as described earlier.

1.7.3 Models for LES

In LESs, mean ﬂuid mechanical and reaction-rate closures are required for replac-

ing the SGS information that is lost as a result of the volume-averaging process of

Eq. (1.19). Turbulent ﬂow models are based on closures developed for non-reactive

28 Fundamentals and Challenges

ﬂow applications. In the Smagorinsky model [93] for constant-density ﬂows, unre-

solved Reynolds stresses are represented by

− u

−

[



− u



]

=−ˆν



∂

∂x

∂

∂x



, (1.47)

where ˆν

is a SGS eddy viscosity given by ˆν

= (C

)

|S|.Here is size of the LES

ﬁlter and

S is the resolved part of the shear stress with its component S

appearing

inside square brackets in Eq. (1.47). However, the Smagorinsky model is found

to be too dissipative and the coefﬁcient C

depends on the ﬂow conﬁguration. In

dynamic or scale-similarity models [94, 95], the coefﬁcient C

(x, t) and the dissipation

are determined locally as part of the solution, by introducing two ﬁlter scales, the

scale  of the LES and a second larger scale

, reﬁltering the LES solution at this

second scale, and using the identity



, which assumes that the smallest of

resolved scales and the largest of unresolved scales behave similarly. The presence

of heat release does not seem to affect this [96]. Further details and application

to variable-density ﬂows can be found in many references cited in the following

discussion. Unresolved scalar ﬂuxes in combustion simulations are often described

in terms of a gradient transport assumption,



− ˜u

=−

ˆν

∂

∂x

, (1.48)

where σ

is a Schmidt number and ˆν

is determined from the model for unresolved

Reynolds stresses. However, by ﬁltering DNS data, Tullis and Cant [97] showed

that counter-gradient scalar ﬂuxes can occur. These ﬂuxes are considered to come

from laminar ﬂamelets, and thus they may be included by modelling the SGS ﬂux as

ρuc − ρ



c = ρ

(c −



c). Also, an alternative view of treating this subgrid ﬂux as a

source under some restrictive assumptions was also expressed [98].

Heat release occurs at scales that are unresolved in combustion LES, so models

are required. These are often developed from models that have previously been

used in RANS calculations. The current LES approaches for premixed ﬂames can

be broadly categorised as (1) eddy break-up (EBU) and presumed PDF models,

(2) FSD models, (3) thickened-ﬂame models, (4) G-equation models, and (5) linear

eddy models (LEM-LES). The transported PDF [99] and CMC [100, 101] methods

have been used as SGS models in the LES of non-premixed ﬂames. Although these

methods can be extended to premixed combustion, no attempts to do so have yet

been made. The methods just noted are subsequently brieﬂy reviewed and discussed

below to identify their essential features. This discussion is not intented to be exhaus-

tive, and interested readers are referred to the cited references for a comprehensive

discussion.

(1) EBU AND PRESUMED PDF MODELS. In EBU modelling for RANS, the averaged

reaction rate is expressed as a sum of two inverse time scales: One is related to

the chemical kinetics and the other one is related to turbulent mixing, as noted by

Spalding, Magnussen, and their co-workers. This modelling is extended [102–104]

to LES by replacing the turbulent mixing time scale with the corresponding one

for the SGS. This mixing time scale is typically expressed [103]asτ

≈ k

sgs

/ε

sgs

with ε

sgs

= c

3/2

sgs

/, where c

is a model parameter. The subgrid kinetic energy k

sgs

1.7 Modelling Strategies 29

is obtained by the solution of a modelled balance equation [103] or the dynamic

procedure. In another approach [103, 105] the ﬁltered reaction rate is obtained

with

˙ω =



sgs

(Y)˙ω(Y)dY, where P

sgs

is the subgrid PDF, which is taken to be

a multidimensional Guassian function. The calculations [103] of a turbulent lean

premixed propane ﬂame stabilised behind a ‘V’ gutter shows that the predicted

mean quantities of engineering interest are insensitive to the SGS combustion model.

However, the choice of numerical solver and grid resolution can affect the details

of predictions in LES of reacting [106] and non-reacting [107] ﬂows, which is a

somewhat less attractive feature.

(2) FSD MODELS. In the FSD and thickened-ﬂame approaches, the general philosophy

to model the ﬁltered reaction rate is to write

˙ω = ρ

, where  = |∇c|is the FSD

per unit volume in a given computational cell, which is usually referred to as a ﬁltered

FSD or sometimes it is called subgrid FSD. An algebraic model for this quantity was

proposed [73]as

 = 4



c(1 − c)



, (1.49)

where 



= |∇c|/|∇c| is the subgrid ﬂame-wrinkling factor, which is modelled by

two methods. Although the simplest one is to treat this factor as unity [73], an

algebraic expression







1 +





was proposed [108, 109] using fractal analysis in which the exponent β is related to

the fractal dimenison

D by β =

D − 2. The typical value of

D varies between 2 and

3[110, 111 ] and it scale dependence was also investigated using dynamic procedure

[109]. The inner cut-off length scale η

can be the Kolmogorov or Gibson length

scales, or δ

[110], or the inverse of mean ﬂame curvature [108, 109], |∂n

/∂x



−1

It seems that a good prediction of

˙ω when these models are used strongly depends on

the choices for the exponent and the inner cut-off length scale. These two quantities

can also depend on the Karlovitz number [112] as the fractal nature of the ﬂame

surface depends on the combustion regime.

In the second method, a transport equation for 



is derived and solved [113–

115] along with additional modelling for generation and removal rates of 



. These

modellings introduce further uncertainities, although the calculations were shown

[113–115] to compare well with measured ﬂuid dynamic quantities.

The algebraic model given in Eq. (1.49)isusedin[116] to calculate turbulent

premixed ﬂames propagating in a channel with a square obstruction. The computed

results showed an underprediction of about 20%–30% in peak pressure and also

a time lag in the pressure history compared with experimental measurements. The

reason for this is unclear.

The FSD required for obtaining the ﬁltered reaction rate can be written [110]as

 =|∇c|



resolved

|∇c|−|∇c|



 

unresolved

=|∇c|+U. (1.50)

30 Fundamentals and Challenges

By taking the resolved part to be from Eq. (1.49)with



= 1, the unresolved part

is obtained [110]as

U ≈ C





c(1 − c)



−

c(1 −





, (1.51)

using the dynamic procedure with a test ﬁlter of size

. The model parameter C

obtained [110] by fractal analysis with δ

as the inner cut-off scale, tends to 1.8

when the ﬁlter size  becomes small (<2 mm). The SGS contribution U can become

signiﬁcant, irrespective of the combustion regime, which can inﬂuence the LES

prediction when algebraic models are used.

In an alternative approach to modelling 



, a transport equation for the ﬁltered

FSD is derived and solved [73, 111, 117–119]. This equation is written as follows after

generalised FSD equation (1.41) is ﬁltered:

∂



∂t

∂

∂x



+ S

d,c





(

− n

)

∂u

∂x



 +



d,c

∂n

∂x



. (1.52)

The ﬁltered convective term and the two terms on the right-hand side of Eq. ( 1.52)

need to be modelled, and they are discussed in [111, 117–119]. An elaborate discus-

sion on this topic can be found in Section 2.2. It was also noted [120] that the physical

realisablity requirement (i.e.,

 ≥ 0) may not always be satisﬁed by the preceding

algebraic and transported FSD models and care needs to be exercised.

(3) THICKENED-FLAME MODEL. The preceding subgrid modelling approaches for the

ﬁltered reaction rate assume the ﬂame front to be a laminar ﬂame, which is typically

smaller than the LES mesh size. Thus the ﬂame-front structure is not captured, and so

alternative modelling methods are needed to predict minor species, pollutants, and

their formation rates. A simple approach developed speciﬁcally to resolve the ﬂame-

front structure in LES involves artiﬁcially thickened ﬂames [111]. An unstretched

laminar ﬂame propagates at a speed S

∼ (ˆαA)

1/2

, where A is the pre-exponential

factor of the heat release rate, ˆα ∼ S



is the thermal diffusivity, and δ



is the ﬂame

thickness, from which it follows that δ



∼ (ˆα/A)

1/2

. Consequently, if the thermal

diffusivity is artiﬁcially increased by a factor F and the pre-exponential factor is

decreased by the same amount, the ﬂame speed remains unchanged, whereas the

ﬂame thickness is increased by the factor F. This quantity is chosen so that the

reaction layer thickness Fδ



can be resolved on the LES computational mesh. Also,

the ﬂame evolution time is artiﬁcially increased by the factor F . An allowance is

then made for SGS ﬂame wrinkling by use of a stretch efﬁciency function [111]

to compensate for the decrease in ﬂame wrinkling because of artiﬁcial thickening.

Other approaches, such as ﬁltered tabulated chemistry [98] or multidimensional

ﬂamelet-generated manifold [121] methods with a presumed subgrid PDF [91] can

be used in conjunction with the thickened-ﬂame approach. Details are discussed in

Section 2.2.

(4) G-EQUATION MODELS. This method is also known as ﬂame-tracking method [122–

125], in which the ﬂame front is treated as a thin interface and thus the standard ﬁlter

kernel cannot be used because the ﬁltering has to be done along this interface where

1.8 Data for Model Validation 31

G is meaningful [126–128]. This introduces an additional quantity, the conditionally

ﬁltered ﬂow velocity, requiring modelling that is typically represented [127]asthe

sum of the Favre ﬁltered velocity



v and a correction obtained by use of the velocity

jump across a laminar front. A form of this ﬁltered equation is written as Eq. (2.64),

and the related modelling is given in Section 2.2. Here we just note that the the

important modelling part of this approach is in the turbulent burning speed S

which is usually written using [129, 130]

= 1 + A







where A and n are model parameters taken to be constants or estimated using the

dynamic procedure as explained in [125]. Other possible modelling of S

is discussed

in [131]. It is widely accepted that other effects, such chemical kinetics, non–unity

Lewis number, etc., can be incorporated into the laminar ﬂame-speed S

part of the

model.

(5) LEM–LES. The LEM approach discussed earlier within the RANS context can

be extended to LES, as was discussed in [132–134]. The essence of this extension

is to limit the stochastic stirring event to scales below the ﬁlter scale  so that the

triplet mapping is limited to the range  ≥ L ≥ η

. This is achieved by replacing  in

Eqs. (1.45) and (1.46)with. Other subtleties in this approach, such as the inﬂuence

of volumetric expansion on the ﬂow ﬁeld or LEM domain size, need to be considered

carefully while this model is implemented. These are explained in [132–134], and an

application of the methodology to simulate soot formation can be found in [135].

1.8 Data for Model Validation

Empiricism is unavoidable in the development of submodels to replace unknown

terms in the averaged transport equations of both RANS and LES, and comparisons

with data from experiments and DNS provide a vital link in the development process.

Until relatively recently, experimental comparisons were mainly restricted to a global

level, in which a RANS or averaged LES prediction of a combustion ﬂow ﬁeld

is compared with averaged data from experiments. However, the RANS or LES

calculation necessarily involves many closure assumptions, so it is difﬁcult to isolate

the performance of a particular submodel in this way. Only in the past few years has

it become possible to make more detailed measurements that are directly relevant

to a speciﬁed unknown term in an averaged transport equation.

An example of the ﬁrst type of comparison is provided by turbulent ﬂame

speeds. An example of the use of turbulent ﬂame-speed data to test a turbulent

combustion model is provided in Section 2.3. The idea that the propagation of a

premixed turbulent ﬂame can be characterised as a burning velocity, by analogy with

laminar ﬂames, has been explored for many years, and a large amount of information

is available

from theoretical analysis [136–140], experiments (see [42] for further

references), RANS calculations (see [140, 141] for further references), and DNS

(see for example [72]), so it provides a way to test the overall performance of a

It is not possible to cite all the work on this topic here. However, the relatively recent works cited

here provide a good start for an interested reader.

32 Fundamentals and Challenges

RANS model against published experimental data. A burning velocity – laminar

or turbulent – can be deﬁned as the mass ﬂow per second of reactants converted

to combustion products, within a speciﬁed streamtube, divided by the density of

unburned reactants and streamtube area, namely

ρA



˙ω

A(n)dn,

where n is a coordinate normal to the local mean ﬂame brush, A(n) is the streamtube

area at location n, A

is the streamtube area at a chosen location n = m, and

integration is extended through the whole streamtube volume within the thickness

of the brush. The propagation speed deﬁned in this way is known as the consumption

speed. It is important to note that the thickness of an averaged turbulent ﬂame brush

is often signiﬁcant in comparison with other scales of the averaged ﬁeld, and the

streamtube area is generally not constant, so care is required in the choice of an

appropriate normalising ﬂame area A

Alternatively, the displacement speed of the ﬂame S

is the propagation speed

relative to the mean ﬂow of a chosen isosurface of the mean ﬂame, for example, the

surface on which

c =

. It is usual to choose an isosurface close to the cold side of

the ﬂame, and then

 1. The displacement and consumption s peeds of a given

combustion ﬂow can be signiﬁcantly different from each other [42, 142]. In addition,

Driscoll [42] pointed out that the turbulent burning velocity should not be regarded as

a universal property, because it is inﬂuenced by the type of burner used to stabilise

the ﬂame. This sensitivity arises because the process of ﬂame wrinkling develops

in different ways, depending on the choice of burner geometry. For example, the

wrinkles on a Bunsen ﬂame grow in amplitude as they are convected downstream,

whereas those on an expanding spherical ﬂame are continually stretched and grow

in wavelength.

Experimental data can also be used to make a more direct test of a speciﬁc

submodel within a complete RANS or LES model. For example, simultaneous, local

time-resolved measurements of velocity u(x, t) and the mass fraction Y

(x, t) provide

information from which both the scalar ﬂux





(x) and the gradient ∂



/∂x

(x) can

be calculated. The validity of the gradient transport submodel of Eq. (1.27) can then

be directly tested. Clear evidence of counter-gradient scalar transport in premixed

turbulent combustion was found in this way, as discussed in Section 2.1. A second

example is provided by the FSD . A reasonable approximation to the mean of

this quantity, which, as we have seen, is central to some mean-reaction-rate models,

can be found (see for example [143, 144]) from the length of isocomposition lines

in 2D laser-sheet images. Alternatively, the area of the isocomposition surface can

be determined from two parallel laser sheets. Recent advances in laser diagnostics

for combustion now offer the prospect of direct experimental validation of a wider

range of important submodels under conditions that cannot be achieved by DNS.

There is a continuing need for well-planned experiments to provide data for com-

parison with turbulent combustion models, and this need is particularly apparent in

the case of premixed and partially premixed combustion at high turbulence inten-

sities. Experimental conﬁgurations should be sufﬁciently simple to ease problems

of numerical simulation, and boundary conditions should be accurately determined.