Seely F.B. Analytical Mechanics for Engineers
Подождите немного. Документ загружается.


ALGEBRAIC
METHOD
51
used. The
principle
is
expressed
by
the
equation
Ra
=
in
which a
(Fig.
5
la)
is the
perpendicular
distance from
the mo-
ment-center,
0,
to
the action line
of the
resultant,
and
SAfo
is
the
algebraic
sum
of the
moments of the
forces
with
respect
toO.
Hence,
if
the
resultant
of
a
coplanar,
non-concurrent,
non-
parallel
system
of
forces is
a
force,
it
may
be determined
completely
by
the
equations,
If
both 2F
X
and 2F
V
are
equal
to zero the resultant is not a
force
and
hence
is
a
couple
the
moment,
C,
of
which,
according
to
the
principle
of
moments,
is
the
algebraic
sum of the moments of
the forces of
the
system,
that
is,
The
center
about
which the
moments of the forces are taken
may
be
any point
in
the
plane
of the forces since the moment of a
couple
is the
same
about all
points
in
the
plane.
The sense
of
rotation
of
the
resultant
couple
is
indicated
by
the
sign
of the
algebraic
summation and
the
aspect
of the
couple,
of
course,
is
the
same
as
that of
the
plane
of the
forces.
If
2F
X
and ^F
v
are
equal
to
zero and
2M
is also
equal
to
zero,
the
resultant is
equal
to zero.
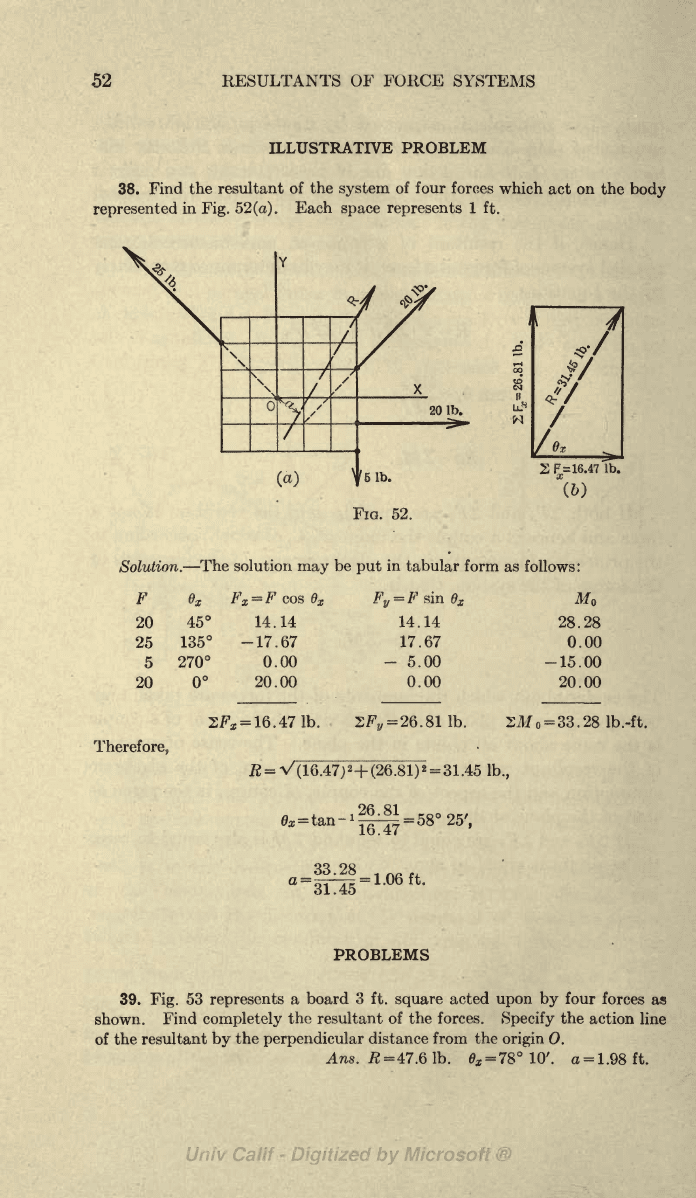
52
RESULTANTS
OF FORCE
SYSTEMS
ILLUSTRATIVE
PROBLEM
38.
Find
the
resultant of
the
system
of four forces
which
act on
the
body
represented
in
Fig.
52
(a).
Each
space represents
1
ft.
w
Solution.
The solution
may
be
put
in
tabular form
as
follows:
F
20
45
Therefore,
14.14
25
135
-17.67
5
270
0.00
20
20.00
F
y
=F sin e
x
14.14
17.67
-
5.00
0.00
16.471b.
R
=
V(16.47)
2
+(26.81)
2
=
31
.45
lb.,
26.81
03
=
tan
-
16.47
=
58
25',
33.28
M
28.28
0.00
-15.00
20.00
o
=
33.
28 Ib.-ft.
PROBLEMS
39.
Fig.
53
represents
a board
3
ft.
square
acted
upon by
four
forces
as
shown.
Find
completely
the
resultant of
the
forces.
Specify
the
action
line
of
the resultant
by
the
perpendicular
distance from
the
origin
0.
Ans.
#
=
47.6 lb.
6^
=
78
10'.
a
=
1.98
ft.
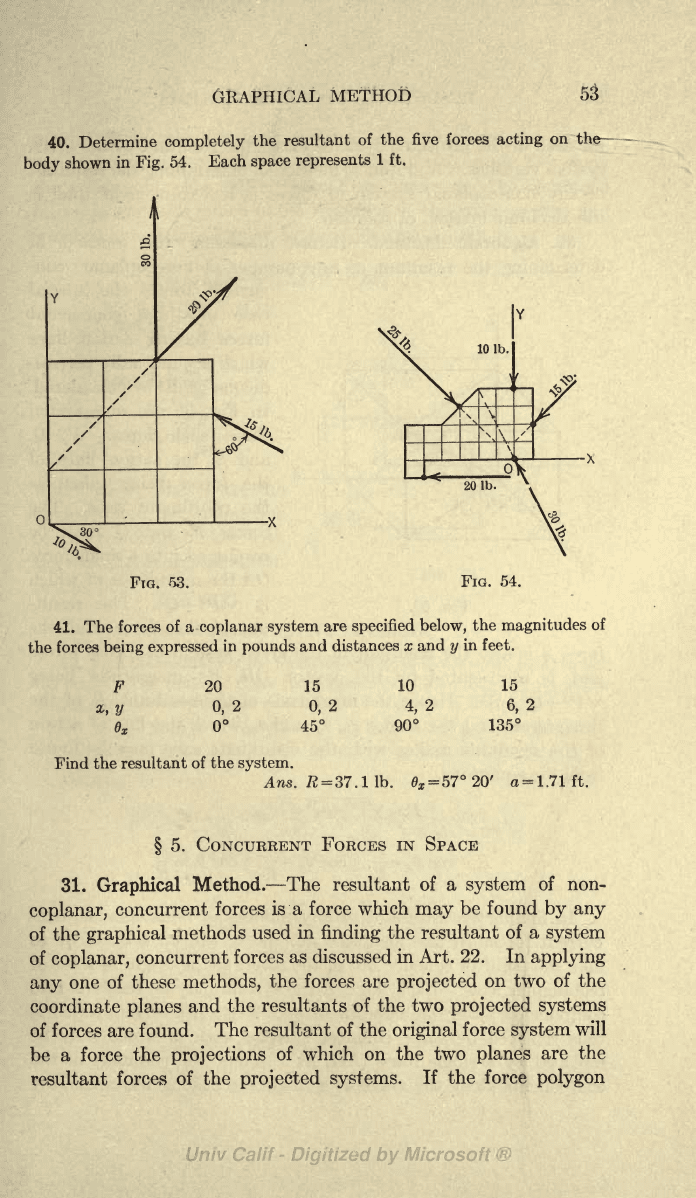
GRAPHICAL
METHOD
53
40.
Determine
completely
the
resultant
of
the five
forces
acting
on the-
body
shown
in
Fig.
54.
Each
space
represents
1
ft.
FIG.
53.
FIG. 54.
41.
The forces
of a
coplanar
system
are
specified
below,
the
magnitudes
of
the
forces
being
expressed
in
pounds
and
distances
x
and
y
in
feet.
20
0,
2
15
0,
2
45
10
4,2
90
15
6,2
135
Find the
resultant
of
the
system.
Ans.
fl
=
37.1 Ib.
6
X
=
57
20' a
=
1.71 ft.
5.
CONCURRENT
FORCES
IN
SPACE
31.
Graphical
Method.
The
resultant
of a
system
of non-
coplanar,
concurrent
forces is a force which
may
be
found
by
any
of the
graphical
methods
used
in
finding
the
resultant
of a
system
of
coplanar,
concurrent
forces
as
discussed
in Art. 22.
In
applying
any
one
of
these
methods,
the
forces
are
projected
on two of
the
coordinate
planes
and
the resultants of
the
two
projected
systems
of forces are found.
The
resultant
of
the
original
force
system
will
be
a force
the
projections
of which on
the two
planes
are
the
resultant
forces
of the
projected
systems.
If
the
force
polygon
54
RESULTANTS OF
FORCE
SYSTEMS
for
each of
the
projected
systems
closes the
resultant of
the
given
system
vanishes. A
graphical
method of
determining
the
resultant
of
any non-coplanar
system
of
forces is less
convenient than is
the
algebraic
method
of
solution.
32.
Algebraic
Method.
Before
discussing
the
method of
determining
the
resultant of
any
number
of
non-coplanar,
con-
current forces the
special
case of three
concurrent
forces
having
action
lines
which
are
mutually perpen-
dicular will
be
considered.
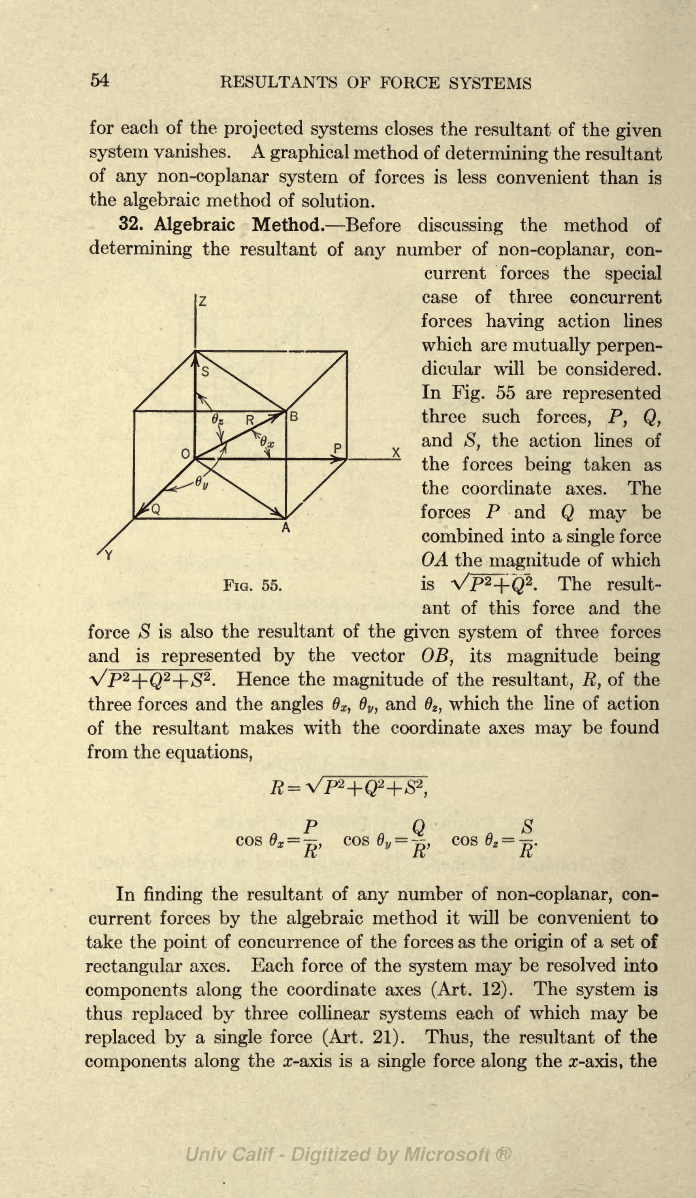
In
Fig.
55 are
represented
three
such
forces,
P,
Q,
and
S,
the action
lines of
the
forces
being
taken
as
the
coordinate
axes. The
forces P
and
Q
may
be
combined into a
single
force
OA
the
magnitude
of
which
FIG.
55.
is
VF^+Q
2
.
The result-
ant of this force and the
force
S is
also the
resultant
of
the
given
system
of
three forces
and is
represented by
the
vector
OB,
its
magnitude being
Vp
2
+Q
2
+$
2
.
Hence the
magnitude
of
the
resultant,
R,
of the
three
forces and
the
angles
6
X
,
V)
and
2
,
which
the
line of
action
of the resultant
makes
with the coordinate
axes
may
be found
from
the
equations,
P
Q
COS0
x
=
-
5
,
0080,
=
,
rr
COS d
z
=
-=:.
In
finding
the resultant
of
any
number of
non-coplanar,
con-
current
forces
by
the
algebraic
method
it
will
be convenient
to
take
the
point
of
concurrence of the forces as
the
origin
of a
set
of
rectangular
axes. Each
force
of the
system
may
be resolved
into
components
along
the coordinate axes
(Art.
12).
The
system
is
thus
replaced by
three
collinear
systems
each
of which
may
be
replaced by
a
single
force
(Art. 21).
Thus,
the
resultant of
the
components along
the
z-axis is a
single
force
along
the
x-axis,
the
ALGEBRAIC
METHOD
55
magnitude
of
which
is
expressed by
2F
X
.
Similarly,
the
ponents
may
be
replaced
by
a
single
force
of
magnitude
2F
y
along
the
i/-axis,
etc.
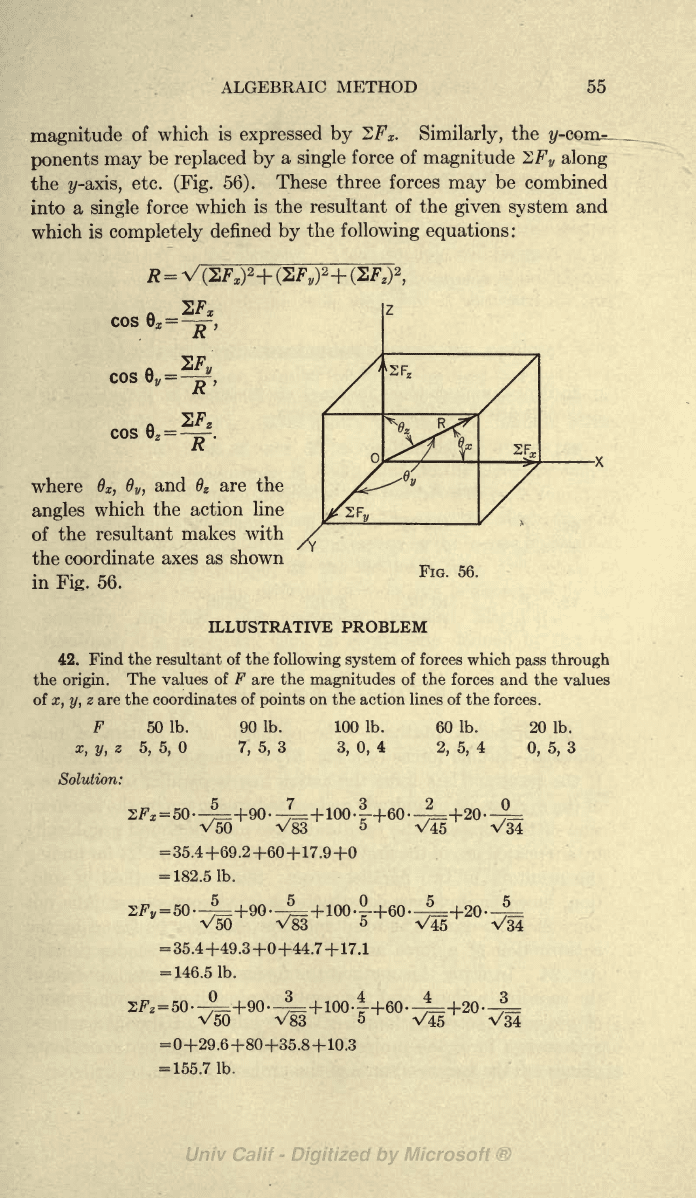
(Fig.
56).
These three forces
may
be combined
into
a
single
force
which
is the resultant of the
given
system
and
which
is
completely
defined
by
the
following equations:
R
=
cos
*
=
cos
v
=
where
6
X
,
8
V
,
and
S
z
are the
angles
which
the action line
of
the
resultant
makes
with
the
coordinate
axes
as
shown
in
Fig.
56.
2F
Z
2F"
FIG. 56.
ILLUSTRATIVE
PROBLEM
42.
Find the resultant
of the
following system
of forces
which
pass
through
the
origin.
The values
of F
are
the
magnitudes
of the
forces and
the values
of
x, y,
z
are
the coordinates
of
points
on the
action
lines of
the forces.
F
50
Ib.
90
Ib. 100 Ib.
60
Ib.
20 Ib.
x,
y,
z
5, 5,
7, 5,
3
3,
0,
4
2,
5,
4
0, 5,
3
Solution:
:50--^L+90.-?=+100-!-+60-
V50
A/83
5
:
35.4+69.2
+60+17.9+0
>
182Jib.
-50.-^+
9o.-L
V50
V83
=
35.4+49.3+0+44.7+17.1
=
146.5 Ib.
-
V34
V45
V45
*N/34
=
0+29.6+80+35.8+10.3
=
155.7
Ib.

56
RESULTANTS
OF
FORCE
SYSTEMS
>
2
+(146.5)
2
+(155.7)
2
=
281
?x
=
cos-
1
^||^
=
cos-
1
.650
=
49 30'.
J
v
=
cos
-
1
i||p
=
cos
-
1
.521
=
58 35'.
?
z
=
cos-
1
^fp^cos-
1
.553
=
56
25'.
PROBLEMS
In the
following
problems
the forces are
concurrent
at
the
origin.
It is
'equired
to find the resultants of the
systems.
43.
F
10
lb.
20
lb. 15
lb.
x,
y,
z
1, 2,
1
2,
2,
3
3,
1,
2
Ans,
72
=
43.1
lb.
0^
=
53
10'.
0^
=
59 30'.
02
=
51
50'.
44.
F
100 lb. 150
lb. 50
lb. 200
lb.
x,
y,
z
2,
2,
1
3, 2,
-2
-4, -3,
-5
3, -2,
4
Ans.
72
=
273 lb.
0^
=
18 10'.
0^=80
45'
2
=
7420'.
46.
F
10
lb.
30
lb.
20
lb.
x,
y,
z
1,
2,
1
1,
4,
2
2,
3,
3
6. PARALLEL
FORCES IN SPACE
33.
Graphical
Method.
The resultant of a
system
of
non-
coplanar,
parallel
forces
(see
Fig.
57)
is
either
a
force or a
couple.
If the
resultant
is a force the
action
line is
parallel
to the
forces
of
the
system,
its
magnitude
and sense
being given by
the
algebraic
sum
of
the forces.
The resultant force
may
be found
graphically
by
a
repeated
use
of the first
method
described in Art. 24
for
finding
the
resultant
of two
parallel
forces.
Since
this
method of solu-
tion,
however,
involves constructions in
various
planes
it is
not
convenient
to
use. The resultant force
may
also be found
by
the
construction
of
a
force and a funicular
polygon
as described in
Art.
24.
In
using
this method
the
forces are
projected
on
two of
the
coordinate
planes
and
the
resultant of each of the
two
systems
of
projected
forces
is found. The resultant
of the
given
force
system
is a force
the
projections
of
which
on the two coordinate
planes
are the
two
resultants of the
projected
forces.
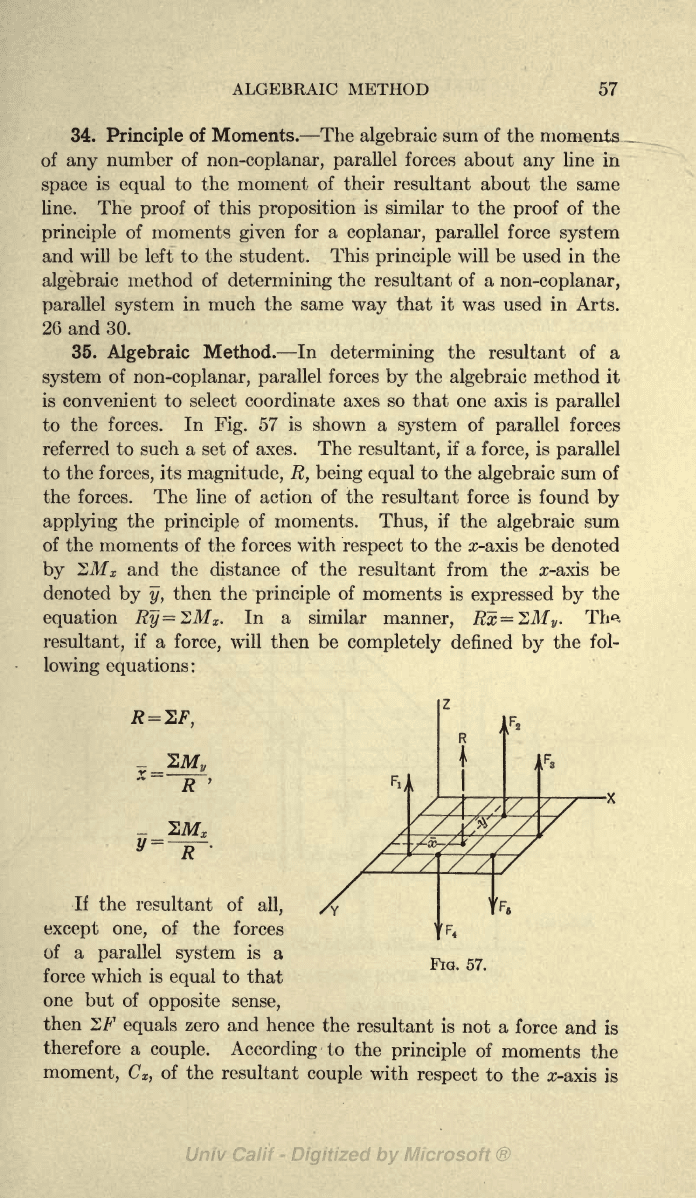
ALGEBRAIC
METHOD
57
34.
Principle
of
Moments.
The
algebraic
sum of
the
moments
of
any
number
of
non-coplanar,
parallel
forces
about
any
line
in
space
is
equal
to the moment
of their
resultant about
the same
line. The
proof
of this
proposition
is similar
to
the
proof
of the
principle
of
moments
given
for
a
coplanar,
parallel
force
system
and will be left to the
student. This
principle
will be used in
the
algebraic
method of
determining
the resultant
of
a
non-coplanar,
parallel
system
in much
the same
way
that
it
was used in
Arts.
26
and 30.
35.
Algebraic
Method.
In
determining
the resultant
of
a
system
of
non-coplanar, parallel
forces
by
the
algebraic
method
it
is
convenient
to select coordinate axes so that
one axis is
parallel
to the forces.
In
Fig.
57
is shown a
system
of
parallel
forces
referred
to
such a set of axes.
The
resultant,
if
a
force,
is
parallel
to
the
forces,
its
magnitude, R, being
equal
to the
algebraic
sum of
the forces. The line of action of the
resultant force is
found
by
applying
the
principle
of moments.
Thus,
if
the
algebraic
sum
of
the moments of the forces with
respect
to the
x-axis be denoted
by
1iM
x
and the
distance
of the
resultant
from the
z-axis be
denoted
by
y,
then
the
principle
of
moments
is
expressed
by
the
equation
Ry
=
^M
x
.
In
a similar
manner,
Rx=2M
y
.
Th*
resultant,
if
a
force,
will
then be
completely
denned
by
the
fol-
lowing equations:
R
y=
R
FIG.
57.
If
the
resultant of
all,
except one,
of
the
forces
of a
parallel
system
is
a
force
which
is
equal
to
that
one
but of
opposite
sense,
then
ZF
equals
zero
and
hence
the
resultant is
not a
force and
is
therefore
a
couple.
According
to
the
principle
of
moments
the
moment,
C
Xj
of
the
resultant
couple
with
respect
to
the
x-axis is
58
RESULTANTS
OF FORCE
SYSTEMS
equal
to the
algebraic
sum of the
moments
of the
forces with
respect
to the
:c-axis,
that
is,
Similarly,
Thus,
the resultant is a
couple
which lies in a
plane parallel
to
the
z-axis,
the moments of
which,
with
respect
to
the x- and
j/-axes,
are
C
z
and
C
v
as
expressed
above.
Methods of
expressing
a resultant
couple
in terms
of
its
component
couples
are discussed
in
Art. 36.
ILLUSTRATIVE PROBLEM
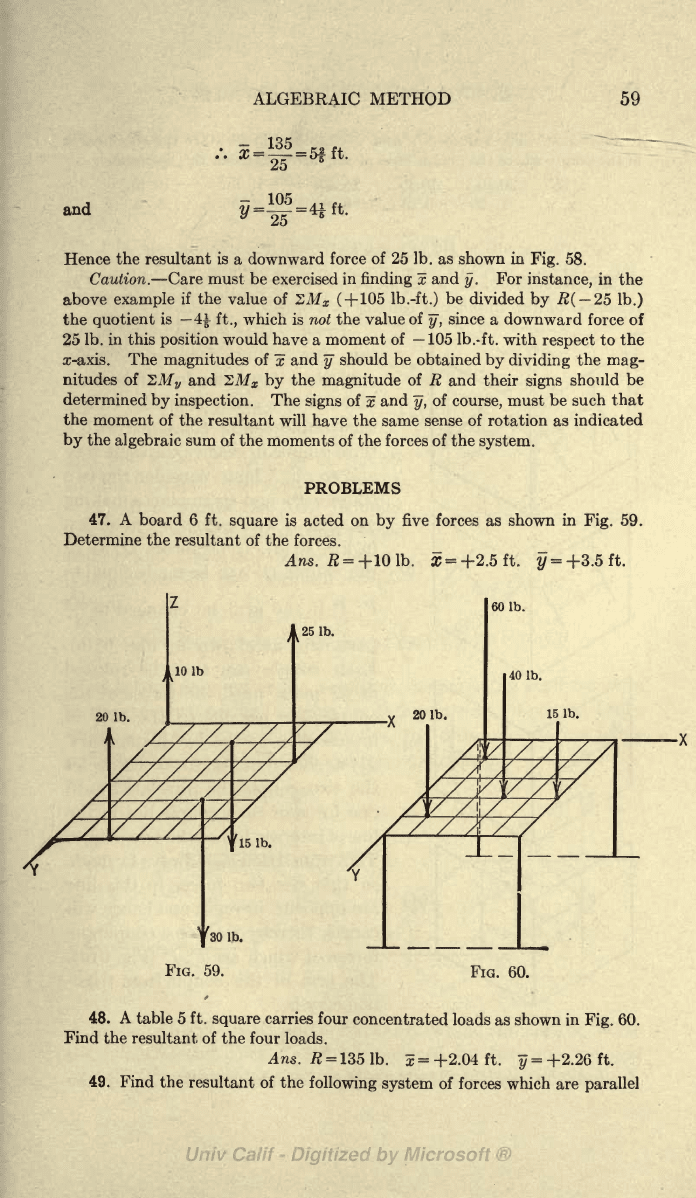
46. Find
the resultant of the
system
of
parallel
forces as shown in
Fig.
58.
Each
space
in the
figure represents
1
ft.
.10 Ib.
tz
15
Ib.
// s
/////*
20
/
i
l
=251b.
FIG. 58.
30
Ib.
Solution
:
-20-30
=
-25
Ib.
SMs=20X2+30X3-10Xl-15Xl.
=
1051b.-ft.
ZM
V
=
10X1+15X3-20X2-30X5.
=
-135
Ib.-ft.
ALGEBRAIC
METHOD
59
and
Hence
the resultant
is
a
downward
force
of 25
Ib. as
shown
in
Fig.
58.
Caution.
Care
must be
exercised in
finding
x
and
y.
For
instance,
in
the
above
example
if the
value of
?M
X
(+105 Ib.-ft.)
be
divided
by
R(
25
Ib.)
the
quotient
is
4
ft.,
which is
not the value
of
y,
since
a
downward force of
25
Ib.
in this
position
would have a
moment of 105
Ib.-ft.
with
respect
to
the
x-axis.
The
magnitudes
of
~x
and
y
should
be
obtained
by
dividing
the
mag-
nitudes of
2M
V
and
*2M
X
by
the
magnitude
of R
and their
signs
should
be
determined
by inspection.
The
signs
of
x
and
y,
of
course,
must be such
that
the
moment of the
resultant will
have
the
same
sense of
rotation as
indicated
by
the
algebraic
sum of
the moments of
the
forces of the
system.
PROBLEMS
47.
A
board 6
ft.
square
is acted on
by
five
forces
as shown
in
Fig.
59.
Determine the
resultant
of
the
forces.
Ans.
#=+101b.
3=
+2.5
ft.
?/
=
+3.5
ft.
20
Ib.
60
Ib.
10
Ib
25
Ib.
15
Ib.
r
30
Ib.
FIG. 59.
FIG.
60.
48. A
table 5
ft.
square
carries
four
concentrated
loads
as
shown in
Fig.
60.
Find the
resultant of the
four
loads.
Ans. #
=
135
Ib.
x
=
+2.04
ft.
=+2.26
ft.
49. Find
the
resultant
of the
following
system
of
forces
which are
parallel
60
RESULTANTS
OP FORCE
SYSTEMS
to the
z-axis.
The
values
of
x and
y,
expressed
in
feet,
are
the
coordinates
of
the
points
where
the
action
lines of the forces intersect the
xy-pl&ne.
F 20
Ib.
10
Ib.
25
Ib.
-15 Ib. -10
Ib.
x, y
1,
3
3,
2
6,
6
2,
5
6,
4
7.
COUPLES
IN
SPACE
36. Resultant of a
System
of
Couples. Proposition.
The
resultant
of
any
number
of
couples
is
a
couple.
Proof.
It is
sufficient to
prove
this
proposition
for two
couples,
only,
since if
two
couples
can
be
com-
bined into a
single
resultant
couple
this
couple
can
be
combined with
a
third
couple
in
exactly
the same
way
and
so on.
Thus,
consider the
two
couples
Pp
and
Qq
in
planes
making
an
angle
a
with
each other
as
shown
in
Fig.
61
(a).
The forces
Q,
Q
of
the
couple
Qq
can
be made
equal
to
P,
P
if
the
arm
be
changed
to
-p-
(Art.
27)
as
shown in
Fig.
61(6).
Each
couple
can
then
be
rotated
in
its
plane
until
the forces
of the
couples
are
parallel
to the
line
of
intersection of
the
two
planes
(Art.
27)
as shown in
Fig.
61(c).
Now
let
the two
couples
be translated
until
one force of
each
couple
lies
in the
line of
intersection of
the two
planes.
This
translation
can
always
be made
so that
the two
forces
in this
line
are
opposite
in sense and hence will
cancel, thereby leaving
a
couple
the
forces of
which are
P,
P
(Fig.
6
Id).
The arm
of this
couple
from
trigo-
nometry
is,
FIG.
61.
Special
Cases. I. If
the
angle
a
equals
90,
that
is,
if the
(d)
