Qiu X.G. (Ed.) High Temperature Superconductors
Подождите немного. Документ загружается.

50 High-temperature superconductors
1
2
3
4
5
6
7
8
9
10
1
2
3
4
5
6
7
8
9
20
1
2
3
4
5
6
7
8
9
30
1
2
3
4
5
6
7
8
9
40
1
2
43X
© Woodhead Publishing Limited, 2011
Sr
2.5
Ca
11.5
Cu
24
O
41
, presented in the previous section, suggested moreover that the
electronic transport in La
2 – x
Sr
x
CuO
4
, at temperatures T < T*, has a 1D character.
The idea of a close connection between the microscopic dynamic 1D charge ordering
and the non-Fermi liquid scattering mechanism, acting in high-T
c
compounds in the
pseudogap regime, therefore becomes very promising. The sensitivity of the charge
transport to the expulsion of charge carriers from the surrounding antiferromagnetic
regions into 1D stripes, static or dynamic, is very instructive.
In view of the interpretation of the different regions in the (T,x) phase diagram,
it is important to discuss the evolution of the charge ordering as a function of
doping. Neutron diffraction measurements on La
2 – x
Sr
x
CuO
4
(Yamada et al. 1997)
showed that the packing of the charge stripes becomes denser with increasing
charge carrier concentration (max. around x ≈ 0.125), essentially keeping the same
doping level within a stripe.
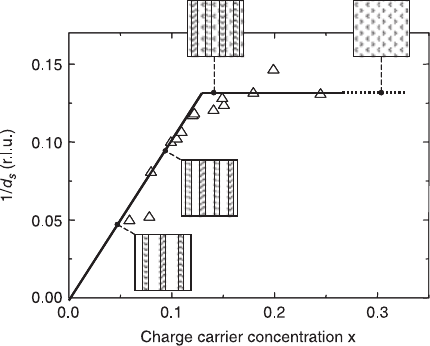
Figure 2.8 shows the inverse inter-stripe distance 1/d
s
as a function of the charge
carrier concentration x, reproduced from (Yamada et al. 1997). Frames, which give
a schematic view of the configuration of the charge stripes in the CuO
2
planes, are
added to the figure at selected x-values. The inverse inter-stripe distance 1/d
s
becomes noticeable beyond x = 0.05, increases approximately linearly with the
Sr-content and starts to saturate around x = 0.125 (= 1/8), where the distance
between the stripes equals 4a, with a the crystallographic lattice parameter (see also
Fig 2.6). In the overdoped region, the inter-stripe distance is almost constant upon
doping (Yamada et al. 1997): the holes enter the antiferromagnetic regions between
the stripes and the doping contrast of the stripes with respect to the surrounding
space between them decreases. It is, however, important to stress that Yamada et al.
2.8 The reverse inter-stripe distance 1/d
s
is shown as a function of the
charge carrier concentration x (data points from Yamada et al. (1997)).
The frames give a schematic view of the stripe configurations in the
CuO
2
plane at selected x-values. The lines are guides to the eye.
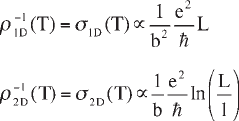
Transport properties of high-T
c
cuprate thin films 51
1
2
3
4
5
6
7
8
9
10
1
2
3
4
5
6
7
8
9
20
1
2
3
4
5
6
7
8
9
30
1
2
3
4
5
6
7
8
9
40
1
2
43X
© Woodhead Publishing Limited, 2011
observed well-defined incommensurate spin fluctuations up to the highest Sr-content
x = 0.25, investigated in their study. Recent (FT-)STM (Hoffman et al. 2002,
Howald et al. 2003, Kohsahak et al. 2007, Wise et al. 2008) and neutron scattering
(Tranquada et al. 1995) studies have convincingly demonstrated the existence of
stripes in high-T
c
cuprates, especially in the underdoped region. This effective
reduction of the dimensionality from 2D to 1D should be definitely taken into
consideration in the analysis of transport properties of high-T
c
cuprates.
A model for quantum transport in doped 1D and 2D Heisenberg systems
In the strongly anisotropic high-T
c
cuprates, the role of the CuO
2
planes is crucial.
The confinement of the charge carriers to the CuO
2
planes reduces the
dimensionality for charge transport to 2D or even to 1D, if stripes are formed. The
similarities between high-T
c
s and ladder cuprates underline the extreme
importance of the microscopic magnetic structure of the Cu
2+
spins. Any model
for high-T
c
superconductivity, attempting to explain the unusual non-Fermi liquid
behavior of the normal state, should therefore account for both the magnetic
structure and the dimensionality. We will present here a model (Moshchalkov
1993, Moshchalkov et al. 1999, 2001) based on three assumptions:
1 The dominant scattering mechanism in high-T
c
superconductors, in the whole
temperature range, is of magnetic origin.
2 The specific temperature dependence of the resistivity
ρ
(T) can be described
by the inverse quantum conductivity
σ
–1
with the inelastic scattering length L
fully controlled by the magnetic correlation length
ξ
m
.
3 The proper 1D or 2D expressions, depending on the effective dimensionality,
should be used for calculating the quantum conductivity.
The quantum conductivity
σ
1D
of a single 1D wire is a linear function of the
inelastic scattering length L whereas the 2D quantum conductivity
σ
2D
is
proportional to ln(L) (Abrikosov 1988). They are represented by:
[2.4]
[2.5]
with b the diameter of the 1D wire or the thickness of the 2D layer and l the elastic
scattering length. These expressions for the resistivity of 1D wires and 2D layers
can be used to calculate
ρ
(T) by inserting the magnetic correlation length
ξ
m
,
which is schematically presented in Fig 2.9, in the place of the inelastic scattering
length L. Hence, the determination of the precise behavior of the resistivity
requires the knowledge of the magnetic correlation lengths in the 1D (
ξ
m1D
) and
the 2D (
ξ
m2D
) case.
52 High-temperature superconductors
1
2
3
4
5
6
7
8
9
10
1
2
3
4
5
6
7
8
9
20
1
2
3
4
5
6
7
8
9
30
1
2
3
4
5
6
7
8
9
40
1
2
43X
© Woodhead Publishing Limited, 2011
Following the striking similarities between the
ρ
(T) curves of pseudo-gapped
high-T
c
cuprates and spin ladders, the spin-correlation length for even-chain
Heisenberg antiferromagnetic spin ladders, found by Monte Carlo simulations
(Greven et al. 1996), is chosen as
ξ
m1D
(Moshchalkov et al. 1999, 2001):
[2.6]
where A ≈ 1.7 and ∆ is the spin gap. The correlation length
ξ
m1D
, given by
expression [2.6] is derived for undoped ladders. It is assumed in (Moshchalkov
et al. 1999) that it can still be applied for weakly doped ladders as well.
In the framework of the 2D Heisenberg model, which is certainly applicable
for the doped CuO
2
planes without any stripes present, the temperature dependence
of the correlation length
ξ
m2D
is expressed as (Hasenfratz and Niedermayer 1991):
[2.7]
with c the spin velocity and F a parameter that can be directly related to the
exchange interaction J in accordance with 2
π
F = J. Equation [2.7] has been derived
for undoped 2D Heisenberg systems but numerical Monte Carlo simulations
(Reefman 1993) demonstrated its validity also for weakly doped systems.
The combination of the expressions for the 1D and 2D spin correlation length
with the proper expressions for the quantum resistivity gives the temperature
dependence of the resistivity. The 1D spin ladder resistivity can be described by
equation 1.8, with J
||
the spin-coupling along the chains and a the spacing between
2.9 Schematic representation of the 2D magnetic correlation length
ξ
m2D
.
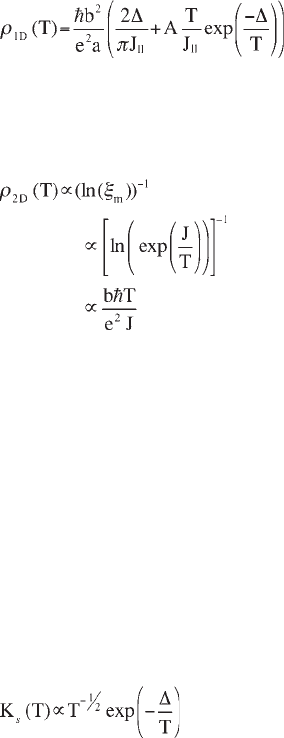
Transport properties of high-T
c
cuprate thin films 53
1
2
3
4
5
6
7
8
9
10
1
2
3
4
5
6
7
8
9
20
1
2
3
4
5
6
7
8
9
30
1
2
3
4
5
6
7
8
9
40
1
2
43X
© Woodhead Publishing Limited, 2011
the conducting ladders (J
||
comes in, to recalculate the theorist units) (Moshchalkov
et al. 1999, 2001):
[2.8]
In the 2D Heisenberg regime, remarkably, the resistivity is a linear function of
the temperature (Moshchalkov et al. 1999, 2001) due to the mutual cancellation
of the logarithmic
ρ
(
ξ
m
) and the exponential
ξ
m
(T) dependencies in the limit
T << 2 J (J is typically 1600 K):
[2.9]
The validity of the proposed 1D quantum conductivity model has been extensively
tested. The model is, for example, perfectly applicable for the ladder compound
Sr
2.5
Ca
11.5
Cu
24
O
41
, earlier mentioned. A fit of the temperature dependent resistivity
ρ
(T) of this compound with expression [2.8] does not only demonstrate an excellent
fit quality over a wide temperature range (25 K < T < 300 K), but it also offers a
reasonable value for the spin gap ∆ ~ (216 ± 20) K (at 8 GPa) (Trappeniers 2000).
Inelastic neutron scattering experiments (Takano et al. 1997) determined a gap
∆ ~ 320 K for the undoped spin ladder SrCu
2
O
3
. Since the spin gap is expected to
be reduced upon doping, both results are in fair agreement. Moreover, the ideas
brought up by the model, lead to an excellent description of the temperature
dependent Knight shift K
s
(T) of the superconducting YBa
2
Cu
3
O
8
system. Troyer
et al. (Troyer et al. 1994) calculated that the Knight shift of a double leg
spin-ladder (undoped) should obey the expression:
[2.10]
Fitting the K
s
(T) data of YBa
2
Cu
3
O
8
(Bucher et al. 1994) with this expression
leads to a spin-gap ∆ = (222 ± 20) K. Note that this value is in an excellent
agreement with ∆ = (224 ± 10) K derived from the resistivity measurements on the
same compound using the quantum conductivity model (Moshchalkov et al. 1999).
Turning back to the La
2 – x
Sr
x
CuO
4
system, we can compare the NMR knight
shift fit using equation [2.10] with the resistivity fit using equation [2.8]. If we fit
the Knight shift above T
c
(see Fig. 2.10) with expression [2.10] for the temperature
dependent K
s
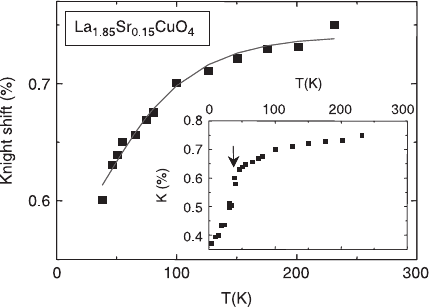
(T), a gap ∆ = (131 ± 20) K is obtained. This value agrees very well
with the pseudogap ∆ = (136 ± 20) K obtained by fitting the resistivity data for the
La
1.85
Sr
0.15
CuO
4
thin film.
54 High-temperature superconductors
1
2
3
4
5
6
7
8
9
10
1
2
3
4
5
6
7
8
9
20
1
2
3
4
5
6
7
8
9
30
1
2
3
4
5
6
7
8
9
40
1
2
43X
© Woodhead Publishing Limited, 2011
It should, however, be mentioned that experimental techniques probing charge
excitations (like ARPES and tunneling experiments) give pseudogap values ∆
p
that are higher (about a factor 2) than the spin excitation gap ∆
S
as observed in
NMR knight shift experiments. Since it is assumed in the 1D quantum transport
model, that the magnetic correlation length dominates the inelastic length, the
agreement of our data with the gap-values determined from the NMR experiments
seems to be natural.
In summary of this section we can state that:
• At T > T*, the resistivity reveals a linear temperature dependence (region I).
This regime is well described by equation [2.9] for quantum transport in a 2D
Heisenberg system with the inelastic length determined by the magnetic (2D)
correlation length.
• At T < T*, where charge stripes can develop, the resistivity is observed to
have a superlinear temperature dependence (region II). This regime can then
be accurately described by equation [2.8], which represents the temperature
dependence of the quantum resistivity in a 1D striped material with the
inelastic length again determined by the magnetic (1D) correlation length.
2.3 Magnetoresistivity in superconducting
La
2 – x
Sr
x
CuO
4
thin films
In what follows, two phenomena are introduced which are crucial for understanding
of the physics behind the magnetoresistivity of the cuprates. First, the main ideas
2.10 The inset depicts the temperature dependence of the Knight shift
of
63
Cu for La
1.85
Sr
0.15
CuO
4
(Ishida et al. 1991). In the main frame, the
normal state Knight shift is shown in more detail, together with a fit with
the expression K(T) = K(0) + K
1D
T
–1/2
exp (
∆
/T). The fitting parameters are
K(0) = (0.60 ± 0.0)%, K
1D
= (3.9 ± 0.2) % and
∆
= (131 ± 20) K.
Transport properties of high-T
c
cuprate thin films 55
1
2
3
4
5
6
7
8
9
10
1
2
3
4
5
6
7
8
9
20
1
2
3
4
5
6
7
8
9
30
1
2
3
4
5
6
7
8
9
40
1
2
43X
© Woodhead Publishing Limited, 2011
behind the Kohler plot are introduced and secondly, there is a description of the
superconducting fluctuations. Both effects influence the
ρ
(H) behavior, and it is
sometimes quite hard to make a clear difference between these effects.
Nevertheless, in what follows we will develop a method which shows the
separation between these effects and a detailed study of the fluctuation behavior.
2.3.1 Kohler’s rule
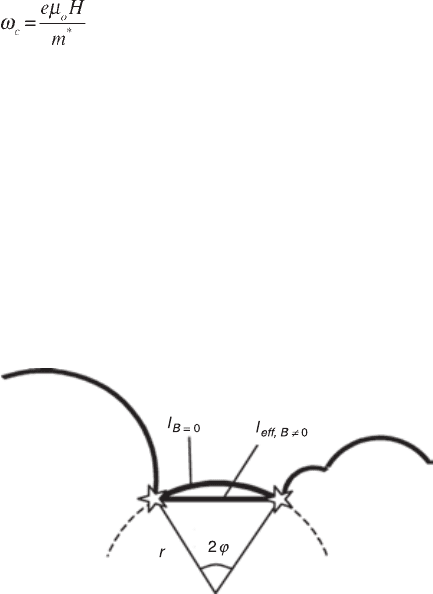
When a metal is placed in a magnetic field H, its resistance usually increases. For
low magnetic fields, it is easy to see that the resistance will depend quadratically
on H. The orbit of an electron in a uniform magnetic field is a spiral whose
projection on a plane perpendicular to the field is a circle (of radius r). The angular
frequency
ω
c
(cyclotron frequency) is determined by the condition that the
centrifugal acceleration r
ω
2
c
is provided by the Lorentz force e
ω
c
r
µ
o
H:
[2.11]
(m* is the effective mass of the charge carriers). The curving of the electron
trajectories in a magnetic field causes the effective mean free path l to decrease,
as visualized in Fig. 2.11. The difference in length between the arc and the chord
is equal to 2r
ϕ
– 2rsin
ϕ
≈ ¹/
³
r
ϕ
3
, where 2
ϕ
is the angular size of the arc. If we
assume that r
ϕ
~ l, then the difference in length is of the order of l(l/r)
2
, i.e., the
relative order of the correction to the resistance is:
∆
ρ
∝
ρ
(l/r)
2
[2.12]
Since l ~
ντ
, with
ν
the electron velocity and
τ
the scattering time, we find that:
∆
ρ
~
ρ
(
ω
c
τ
)
2
[2.13]
2.11 Schematic representation of the decrease of the effective mean
free path due to an applied magnetic field; r is the radius of the curved
electron trajectory and 2
ϕ
is the angular size of the arc defined by two
collisions (stars). The mean free paths at zero and non-zero magnetic
field are indicated.
56 High-temperature superconductors
1
2
3
4
5
6
7
8
9
10
1
2
3
4
5
6
7
8
9
20
1
2
3
4
5
6
7
8
9
30
1
2
3
4
5
6
7
8
9
40
1
2
43X
© Woodhead Publishing Limited, 2011
leading to the expected quadratic variation of the resistivity with respect to an
applied magnetic field.
Exact calculations of the magnetoresistivity are very complicated. Due to the
assumption that every electron has the same thermal speed, the simple Drude
model leads to a resistivity that is independent of the magnetic field. The Boltzmann
transport equation, on the other hand, departs from a distribution of electron
velocities. Solving this equation is, however, far from evident. In low magnetic
fields (
ω
c
τ
< 1), the Boltzmann equation leads to the usual quadratic field
dependence of the resistivity (Ziman 1964). In the intermediate field region
(
ω
c
τ
~ 1), few (if any) theories of magnetoresistivity exist. In high fields (
ω
c
τ
>> 1),
the electrons are able to complete many cyclotron orbits between collisions. In this
case, it is the topology of the Fermi surface and not the details of the scattering
mechanisms that dominates the character of the magnetoresistance. Solving the
Boltzmann transport equation, it can be found that the transverse magnetoresistance
tends to saturate in high fields, or increases as H
2
, depending on whether the charge
carriers are situated on closed or open orbits (Ziman 1964, Abrikosov 1988).
To overcome the problems in describing the magnetoresistance of metals in the
different field regimes, one often uses the so-called Kohler’s rule (Kohler 1938,
Abrikosov 1988). This rule, based on the Boltzmann equation, departs from the
idea of a universal mean free path l. The resistance must be inversely proportional
to this path length. As the magnetic field increases, the role of the mean free path
is gradually taken over by the radius r ~ v/
ω
c
. It may therefore be assumed that
ρ
(H,T)/
ρ
(0,T) depends only on the ratio l/r. But, since r ~ H
–1
and l ~ [
ρ
(0,T)]
–1
,
we may consider
ρ
(H,T)/
ρ
(0,T) to be dependent only on the combination H/
ρ
(0,T).
Subtracting unity from
ρ
(H,T)/
ρ
(0,T), we get (Kohler 1938):
[2.14]
where f is a function that is independent of the temperature. This is what is called
Kohler’s rule. In the case that the magnetoresistance depends on the quadratic on
the field, data are often presented in a ‘Kohler plot’:
[2.15]
Obviously, this rule is only an approximation. For example, deviations may occur
in anisotropic metals in the presence of open orbits, or in metals on which the field
has other effects besides the curving of the electron trajectories.
2.3.2 Superconducting fluctuations
Within the phenomenological Ginzburg-Landau (GL) model, a superconducting
material is determined by the lowest energy eigenfunction
ψ
of the GL equation.
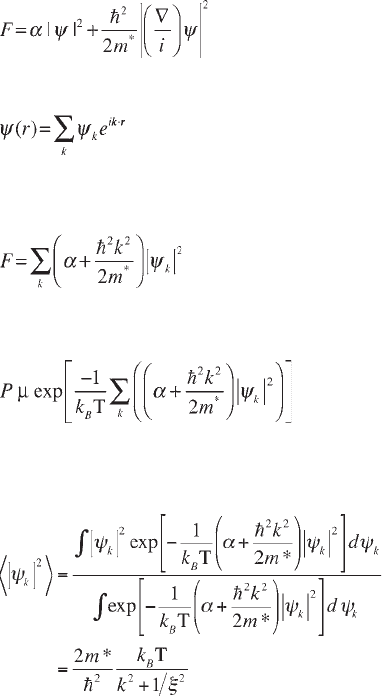
Transport properties of high-T
c
cuprate thin films 57
1
2
3
4
5
6
7
8
9
10
1
2
3
4
5
6
7
8
9
20
1
2
3
4
5
6
7
8
9
30
1
2
3
4
5
6
7
8
9
40
1
2
43X
© Woodhead Publishing Limited, 2011
While this is indeed the most probable state, the presence of thermal energy k
B
T
implies that the system will fluctuate into other low-lying excited states with a
finite probability. Above T
c
, the free energy F(
ψ
) is at its minimum when |
ψ
| = 0.
There is however a probability exp[ – F(
ψ
)/k
B
T] that thermal fluctuations drive
|
ψ
| from 0 to a finite value |
ψ
| > 0. These superconducting fluctuations |
ψ
| > 0
above T
c
(
α
> 0) can be described in the framework of the Ginzburg-Landau
formalism (Tinkham 1996). We will consider the case far enough above T
c
so that
the effects of the quartic term in the free energy can be neglected. In the absence
of any field (A = 0), the Ginzburg-Landau free energy, relative to the normal state,
is given by:
[2.16]
We can expand
ψ
(r) in Fourier series, so that:
[2.17]
Inserting this into [2.16] and using the orthogonality of the terms in a Fourier
series, we find:
[2.18]
This immediately gives us the probability P that the fluctuations realize a particular
configuration
ψ
(r). It is proportional to:
[2.19]
The average contribution 〈|
ψ
k
|
2
〉 of each mode to the free energy can be calculated
with standard Boltzmann statistical mechanics:
[2.20]
with (2m
α
/ħ
2
)
1/2
= 1/
ξ
, the inverse coherence length. Please notice that expression
[2.20] defines the spatial variation of the fluctuations. We see that Fourier
components with k > 1/
ξ
come in with reduced weight. In other words, the
fluctuations occur on a scale larger than
ξ
.
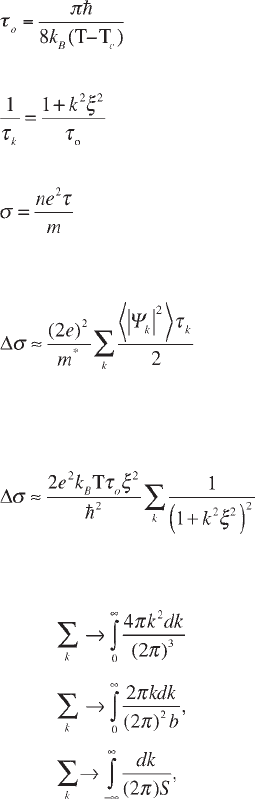
58 High-temperature superconductors
1
2
3
4
5
6
7
8
9
10
1
2
3
4
5
6
7
8
9
20
1
2
3
4
5
6
7
8
9
30
1
2
3
4
5
6
7
8
9
40
1
2
43X
© Woodhead Publishing Limited, 2011
Of course, if we want to describe a property such as electrical conductivity, we
also need a model of the time dependence since the contribution of a given fluctuation
to the conductivity above T
c
is proportional to its lifetime. Such a model is provided
by the linearized time-dependent Ginzburg-Landau equation. In this case, the
parameter
τ
o
is the temperature-dependent relaxation time of the (k = 0) mode:
[2.21]
The higher-energy modes with k > 0 decay more rapidly with the relaxation rates:
[2.22]
In analogy with the Drude model:
[2.23]
which provides the simplest description of the normal conductivity, we might
expect the fluctuations to contribute an extra term:
[2.24]
It means that ordinary scattering processes are ineffective until a given fluctuation
relaxes. The factor ½ comes in, because |
ψ
k
|
2
relaxes twice as fast as
ψ
k
. Inserting
relations [2.20] and [2.22] into [2.24], we obtain:
[2.25]
The summation over k can be converted to an appropriate integration depending
on the dimensionality of the electron motion:
In 3D:
[2.26]
In 2D:
where b is the layer thickness [2.27]
In 1D:
where S is the cross-sectional area [2.28]
Following this prescription, we obtain the result, which differs from the exact
calculations only by small numerical factors. In particular, the temperature
dependence of ∆
σ
is correctly found to be (T – T
c
)
–(4 – d)/2
, where d (= 1, 2, 3) is
the dimensionality of the system. A more precise result was derived by Aslamazov
and Larkin (Aslamazov and Larkin 1968):
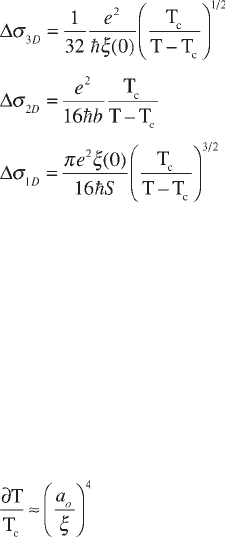
Transport properties of high-T
c
cuprate thin films 59
1
2
3
4
5
6
7
8
9
10
1
2
3
4
5
6
7
8
9
20
1
2
3
4
5
6
7
8
9
30
1
2
3
4
5
6
7
8
9
40
1
2
43X
© Woodhead Publishing Limited, 2011
[2.29]
[2.30]
[2.31]
These expressions, referred to as the paraconductive or Aslamazov-Larkin
contribution, describe the excess conductivity caused by non-equilibrium Cooper
pairs above T
c
. The critical exponents obtained in the framework of the theory of
Aslamasov and Larkin turn out to be very robust. Later revisions of the theory of
paraconductivity (Bulaevski et al. 1986, Narozhny 1994, Damianov and Mishonov
1997, Sadovski 1997, Larkin and Varlamov 2005) resulted in the same critical
exponents. Only the overall coefficients depend on the characteristics of the model.
Ginzburg (Ginzburg 1968, Abrikosov 1988, Levanyuk 1959) estimated the
temperature range where the fluctuations are relevant in a bulk isotropic
superconductor (3D):
[2.32]
where a
o
is the interatomic distance. In conventional superconductors, for which
this ratio takes values around 10
–12
–10
–14
, fluctuation phenomena are thus barely
visible. Ratio equation [2.32] is much larger for high-T
c
superconductors because
their coherence length is typically comparable to the atomic distance. Moreover,
Aslamasov and Larkin (Aslamasov and Larkin 1968) calculated that the exponent
v of the ratio (a
o
/
ξ
), which enters in [2.32], drastically decreases as the effective
dimensionality of the electron motion diminishes:
ν
= 4 for 3D, but
ν
= 1 for 2D.
The latter is a more appropriate choice with respect to the layered cuprates. We
conclude that fluctuation phenomena are prominently present in high-T
c
compounds because of their extreme anisotropy and short coherence length.
These fluctuations lead to visible precursor effects of the superconducting phase,
occurring while the system is still in the normal phase far from T
c
.
The Aslamazov-Larkin (AL) fluctuations described above and, caused by the
appearance of fluctuating Cooper pairs above T
c
, contribute directly to the
conductivity. Two other mechanisms are known which affect the conductivity in
an indirect way: the density of states (DOS) and the Maki-Thompson (MT)
contribution. The first indirect fluctuation correction follows from a decrease of
the one-electron density of states at the Fermi level. Indeed, if some electrons are
involved in pairing above T
c
, they cannot simultaneously participate in normal
state charge transfer as one-particle excitations. Since the total number of the
electronic states cannot be changed by the Cooper interaction, the energy is
